For students looking to enhance their understanding of complex subjects, having access to reliable solutions is essential. These resources provide clear explanations, helping learners grasp essential principles and apply them effectively. With the right guidance, even the most challenging problems can become more approachable.
By focusing on key topics, this section aims to offer structured support for learners working through assessments. It outlines how to use provided materials to identify correct methodologies and ensure a deeper comprehension of the material. Whether tackling calculations or conceptual questions, mastering the content becomes more achievable with systematic practice and thoughtful review.
Utilizing available references allows individuals to pinpoint areas for improvement, reinforcing confidence in their skills. Approaching each question with a clear strategy not only aids in obtaining correct results but also nurtures critical thinking and problem-solving abilities.
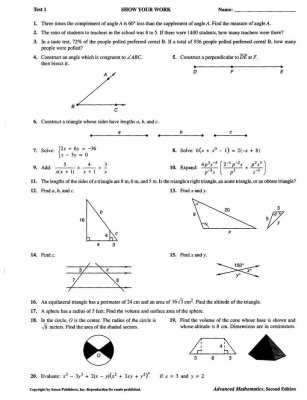
Complete Guide to Saxon Math Course 3 Test
Understanding how to approach and solve problems in structured assessments can greatly improve a learner’s ability to succeed. This guide provides a comprehensive overview of strategies and techniques to effectively navigate through the exercises presented in your educational materials. By breaking down the steps and understanding the underlying concepts, students can maximize their performance and build a solid foundation for future challenges.
Preparing for the Assessment

Preparation is key to achieving success. Start by reviewing the topics covered in the lessons and practicing various types of problems. Make sure to focus on areas that might present difficulties. Utilize practice resources that mirror the format and difficulty level of the evaluation, allowing for better familiarity with the types of questions expected. This proactive approach will increase confidence and reduce any anxiety associated with the test.
Effective Problem-Solving Techniques
When tackling problems, it’s important to break each question down into smaller, more manageable parts. Take your time to analyze what is being asked and plan out your steps before starting to solve. Always double-check your calculations, as small mistakes can lead to incorrect answers. Additionally, use the provided resources to understand the reasoning behind each solution, ensuring that you don’t just memorize answers, but truly comprehend the methods behind them.
Overview of Saxon Math Course 3
This section introduces the foundational structure and objectives of a structured learning program aimed at building essential skills. Designed for learners at a specific level, the curriculum focuses on reinforcing previously learned material while gradually introducing more complex concepts. The approach is designed to ensure steady progression, ensuring that students fully understand each step before moving on to the next.
Key Areas of Focus

The program covers a wide range of topics, each carefully designed to build upon prior knowledge. Key areas include:
- Basic arithmetic operations and their applications
- Introduction to more complex number concepts
- Developing problem-solving strategies
- Understanding geometric principles
- Building a strong foundation in fractions, decimals, and percentages
Learning Approach and Support
The learning approach emphasizes consistent practice, gradual skill-building, and regular assessments. Each lesson is designed to:
- Introduce new concepts in a manageable way
- Provide ample opportunities for practice and reinforcement
- Allow students to track their progress through evaluations
- Offer support materials to ensure comprehension of complex topics
Common Challenges in Saxon Math Course 3

Students often face several obstacles when working through structured learning materials. These difficulties can arise from the increasing complexity of the topics being studied or from a lack of understanding in certain foundational areas. Identifying and addressing these challenges early on is crucial for continued success and progress.
Some common hurdles include:
- Difficulty in understanding more advanced number operations and their applications
- Struggling with multi-step problem-solving approaches
- Challenges in applying concepts to real-world scenarios
- Confusion when transitioning from simple to complex mathematical principles
- Issues with retaining and recalling formulas and methods
Recognizing these common obstacles allows students to take proactive steps in overcoming them, ensuring that they can continue advancing through the material with confidence.
Step-by-Step Test Solution Breakdown
Breaking down problems into manageable steps is essential for understanding complex concepts. This approach helps to clarify each part of the problem, ensuring that the solution is both accurate and well-understood. By following a structured method, students can not only arrive at the correct answers but also strengthen their grasp of the underlying principles.
Understanding the Problem
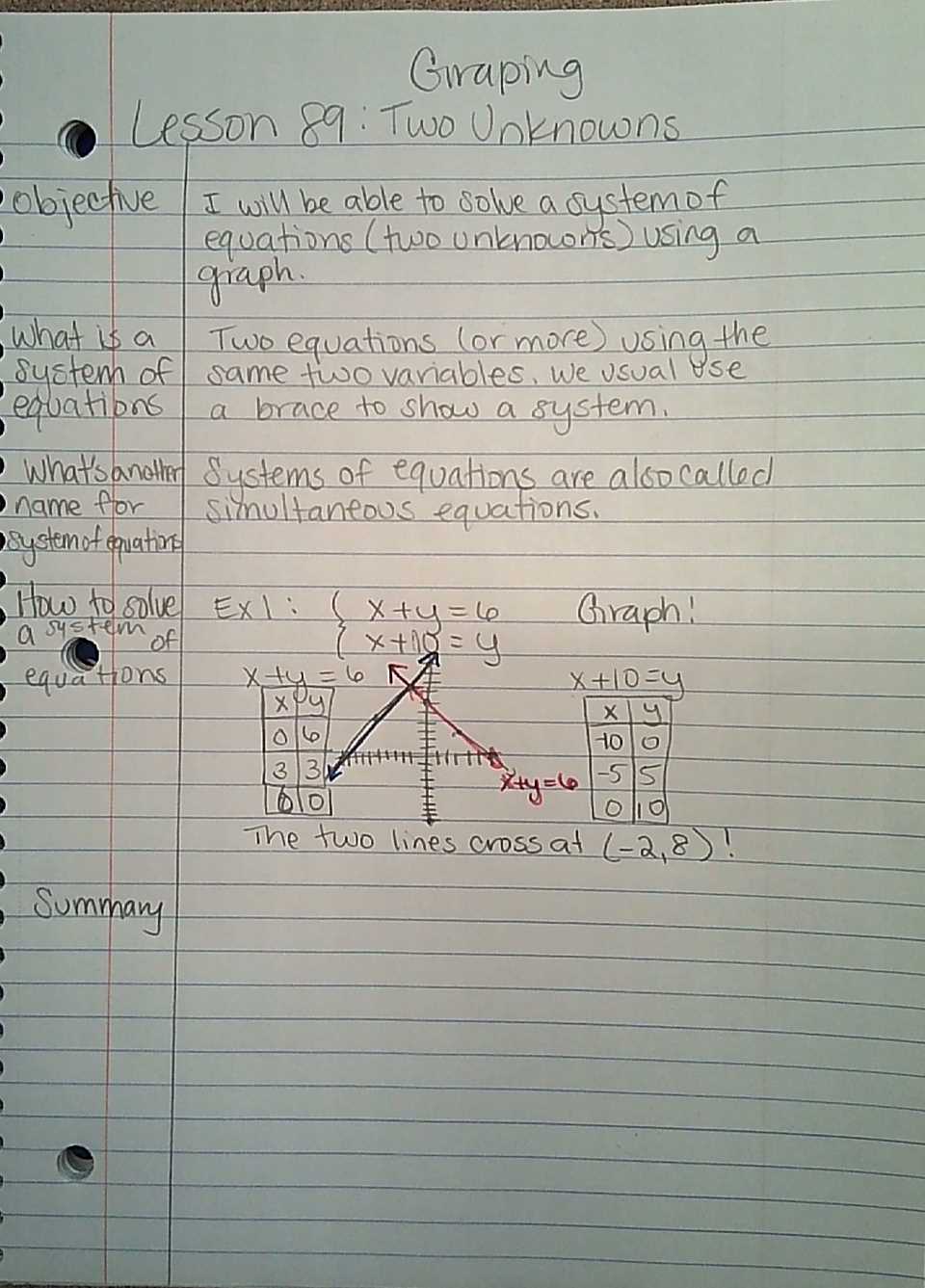
The first step in any problem is carefully reading and understanding what is being asked. Identify the key information provided and determine what needs to be solved. Highlight important values, units, or conditions to make the task clearer. This ensures that no critical details are overlooked before moving forward.
Breaking Down the Solution Process
Once the problem is understood, the next step is to break it down into smaller, logical parts. Begin by addressing one component at a time. Whether it’s simplifying an equation, performing a calculation, or applying a formula, tackling each step methodically ensures that you don’t miss any crucial elements. After completing each step, double-check your work to confirm accuracy before proceeding to the next stage.
Understanding Key Concepts in Math 3
Grasping essential principles is critical for success in any learning program. Understanding the core ideas behind each lesson ensures that students can apply what they’ve learned to solve problems effectively. This section highlights the most important concepts that form the foundation of the subject, helping learners navigate through the material with confidence.
Core Concepts to Master
Several key topics are central to building a strong understanding of the subject. These include:
- Operations with fractions and decimals
- Understanding and working with percentages
- Geometric shapes and their properties
- Solving word problems using logical reasoning
- Introduction to algebraic thinking and patterns
Applying Concepts in Practice
Mastering theoretical knowledge is only the first step. To truly understand these concepts, students must practice applying them in different contexts. This helps in reinforcing the learned material and developing problem-solving skills. Regular practice ensures retention and builds confidence in handling new challenges as they arise.
Tips for Mastering Saxon Math Problems
Becoming proficient in solving complex problems requires a combination of strategy, practice, and understanding key principles. By using effective techniques and developing a methodical approach, learners can improve their problem-solving abilities and tackle challenges with confidence. This section provides practical advice for mastering the material and succeeding in assessments.
Develop a Consistent Practice Routine
Consistency is crucial when it comes to mastering any skill. Set aside dedicated time each day to review lessons and practice solving problems. This will help reinforce what you’ve learned and increase retention over time. Regular practice also allows you to identify areas where you may need further clarification, ensuring that you stay on track and continue progressing.
Break Down Problems Step-by-Step
When approaching each problem, take your time to break it down into manageable parts. Focus on understanding the goal of the question and identifying the steps needed to reach the solution. Solve the problem one step at a time, double-checking your work at each stage to avoid mistakes. This methodical approach not only improves accuracy but also builds confidence in your ability to tackle complex problems.
How to Prepare for the Test
Proper preparation is the key to performing well on any evaluation. To achieve success, it’s important to review the material systematically, practice regularly, and address any areas of difficulty. By organizing your study time and focusing on both strengths and weaknesses, you can approach the assessment with confidence.
Review Key Concepts and Principles
Start by revisiting the fundamental topics covered in the lessons. Pay special attention to concepts that are frequently tested or seem challenging. Focus on understanding the reasoning behind each solution rather than just memorizing steps. Breaking down each concept into simpler parts can make complex ideas more digestible and easier to recall during the test.
Practice with Similar Problems
In addition to reviewing notes, practice solving problems similar to those that will appear on the evaluation. Working through a variety of practice questions helps to reinforce what you’ve learned and increases your familiarity with different problem types. Make sure to time yourself, as practicing under time constraints can simulate the actual testing environment and improve your time-management skills.
Importance of Practice in Saxon Math
Consistent practice is essential for mastering any subject. It helps reinforce the concepts learned, making them easier to recall and apply when needed. Without regular practice, even well-understood material can become difficult to retain. In this section, we explore why practice is a crucial component of success in any structured learning program.
Reinforcing Key Concepts
Frequent practice helps solidify the foundation of each topic. By revisiting problems and applying concepts multiple times, learners increase their confidence and ability to solve problems independently. The more familiar you become with different types of problems, the more easily you can identify patterns and strategies that lead to correct solutions.
Tracking Progress and Identifying Weaknesses
Regular practice also allows students to track their progress. As you continue working through problems, you’ll begin to notice areas of improvement as well as topics that may require further attention. This self-assessment is a vital tool in ensuring that no aspect of the material is overlooked.
| Practice Frequency | Effectiveness |
|---|---|
| Daily practice | Enhances long-term retention and fluency |
| Weekly review | Helps consolidate learned concepts and strategies |
| Random practice tests | Prepares for real-world problem-solving situations |
What to Expect on the Math Assessment
When preparing for an evaluation, it’s essential to understand what type of content will be covered and how the problems will be presented. Knowing what to expect can help reduce anxiety and ensure that you’re fully prepared to perform at your best. This section provides an overview of the common topics and structure of the evaluation, helping students understand the key areas to focus on.
| Topic | Type of Questions |
|---|---|
| Operations with fractions | Solving and simplifying problems with fractions, including addition, subtraction, multiplication, and division. |
| Decimals and percentages | Calculations involving decimals and converting between decimals, fractions, and percentages. |
| Word problems | Real-life scenarios requiring logical reasoning to solve, including multi-step problems. |
| Basic algebraic concepts | Solving simple equations, understanding variables, and applying basic algebraic operations. |
| Geometry | Questions related to shapes, their properties, and measurements such as area and perimeter. |
During the evaluation, problems will be designed to test both your conceptual understanding and ability to apply the learned principles to various situations. Expect to encounter a mixture of question types, from multiple-choice to open-ended problems that require clear and detailed solutions. Knowing the format and preparing accordingly can make the assessment process much more manageable.
Correct Answer Formats and Explanation
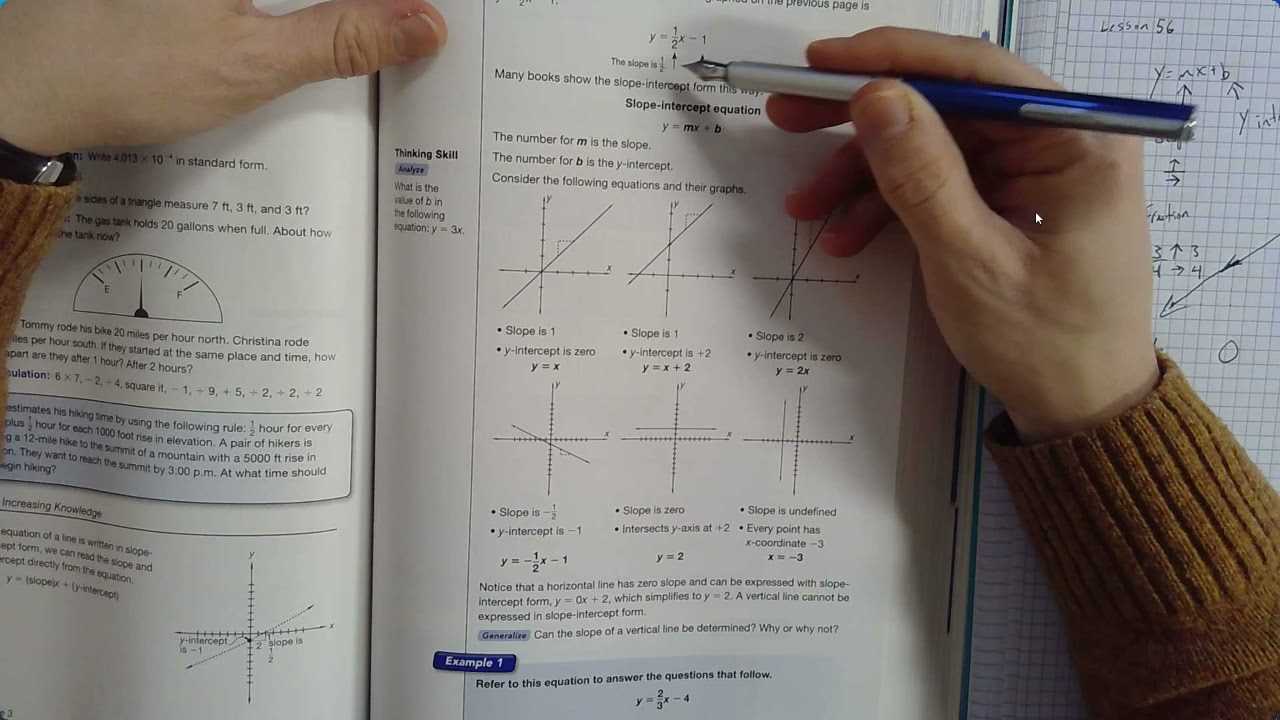
When approaching assessments, it’s important not only to find the correct solution but also to present it in a clear and structured manner. Understanding the expected format for answers ensures that your work is accurately evaluated and that you fully demonstrate your understanding. This section outlines how to format responses correctly and provides an explanation of common formats used in problem-solving exercises.
For most questions, the answer format should be straightforward and include all necessary steps or reasoning. Clarity and organization are key when presenting your solutions. Below are some common formats and examples:
- Numerical answers: If a question requires a specific number or value, write the final result clearly, ensuring that units are included if necessary.
- Step-by-step solutions: For problems requiring a process, break down each step and show the method used to arrive at the final answer. This demonstrates your understanding of the underlying concepts.
- Word problem solutions: For scenarios that require written explanations, structure your answer logically, explaining the steps taken to solve the problem and the reasoning behind your approach.
Example: For a question asking to solve for x in the equation 3x + 4 = 10, the answer should include both the steps taken to isolate x and the final value. The correct format would be:
- Step 1: Subtract 4 from both sides: 3x = 6
- Step 2: Divide both sides by 3: x = 2
In summary, correct answer formats not only help communicate your solution clearly but also showcase your logical approach to problem-solving. Proper presentation makes it easier for evaluators to follow your work and understand your process, leading to a more accurate assessment of your skills.
Common Mistakes and How to Avoid Them
When working through academic exercises, it’s easy to make errors, especially when under pressure or when the material seems complex. Recognizing these common mistakes and learning how to prevent them is a crucial step in mastering the content. In this section, we’ll explore some of the most frequent errors students encounter and offer strategies to help avoid them, ensuring a more accurate and efficient approach to problem-solving.
One of the most common mistakes is rushing through problems without properly understanding the question. Often, students may overlook key details or misinterpret instructions, leading to incorrect solutions. To avoid this, take time to read each question carefully, and ensure you understand exactly what is being asked before starting. This is especially important for word problems, where the phrasing can sometimes be tricky.
Another frequent error is failing to check the work. Many students may solve a problem correctly but forget to verify the result, leading to careless mistakes. Always double-check your calculations and reasoning to ensure accuracy. This extra step can help catch simple miscalculations that might otherwise go unnoticed.
Additionally, mixing up different mathematical operations or steps in the wrong order can lead to incorrect results. This often happens with more complex problems, such as equations involving multiple operations. To avoid this, it’s helpful to write out each step clearly and systematically, following the proper order of operations, such as PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction).
Finally, neglecting units of measurement can be another source of confusion, especially in problems involving dimensions, area, or volume. Always ensure that the correct units are included in your final answer, and that you are consistent in their usage throughout the process.
By being mindful of these common mistakes and actively working to avoid them, you can greatly improve your performance and ensure more accurate results in your work. Taking a methodical and careful approach will lead to greater confidence and mastery of the material.
Tracking Progress with Math Solution Guides

Monitoring progress while working through academic exercises is an essential part of the learning process. By regularly checking how well you are grasping the material, you can identify areas that need more focus and improve your overall understanding. Using solution guides can be a valuable tool for tracking your progress and ensuring that you are on the right track. This section outlines how to effectively use such resources to gauge your advancement and address any gaps in your knowledge.
How to Use Solution Guides for Effective Tracking

Solution guides offer the final steps and results for various problems, which can be used to compare your solutions with the correct ones. Here are some key ways to utilize these resources:
- Self-Assessment: After solving a problem, check your solution against the provided one. If your result differs, carefully review the steps you took to see where the mistake occurred.
- Identify Recurrent Issues: If you notice that the same types of errors occur frequently, it indicates that further study or practice is needed in that specific area.
- Confirm Understanding: Use the solution guide to verify that you understand the steps leading to the answer, not just the final result. This ensures that you are following the correct reasoning process.
Benefits of Tracking Progress

By consistently checking your progress, you can see how your skills develop over time. Tracking your results allows you to:
- Build confidence as you see improvement in your ability to solve problems.
- Identify specific areas where you may need additional practice or tutoring.
- Ensure that you are fully prepared for more complex challenges.
Incorporating solution guides into your study routine not only helps you stay on track but also promotes a deeper understanding of the material. By regularly reviewing your progress, you’ll be better equipped to tackle increasingly difficult problems with confidence.
Improving Test Scores with Solution Guides
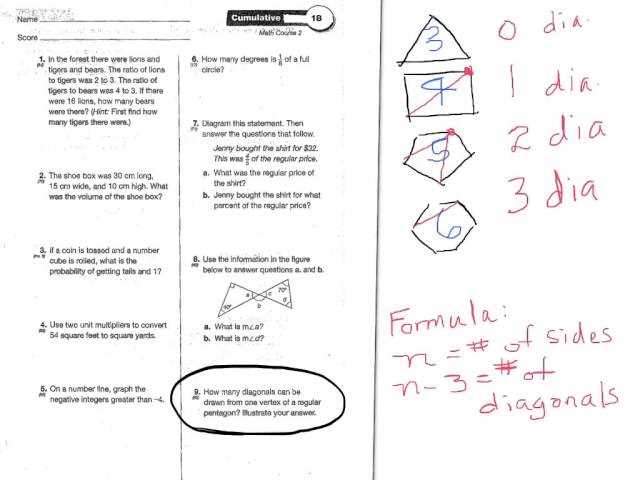
Achieving higher scores on assessments is often the goal of students, and effective preparation plays a crucial role in this process. One of the most valuable tools for improving performance is using solution guides. These resources provide detailed explanations of how to arrive at the correct answers, helping students not only check their results but also understand the reasoning behind them. This section will explore how utilizing solution guides can lead to better outcomes on assessments and enhance overall learning.
How Solution Guides Help Improve Scores
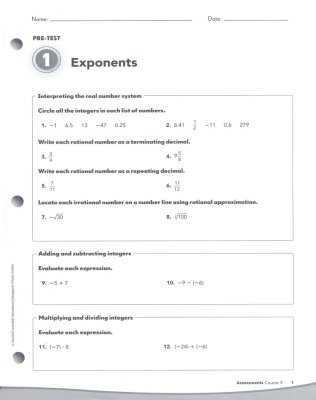
Solution guides offer more than just the final answers–they provide insight into the methodology and problem-solving techniques that lead to correct solutions. By reviewing the steps involved, students can identify areas for improvement and enhance their skills. Here’s how solution guides can be helpful:
- Clarify Mistakes: If a student’s solution differs from the one provided, comparing both steps helps to pinpoint where the misunderstanding occurred.
- Understand the Process: Understanding the process behind the solution is more important than memorizing steps. This ensures that students can tackle similar problems in the future.
- Reinforce Learning: Repeatedly working through problems with the guidance of the solution keys helps reinforce important concepts and techniques.
- Build Confidence: Being able to confirm that your solution matches the correct one builds confidence and reduces test anxiety.
Strategies for Using Solution Guides Effectively
To make the most of solution guides, students should approach them strategically. Here are some tips to maximize their usefulness:
- Work on Problems First: Before consulting the solution guide, attempt the problems independently. This allows you to identify gaps in your understanding.
- Analyze Step-by-Step: Don’t just look at the final answer–study the steps leading to it. This helps you understand the reasoning and apply similar strategies in the future.
- Review and Practice Regularly: Regular review of solved problems and the underlying concepts can help reinforce what you’ve learned and improve retention.
- Seek Help if Needed: If you’re still unsure about a particular step after reviewing the solution guide, don’t hesitate to ask for clarification from a teacher or tutor.
By incorporating solution guides into your study routine, you can improve your test scores while building a stronger understanding of the material. Consistent practice and reviewing problem-solving techniques will not only help in assessments but also foster long-term academic success.
Addressing Test Anxiety in Learning
Test anxiety is a common challenge faced by students, especially when it comes to subjects that involve problem-solving and logical reasoning. The pressure to perform well can lead to stress and hinder one’s ability to think clearly during assessments. Addressing test anxiety is crucial to achieving better academic results and fostering a positive learning experience. This section will explore strategies to manage and overcome test-related stress, promoting a calm and focused approach to problem-solving.
Understanding the Causes of Test Anxiety
Test anxiety can arise from a variety of factors, including fear of failure, past negative experiences, or pressure to meet high expectations. Recognizing the root causes of stress is the first step in managing it effectively. Common contributors include:
- Fear of making mistakes: Students often worry about incorrect answers, leading to self-doubt and increased stress levels.
- Pressure to perform: The desire to meet personal or external expectations can create unnecessary pressure, causing anxiety.
- Previous struggles: Past difficulties with problem-solving or poor performance on assessments can fuel anxiety and create a cycle of stress.
- Lack of preparation: Not feeling fully prepared for an assessment can trigger anxiety, leading to a sense of panic.
Effective Strategies for Managing Test Anxiety
By incorporating certain techniques into study habits, students can manage test anxiety and improve their overall performance. Here are some proven strategies:
- Practice relaxation techniques: Breathing exercises, meditation, and mindfulness can help calm the mind and reduce stress levels.
- Stay organized: Plan study sessions well in advance to avoid last-minute cramming, which can increase anxiety.
- Break tasks into smaller steps: Tackle problems one step at a time instead of feeling overwhelmed by the entire assessment.
- Focus on the process, not the outcome: Shift your mindset from worrying about results to focusing on solving problems methodically and logically.
- Get plenty of rest: Adequate sleep is essential for cognitive function, concentration, and stress management.
- Maintain a positive attitude: Encourage self-compassion and remind yourself that mistakes are a natural part of learning and growth.
By adopting these strategies, students can reduce test anxiety and approach assessments with a clearer, more focused mindset. Managing stress effectively leads to better performance, increased confidence, and a more enjoyable learning experience.
Supplementary Resources for Effective Learning
Incorporating additional resources can significantly enhance the learning process, providing extra support and reinforcing key concepts. Whether you are seeking visual aids, interactive tools, or extra practice, supplementary materials can be invaluable for mastering challenging topics. This section explores various resources that can help deepen understanding and improve skills in areas of difficulty.
Interactive Tools and Online Platforms

Digital resources offer a variety of interactive options to reinforce learning. Platforms designed for practice and feedback allow learners to test their knowledge and track progress in real time. Some popular types of online tools include:
- Interactive problem solvers: Websites that allow students to work through problems step by step, providing instant feedback and explanations.
- Online tutoring services: Personalized tutoring sessions from experts who can assist with specific areas of difficulty and guide learners through challenging concepts.
- Video tutorials: Instructional videos that break down complex topics, offering visual and auditory explanations for better comprehension.
Workbooks and Practice Sheets
For those who prefer hands-on practice, printed workbooks and practice sheets can be a great addition. These resources offer additional problems to solve and often include detailed solutions to guide learners. Key benefits of using practice books include:
- Reinforcement of concepts: By practicing regularly, learners can strengthen their understanding and build confidence in their skills.
- Variety of exercises: Workbooks often provide a wide range of problem types, allowing students to develop a well-rounded grasp of the material.
- Clear explanations: Many workbooks include step-by-step solutions, helping learners understand the reasoning behind each problem-solving technique.
By utilizing these supplementary resources, learners can reinforce their understanding, gain confidence, and develop the necessary skills to succeed in their studies. Combining different tools, such as online platforms and printed materials, ensures a balanced approach to mastering new concepts.
When to Seek Help in Your Studies
Recognizing when you are struggling with certain concepts is crucial for your academic success. Sometimes, even after multiple attempts at understanding the material, progress may feel slow or challenging. Knowing when to reach out for assistance can help prevent frustration and keep you on track toward mastering the content. This section outlines key indicators that suggest it might be time to seek help and how to approach it effectively.
Signs You Need Assistance
If you’re unsure when to ask for help, here are some common signs that indicate you might benefit from additional support:
| Sign | What to Do |
|---|---|
| Consistently struggling with specific topics | Consider seeking help to clarify any misunderstandings or gaps in your knowledge. |
| Difficulty completing exercises or assignments | Review your study techniques and seek assistance to ensure you are on the right path. |
| Noticing a lack of progress despite studying | It may be time to consult a tutor or use online resources for a fresh perspective. |
| Feeling overwhelmed by the material | Break down the content into manageable parts and seek guidance to better understand the structure. |
Effective Ways to Get Help
When you decide to seek assistance, consider the following options:
- Consult a tutor: A tutor can provide personalized support, helping you grasp complex topics and reinforce your understanding.
- Join study groups: Collaborating with classmates allows for shared insights and explanations that can help you view concepts from different perspectives.
- Use online resources: Many websites offer tutorials, videos, and forums where you can get help from peers or experts.
- Ask your instructor: Don’t hesitate to approach your teacher or instructor with questions. They can offer valuable feedback and direct you to additional resources.
By recognizing the signs that indicate when you need assistance, you can take proactive steps to overcome challenges. Seeking help when necessary ensures that you stay on track and deepen your understanding of the material, ultimately leading to better academic outcomes.