
As you prepare for your upcoming assessment, it’s essential to focus on the core principles and problem-solving techniques that will guide you through various challenges. The key to performing well lies in understanding fundamental concepts, practicing regularly, and developing a methodical approach to each type of problem you may encounter.
By reinforcing your knowledge of essential formulas, strategies, and logical steps, you can approach your test with confidence. Whether you’re working with angles, shapes, or abstract equations, the right techniques will help you tackle each question with clarity. Take time to review the most common problem types and make sure you’re comfortable with all required methods.
Preparation is the foundation of success. Focus on mastering critical topics and solving a variety of problems to build your confidence. This approach will ensure you’re well-equipped to handle whatever the test may bring. With the right preparation, you’ll be able to handle all the material with ease.
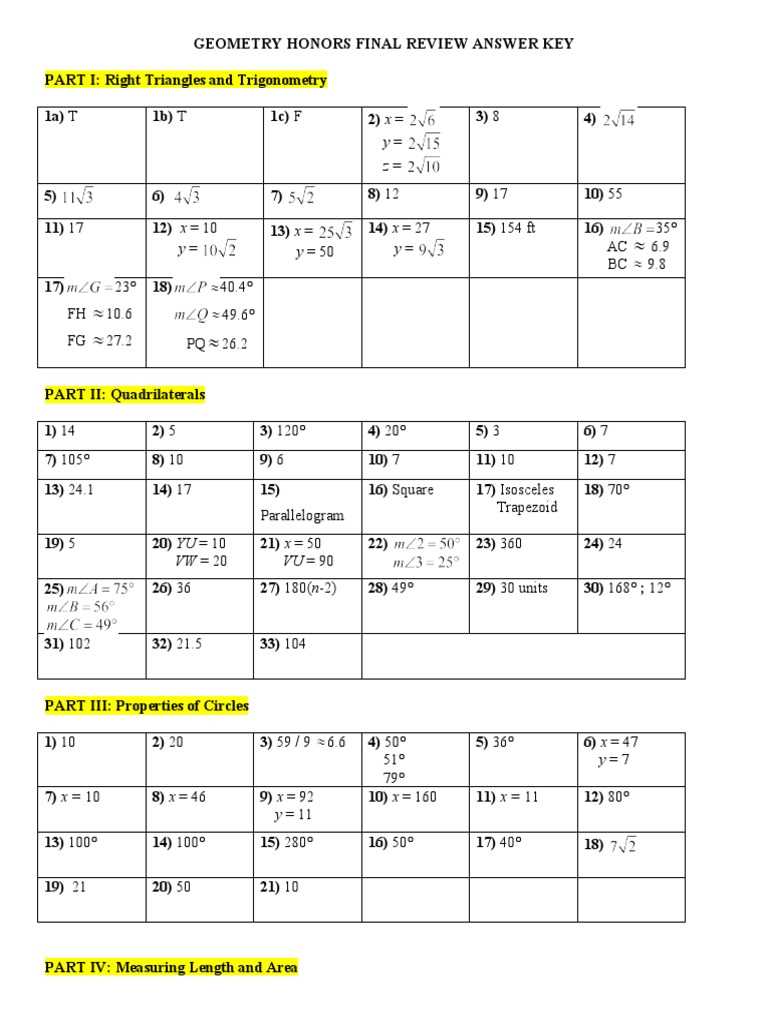
Geometry Final Review Answers
Understanding the core concepts and mastering key problem-solving techniques are essential when preparing for any assessment. Focusing on critical topics, reinforcing your knowledge, and practicing a variety of problems will help you perform confidently. The more familiar you are with different types of questions and their corresponding methods, the better prepared you will be for success.
Key Concepts to Focus On
Make sure you are comfortable with essential formulas and logical steps involved in solving each type of problem. Practice solving various problems that involve shapes, angles, and calculations. It’s also crucial to familiarize yourself with the relationships between different geometric elements, as this will often make the problem-solving process more intuitive. The ability to recognize patterns and apply known principles will give you a clear advantage during your assessment.
Effective Problem-Solving Strategies
Approach each problem systematically. Break down the question into smaller steps and tackle each part individually. Start by identifying the given information, followed by applying the relevant concepts. Don’t hesitate to draw diagrams to visualize complex problems, as this often helps simplify the process. Practicing these strategies will build both your understanding and speed, ensuring you can efficiently address each question on the test.
Key Concepts to Master in Geometry
To excel in any assessment involving shapes, angles, and spatial reasoning, it’s essential to grasp the fundamental principles that form the basis of all related problems. These core ideas serve as the building blocks for more advanced topics, allowing you to approach even the most complex questions with confidence. A solid understanding of these concepts is vital for achieving success.
Focus on mastering the relationships between different figures and their properties. Pay special attention to angles, sides, and symmetries within various shapes. Additionally, understanding how to apply formulas for calculating areas, perimeters, and volumes will be crucial for solving a wide range of problems. Reinforcing your knowledge of these essential topics will not only improve your problem-solving skills but also give you a deeper understanding of how geometric concepts are interrelated.
Step-by-Step Guide to Solving Geometry Problems
When faced with a complex problem involving shapes and measurements, it’s important to approach the task methodically. Breaking down the question into smaller, manageable steps allows for a clearer understanding and leads to more accurate solutions. By following a structured approach, you’ll be able to solve even the most challenging problems efficiently and confidently.
Below is a general framework for solving problems that involve spatial reasoning and calculations. This guide outlines each step of the process, from identifying known variables to applying the appropriate formulas.
| Step | Description |
|---|---|
| 1. Understand the Problem | Read the question carefully and identify what is given and what needs to be found. Draw a diagram if necessary to visualize the situation. |
| 2. Identify Relevant Formulas | Determine which mathematical principles or formulas apply to the problem. These could include area, perimeter, volume, or angle relationships. |
| 3. Organize the Information | List the known values and any relationships between them. Make sure all the information is clearly laid out. |
| 4. Solve the Problem | Apply the appropriate formulas step-by-step. Perform calculations carefully, ensuring each step is logical and consistent. |
| 5. Check Your Work | Review your calculations and the solution to ensure everything aligns. Recheck key steps to avoid simple errors. |
By following these steps, you will systematically work through any challenge, ensuring accuracy and a deeper understanding of the underlying principles.
Important Theorems for Geometry Finals
Understanding key theorems is crucial for solving problems that involve shapes, angles, and spatial relationships. These fundamental principles not only simplify calculations but also provide a foundation for deriving more complex results. Mastery of these theorems ensures that you can quickly apply the right tools to any given question, enhancing both accuracy and efficiency.
Below is a list of essential theorems that you should be familiar with. These will help you tackle a wide range of problems and serve as a basis for solving more intricate questions.
| Theorem | Description |
|---|---|
| Pythagorean Theorem | Relates the sides of a right triangle: a² + b² = c², where c is the hypotenuse and a, b are the other two sides. |
| Triangle Sum Theorem | The sum of the interior angles of any triangle is always 180°. |
| Angle Bisector Theorem | States that the angle bisector divides the opposite side of a triangle into two segments that are proportional to the adjacent sides. |
| Parallel Postulate | If a line is parallel to one of two intersecting lines, the alternate interior angles formed are congruent. |
| Area of a Circle | Calculates the area of a circle using the formula A = πr², where r is the radius. |
These theorems form the backbone of many problems, and understanding how and when to apply them will give you a significant advantage during any assessment involving spatial reasoning and calculations.
Tips for Geometry Exam Success
Achieving success in an assessment that involves spatial reasoning and mathematical problem solving requires more than just memorizing formulas. It’s about developing strong strategies, practicing consistently, and managing your time effectively. By preparing in a focused and organized way, you can approach the exam with confidence and increase your chances of performing at your best.
Time Management and Planning
One of the most important skills during any exam is time management. Allocate time for each section based on the difficulty and number of questions. Prioritize problems you are most comfortable with, leaving the more complex ones for later. This ensures that you maximize your performance on easier questions while still giving yourself time to tackle more challenging problems.
Practice Regularly and Review Key Concepts
Consistent practice is essential for mastering the material. Work through a variety of problems, paying attention to different types of questions that may appear on the exam. Review core principles and common problem-solving techniques. By repeating similar problems and concepts, you’ll develop muscle memory, allowing you to solve questions quickly and accurately under exam conditions.
How to Tackle Word Problems Effectively

Word problems can often seem daunting due to the amount of information presented in a narrative format. However, breaking down the problem and translating the words into mathematical operations can make the process much more manageable. With the right approach, these types of problems become less about interpreting complex language and more about applying known principles and formulas.
Read the Problem Carefully
The first step in solving any word problem is to read it thoroughly. Make sure you understand what is being asked before you start solving. Identify the key pieces of information, such as known values, variables, and the specific question. Underline or highlight the important details to make them easier to reference as you work through the problem.
Translate Words into Mathematical Operations
Once you’ve identified the key information, the next step is to translate the narrative into equations or formulas. For example, if the problem involves distances, time, and speed, think of the appropriate formula (like distance = rate × time). Organize the given data and convert the verbal description into something you can solve mathematically. This is where the ability to recognize relationships and patterns in the problem becomes essential.
Stay organized throughout the process. Write down each step and perform calculations carefully. By staying focused and methodical, you can simplify even the most complex word problems.
Common Mistakes to Avoid in Geometry
Even the most skilled individuals can make mistakes when solving problems that involve spatial relationships and measurements. It is easy to overlook small details or make assumptions, which can lead to incorrect solutions. By understanding common pitfalls and developing strategies to avoid them, you can improve your problem-solving accuracy and boost your confidence during any assessment.
Errors in Formula Application
One of the most common mistakes is applying the wrong formula or using it incorrectly. Each problem requires specific formulas, and misapplying them can lead to incorrect results. Ensure you are using the right equation for the task at hand and that you are inserting values correctly. Here are some typical formula-related errors to avoid:
- Forgetting to square the radius when calculating the area of a circle.
- Mixing up the formulas for surface area and volume of 3D shapes.
- Using the wrong Pythagorean theorem formula for non-right triangles.
Misinterpreting Diagrams and Information
Another common mistake is failing to accurately interpret diagrams or the given information. Often, diagrams are drawn to scale, and it’s important to refer to them as you solve the problem. Here are some tips for avoiding misinterpretation:
- Always check the labels and markings on diagrams to ensure you are using the correct data.
- Be cautious of assumptions–never assume angles or sides are equal unless explicitly stated.
- Double-check the relationships between different geometric elements (e.g., parallel lines or congruent angles).
By staying mindful of these common errors and being methodical in your approach, you can significantly improve your accuracy and problem-solving skills.
Geometry Formulas You Must Know
When solving problems involving shapes, sizes, and spatial relationships, knowing the essential formulas is key to finding the correct solutions. These formulas help you quickly calculate areas, perimeters, volumes, and other important measurements. Mastering these core equations ensures that you can confidently approach a variety of challenges in your studies.
Below is a list of important formulas that you should be familiar with:
Area and Perimeter Formulas
- Rectangle Area: A = length × width
- Rectangle Perimeter: P = 2(length + width)
- Triangle Area: A = 1/2 × base × height
- Circle Area: A = πr²
- Circle Circumference: C = 2πr
Volume and Surface Area Formulas
- Cube Volume: V = s³ (where s is the side length)
- Cylinder Volume: V = πr²h (where r is radius and h is height)
- Sphere Volume: V = 4/3πr³
- Surface Area of a Cube: SA = 6s²
- Surface Area of a Cylinder: SA = 2πr² + 2πrh
These essential formulas are the foundation for solving a wide range of problems. Familiarizing yourself with these will allow you to efficiently work through exercises and increase your overall mathematical fluency.
Understanding Proofs in Geometry
In mathematics, proving statements or theorems is an essential skill that allows you to demonstrate the validity of a claim based on logical reasoning. Unlike simply applying formulas, proofs require you to show how one fact leads to another, ultimately establishing the truth of the proposition. Understanding how to approach and structure proofs is key to solving many problems that involve relationships between shapes, angles, and other properties.
There are different types of proofs, each requiring its own method of reasoning. The process generally involves a step-by-step logical argument, starting from known facts and reaching the conclusion through valid deductions. Below are some tips and common methods for approaching proofs:
Steps to Approach a Proof
- Understand the Problem: Carefully read the statement you need to prove. Identify the known information and what you are asked to prove.
- Plan Your Proof: Think about the relationships and properties that apply to the situation. What theorems or definitions could be useful?
- Write the Proof: Begin with your known facts, use logical steps to move towards the conclusion, and make sure each step is justified.
- Conclude Your Proof: Ensure that the final statement follows directly from your reasoning, completing the logical chain.
Types of Proofs
- Direct Proof: You start from known facts and deduce the result in a straightforward manner, using definitions and theorems.
- Proof by Contradiction: Assume the opposite of what you want to prove, and show that this assumption leads to a contradiction.
- Proof by Induction: This is often used for statements that involve integers or sequences. You prove the base case and then show that if the statement holds for a particular case, it holds for the next one as well.
By practicing proofs and understanding the logical structure behind them, you can enhance your problem-solving abilities and tackle more complex questions with confidence.
Time Management for Geometry Exams
Effective time management is crucial when preparing for and taking exams that involve complex problem-solving. Properly allocating your time ensures that you can complete all sections of the test with accuracy and confidence. By adopting a strategic approach to time management, you can avoid rushing through problems and reduce stress during the exam.
Here are some essential tips to help you manage your time effectively during the exam:
Before the Exam
- Practice with Timed Tests: Simulate the exam environment by timing yourself during practice problems. This helps you understand how long it takes to solve different types of questions.
- Review Key Concepts: Prioritize studying the most important formulas and theorems. Spend time on areas where you are less confident to ensure you are well-prepared.
- Plan Your Study Schedule: Break down your study time into manageable blocks, focusing on different topics each day. This way, you can cover all material without feeling overwhelmed.
During the Exam
- Read Instructions Carefully: Make sure you understand the requirements of each question before you start working. Misinterpreting the instructions can waste valuable time.
- Start with Easier Problems: Begin with questions that you find straightforward to gain confidence and save time for more challenging ones later.
- Allocate Time for Each Section: Divide your total exam time by the number of sections or problems. Stick to the allocated time for each section to avoid spending too much time on one question.
- Leave Difficult Problems for Later: If a problem is taking too long, move on to the next one and come back to it if time permits.
By staying organized and following a structured approach, you can make the most of your time during the exam and increase your chances of success.
Strategies for Memorizing Geometry Concepts
Mastering mathematical concepts requires not only understanding the underlying principles but also committing essential formulas, theorems, and properties to memory. A solid grasp of these concepts allows you to apply them efficiently when solving problems. However, memorization can be challenging, especially when dealing with complex formulas and abstract ideas. Using effective memorization strategies can help reinforce your knowledge and improve recall during exams.
Here are some techniques that can enhance your ability to memorize key concepts:
Active Recall and Practice
One of the most effective methods for retaining information is active recall. Instead of passively reviewing notes, actively quiz yourself on formulas and definitions. Write down key formulas, then cover them and try to recall them from memory. Repetition is key–frequent practice will strengthen your memory over time. Additionally, solving problems that involve these concepts will help reinforce your understanding and make the formulas easier to recall when needed.
Visualization Techniques
Visualizing concepts can be a powerful tool for memorization, especially when dealing with geometric shapes and spatial reasoning. Draw diagrams, label important parts, and associate the formulas with specific shapes. Using color-coded notes or creating flashcards with images and definitions can also improve memory retention. Associating new information with familiar visuals or real-world examples makes it easier to recall during problem-solving tasks.
By incorporating these memorization strategies into your study routine, you can enhance both your understanding and retention of key mathematical principles, setting you up for success in your exams.
Practice Problems with Solutions
Working through practice problems is one of the best ways to reinforce your understanding and improve problem-solving skills. By applying the concepts you’ve learned to real problems, you can identify areas that need improvement and gain confidence in your ability to tackle similar questions during an exam. Below are some practice problems along with step-by-step solutions to help you strengthen your skills and prepare for your next challenge.
Practice Problem 1
Problem: Find the area of a triangle with a base of 8 units and a height of 5 units.
| Step | Explanation |
|---|---|
| 1 | Recall the area formula for a triangle: Area = (1/2) * base * height. |
| 2 | Substitute the known values into the formula: Area = (1/2) * 8 * 5. |
| 3 | Multiply: Area = 20 square units. |
Solution: The area of the triangle is 20 square units.
Practice Problem 2
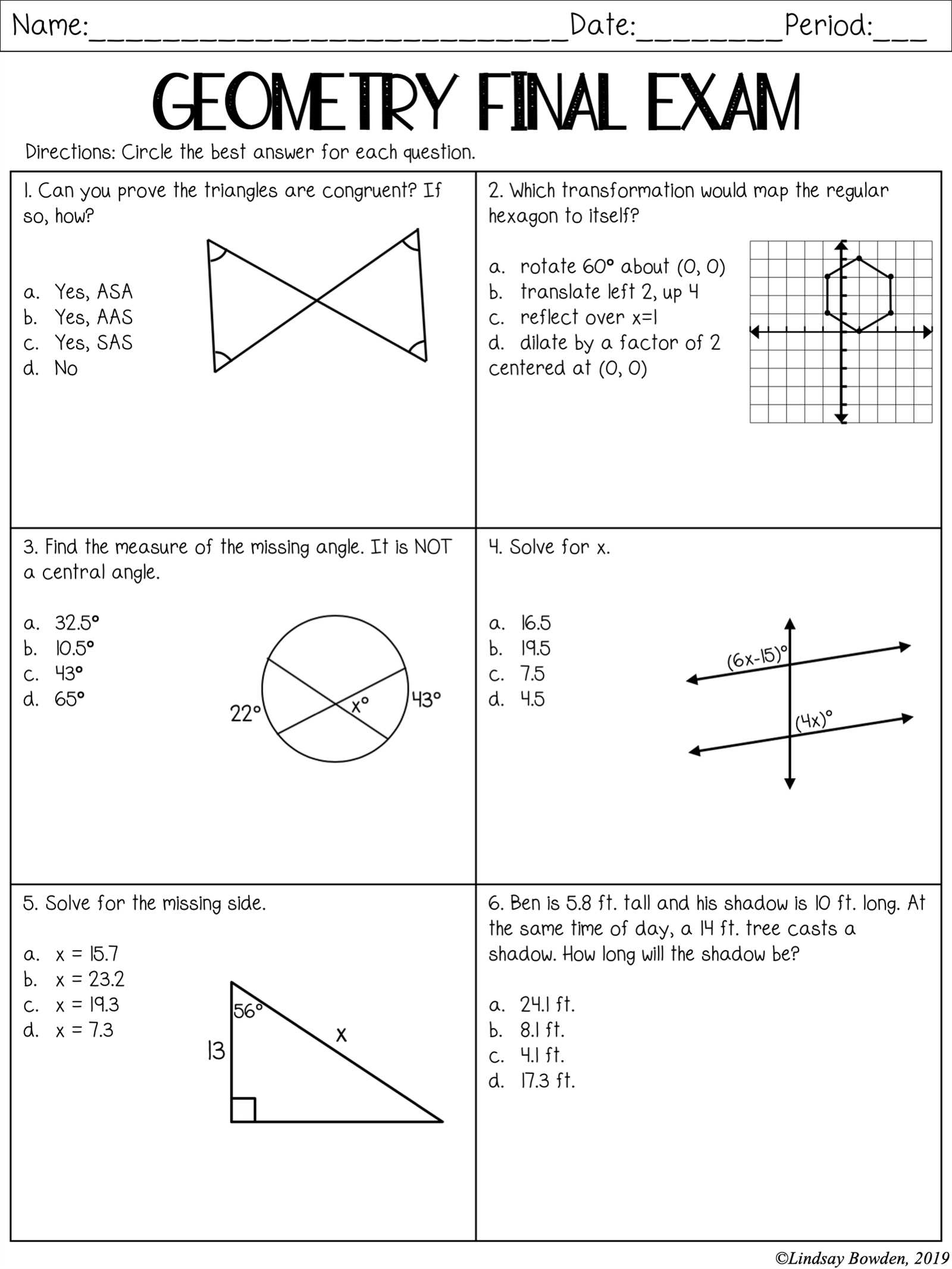
Problem: Solve for x in the equation 2x + 5 = 15.
| Step | Explanation |
|---|---|
| 1 | Subtract 5 from both sides: 2x = 10. |
| 2 | Divide both sides by 2: x = 5. |
Solution: x = 5.
These practice problems will help you build familiarity with the methods and steps needed to solve similar problems. Keep practicing, and with time, you will improve both your speed and accuracy in solving problems effectively.
Breaking Down Equations
Understanding and solving equations effectively requires breaking them down into manageable steps. Often, complex equations may seem intimidating, but by dissecting them and focusing on one part at a time, you can approach the solution methodically. This approach not only simplifies the process but also helps you grasp the underlying concepts, making problem-solving more intuitive.
Step-by-Step Breakdown

To solve any equation, follow these systematic steps to ensure accuracy:
- Identify Known Values: Start by noting all given values and variables. This will help you understand what needs to be solved and what tools or formulas are needed.
- Simplify the Equation: Eliminate any unnecessary terms or simplify expressions. Combine like terms to make the equation more straightforward.
- Apply Relevant Formulas: Depending on the type of problem, use appropriate formulas that relate the known values to the unknowns. For example, in problems involving areas or volumes, refer to the corresponding geometric formula.
- Isolate the Variable: If you’re solving for a specific variable, rearrange the equation so that the unknown is on one side of the equation, making it easier to solve.
- Check Your Solution: Once you have solved for the unknown, substitute your solution back into the original equation to verify that it holds true.
Example Problem
Consider the following equation: 2x + 5 = 15.
To solve this:
- Start by subtracting 5 from both sides to isolate the term with x: 2x = 10.
- Next, divide both sides by 2 to solve for x: x = 5.
- Finally, substitute x = 5 back into the original equation to check your work: 2(5) + 5 = 15, which is correct.
By following these steps and practicing regularly, you’ll become more comfortable with solving equations and identifying key components within them. Remember that breaking down an equation into smaller, simpler parts is a crucial skill that will benefit you in various problem-solving scenarios.
How to Prepare for Test Day
Proper preparation is key to performing well on any exam. The day before the test can be stressful, but with the right strategies, you can walk into the exam room feeling confident and ready. It’s important to ensure you’ve reviewed all necessary concepts, honed your problem-solving skills, and are mentally prepared for the challenges ahead. Time management and focused study can make all the difference in achieving success.
Organize Your Study Sessions
Effective preparation starts with organization. Here’s how to make the most of your study time:
- Review Key Concepts: Focus on the areas that you find most challenging. Whether it’s formulas, properties, or specific problem types, ensure you’re clear on the fundamentals.
- Practice Problems: The more problems you solve, the better you’ll understand how to apply concepts. Practice with both easy and complex questions to ensure you’re prepared for any situation.
- Take Breaks: Studying for long periods without rest can lead to burnout. Take short breaks every 30-45 minutes to keep your mind fresh.
Test Day Checklist
On the day of the exam, preparation goes beyond just your academic knowledge. Be sure to take the following steps:
- Get a Good Night’s Sleep: A well-rested mind is essential for clarity and focus. Aim for at least 7-8 hours of sleep the night before the test.
- Prepare Your Materials: Ensure you have all the necessary materials for the test, such as pens, pencils, an eraser, and any authorized tools (e.g., calculator, ruler).
- Eat a Healthy Breakfast: A nutritious breakfast will give you the energy you need to focus during the test. Avoid heavy or sugary foods that could make you feel sluggish.
By organizing your time effectively, reviewing key concepts, and ensuring you’re well-rested and prepared on test day, you’ll be setting yourself up for success. Remember, a calm and focused mindset is just as important as your academic preparation!
Reviewing Angles and Triangles Quickly
Mastering angles and triangles is essential for solving many problems efficiently. A clear understanding of their properties and relationships allows you to quickly identify solutions to various types of questions. Focusing on the key concepts and using strategies for quick recognition of patterns can save you valuable time during any test. Here, we’ll explore ways to quickly review these important shapes and figures to boost your readiness.
Understanding Angle Relationships
Angles play a significant role in many types of problems. Recognizing different angle relationships can help you solve questions more quickly:
- Complementary Angles: Two angles that add up to 90°.
- Supplementary Angles: Two angles that add up to 180°.
- Vertical Angles: Opposite angles formed by two intersecting lines, which are always equal.
- Adjacent Angles: Two angles that share a common side and vertex but do not overlap.
Key Properties of Triangles
Triangles are fundamental in solving problems related to shapes. Understanding the properties and different types of triangles is crucial for quick problem-solving:
- Equilateral Triangle: All sides and angles are equal, with each angle measuring 60°.
- Isosceles Triangle: Two sides are equal, and the angles opposite those sides are also equal.
- Scalene Triangle: All sides and angles have different measurements.
- Right Triangle: One angle is exactly 90°.
By familiarizing yourself with these key properties and relationships, you can quickly identify the necessary steps to solve problems involving angles and triangles. Practice identifying these figures in various contexts to sharpen your skills and improve your speed on any related questions.
Using Visual Aids for Geometry Problems
Visual aids are powerful tools when solving problems that involve shapes, angles, and measurements. They help clarify complex relationships and make abstract concepts easier to understand. By creating or studying diagrams, students can break down problems into manageable parts, visually mapping out the steps necessary for a solution. This method also helps in reducing errors and improving overall problem-solving efficiency.
Diagrams and Drawings: Drawing a clear and accurate diagram is one of the best ways to tackle spatial problems. It allows you to visualize the figures, identify key components such as angles and sides, and establish relationships between them. Always label important elements like vertices, lines, and measurements to avoid confusion.
Graphs and Charts: For problems that involve coordinate geometry or working with formulas, using graphs can be extremely helpful. Plotting points, lines, and shapes on a coordinate plane can make it easier to calculate distances, slopes, and areas. Additionally, using tables and charts to organize data can assist in breaking down steps and ensuring accuracy.
Color Coding: Sometimes, using different colors to highlight different elements of a diagram or problem can be beneficial. For example, you could color-code different parts of a triangle, like the sides, angles, or altitudes, making it easier to track and understand their relationships. Color coding is especially useful for distinguishing between known and unknown variables.
Incorporating visual aids into your study routine can help you grasp concepts more easily and prepare you for success when facing problems on exams. Practice creating diagrams and visualizing the relationships between different elements of a problem to develop a deeper understanding and improve your problem-solving skills.