
Mastering core concepts and techniques is essential to excel in any assessment. This guide offers a structured approach to help you revisit key topics and strengthen your problem-solving skills. Whether you’re revising for a major test or looking to enhance your understanding, it’s important to focus on the areas that matter most.
Focusing on critical areas will ensure you approach your exam with confidence. A solid grasp of fundamental principles will allow you to tackle complex problems with ease. By reviewing specific techniques and strategies, you’ll be able to improve your ability to solve various types of exercises.
Revising the material systematically allows for a deeper understanding of the concepts involved. Practice is key–the more problems you work through, the more proficient you become. Focus on breaking down each section and applying what you’ve learned to real-world examples.
Algebra 1 Midterm Review Packet Answers
Preparing for a significant examination requires a clear understanding of the material and an effective approach to problem-solving. This section aims to guide you through key exercises and topics commonly found in practice assessments, helping you focus on areas that may need more attention. By mastering these concepts, you can approach your test with confidence and efficiency.
It is essential to review the main topics thoroughly and understand how they interconnect. Topics such as solving equations, working with inequalities, and graphing functions are foundational skills that will help you tackle a wide variety of questions. With a systematic approach to practice, you’ll be able to solve problems with greater speed and accuracy.
Familiarity with different question types allows you to anticipate what you might encounter and handle unexpected challenges during the test. By revisiting key exercises and developing strategies for each type, you will not only reinforce your knowledge but also become adept at applying these concepts under exam conditions.
Key Concepts for Algebra 1 Midterm
To succeed in any major assessment, it is crucial to understand the core principles that form the foundation of the subject. Mastering the essential topics not only helps you tackle problems more efficiently but also allows you to apply your knowledge to a wide range of questions. Here are the fundamental ideas that should be part of your preparation:
- Linear Equations: Understanding how to solve simple and complex equations, including the steps of isolating variables and using properties of equality.
- Quadratic Functions: Familiarity with different forms of quadratic equations and their solutions, including factoring and using the quadratic formula.
- Inequalities: Grasping how to work with linear and quadratic inequalities, and understanding how to graph their solutions on a number line.
- Systems of Equations: Knowing methods such as substitution and elimination to solve for multiple variables simultaneously.
- Exponents and Powers: Mastery of laws of exponents, including multiplication, division, and negative exponents.
- Graphing Functions: Understanding how to plot equations on a coordinate plane and identify key features such as slopes, intercepts, and vertex points.
These are just a few of the critical areas to review. By focusing on these concepts, you will strengthen your problem-solving skills and improve your ability to manage the challenges you may encounter on your exam.
Understanding Equations and Inequalities
Equations and inequalities are fundamental tools used to express relationships between variables. Understanding how to manipulate and solve them is essential for mastering many types of problems. Whether dealing with simple linear expressions or more complex situations, recognizing how to balance both sides of an equation or inequality is key to finding the correct solution.
In equations, the goal is to find the value of an unknown variable that makes both sides of the expression equal. This often involves isolating the variable using various algebraic techniques such as adding, subtracting, multiplying, or dividing both sides. Inequalities, on the other hand, represent a range of possible solutions, where the relationship between two expressions is not necessarily equal but involves greater than, less than, or equal to relationships.
Solving these expressions requires careful attention to the rules that govern them. Operations performed on one side of the equation or inequality must be done to the other side to maintain balance. Being proficient in these operations allows you to confidently tackle problems and understand the underlying principles that drive more complex equations and inequalities.
Solving Linear Equations Step-by-Step
Solving linear equations involves finding the value of an unknown that satisfies a given mathematical relationship. The process of solving these equations is straightforward and involves a series of logical steps that simplify the expression until the variable is isolated on one side. By following a consistent approach, you can solve equations with ease and accuracy.
Start by eliminating any constants or coefficients attached to the variable. This usually involves using basic operations such as addition, subtraction, multiplication, or division. The goal is to isolate the variable by performing the same operation on both sides of the equation to maintain balance.
Once the variable is isolated, check your solution by substituting it back into the original equation to ensure both sides are equal. This process helps verify that the solution is correct. With practice, solving these types of equations becomes quicker and more intuitive, enabling you to handle increasingly complex expressions with confidence.
Mastering Quadratic Equations and Functions
Quadratic equations and their corresponding functions are key components of many mathematical problems. These expressions involve variables raised to the second power, and solving them requires specific techniques that help identify the roots or solutions of the equation. Understanding how to approach these problems effectively is essential for working with more complex mathematical scenarios.
One of the most common methods for solving these equations is factoring. This involves breaking down the quadratic expression into simpler binomials that can be easily solved. Another powerful technique is using the quadratic formula, which provides a straightforward way to find the solutions for any quadratic equation. Completing the square is another method that can be useful in certain situations, especially when the equation is not easily factorable.
In addition to solving the equation, understanding the graphical representation of a quadratic function is crucial. The graph of a quadratic function forms a parabola, and its key features–such as the vertex, axis of symmetry, and roots–can provide valuable insights into the behavior of the function. Mastering these techniques will enable you to solve and graph quadratic functions with confidence and precision.
Strategies for Simplifying Expressions
Simplifying expressions is a crucial skill that helps streamline complex mathematical problems. The process involves reducing an expression to its simplest form, making it easier to work with and solve. By applying the right strategies, you can eliminate unnecessary components and focus on the essential terms.
Combining Like Terms
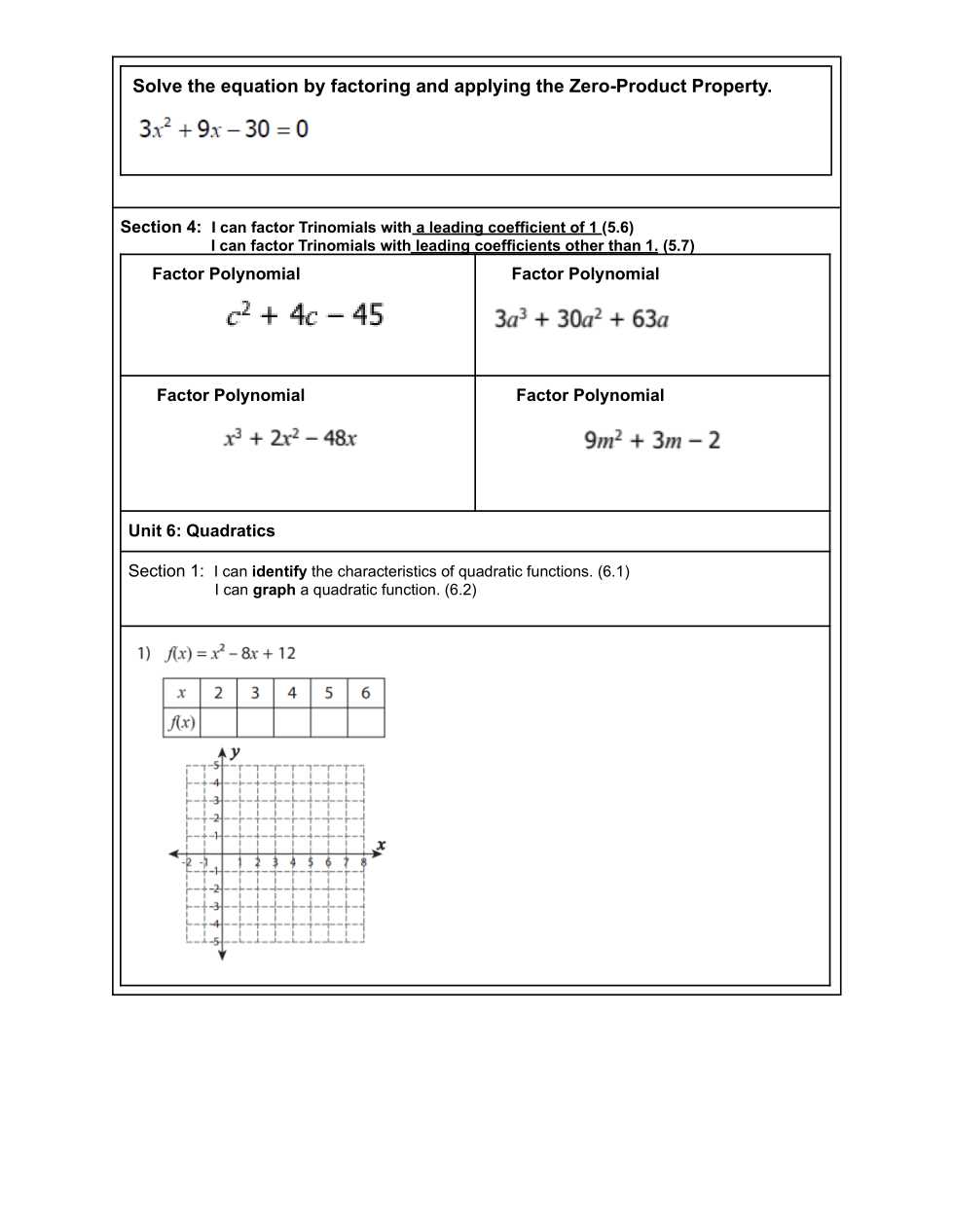
One of the first steps in simplification is identifying and combining like terms. Like terms are terms that have the same variable raised to the same power. By adding or subtracting these terms, you can reduce the expression to a more manageable form, which makes further operations much simpler.
Distributive Property
The distributive property is another powerful tool for simplifying expressions. This property allows you to multiply a term outside of parentheses by each term inside the parentheses. By distributing, you can eliminate parentheses and simplify the expression, making it easier to combine terms and solve.
Working with Systems of Equations
Systems of equations involve solving multiple equations simultaneously to find the values of the variables that satisfy all equations at once. These problems often arise when dealing with real-world scenarios where more than one condition must be met. Understanding how to solve these systems efficiently is essential for tackling complex problems in various fields of study.
There are several methods to solve a system of equations, each with its advantages depending on the structure of the equations. One common method is substitution, where you solve one equation for a variable and then substitute that expression into the other equation. Another widely used technique is elimination, which involves adding or subtracting the equations to eliminate one variable, making it easier to solve for the remaining one.
Mastering these methods will not only help in solving standard problems but will also improve your ability to handle more advanced systems, including those with more than two variables. The key to success is practicing these techniques and applying them strategically depending on the form of the equations.
Factoring Techniques for Algebra
Factoring is a key skill for simplifying expressions and solving equations. The process involves breaking down a complex expression into simpler components that, when multiplied, give the original expression. Mastering various factoring techniques is essential for solving higher-level problems and making complex expressions more manageable.
Factoring by Grouping
One effective technique is factoring by grouping. This method is used when an expression contains four terms. The goal is to group the terms into pairs, factor each pair separately, and then factor out the greatest common factor from the entire expression. This technique is particularly useful when dealing with polynomials that don’t immediately appear factorable.
Factoring Trinomials
Another common method is factoring trinomials, particularly those that are quadratic in form. In this technique, you look for two numbers that multiply to give the constant term and add up to the coefficient of the middle term. This allows you to break the expression into two binomials. Recognizing patterns in trinomials makes this technique highly efficient and valuable for solving related problems.
Graphing Linear and Quadratic Functions
Graphing functions is an essential skill for visualizing mathematical relationships. By plotting equations on a coordinate plane, you can easily observe how variables interact and understand the behavior of different types of functions. This section focuses on graphing two of the most common types of functions: linear and quadratic.
Graphing Linear Functions
Linear functions represent relationships where the change between variables is constant. When graphing these functions, the result is a straight line. To plot the graph, you can start by identifying key elements such as the slope and the y-intercept. The slope indicates the angle of the line, while the y-intercept is the point where the line crosses the vertical axis. Using these two points, you can quickly draw the line that represents the function.
Graphing Quadratic Functions
Quadratic functions, on the other hand, create a parabolic curve when graphed. These functions involve a variable raised to the second power, and their graphs open either upwards or downwards depending on the coefficient of the squared term. To graph quadratic functions, it is useful to find the vertex, the axis of symmetry, and the x-intercepts (if they exist). The vertex represents the highest or lowest point of the parabola, and understanding its position helps in sketching the graph accurately.
How to Solve Word Problems Effectively
Word problems often seem challenging because they require translating real-world situations into mathematical expressions. However, by following a structured approach, you can break down the information step by step and arrive at a solution with confidence. The key is to understand the problem thoroughly and identify the essential elements that need to be calculated.
Start by carefully reading the problem to ensure you understand what is being asked. Highlight or underline important information, such as numerical values and key terms. Next, define your variables–decide what each unknown represents. Once you have identified the variables, write an equation that models the relationship described in the problem.
After formulating the equation, solve for the unknowns using appropriate methods, such as arithmetic operations or algebraic manipulation. Check your solution by substituting the values back into the original context of the problem to verify they make sense. With practice, you’ll become more proficient at identifying patterns and applying the right techniques to efficiently solve word problems.
Reviewing Rational Expressions and Equations

Rational expressions and equations involve ratios of polynomials and require specific techniques for simplification and solving. Understanding how to handle fractions with variables in the numerator and denominator is essential for solving complex problems in various mathematical contexts. These expressions can be simplified, multiplied, divided, and solved by following systematic steps.
Simplifying Rational Expressions
To simplify rational expressions, the first step is to factor both the numerator and the denominator. After factoring, cancel out any common factors between the numerator and denominator. This reduction makes the expression easier to work with and often reveals the simplest form of the equation. It’s important to check for restrictions, ensuring that the variables do not cause division by zero.
Solving Rational Equations
Solving equations involving rational expressions often requires eliminating the denominators by multiplying both sides of the equation by the least common denominator (LCD). Once the denominators are cleared, the equation becomes easier to solve by traditional methods such as isolating the variable or factoring. Be sure to check for any extraneous solutions that might arise due to the restrictions on the domain of the original equation.
Operations with Polynomials Explained
Working with polynomials involves performing various operations such as addition, subtraction, multiplication, and division. These operations are fundamental for simplifying expressions and solving complex equations. Understanding how to manipulate polynomials effectively is essential for progressing in mathematics, especially when dealing with higher-degree terms and multiple variables.
Addition and Subtraction of Polynomials
When adding or subtracting polynomials, the key is to combine like terms. Like terms are terms that have the same variable raised to the same power. Here’s how you approach these operations:
- Identify and group the like terms together.
- For addition, sum the coefficients of the like terms.
- For subtraction, subtract the coefficients of the like terms, being careful to distribute the negative sign if needed.
After combining like terms, the result is a simplified polynomial that represents the sum or difference of the original expressions.
Multiplication of Polynomials
Multiplying polynomials involves using the distributive property to multiply each term in the first polynomial by every term in the second. This process is commonly known as the FOIL method when dealing with binomials. Here’s a step-by-step approach:
- Multiply each term in the first polynomial by each term in the second polynomial.
- Combine any like terms that result from the multiplication.
This method allows you to expand the expression and obtain the product of the polynomials. The result can then be simplified by combining like terms if necessary.
Understanding Functions and Their Graphs
Functions are essential concepts in mathematics that describe the relationship between two sets of values. The output depends on the input, and this relationship is often represented graphically. By understanding the behavior of functions and how their graphs are plotted, you can gain insights into how different variables interact and how changes in one variable affect the others.
Key Characteristics of Functions
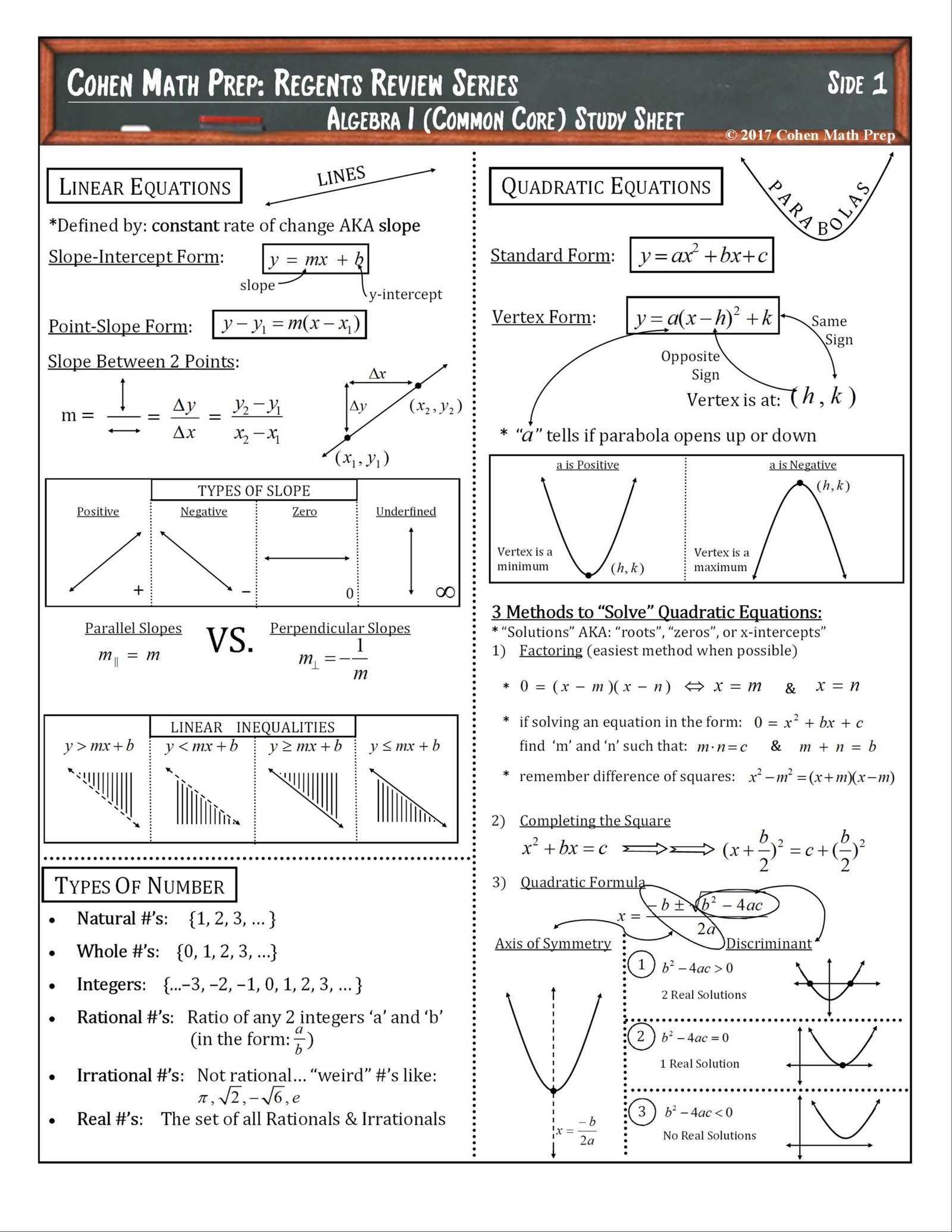
Functions can be represented in various forms such as equations, tables, or graphs. When analyzing a function, focus on the following key features:
- Domain: The set of all possible input values (independent variable).
- Range: The set of all possible output values (dependent variable).
- Intercepts: The points where the graph crosses the axes, such as the x-intercept and y-intercept.
Graphing Functions
To graph a function, it is helpful to create a table of values, plotting corresponding points on the coordinate plane. Here’s a general approach for graphing a function:
- Choose a set of input values for the independent variable (typically x).
- Calculate the corresponding output values by substituting the input into the function.
- Plot the calculated points on the graph and draw the curve or line that connects them.
By observing the graph, you can identify the function’s general behavior, such as whether it is increasing, decreasing, or constant over certain intervals. This visual representation is crucial for understanding how the function behaves across different values of the independent variable.
Exploring Exponents and Powers in Mathematics
Exponents and powers are fundamental concepts that allow us to express large numbers in a more compact form and simplify complex calculations. They represent repeated multiplication and are essential for understanding many mathematical operations. Mastering the rules of exponents helps streamline computations and provides a deeper understanding of mathematical relationships.
Basic Rules of Exponents
Exponents dictate how many times a number (the base) is multiplied by itself. Here are some of the key rules for working with exponents:
- Product Rule: When multiplying two numbers with the same base, add the exponents. For example, am × an = am+n.
- Quotient Rule: When dividing two numbers with the same base, subtract the exponents. For example, am ÷ an = am-n.
- Power of a Power: When raising a power to another power, multiply the exponents. For example, (am)n = am×n.
Negative Exponents and Zero Exponents
Understanding negative and zero exponents is crucial when simplifying expressions:
- Negative Exponents: A negative exponent indicates that the base should be taken as the reciprocal. For example, a-n = 1/an.
- Zero Exponent: Any number raised to the power of zero equals one. For example, a0 = 1, assuming a ≠ 0.
By applying these rules, you can simplify expressions and solve problems involving powers with greater ease and accuracy.
Tips for Tackling Practice Questions
Approaching practice problems strategically can significantly improve your ability to solve them correctly and efficiently. Whether you’re preparing for a test or working on strengthening your understanding of key concepts, the right approach can make all the difference. Here are some essential tips to help you handle practice questions with confidence.
1. Break Down the Problem
Before diving into calculations, take a moment to carefully read the problem and identify key elements. Break it down into smaller, more manageable steps. Understanding the structure of the problem is crucial for applying the correct methods. Ask yourself:
- What am I trying to find?
- What information is given, and how does it relate?
- What techniques or formulas do I need to use?
2. Show Your Work
While it may seem time-consuming, writing down each step of your solution helps keep track of your progress and ensures that you don’t skip important steps. This also makes it easier to spot errors and correct them as you go along. Even if the final answer seems obvious, showing your work demonstrates a clear understanding of the process.
By following these tips, you can develop better problem-solving habits, minimize mistakes, and approach practice questions with a clearer and more organized mindset.
Common Mistakes to Avoid

When working with mathematical problems, it’s easy to make simple errors that can lead to incorrect answers. Understanding where these mistakes occur and how to avoid them can significantly improve your problem-solving skills. Below are some of the most common mistakes people make and tips on how to avoid them.
| Common Mistakes | How to Avoid Them |
|---|---|
| Misunderstanding Operations Order | Always remember the order of operations (PEMDAS): Parentheses, Exponents, Multiplication and Division, Addition and Subtraction. |
| Sign Errors in Multiplication/Division | Double-check signs when multiplying or dividing negative numbers. A negative times a negative equals a positive. |
| Forgetting to Simplify Fractions | After solving equations or expressions, make sure to simplify fractions to their lowest terms. |
| Dropping or Misplacing Parentheses | Always check that parentheses are correctly placed, especially when dealing with negative signs or multiple operations. |
| Overlooking the Distribution Property | When multiplying terms, make sure you apply the distributive property correctly to each part of the expression. |
By being aware of these common mistakes and focusing on these areas, you’ll be able to approach problems with greater precision and confidence.
Preparing for the Exam
Proper preparation for any major exam requires understanding the material, practicing key concepts, and managing time effectively. It’s important to focus on the areas that may require extra attention, ensure all fundamental concepts are clear, and use a variety of study methods to reinforce learning. The following strategies will help you prepare efficiently for the upcoming assessment.
Study Techniques for Success

To achieve the best results, it’s crucial to break down your study sessions into manageable parts. Prioritize the most challenging areas and focus on them while also revisiting simpler topics to keep them fresh in your mind.
| Study Strategy | Benefits |
|---|---|
| Practice Problem Solving | Helps reinforce concepts and identify areas that need further review. |
| Break Down Complex Topics | Makes challenging concepts more approachable by dividing them into smaller, manageable sections. |
| Use Flashcards for Key Terms | Helps improve memory retention of important definitions and formulas. |
| Work with Study Groups | Allows for different perspectives on difficult topics and encourages collaborative problem-solving. |
| Review Mistakes from Practice Tests | Provides insight into where you went wrong, helping to avoid similar errors in the future. |
Effective Time Management
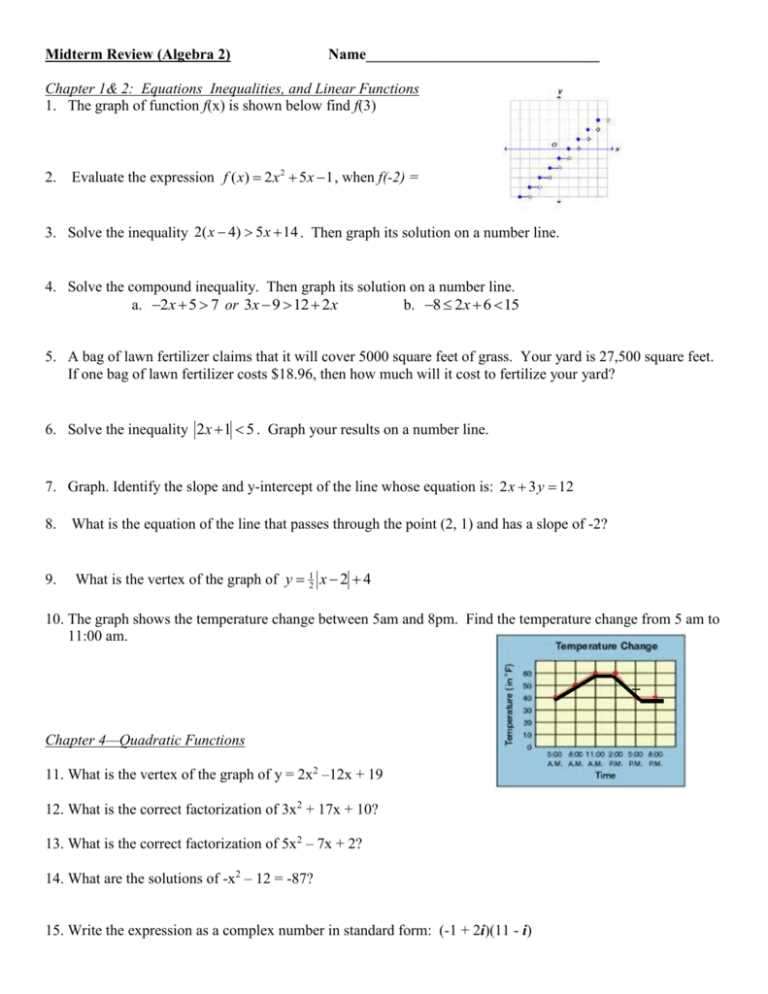
Setting a study schedule is essential for balancing your time between various subjects and topics. Make sure to allocate sufficient time for each area and stick to the schedule as much as possible. Regular breaks are also important to maintain focus and avoid burnout.
By following these strategies and maintaining a consistent study routine, you’ll be well-prepared to tackle the exam with confidence.