Mathematical evaluations can be challenging for many students, but with the right approach, they can become an opportunity to showcase knowledge and problem-solving skills. Whether you’re preparing for a standardized test or a classroom evaluation, understanding key concepts and practicing problem types is crucial for success.
Mastering the basics is the first step. Solidifying your understanding of core principles ensures you can confidently tackle various problems. Regular practice not only improves speed but also enhances your ability to recognize patterns and apply strategies effectively.
On the day of the assessment, having a clear strategy for tackling each section is essential. Time management is just as important as accuracy. By familiarizing yourself with typical questions and practicing in simulated conditions, you’ll be better prepared to perform at your best when it matters most.
Mathematics Assessment Preparation
Preparing for a math assessment requires a combination of reviewing essential concepts, honing problem-solving techniques, and practicing under test conditions. Success in these evaluations largely depends on the ability to apply mathematical principles quickly and accurately. This section will guide you through effective strategies to ensure you’re fully prepared for the upcoming challenges.
Key Concepts Review
Before diving into practice questions, it’s important to go over the fundamental topics that will be tested. Focus on strengthening your understanding of equations, functions, graphing, and data interpretation. Revisiting these areas will allow you to solve problems with greater confidence and precision.
Effective Practice Strategies
Regular practice is essential. Start by working through sample questions that mirror the format and difficulty level of the actual test. Time yourself to improve speed while maintaining accuracy. Additionally, review any errors to understand where improvements can be made. The more you practice, the more familiar you will become with the problem-solving methods and patterns that appear frequently.
Overview of Mathematics Assessment
The mathematics assessment is designed to evaluate a student’s grasp of essential mathematical principles. It covers a broad range of topics that test not only computational skills but also the ability to solve real-world problems and interpret data. Understanding the structure and expectations of this evaluation is key to performing well.
Test Structure
The assessment typically consists of multiple-choice questions, short-answer items, and extended-response questions. Each section focuses on different aspects of mathematical understanding, from basic calculations to more complex problem-solving scenarios. Students are expected to demonstrate both their knowledge of core concepts and their ability to apply them in various contexts.
Scoring Criteria
Scoring is based on both the accuracy and method used to arrive at solutions. Partial credit may be awarded for correct steps in problem-solving, even if the final answer is incorrect. This encourages students to show their thought processes clearly, as it helps examiners assess their understanding of key methods.
Key Topics Covered in Mathematics
This section focuses on the essential topics that form the foundation of the math evaluation. Understanding these key areas will equip students with the necessary tools to solve various types of problems efficiently. Mastery of these concepts is crucial for achieving success in the assessment.
Equations and Expressions
One of the core areas is solving linear equations and simplifying expressions. Students need to be familiar with methods for isolating variables, working with coefficients, and applying properties of equality to find solutions. These skills are essential for more advanced problem-solving tasks.
Functions and Graphing
Another key topic is understanding functions and their representations on graphs. This includes recognizing different types of functions, such as linear and quadratic, and interpreting their graphs. Being able to identify slope, intercepts, and transformations of functions is vital for tackling many test questions.
How to Approach Mathematics Questions
Effective problem-solving begins with a clear understanding of the question and a strategy to tackle it. By breaking down the problem into manageable steps, you can approach each question with confidence and clarity. The key is to stay organized and systematic throughout the process.
Read the Problem Carefully
Before starting, ensure that you fully understand what the problem is asking. Take a moment to identify the given information and what you need to find. This helps avoid unnecessary mistakes and ensures you focus on the relevant aspects of the question.
Use Logical Steps to Solve
Once you’ve understood the problem, approach it step by step. Start by setting up the necessary equations or expressions based on the given details. Then, systematically solve for the unknowns, checking each step to ensure accuracy. If needed, refer back to the original question to confirm that you are on the right track.
Understanding Mathematics Test Format
Knowing the structure of a math assessment is essential for effective preparation. Familiarity with the format helps you anticipate the types of questions that will be asked and organize your time efficiently during the test. Here’s a breakdown of the typical sections you will encounter in this evaluation.
Question Types
The test consists of different types of questions designed to assess your problem-solving abilities and mathematical knowledge. These usually include:
- Multiple-choice questions: Choose the correct answer from several options.
- Short-answer questions: Provide a numerical or written solution without explanation.
- Extended-response questions: Solve complex problems and show your work in detail.
Time Management
Each section has a set time limit, so managing your time is crucial. Here are some tips for staying on track:
- Start with the questions you find easiest to gain confidence and momentum.
- Allocate more time for the extended-response questions, as they require more detailed work.
- Leave time at the end to review your answers and check for errors.
Common Challenges in Mathematics Assessments
Many students face obstacles when taking a mathematics test, which can lead to unnecessary stress and lower performance. Identifying common difficulties ahead of time allows for better preparation and more effective strategies to tackle the assessment confidently.
Understanding Complex Problems
One of the most common challenges is deciphering complex problems that involve multiple steps. Often, students struggle with breaking down the problem into simpler parts or identifying the best approach to solve it. This can lead to confusion and mistakes.
Time Pressure
Another significant challenge is managing time effectively during the test. With limited time for each section, students may rush through problems, leading to errors or incomplete solutions. Prioritizing tasks and staying organized can help mitigate this issue.
Key Obstacles
The following table summarizes some common challenges students face and strategies to overcome them:
| Challenge | Strategy |
|---|---|
| Difficulty in understanding complex problems | Break the problem into smaller steps and rephrase the question. |
| Time management issues | Practice time management with mock tests and prioritize easier questions. |
| Not remembering key formulas | Create a formula sheet and practice recalling important equations. |
| Confusing similar concepts | Review related topics and focus on understanding the differences between them. |
Strategies for Time Management During the Test
Effective time management is crucial when taking a mathematics assessment. With a set time limit for each section, it’s essential to allocate your time wisely to ensure you can complete all questions. Proper planning and strategic thinking during the test can significantly improve your performance.
Prioritize Easier Questions First
Start by tackling the questions you feel most confident about. This allows you to build momentum and secure quick points. By addressing easier questions first, you can avoid wasting time on more difficult ones and ensure that you don’t run out of time.
Allocate Time for Review
Before starting the test, set aside time at the end to review your answers. This will give you the opportunity to check for any mistakes or oversights. If you’re unsure about a question, mark it and come back to it later when you have more time to think it through.
Essential Mathematical Formulas to Know
Having a solid grasp of key mathematical formulas is essential for solving problems quickly and accurately during a test. These formulas provide the foundation for solving equations, graphing functions, and analyzing data. Below are some of the most important formulas that students should be familiar with for effective problem-solving.
Core Formulas
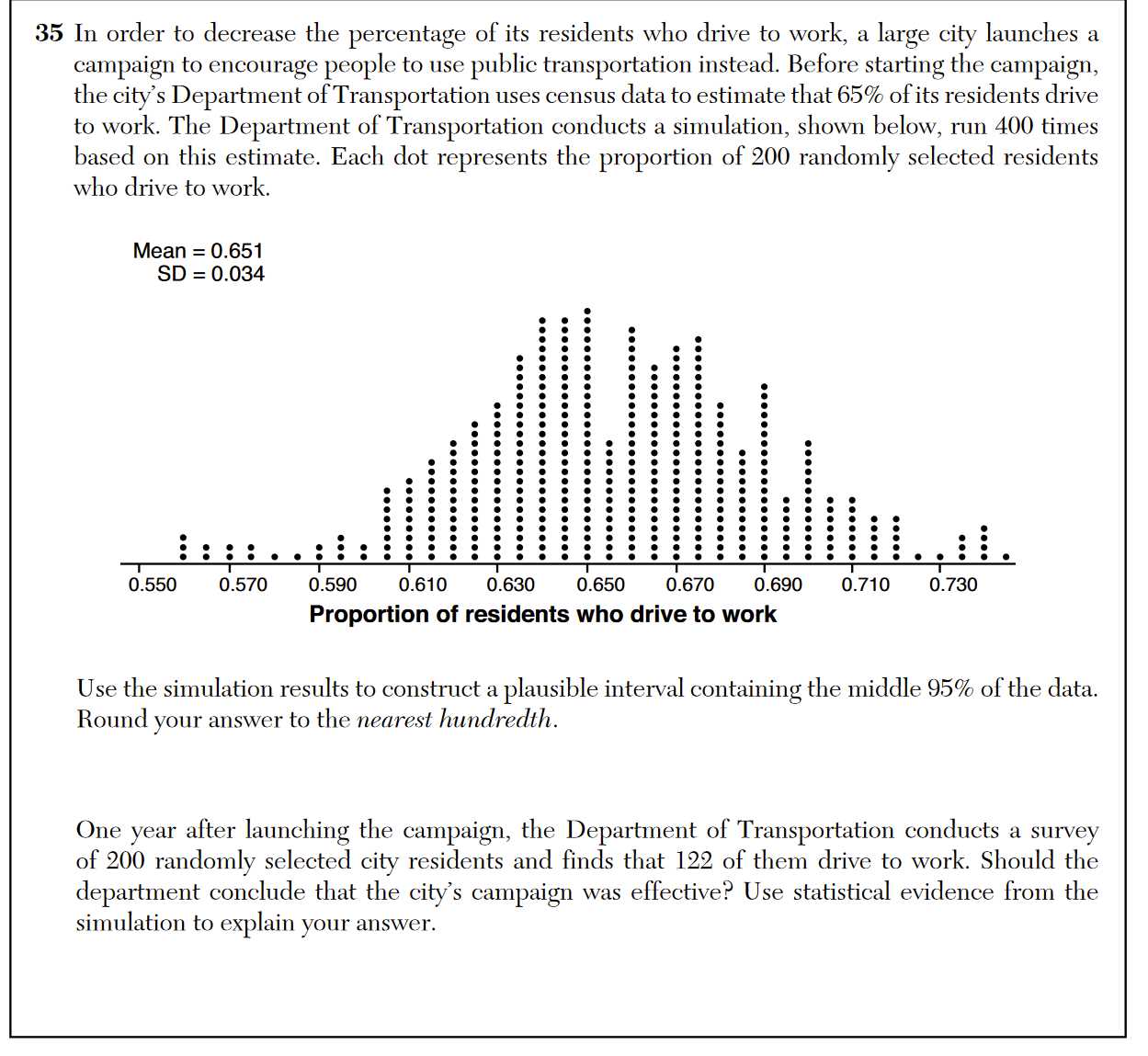
Below are the fundamental formulas that are frequently used in various mathematical problems:
- Linear Equation: y = mx + b (Slope-intercept form)
- Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a
- Distance Formula: d = √((x₂ – x₁)² + (y₂ – y₁)²)
- Pythagorean Theorem: a² + b² = c²
- Slope of a Line: m = (y₂ – y₁) / (x₂ – x₁)
- Factoring Difference of Squares: a² – b² = (a – b)(a + b)
Additional Important Concepts
In addition to the basic formulas, understanding the following concepts will also help with solving more advanced problems:
- Point-Slope Form: y – y₁ = m(x – x₁)
- Exponential Growth: y = a(1 + r)^t (where ‘r’ is the rate of growth)
- System of Equations: Methods such as substitution or elimination to find the values of variables.
Practice Problems for Mathematics Assessment
Practicing various problems is essential for reinforcing your understanding of key mathematical concepts. Working through different types of questions helps to sharpen problem-solving skills and prepares you for the format and difficulty of the test. Below are several practice problems designed to help you improve your skills in solving mathematical challenges.
Problem 1: Solve for x
Solve the following linear equation: 3x – 7 = 11
Problem 2: Simplify the Expression
Simplify the following expression: (5x + 2) – (3x – 4)
Problem 3: Graph the Equation
Graph the equation y = 2x + 3 on a coordinate plane.
Problem 4: Factor the Expression
Factor the following quadratic expression: x² – 5x + 6
Problem 5: Solve the System of Equations
Solve the system of equations:
- 2x + y = 10
- 3x – y = 4
How to Interpret Graphs in Mathematics

Interpreting graphs is a vital skill for solving mathematical problems. Graphs provide a visual representation of relationships between variables, making it easier to understand trends, patterns, and behavior of equations. Understanding how to read and analyze these graphs is crucial for making accurate predictions and solving real-world problems.
Identifying the Axes
The first step in interpreting any graph is to identify the axes. The horizontal axis (often referred to as the x-axis) typically represents the independent variable, while the vertical axis (the y-axis) shows the dependent variable. Understanding what each axis represents is essential for making sense of the graph.
Understanding the Shape of the Graph
Different types of functions and relationships produce distinct shapes on a graph. For example, a linear equation will form a straight line, while a quadratic function will create a parabola. Identifying these shapes can give you immediate insights into the nature of the relationship between the variables.
Analyzing Key Points
Key points such as the intercepts, slopes, and vertices are crucial for interpreting graphs. The x-intercept is where the graph crosses the x-axis, and the y-intercept is where it crosses the y-axis. The slope indicates the steepness of the graph and how one variable changes in relation to another. The vertex of a parabola is its highest or lowest point, depending on the direction of the curve.
Tips for Solving Word Problems Effectively
Word problems often require more than just mathematical calculations; they test your ability to interpret real-life situations and translate them into mathematical expressions. By following a structured approach, you can break down complex problems into simpler steps and solve them with confidence.
Understand the Problem First
Before jumping into the calculations, take time to carefully read the problem. Identify the key information and what is being asked. Highlight or underline important numbers, relationships, or conditions that will help you form an equation or solution. Understanding the context is crucial to finding the right approach.
Translate Words into Equations
Once you understand the problem, the next step is to translate the words into a mathematical equation. Look for keywords like “total,” “difference,” or “per,” which can guide you in setting up the correct expression. For example, “the sum of” indicates addition, while “the product of” suggests multiplication. This step is essential for setting up the proper framework for solving the problem.
Break the Problem into Smaller Parts
If the problem seems overwhelming, break it into smaller, more manageable parts. Solve one step at a time, and check your progress along the way. This approach not only makes the problem less intimidating but also ensures you stay organized and focused on the solution.
Check Your Work
After solving the problem, always take a moment to review your answer. Does it make sense in the context of the problem? Verify your calculations and ensure that your solution answers the question asked. Taking this extra step can help catch any mistakes and prevent incorrect answers.
Using a Calculator on the Test
In many assessments, the use of a calculator can significantly speed up the solving process, particularly for complex calculations or large numbers. However, knowing when and how to use this tool effectively is key to maximizing its benefits while maintaining accuracy throughout the test.
Choosing the Right Calculator
Before using a calculator, it’s important to ensure that it complies with the allowed specifications for the test. Typically, scientific or graphing calculators are permitted, but some tests may restrict the use of advanced functions. Familiarize yourself with the features of the calculator you plan to use, as this will save time during the test and help you focus on solving the problems rather than navigating the device.
When to Use a Calculator
While a calculator can be useful for calculations such as multiplication, division, and square roots, it’s crucial to understand when its use is appropriate. For simpler arithmetic or when estimating answers is sufficient, using a calculator may not be necessary. However, for complex formulas, large numbers, or tasks involving multiple steps, a calculator is a helpful tool that can reduce errors and increase efficiency.
Test-Taking Techniques for Mathematics

Success on any assessment requires not only understanding the material but also mastering strategies to approach questions efficiently. Developing effective test-taking techniques can help you manage time, reduce stress, and maximize your performance. By applying a few simple strategies, you can improve your chances of achieving the best results.
Read Instructions Carefully
One of the most common mistakes students make is rushing through the instructions. Before you begin answering questions, carefully read the directions to ensure you understand what is being asked. This is especially important when solving problems that require specific steps or methods. Taking time to understand the instructions can prevent costly errors.
Start with the Easiest Questions
When faced with a variety of questions, it’s often helpful to start with the ones that seem the easiest. This builds confidence and ensures you secure quick points. Tackling simple problems first also allows you to allocate more time to more complex questions without the pressure of running out of time.
Eliminate Obvious Wrong Answers
If the test includes multiple-choice questions, eliminate any answers that are clearly incorrect. By narrowing down the options, you increase your chances of selecting the correct answer. Even if you are unsure of the exact solution, reducing the number of possible answers can significantly improve your odds.
Manage Your Time Wisely
Time management is key during any test. Allocate a set amount of time to each section and stick to it. If you encounter a difficult problem, don’t spend too much time on it–move on to other questions and return to the tricky ones later if you have time. Keeping track of time ensures you don’t miss out on easier problems while spending too long on one question.
How to Review Mathematical Concepts
Mastering the key principles in mathematics requires more than just memorizing formulas. To truly understand the material, it’s important to engage in active review, reinforce problem-solving techniques, and test your skills regularly. By focusing on the core concepts and practicing various types of problems, you can ensure that you are well-prepared for any test or assessment.
Identify Core Topics
Before beginning your review, it’s essential to identify the most important topics that you need to focus on. These include foundational concepts that are frequently tested and that build upon each other. The following list highlights some key areas to consider:
- Linear equations and inequalities
- Exponents and powers
- Factoring polynomials
- Graphing linear functions
- Systems of equations
- Quadratic equations and functions
Practice with Sample Problems
To truly grasp mathematical concepts, practice is essential. Work through as many problems as possible, especially those that challenge you. Solving a variety of problems helps reinforce different methods and deepens your understanding. Try these strategies to improve your practice:
- Work on problems of varying difficulty levels
- Review incorrect answers to understand your mistakes
- Time yourself when solving problems to improve speed
- Utilize online resources or textbooks for additional practice questions
Consistent practice allows you to build confidence and refine your problem-solving skills, ensuring a more thorough understanding of the material.
Common Mistakes to Avoid in Mathematics
While studying and solving problems in mathematics, it’s easy to make mistakes that can hinder progress or lead to incorrect solutions. Recognizing these common errors early on can help you avoid them and improve your understanding. By being aware of these pitfalls, you can approach problems with more precision and confidence.
Here are some of the most frequent mistakes to watch out for:
- Misinterpreting the problem: Carefully read each question and ensure that you understand what is being asked. Skipping this step can lead to solving the wrong problem.
- Forgetting to simplify: Always simplify expressions and equations whenever possible. This can prevent confusion in later steps and help you avoid unnecessary mistakes.
- Incorrect order of operations: Remember to follow the correct order when performing mathematical operations. Use PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) to stay organized.
- Sign errors: Pay close attention to positive and negative signs. A small mistake with signs can drastically change the outcome of your calculation.
- Skipping steps: It’s tempting to skip over steps to save time, but this can lead to missed errors. Always show your work to ensure that no critical step is overlooked.
Avoiding these mistakes will improve accuracy and efficiency. It’s important to regularly review your work to catch any errors and ensure you’re on the right track.
Why Mathematics Assessments Are Important
Mathematics assessments play a significant role in evaluating a student’s understanding of core concepts and problem-solving skills. These tests not only measure knowledge but also prepare students for future academic and professional challenges. By successfully completing these evaluations, students demonstrate their mastery of foundational concepts that are vital for more advanced studies.
Here are a few reasons why performing well in these assessments is essential:
- Foundation for Advanced Learning: Mastering basic mathematical principles is crucial for tackling more complex subjects in high school and beyond, such as calculus and statistics.
- College and Career Readiness: A strong performance reflects a student’s ability to approach and solve problems, which is valuable in a wide range of academic and professional fields.
- Improved Problem-Solving Skills: These tests challenge students to think critically, promoting logical reasoning and analytical skills that are applicable in everyday life and future careers.
- Assessing Progress: The assessments help identify areas of strength and weakness, allowing students and educators to focus efforts on areas needing improvement.
- Building Confidence: Successfully navigating such challenges boosts confidence, preparing students for future tests, exams, and life’s various challenges.
In conclusion, performing well in these assessments is not just about passing a test–it’s about laying a strong foundation for future academic success and career opportunities.
What to Expect on Test Day
Test day is an important moment where preparation and focus come together. On this day, students will be asked to demonstrate their understanding of key concepts they have studied. While the atmosphere may feel tense, knowing what to expect can help reduce anxiety and improve performance. Proper preparation allows for a smoother, more confident test-taking experience.
Here’s what you can expect:
- Arrival and Check-In: You will need to arrive at the test center early to check in. Be sure to bring any necessary identification and materials, such as a valid ID and approved calculator.
- Test Instructions: Before you start, an instructor or proctor will provide instructions on how to complete the test, explain the format, and outline any rules you must follow during the process.
- Test Environment: You will be in a quiet, controlled environment with other students. Expect a calm, focused atmosphere where you are expected to stay silent and work independently.
- Time Limit: There will be a set time limit for completing the assessment. Make sure to manage your time wisely, budgeting enough moments to review your work and address difficult questions.
- Varied Question Types: The test will likely contain a combination of multiple-choice questions, short-answer problems, and word-based tasks. Be ready to apply different strategies for each type.
- Breaks: Some tests allow for short breaks, but this will depend on the rules set for that particular assessment. Use breaks wisely to refresh your mind.
- Final Steps: Once you finish, double-check your responses before submitting. Ensure you’ve answered all questions and filled in any necessary sections before handing in your paper.
In conclusion, staying prepared, calm, and focused is key. With the right mindset and organization, test day can be a manageable and successful experience.
After the Exam: What’s Next?
Once you’ve completed the assessment, it’s natural to feel a sense of relief, but the process doesn’t end there. What follows is just as important as the preparation and the test itself. Knowing what to do after you’ve finished can help you stay organized and focused on your next steps. Whether you’re waiting for results or moving on to new goals, managing your time and expectations can lead to better outcomes.
Next Steps After Completing the Test

After finishing the assessment, here’s what to expect:
- Relax and Recharge: Take some time to unwind after the test. Rest and relax to allow your mind to recover from the intensity of the task.
- Review Performance: Once your results are available, carefully go over the feedback. Focus on areas where you can improve for future assessments or tasks.
- Set New Goals: Use the feedback from the assessment to set new learning objectives. Address any gaps in your knowledge and continue to refine your skills.
- Celebrate Your Effort: Regardless of the outcome, acknowledge the effort you put into preparing and completing the task. Every experience is an opportunity for growth.
Understanding Your Results
Once the results are in, it’s time to assess how you did. Understanding the feedback will guide your next steps. Below is a helpful overview of how to interpret your performance:
| Performance Level | Action to Take |
|---|---|
| Excellent | Celebrate your success and continue to challenge yourself with more advanced material. |
| Good | Review any mistakes and keep up with your studies to maintain your performance. |
| Needs Improvement | Focus on the areas where you struggled, and seek additional help if necessary to strengthen your understanding. |
| Unsatisfactory | Take time to revisit key concepts, work with a tutor or study group, and approach the material from different angles. |
After the test, the journey continues. Your results will help inform your next steps, whether it’s additional learning, personal reflection, or even preparing for future assessments. Always focus on continuous improvement and stay motivated!