
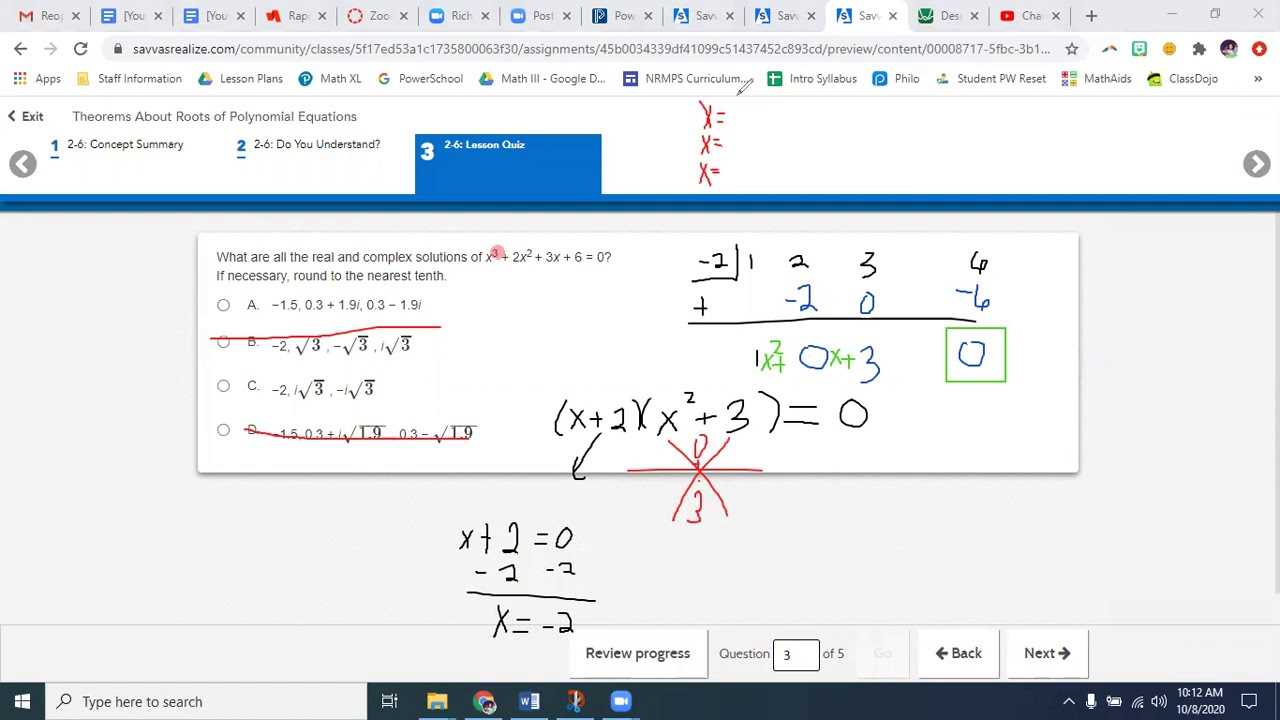
In this section, we focus on mastering essential concepts in the world of shapes, sizes, and spatial reasoning. By working through various exercises, students can sharpen their problem-solving abilities and enhance their understanding of fundamental principles. The exercises are designed to build a strong foundation for more advanced topics and offer practical insights into the relationships between different elements within a mathematical system.
Each problem encourages critical thinking and a methodical approach to finding solutions. It is important to approach every challenge step by step, ensuring all variables are considered and all calculations are precise. Mastering these key concepts provides the tools needed to tackle more complex scenarios in the future with confidence and clarity.
Solving Mathematical Problems Step by Step
In this section, we explore effective strategies for tackling various exercises involving shapes and their properties. Each problem requires careful analysis and application of core concepts, allowing you to improve your skills and understanding of mathematical relationships. By working through these challenges, you will develop a systematic approach that helps break down complex scenarios into manageable parts.
Below is a table summarizing the key steps and methods used to solve each problem, along with examples that illustrate how to approach them. This will guide you through the process and provide a clearer path to finding solutions.
| Step | Description | Example |
|---|---|---|
| 1 | Identify the given information and what needs to be determined. | For instance, the length of a side in a triangle. |
| 2 | Apply relevant principles or formulas to the given information. | Use the Pythagorean theorem for right-angled triangles. |
| 3 | Calculate the unknown values step by step. | Find the hypotenuse of a right triangle with known side lengths. |
| 4 | Verify the accuracy of the results. | Ensure that the calculated side length satisfies the triangle’s properties. |
By following these steps, you’ll be able to solve problems efficiently and confidently. Practice regularly to strengthen your skills and deepen your understanding of mathematical principles.
Key Concepts in Geometry Practice B
Understanding the foundational principles of shapes and spatial relationships is essential for solving problems effectively. This section focuses on building a deeper knowledge of geometric properties and how they can be applied to real-world scenarios. By grasping these core concepts, students will be able to approach more complex challenges with confidence and clarity.
Relationships Between Shapes
One of the primary aspects of this study is understanding how different geometric figures interact with one another. This includes analyzing the properties of angles, sides, and vertices, and recognizing how these elements form the structure of various shapes. Mastery of these relationships allows for accurate predictions and problem-solving in a variety of contexts.
Applying Theorems and Formulas
Another important concept is the ability to apply mathematical theorems and formulas to find unknown values. By learning how to use well-established rules, such as the Pythagorean theorem or area formulas, students can quickly solve problems involving angles, distances, and areas. The key to success is knowing when and how to apply these formulas in different situations.
Step-by-Step Solutions for Geometry Problems
Breaking down complex problems into manageable steps is a key approach to mastering mathematical challenges. By following a structured process, you can systematically work through each part of the problem, ensuring that no detail is overlooked. This method not only helps in finding accurate solutions but also builds a strong foundation for tackling more difficult tasks in the future.
Step 1: Carefully examine the problem to identify all the given information and what needs to be determined. Pay close attention to the numbers, shapes, and relationships provided.
Step 2: Choose the appropriate method or formula based on the type of problem you’re solving. For example, if you’re dealing with a triangle, you might apply the Pythagorean theorem or trigonometric ratios.
Step 3: Perform calculations step by step, ensuring that each stage follows logically from the previous one. Take time to check your work as you go to prevent errors.
Step 4: Once you have reached a solution, verify it by considering whether it fits with the problem’s constraints and whether it makes sense in the context of the question.
By practicing this method, you will become more confident in solving similar problems efficiently and accurately, making the learning process more effective and rewarding.
Understanding Geometric Proofs and Techniques

Mastering the art of proving geometric statements is an essential skill in mathematics. A proof provides a logical argument that demonstrates the validity of a claim based on known facts, definitions, and postulates. Developing a deep understanding of the techniques used in these logical arguments will enhance problem-solving abilities and allow for clear reasoning in complex scenarios.
There are several key strategies used in geometric reasoning:
- Direct Proof: This technique involves starting with known facts and logically building towards the desired conclusion. It is often used when the relationship between elements is clear and straightforward.
- Indirect Proof: Also known as proof by contradiction, this method assumes the opposite of what you want to prove and shows that it leads to a contradiction, thereby proving the original statement.
- Proof by Induction: This technique is used to prove statements for an infinite number of cases by proving it true for a base case and then showing that if it’s true for one case, it must be true for the next.
In addition to the types of proof, it’s important to use certain techniques for working with geometric figures:
- Use of Properties: Knowing the properties of shapes, such as the angles in a triangle or the relationship between parallel lines, is key to making valid conclusions.
- Constructing Auxiliary Lines: Often, adding extra lines or segments to a diagram can help clarify relationships and make the proof easier to follow.
- Using Algebraic Methods: Sometimes, incorporating algebra to solve for unknown variables can simplify the process of proving geometric relationships.
By understanding and practicing these techniques, you can approach geometric proofs with confidence, using logical reasoning to establish the truth of various propositions.
Common Mistakes in Geometry Practice B

When solving problems involving shapes and spatial relationships, students often encounter certain pitfalls that can lead to incorrect results. These mistakes can arise from misinterpreting the question, applying the wrong formulas, or overlooking critical details in diagrams. Understanding these common errors is crucial for improving accuracy and building confidence in solving similar problems in the future.
Misinterpreting Problem Statements: One frequent mistake is misunderstanding the information given in the problem. It’s essential to carefully read the question and identify what is being asked before beginning the calculations. Skipping this step can lead to working on the wrong aspects of the problem.
Incorrect Application of Formulas: Another common error occurs when students apply the wrong formula or fail to account for all variables in a formula. For example, using the wrong area or perimeter formula for a given shape can result in an incorrect solution. Always double-check which formula is most appropriate for the specific type of problem.
Overlooking Diagram Details: Diagrams are vital tools for understanding geometric relationships. Sometimes, students fail to notice important information, such as angle measures or specific side lengths, which can lead to incorrect conclusions. It is important to carefully examine all elements of the diagram before proceeding with the calculations.
Forgetting Units of Measurement: Often, students neglect to include units when calculating distances, areas, or volumes. This oversight can result in answers that are technically correct but incomplete. Always remember to include appropriate units in your final solution.
By being aware of these common mistakes, you can take steps to avoid them and improve your problem-solving skills. Practice and attention to detail will help ensure more accurate results and a deeper understanding of mathematical concepts.
How to Approach Geometry Word Problems
Word problems in mathematics can seem daunting at first, but breaking them down into smaller, manageable steps can make them easier to solve. These types of problems often involve a combination of concepts and require careful analysis to understand the information provided. By following a systematic approach, you can approach each word problem with clarity and confidence.
Here are the key steps to take when solving these problems:
- Read the Problem Carefully: Start by thoroughly reading the problem to understand what is being asked. Identify all the relevant information, including numbers, shapes, and relationships. Pay attention to keywords that indicate operations, such as “sum,” “difference,” “product,” or “perimeter.”
- Identify Known and Unknown Values: Highlight or underline the given values in the problem. Determine what you need to find, such as the length of a side, the area of a shape, or the measure of an angle. This will help you focus on the essential elements of the problem.
- Draw a Diagram: If the problem involves geometric figures, drawing a diagram can help visualize the situation. Label the known values and add any additional information that may help in solving the problem.
- Choose the Right Strategy: Decide which formulas or theorems are applicable to the problem. Whether you’re working with areas, angles, or side lengths, selecting the correct method is crucial for accurate results. Look for patterns or relationships in the problem that can guide you in your approach.
- Perform the Calculations: Carry out the necessary calculations step by step. Be careful with operations and ensure each calculation is correct before moving to the next step. Double-check the application of formulas and values.
- Review Your Solution: Once you’ve completed the calculations, check your solution by reviewing the problem’s requirements. Ensure that your answer makes sense in the context of the question and that all units are correct.
By following these steps, you will improve your ability to solve word problems efficiently. Practice regularly to build confidence and sharpen your skills in tackling similar challenges.
Reviewing Important Geometric Theorems
In mathematics, understanding the key principles that govern shapes and their properties is essential for solving a wide range of problems. Geometric theorems serve as foundational truths that help explain relationships between angles, sides, and other elements within figures. By reviewing and mastering these theorems, you can approach complex problems with a clear strategy and improve your overall problem-solving skills.
Basic Theorems and Their Applications
Some of the most important theorems form the basis of many geometric solutions. These include:
- The Pythagorean Theorem: This theorem is crucial for working with right triangles. It states that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides. This principle is widely used to find unknown side lengths.
- Angle Sum Theorem: This theorem asserts that the sum of the interior angles of any triangle is always 180 degrees. It is fundamental for calculating angles in various geometric figures.
- Congruence and Similarity Theorems: These theorems help identify when two figures are identical in shape and size (congruent) or identical in shape but not necessarily size (similar). These principles are key when working with transformations and proportional reasoning.
Advanced Theorems for Complex Problems
For more advanced geometric problems, several higher-level theorems provide powerful tools to solve complex challenges:
- Thales’ Theorem: This theorem states that if A, B, and C are points on a circle where line BC is a diameter, then the angle ∠ABC is a right angle. This is particularly useful in circle geometry.
- Ceva’s Theorem: This theorem helps in triangle geometry, where it describes a condition for concurrent lines within a triangle. It is used in advanced geometric proofs and constructions.
Mastering these theorems not only aids in solving problems but also deepens your understanding of how different geometric concepts connect. Regular review of these important principles will enhance your ability to tackle even the most difficult problems.
Tips for Solving Geometry Equations Effectively
Solving equations involving shapes and their properties requires both logical reasoning and a solid understanding of key mathematical principles. Whether you’re working with areas, perimeters, angles, or other measurements, applying the right techniques can make the process smoother and more efficient. By following a few useful tips, you can improve your approach and achieve more accurate results with less effort.
Understand the Problem Fully
The first step in solving any equation is to fully understand the problem. Take your time to read the question carefully and identify what is being asked. Pay attention to any given values and relationships between the elements of the figure. A clear understanding of the problem ensures you apply the correct formula or approach.
Use the Correct Formula and Variables
Equations in mathematics often require the use of specific formulas for different shapes and situations. It is essential to choose the right one based on the information provided. For example, if the problem involves a triangle, you may need to use the area formula or the Pythagorean theorem. Always check that you are using the correct variables and that they are substituted into the formula accurately.
Here are a few tips to consider when working with geometric equations:
- Double-check the units: Ensure that all measurements are in the same units before performing any calculations. Converting between units may be necessary to avoid errors.
- Break down complex problems: If the equation involves multiple steps, break it down into smaller, more manageable parts. Solve one step at a time to prevent confusion.
- Work with diagrams: Drawing a diagram or labeling a given figure can help you visualize the problem and make the necessary calculations more straightforward.
- Check your solution: After solving the equation, review your work to ensure that your answer makes sense and fits with the problem’s requirements.
By applying these tips, you can approach geometric equations with greater confidence and accuracy. With practice, you’ll find yourself solving problems more quickly and efficiently.
Visualizing Geometry Problems for Better Clarity
One of the most effective strategies for solving complex problems in mathematics is visualization. When dealing with spatial relationships, angles, and measurements, creating a mental or physical image of the problem can provide significant insight. By translating abstract information into a visual form, you can make connections between different elements and approach the problem with a clearer perspective.
Drawing a diagram or sketching a figure is often the first step in simplifying a problem. This method helps break down the information into understandable components, making it easier to identify relevant variables and relationships. Additionally, visualization can reveal patterns that may not be immediately obvious from the problem’s description alone.
Key Benefits of Visualization
There are several advantages to visualizing problems:
- Improves understanding: A clear visual representation can make the problem easier to grasp, allowing you to see the connections between different parts of the figure.
- Helps with accuracy: By labeling points, lines, and angles, you ensure that all given information is accounted for, reducing the chance of errors in calculations.
- Guides problem-solving: A well-drawn diagram often provides clues about the correct steps to take, whether it’s identifying parallel lines, symmetry, or geometric properties.
- Enhances memory: Creating a visual representation can help reinforce the concepts and formulas related to the problem, making them easier to recall when needed.
Practical Tips for Effective Visualization
Here are some practical tips to help you visualize problems more effectively:
- Use accurate measurements: Ensure your drawings are to scale and that all relevant dimensions are included. This will help you avoid mistakes when applying formulas.
- Label all parts of the figure: Labeling points, lines, and angles with appropriate symbols or variables makes it easier to reference these elements during calculations.
- Use colors or shading: Differentiating parts of the figure with color or shading can make key elements stand out and help with organization.
By incorporating visualization into your problem-solving process, you can improve your understanding, reduce errors, and solve problems more efficiently. With practice, this method will become an invaluable tool in your mathematical toolkit.
Breaking Down Complex Geometry Figures
When faced with intricate shapes or multi-part figures, it can often feel overwhelming to tackle the problem as a whole. However, by breaking down a complex figure into smaller, more manageable sections, you can simplify the process and approach each part with a clear strategy. This method not only makes calculations easier but also helps in identifying relationships between different elements of the figure.
The key to solving problems involving complicated figures lies in recognizing familiar shapes within the larger structure. Once these familiar elements are identified, you can apply known formulas and principles to each smaller section and then combine the results for the overall solution.
Steps to Break Down Complex Figures
Here are some steps to help you break down complex figures effectively:
- Identify basic shapes: Look for simple, recognizable shapes such as triangles, rectangles, or circles within the larger figure. These basic shapes often have well-known properties and formulas that you can use to calculate areas, perimeters, and other measurements.
- Divide the figure: If the figure is irregular, try dividing it into smaller, simpler sections. This can often be done by drawing lines to create rectangles, triangles, or other common shapes, making each part easier to analyze.
- Label each section: Clearly label the dimensions, angles, and points of each smaller shape. This will help ensure accuracy when applying formulas or performing calculations.
- Use symmetry: If the figure has symmetrical properties, use these to your advantage. Often, symmetrical sections can be treated as identical, reducing the number of calculations required.
Combining Results for the Final Answer
Once you’ve solved for the individual sections, you can combine the results to find the overall measurement, such as the total area or perimeter. Make sure to double-check your work as you sum the values to avoid any errors. Sometimes, subtracting certain sections from the larger figure is necessary, especially in cases where there are overlapping or missing areas.
By breaking down complex shapes into simpler components, you can approach each problem more systematically and with greater confidence. This strategy helps you focus on one piece at a time, ensuring that you apply the correct methods and formulas to each section.
Importance of Accuracy in Geometric Calculations
Precision is essential when working with mathematical problems, especially those involving shapes and measurements. Small mistakes in calculations can lead to large errors in the final result, affecting not only the answer but also the understanding of the underlying principles. Whether you’re solving for area, volume, or angles, each step must be executed with careful attention to detail to ensure correct outcomes.
Inaccurate measurements or misapplication of formulas can distort the solution, making it impossible to draw reliable conclusions from the results. This is why developing a keen sense of accuracy and double-checking your work is vital. The smallest oversight can propagate through calculations, resulting in significant deviations from the correct solution.
Common Sources of Error
There are several common sources of error in geometric calculations that can compromise the accuracy of your results:
- Misreading measurements: Incorrectly reading values from diagrams or tables can lead to errors. Always ensure that you accurately interpret the dimensions provided in the problem.
- Incorrect application of formulas: Using the wrong formula for a specific shape or problem type can result in incorrect answers. Double-check the formulas you are using to ensure they are appropriate for the given problem.
- Rounding errors: Rounding intermediate values too early or too much can cause significant inaccuracies in the final answer. Avoid rounding until the final step of the calculation to maintain precision.
- Failure to check work: Skipping the step of reviewing calculations increases the risk of missing simple mistakes. Always verify your results to confirm accuracy.
Improving Accuracy in Calculations
Here are some strategies to improve the accuracy of your geometric calculations:
- Take your time: Rushing through problems increases the likelihood of mistakes. Take the necessary time to understand each step and ensure everything is calculated correctly.
- Use a calculator carefully: If using a calculator, make sure that each entry is correct and that you use the correct order of operations.
- Work in stages: Break down complex problems into smaller steps. This makes it easier to identify any errors that may occur at each stage.
- Double-check measurements: Verify all given dimensions and angles before starting any calculations to ensure the accuracy of the data.
Ultimately, accuracy is key to solving any problem involving measurements and shapes. By focusing on precision, double-checking your work, and being mindful of common errors, you can ensure that your calculations are correct and reliable.
Using Geometry Formulas in Practice B

In any mathematical problem involving shapes and measurements, applying the correct formulas is essential for obtaining accurate results. These formulas serve as the foundation for solving various types of problems, from calculating areas and volumes to determining angles and distances. By familiarizing yourself with the appropriate formulas and understanding when to apply them, you can streamline the problem-solving process and achieve reliable solutions.
Whether you’re working with basic figures such as triangles and rectangles, or more complex shapes like polygons or circles, knowing how to properly use these mathematical tools is crucial. The key is to recognize which formula applies to the situation at hand and to use it in conjunction with the given measurements to find the desired quantity.
Key Formulas to Know
Here are some commonly used formulas that are useful in solving various problems:
- Area of a Rectangle: Area = length × width
- Area of a Triangle: Area = 1/2 × base × height
- Area of a Circle: Area = π × radius²
- Perimeter of a Rectangle: Perimeter = 2 × (length + width)
- Pythagorean Theorem: c² = a² + b², used for right triangles to find the hypotenuse
Applying Formulas Effectively
To successfully apply formulas, it’s important to follow a systematic approach:
- Identify the shape: Start by identifying the type of shape or figure in the problem. This will help you select the right formula for the calculations.
- Gather known values: Extract all the necessary measurements from the problem statement. These could include lengths, widths, heights, radii, or angles, depending on the shape.
- Substitute values into the formula: Carefully insert the known values into the appropriate formula. Pay attention to units and make sure everything is consistent.
- Perform the calculations: Carry out the arithmetic to solve for the unknown value. Be mindful of rounding and always double-check your calculations for accuracy.
Mastering these formulas is essential for solving problems efficiently and correctly. Once you become familiar with the formulas and develop a strong understanding of how to apply them, you’ll be able to tackle a wide variety of problems with confidence.
Strategies to Improve Geometry Problem-Solving
Improving your problem-solving skills in mathematical challenges involves developing a strategic approach that helps you break down complex problems into manageable steps. Success lies in understanding the core principles, practicing consistently, and applying effective methods to arrive at accurate solutions. By refining your techniques, you can enhance both your speed and accuracy in solving a wide range of problems.
To solve problems efficiently, it’s essential to build a strong foundation of concepts and employ logical steps to reach the desired outcome. This involves recognizing patterns, mastering key formulas, and approaching each problem systematically. Below are several strategies to help improve your problem-solving abilities.
Effective Problem-Solving Strategies
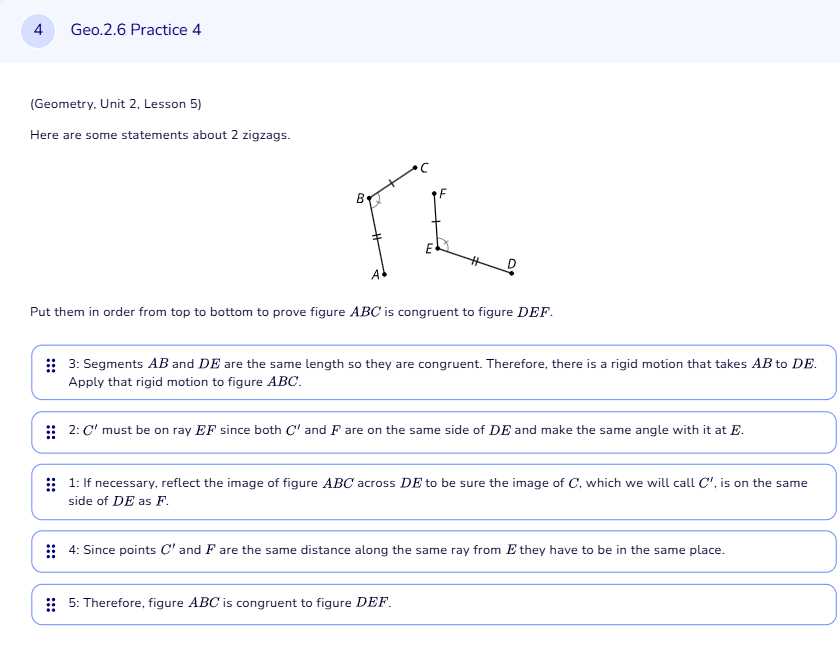
Consider incorporating the following approaches to improve your performance:
- Understand the Problem Thoroughly: Before jumping into calculations, take time to read and understand the problem. Identify what is given, what needs to be found, and any relationships between the variables.
- Visualize the Problem: Drawing diagrams or graphs can provide a clearer understanding of the situation. Visualizing the problem helps in identifying key relationships and applying appropriate formulas.
- Break the Problem into Smaller Parts: Complex problems can often be overwhelming. Break them down into simpler steps or smaller components. Solve each part individually and then combine the results for the final answer.
- Use Logical Reasoning: Apply deductive reasoning to determine how different elements of the problem are related. Ensure each step follows logically from the previous one to avoid errors.
- Check Your Work: After solving, review your calculations and verify that the answer makes sense. Cross-checking can help you spot mistakes and ensure the solution is correct.
Practice and Persistence
Practice is essential for improving your problem-solving abilities. The more problems you solve, the more familiar you’ll become with the types of challenges you face. Try to solve a variety of problems to build confidence and expand your skills. Over time, persistence and experience will lead to quicker and more accurate solutions.
By implementing these strategies and regularly practicing problems, you’ll develop the skills necessary to solve complex tasks with confidence and ease.
Explaining the Role of Angles in Geometry
Angles play a crucial role in understanding shapes and their properties, as they help define the relationships between different lines, planes, and figures. By studying angles, we gain insights into how objects interact with each other in space. Angles serve as the foundation for numerous geometric principles, from basic constructions to more advanced problem-solving techniques. Understanding how angles behave and how they are used in various scenarios is essential for mastering the subject.
In many mathematical tasks, angles help to measure and quantify the rotation between two intersecting lines or surfaces. This measurement is pivotal in tasks such as determining the dimensions of shapes, calculating area, or solving for unknown values in equations. The classification of angles–whether acute, obtuse, right, or reflex–also provides important information for geometric analysis and proofs.
Types of Angles
Different types of angles are fundamental in geometric calculations. Below are the common types of angles and their characteristics:
| Angle Type | Description |
|---|---|
| Acute Angle | An angle smaller than 90 degrees. |
| Right Angle | An angle exactly equal to 90 degrees. |
| Obtuse Angle | An angle greater than 90 degrees but less than 180 degrees. |
| Reflex Angle | An angle greater than 180 degrees but less than 360 degrees. |
| Straight Angle | An angle exactly equal to 180 degrees. |
Understanding these different types of angles allows for more accurate problem solving and better interpretation of geometric concepts. The role of angles is not only limited to basic geometry but also extends to real-world applications, such as architecture, engineering, and design, where precise measurements are critical.
How to Check Your Work
Verifying the accuracy of your calculations and solutions is an essential step in mathematical problem-solving. Often, errors can occur during the process of reasoning or when applying formulas, so it’s important to follow a systematic approach to check your work. By thoroughly reviewing each step and making sure that every detail is correct, you can ensure that your conclusions are valid.
Start by checking the logic behind your approach. Make sure the steps you followed are consistent with the problem’s requirements. If you used a formula, ensure that you applied it correctly, with the right values in place. Re-evaluating your work from a different perspective can also help spot potential mistakes you might have missed initially.
Steps to Review Your Work
- Double-check your calculations: Ensure all arithmetic operations, such as addition, subtraction, multiplication, and division, are correct.
- Reassess your assumptions: Review the problem’s conditions and confirm you haven’t made any incorrect assumptions about the situation.
- Compare with examples: Refer to similar problems or examples to see if your approach aligns with the standard method for solving such problems.
- Revisit your final answer: Does your final result make sense in the context of the problem? Look for any logical inconsistencies.
Using Technology for Validation
In addition to manually checking your work, you can use various online tools or software to verify your results. Many calculators or geometric tools provide instant feedback, which can help confirm that your solutions are correct or highlight areas where you might need further review. These tools can be especially useful for more complex problems where manual verification may be time-consuming or prone to error.
By following these steps, you can boost your confidence in the accuracy of your work and minimize the chances of making avoidable mistakes.
Preparing for Tests with Practice B
Effective preparation for assessments requires a deep understanding of the material, and consistent practice is key to mastering concepts. One of the most effective ways to prepare is through targeted exercises that reflect the test’s format and difficulty level. By engaging in comprehensive practice, you can strengthen your problem-solving skills and improve your ability to apply theoretical knowledge in practical situations.
Working through practice sets not only helps reinforce the concepts but also builds your confidence. It’s essential to focus on areas where you feel less secure and tackle problems that challenge your understanding. Repeated practice of different problem types will make you more familiar with various approaches, and this familiarity can improve your overall performance during the test.
Here are a few strategies to optimize your preparation:
- Review key concepts: Ensure you fully understand the foundational ideas before moving on to more complex topics.
- Work on time management: Set a timer and simulate test conditions to practice solving problems within a given timeframe.
- Identify common mistakes: Pay attention to any recurring errors and make an effort to address them before the test.
- Track your progress: Keep a log of the types of problems you’ve completed and focus on areas that need improvement.
By dedicating time to consistent practice and implementing these strategies, you will be well-prepared to tackle your assessment with confidence and precision.