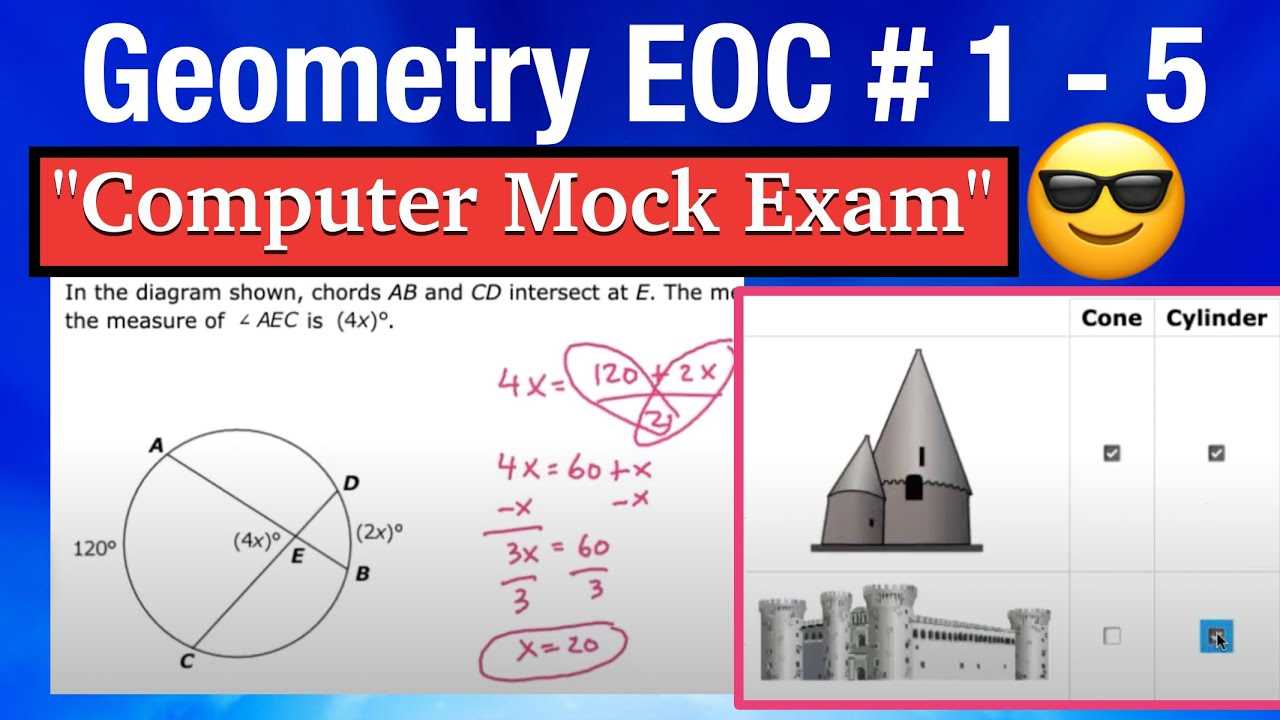
Understanding the principles behind shapes, sizes, and spatial relationships is key to mastering various math challenges. By breaking down complex problems into simpler components, students can develop a deeper comprehension of the subject matter and improve their overall performance. Through practice and familiarity with different problem-solving techniques, anyone can gain confidence and achieve success in this area.
Whether it’s working through puzzles or tackling specific exercises, learning to navigate mathematical concepts is essential for both academic achievement and real-world applications. The ability to recognize patterns, apply formulas, and reason logically opens doors to a wide range of fields, from engineering to architecture, and even everyday decision-making.
In this guide, we will explore the strategies and solutions that will help you tackle mathematical challenges effectively. By honing your skills and familiarizing yourself with key principles, you can make learning more efficient and enjoyable.
Math Nation Geometry Answers Explained
When it comes to solving problems involving shapes, sizes, and spatial reasoning, having a clear understanding of the fundamental principles is crucial. These concepts allow individuals to break down complex problems into manageable parts, making them easier to solve. The process of finding the right solution often involves recognizing key elements and applying the appropriate strategies.
For students aiming to improve their skills, it is essential to familiarize themselves with the following steps:
- Identifying the given information and what needs to be solved
- Choosing the correct formula or theorem based on the problem
- Performing the necessary calculations while keeping track of units and dimensions
- Verifying the solution to ensure it makes sense in the context of the problem
By following these steps, learners can approach even the most challenging problems with confidence. The key is consistent practice and learning from previous mistakes. As one progresses, the application of these concepts will become more intuitive, and solving new problems will feel less daunting.
Understanding the structure of mathematical exercises can also help to anticipate common pitfalls and avoid errors. The more familiar one becomes with typical problem types, the quicker they can identify the best approach and arrive at the correct solution. It’s not just about memorizing formulas–it’s about developing the analytical skills necessary to solve a wide range of puzzles effectively.
How to Solve Geometry Problems Effectively
Approaching spatial reasoning challenges requires both systematic planning and an understanding of core principles. To solve problems efficiently, it’s important to break them down into smaller, more manageable steps. The process involves recognizing the key elements of the problem, applying relevant formulas or theories, and verifying the results. With the right strategy, complex problems can be solved more quickly and accurately.
Step-by-Step Problem-Solving Approach
Here are some effective strategies to follow when tackling any spatial reasoning challenge:
- Read the Problem Carefully: Begin by understanding the problem’s context and identifying the unknowns.
- Identify Key Elements: Look for key data, shapes, and relationships that will help guide the solution.
- Select the Right Method: Choose the appropriate formula, theorem, or strategy based on the problem’s requirements.
- Work Through the Calculations: Execute the necessary steps methodically, checking each stage for accuracy.
- Verify the Solution: Cross-check the solution to ensure it is reasonable and satisfies the original conditions.
Common Pitfalls to Avoid
When solving spatial challenges, there are a few common mistakes to be aware of:
- Ignoring or misinterpreting key information from the problem statement.
- Relying on incorrect formulas or making assumptions without verifying them.
- Forgetting to consider the units or dimensions, which can lead to incorrect results.
- Failing to check the solution for reasonableness or practical feasibility.
By practicing these steps consistently and learning from past mistakes, you can develop stronger problem-solving skills and become more efficient in handling complex challenges.
Key Concepts in Geometry to Master
To excel in problems involving shapes, sizes, and their relationships, it is essential to grasp several core ideas that form the foundation of the subject. Mastering these concepts allows you to approach a wide range of challenges with confidence and precision. The ability to apply fundamental principles effectively is key to solving even the most complex tasks.
Essential Shapes and Their Properties
Understanding the characteristics of basic figures is crucial for solving problems accurately. Here are some of the key elements to focus on:
- Triangles: Recognize different types (equilateral, isosceles, scalene) and their properties, such as angles and side relationships.
- Quadrilaterals: Study the properties of squares, rectangles, trapezoids, and parallelograms, focusing on sides, angles, and symmetry.
- Circles: Learn about radius, diameter, circumference, and how to calculate area and arc length.
Spatial Relationships and Theorems
In addition to understanding individual shapes, it’s important to be familiar with key theorems and relationships that help solve problems:
- Pythagorean Theorem: Understand how to calculate the length of the sides of right triangles.
- Congruence and Similarity: Know the conditions under which shapes are congruent or similar and how to use these properties in problem solving.
- Area and Volume: Master formulas for calculating the area of 2D shapes and the volume of 3D objects.
Mastering these key concepts is the first step toward becoming proficient in the subject and handling more advanced topics with ease.
Understanding the Basics of Shapes
To solve challenges involving space and form, it is essential to first understand the fundamental structures that make up all complex shapes. Whether it’s a simple two-dimensional figure or a more intricate three-dimensional object, every form has its own set of defining properties. Recognizing these basic elements will help in applying formulas and solving problems with accuracy.
Shapes can generally be classified into two categories: flat figures that exist in a single plane and solid objects that occupy three-dimensional space. Each category has its own unique set of properties that govern its area, perimeter, volume, and surface area. Mastering the basic shapes is the first step toward solving more advanced problems efficiently.
Key Two-Dimensional Shapes
Flat figures, or 2D shapes, are essential in understanding spatial relationships and measurement. Here’s an overview of common two-dimensional shapes and their key properties:
| Shape | Properties | Formula (Area) |
|---|---|---|
| Square | All sides equal, all angles 90° | side² |
| Rectangle | Opposite sides equal, all angles 90° | length × width |
| Circle | All points equidistant from the center | π × radius² |
| Triangle | Three sides, three angles | 1/2 × base × height |
Each of these shapes has unique properties that are vital when solving related problems. By understanding their characteristics, you can easily calculate the necessary dimensions and solve for unknown values.
Using Formulas for Geometry Solutions
To solve problems involving space, dimensions, and various shapes, applying the correct formulas is essential. These mathematical expressions help quantify key aspects of figures, from areas and perimeters to volumes and surface areas. Understanding how and when to use specific formulas enables faster and more accurate problem-solving.
Formulas are designed to simplify complex calculations. Whether you’re dealing with simple two-dimensional figures or more intricate three-dimensional objects, each formula serves a specific purpose that relates directly to the properties of the shape. With regular practice, recalling and applying these formulas becomes more intuitive.
Common Formulas for Two-Dimensional Figures
Here are some key formulas for basic flat shapes:
- Rectangle: Area = length × width
- Square: Area = side²
- Triangle: Area = 1/2 × base × height
- Circle: Area = π × radius², Circumference = 2 × π × radius
Key Formulas for Three-Dimensional Shapes
For solid objects, these formulas are commonly used:
- Cube: Volume = side³, Surface Area = 6 × side²
- Sphere: Volume = 4/3 × π × radius³, Surface Area = 4 × π × radius²
- Cylinder: Volume = π × radius² × height, Surface Area = 2 × π × radius × (radius + height)
By mastering these formulas and understanding their applications, you can efficiently calculate the unknowns in a variety of spatial problems.
Common Mistakes in Geometry and Fixes
When working with shapes, sizes, and spatial relationships, it’s easy to make mistakes that can lead to incorrect solutions. These errors often stem from misunderstandings of key concepts, misapplying formulas, or overlooking important details in the problem. Recognizing and correcting these common mistakes is essential for improving accuracy and solving problems effectively.
Below are some of the most frequent mistakes students make when dealing with spatial problems, along with tips on how to avoid or fix them:
1. Incorrect Use of Formulas
Problem: Using the wrong formula for a shape or applying a formula incorrectly is one of the most common errors.
Fix: Always double-check the properties of the shape or object you’re working with to ensure you’re using the correct formula. For example, be sure to differentiate between surface area and volume formulas for 3D objects.
2. Forgetting to Account for Units
Problem: Failing to convert or properly handle units (such as inches to centimeters) can lead to inaccurate results, especially when dealing with measurements of length, area, or volume.
Fix: Always ensure that units are consistent before performing calculations. Convert units where necessary, and remember to apply the correct unit for the final answer (e.g., square units for area, cubic units for volume).
3. Misinterpreting the Problem
Problem: Sometimes, a question may include extra or misleading information that causes confusion. Misunderstanding what is being asked can lead to mistakes in the approach or solution.
Fix: Read the problem carefully, highlighting key information. Identify what is being asked and ensure that the data provided matches the context of the question. Break down the problem into smaller parts if necessary.
4. Rounding Too Early
Problem: Rounding intermediate results before completing all steps can lead to small errors that compound, resulting in an inaccurate final answer.
Fix: Avoid rounding numbers until the very end of the calculation process. Use exact values for intermediate steps, and round only once you have reached the final answer.
By recognizing these common pitfalls and applying the fixes above, you’ll be able to improve your problem-solving approach and achieve more accurate results.
Practice Problems to Improve Skills
One of the best ways to enhance your understanding of shapes, measurements, and spatial relationships is through consistent practice. Solving problems regularly allows you to reinforce key concepts, develop problem-solving strategies, and increase your overall confidence. By working through a variety of exercises, you’ll be better equipped to handle more complex challenges when they arise.
Here are a few practice problems that can help sharpen your skills in solving spatial-related questions:
Problem 1: Area of a Triangle
Calculate the area of a triangle with a base of 8 cm and a height of 5 cm.
Problem 2: Surface Area of a Cube
Find the surface area of a cube with a side length of 6 cm.
Problem 3: Volume of a Cylinder
Determine the volume of a cylinder with a radius of 4 cm and a height of 10 cm.
Problem 4: Perimeter of a Rectangle
What is the perimeter of a rectangle that has a length of 12 cm and a width of 7 cm?
Problem 5: Angle Sum of a Polygon
Find the sum of the interior angles of a hexagon.
By working through these types of problems, you can reinforce your understanding of essential concepts and improve your ability to solve complex problems with ease.
Why Geometry Matters in Real Life
Understanding shapes, space, and measurement is crucial in many aspects of daily life. From designing buildings to navigating through a city, the concepts used to calculate areas, distances, and volumes are constantly applied in real-world situations. This branch of knowledge provides practical solutions to everyday challenges, enabling us to interact with and manipulate our environment more effectively.
In addition to its obvious applications in fields like construction and design, spatial reasoning is also vital in fields such as engineering, technology, and even art. Mastering these concepts not only helps in technical tasks but also encourages critical thinking and problem-solving skills.
Applications in Construction and Architecture
In construction and architecture, precise measurements and calculations are essential for designing structures that are safe, functional, and aesthetically pleasing. Builders and architects use spatial reasoning to plan the layout of rooms, calculate the amount of materials needed, and ensure that all parts fit together properly.
Real-Life Examples
Here are some areas where spatial concepts are applied:
| Field | Application |
|---|---|
| Architecture | Designing building layouts, determining dimensions, and calculating structural integrity. |
| Engineering | Designing machines, bridges, and other systems that require precise spatial measurements. |
| Art | Creating accurate drawings, sculptures, and other works that require a sense of proportion and space. |
| Technology | Programming virtual environments and working with computer graphics that rely on precise measurements. |
By grasping the concepts behind space and measurements, we gain the tools needed to solve problems that are vital in both professional and everyday contexts. These skills are foundational not only for various careers but also for making informed decisions in practical situations.
Tips for Studying Geometry More Efficiently
Studying spatial concepts and shapes can sometimes feel challenging, but with the right strategies, you can significantly improve your understanding and problem-solving skills. Organizing your study routine, practicing regularly, and focusing on key principles are all essential for mastering this subject. By adopting effective study habits, you can make learning both more efficient and enjoyable.
Here are some practical tips to help you study more effectively:
1. Break Down Complex Problems
When facing a difficult problem, try breaking it down into smaller, more manageable steps. This will help you focus on each part of the problem individually and avoid feeling overwhelmed. Tackle one element at a time, such as calculating areas before moving on to volume or perimeter.
2. Use Visual Aids
Visualizing shapes and diagrams is essential when studying spatial concepts. Draw diagrams to better understand how different components of a figure relate to each other. Use colored pencils or highlighters to differentiate parts of the figure, helping you keep track of the information.
3. Practice Regularly
The more problems you solve, the more comfortable you’ll become with the concepts. Consistent practice is key to mastering the material. Work through practice problems from textbooks, online resources, or past exams to reinforce your skills.
4. Focus on Understanding, Not Memorization
While memorizing formulas can be useful, it’s more important to understand why a formula works the way it does. Understanding the logic behind calculations will help you apply concepts to different scenarios more effectively.
5. Teach What You’ve Learned
One of the best ways to solidify your knowledge is by teaching someone else. If you can explain a concept clearly to others, it shows that you’ve fully grasped it. Try teaching a friend or explaining a concept out loud to yourself as if you were the instructor.
By incorporating these strategies into your study routine, you’ll be able to improve your grasp on spatial concepts and become more confident in solving problems efficiently.
Helpful Online Resources for Geometry
The internet offers a wealth of resources to help you grasp spatial concepts and sharpen your problem-solving skills. Whether you’re looking for interactive lessons, practice problems, or visual explanations, there are numerous platforms that can make studying much more engaging and effective. These online tools provide both free and paid options, catering to different learning preferences and levels of understanding.
Here are some useful online platforms to explore:
1. Interactive Learning Platforms
- Khan Academy – Offers free video lessons and exercises on various topics, including spatial reasoning and problem-solving.
- IXL – A subscription-based site that provides personalized practice problems and detailed explanations.
- Brilliant – Offers interactive courses and problem sets designed to deepen understanding of concepts and develop critical thinking skills.
2. Practice Problem Websites
- Geometry Monster – A site filled with practice problems, ranging from basic to advanced, with step-by-step solutions.
- Art of Problem Solving – Offers a variety of problem-solving resources, including an online community where you can discuss strategies with other learners.
- PatrickJMT – Provides free video tutorials that walk you through specific problems, explaining each step along the way.
Using these platforms, you can complement your textbook learning with additional practice, visual aids, and explanations, all of which will help strengthen your understanding of key concepts.
Geometry Tools That Can Aid Learning
Effective learning often requires the right tools to simplify complex concepts and improve understanding. There are several resources and devices that can make studying shapes, space, and measurements more intuitive. From physical tools to digital apps, these tools can help you visualize problems, practice calculations, and enhance your problem-solving abilities.
1. Digital Tools and Apps
Technology has provided a wide range of digital tools designed to aid in the study of spatial reasoning and measurement. Here are some popular options:
- GeoGebra – A powerful tool that allows users to visualize geometric figures, graphs, and equations interactively. Perfect for experimenting with different shapes and exploring their properties.
- Desmos – Known for its graphing capabilities, Desmos can help students understand the relationship between shapes and equations.
- Microsoft Math Solver – An app that offers step-by-step solutions for problems related to space and measurements, helping students better understand the process of solving geometric problems.
2. Physical Tools for Hands-On Learning
For those who prefer a tactile learning experience, physical tools can be highly beneficial in visualizing geometric concepts. Here are some examples:
- Protractors – Essential for measuring and drawing angles accurately, helping students understand angle relationships and their applications in real-world scenarios.
- Compasses – Used for drawing perfect circles, which is useful in studying properties of circular shapes and understanding their various formulas.
- Geometric Solids – Physical models of 3D shapes like cubes, spheres, and pyramids can help learners visualize and manipulate solid objects.
Incorporating these tools into your study routine can provide a more hands-on and engaging approach to mastering spatial concepts and their real-world applications.
Understanding Angles and Their Applications
Angles are a fundamental concept that plays a critical role in various aspects of science, engineering, art, and architecture. They are formed when two lines meet at a point, and their measurement reveals the amount of rotation between these lines. Understanding angles not only helps solve geometric problems but also applies to real-world scenarios, from constructing buildings to designing computer graphics.
1. Types of Angles
Angles can be categorized based on their measurement. The most common types are:
- Acute Angle – An angle less than 90 degrees.
- Right Angle – An angle exactly 90 degrees, often represented by a square corner.
- Obtuse Angle – An angle greater than 90 degrees but less than 180 degrees.
- Reflex Angle – An angle greater than 180 degrees but less than 360 degrees.
2. Real-World Applications of Angles
Angles are not just theoretical constructs; they are used in various practical fields. Some examples include:
- Construction and Architecture – Architects and builders use precise angle measurements to ensure that structures are stable and aesthetically pleasing.
- Navigation – Angles are crucial in determining direction and positioning, especially in sailing, aviation, and GPS technology.
- Art and Design – Artists and designers utilize angles to create perspective, symmetry, and balance in their work.
- Engineering – Engineers apply angle principles in the design of mechanical parts, electrical circuits, and vehicles.
By understanding how angles work and their practical applications, you can gain a deeper appreciation for the world around you and enhance your problem-solving skills in various fields.
How to Approach Word Problems in Geometry
Word problems in spatial reasoning often seem challenging at first, as they require translating real-world scenarios into mathematical concepts. The key to solving these problems effectively lies in understanding the situation described and identifying the relevant information. By breaking the problem down into manageable steps and using appropriate techniques, you can approach even the most complex situations with confidence.
1. Read and Understand the Problem
The first step in tackling word problems is to carefully read the entire question. Make sure you understand the context and identify key details such as measurements, relationships between objects, and what is being asked. Look for important clues such as:
- Numbers – Pay attention to any given values, such as lengths, areas, or angles.
- Keywords – Words like “sum,” “difference,” or “perpendicular” can indicate the mathematical operations or relationships you need to consider.
- Diagrams – Often, problems include visual aids that can help you visualize the situation and make connections.
2. Plan Your Approach
Once you’ve understood the problem, the next step is to plan how to solve it. Break the problem into smaller parts and determine which mathematical formulas or concepts apply. For example:
- If you’re dealing with areas or perimeters, recall the formulas for basic shapes like circles, squares, or triangles.
- Consider using algebra to express unknowns and solve for them step-by-step.
- Don’t forget to check for any special relationships between objects, such as parallel lines, symmetry, or angles that are known to be equal.
By following a logical sequence and utilizing the correct tools, you can systematically solve word problems and build your problem-solving skills in the process.
Exploring 2D and 3D Geometry Differences
The study of shapes and their properties can be divided into two primary categories: two-dimensional (2D) and three-dimensional (3D). While both involve understanding space, their characteristics and applications differ significantly. In 2D, shapes lie flat on a surface, while in 3D, objects have depth, giving them volume. This distinction influences how we measure, calculate, and interpret these figures in various fields such as architecture, art, and engineering.
1. Key Differences Between 2D and 3D
The most obvious difference between these two types of figures is the number of dimensions they occupy. Some important distinctions include:
- Dimensions – 2D figures exist on a flat plane, while 3D objects extend into depth, creating a volumetric space.
- Measurement – In 2D, we focus on properties such as area and perimeter, whereas in 3D, we calculate volume and surface area.
- Shapes vs Objects – 2D refers to simple shapes like squares, triangles, and circles, while 3D refers to solids like cubes, spheres, and pyramids.
2. Real-World Applications of 2D and 3D
The distinction between 2D and 3D is not just theoretical; it has practical applications across various fields:
- 2D Applications – Used in graphic design, blueprints, maps, and printing where flat representations are needed.
- 3D Applications – Important in fields like product design, animation, construction, and virtual reality, where depth and volume are crucial.
Understanding these differences helps in solving problems, designing products, and even visualizing complex structures in the real world. As you delve deeper into these concepts, you’ll gain a clearer perspective on how two-dimensional and three-dimensional shapes influence both theoretical studies and practical tasks.
Geometry Vocabulary You Need to Know
Understanding the terminology related to shapes, spaces, and measurements is essential when studying spatial concepts. Whether you’re solving problems or learning new concepts, having a solid grasp of key terms will enhance your ability to navigate complex topics. In this section, we will cover some of the most important terms and definitions that will help you build a strong foundation for further study.
1. Basic Terms and Definitions
To begin with, it’s important to familiarize yourself with the following common terms:
- Point – A location in space with no dimensions, typically represented by a dot.
- Line – A straight path that extends infinitely in both directions, containing an infinite number of points.
- Angle – The space between two intersecting lines, measured in degrees.
- Plane – A flat surface that extends infinitely in all directions.
- Shape – The outline or form of an object, typically defined by its sides and angles.
2. Advanced Vocabulary for 3D Objects
For more complex studies, it’s essential to expand your vocabulary to include terms related to three-dimensional objects:
- Volume – The amount of space an object occupies, measured in cubic units.
- Surface Area – The total area of the outer surfaces of a 3D object.
- Solid – A three-dimensional object that has length, width, and depth, such as cubes, spheres, and pyramids.
- Edge – The line where two surfaces of a 3D object meet.
- Face – A flat surface that forms part of the boundary of a 3D object.
Mastering this vocabulary is crucial for understanding and communicating effectively in spatial studies. As you encounter more complex problems and concepts, a solid grasp of these terms will serve as the foundation for solving and explaining them accurately.
The Role of Proofs in Geometry
In any field that involves reasoning and logical deduction, proving statements is a critical step in validating and solidifying concepts. In spatial studies, proofs serve as the foundation for understanding the relationships between shapes, angles, and other elements. They allow students to move from assumptions and observations to logical conclusions, ensuring that the results are universally true, not just specific to one scenario.
Proofs are used to establish the validity of geometric properties, theorems, and formulas. By demonstrating that a conclusion follows from a set of accepted axioms or previously established results, proofs help create a structured and reliable body of knowledge. This process not only confirms the correctness of ideas but also deepens the understanding of how different concepts are interconnected.
There are various types of proofs used in spatial reasoning, each with its own approach and purpose. Some of the most common methods include:
- Direct Proofs – These proceed step by step from known facts to reach the desired conclusion.
- Indirect Proofs – Also known as proof by contradiction, this method assumes the opposite of what you want to prove and shows that this assumption leads to a contradiction.
- Constructive Proofs – These involve creating an example or specific case that demonstrates the truth of a statement.
- Proofs by Mathematical Induction – Used for statements involving integers or sequences, this method proves a base case and shows that if one case holds, the next one does as well.
Understanding how to construct and interpret proofs is essential for students aiming to master spatial reasoning. They not only provide certainty in calculations but also sharpen analytical thinking, which is valuable in various real-world applications.
Preparing for Geometry Exams Successfully
Success in exams that focus on spatial reasoning and shape analysis depends on both understanding the material and applying that knowledge efficiently under time pressure. To ensure you are fully prepared, it’s essential to approach your studies strategically, focusing not only on mastering core concepts but also on practicing problem-solving techniques that may appear in the test. Proper preparation combines active learning, consistent practice, and effective time management to ensure you’re ready for any challenge.
Here are several tips to help you prepare for your upcoming spatial reasoning exam:
Review Key Concepts and Theorems
Focus on the fundamental principles that are most likely to appear in your exam. Make sure you understand the core properties of shapes, angles, and measurements. Review definitions, formulas, and important theorems. Writing out and summarizing the key ideas in your own words can be a powerful method to reinforce your understanding.
Practice with Sample Problems
The best way to solidify your understanding is by solving a variety of practice problems. Try to work through as many different types of questions as you can, from basic calculations to more complex proofs. By practicing consistently, you’ll improve your ability to apply concepts efficiently and accurately during the exam.
Incorporating timed practice sessions can also help you manage your time effectively on the actual exam day. Being familiar with the structure and types of questions you might encounter will allow you to feel more confident and perform better when it counts.