
Preparing for a challenging test requires more than just reviewing notes. It involves honing specific skills through practice and applying your knowledge under timed conditions. Engaging with realistic practice questions allows you to identify weak points and build confidence before the actual assessment.
By focusing on a variety of problem types, you can improve your ability to analyze data, interpret results, and make sound conclusions. These exercises offer the opportunity to familiarize yourself with common question formats and enhance your critical thinking.
Efficient practice not only boosts accuracy but also improves your ability to recall essential formulas and methods during the actual test. Repetition and understanding are key to mastering the material and performing well when it counts the most.
Practice Test for Statistical Concepts
Engaging in practice sessions helps solidify your understanding and prepares you for real-world assessments. By working through various problems, you enhance your ability to tackle different question formats and refine your problem-solving skills. This section presents a series of exercises designed to test your knowledge and provide immediate feedback on your performance.
Here are some areas covered in the test:
- Data interpretation and analysis
- Probability calculations and distributions
- Hypothesis testing and decision-making
- Graphical representation of data
- Descriptive measures and their applications
After completing each question, review the correct solutions to understand where you may have made errors and to reinforce the correct approach. This process is vital for improving accuracy in your future attempts.
By consistently working through these practice problems, you’ll develop a deeper comprehension of the material and build the skills necessary for tackling more complex tasks confidently.
Why Practice Tests Are Essential

Repetition and practical application are key to mastering any subject. Engaging in simulated assessments helps reinforce concepts, identify areas of weakness, and improve overall performance. By exposing yourself to a variety of question types and scenarios, you prepare not only for the content but also for the format and pressure of real assessments.
Improving Time Management
Working through mock tasks under timed conditions develops your ability to manage time effectively during the actual challenge. You’ll learn to prioritize questions, pace yourself, and ensure you complete all sections. These skills are crucial for reducing stress and optimizing performance on the day of the test.
Boosting Confidence and Reducing Anxiety
Frequent practice leads to greater familiarity with the subject, which, in turn, builds confidence. The more you practice, the less daunting the real test becomes. This reduces test anxiety and allows you to approach the assessment with a calm and focused mindset.
Key Concepts to Focus On
Mastering the core principles of any subject requires a solid understanding of the foundational concepts. By focusing on these key areas, you ensure a strong grasp of the material, which will improve your ability to solve problems accurately and efficiently. In this section, we highlight the most important concepts to prioritize during your preparation.
- Data Collection and Interpretation: Learn how to gather relevant information and interpret it effectively.
- Probability and Distributions: Understand how different distributions work and how to calculate probabilities.
- Central Tendency Measures: Familiarize yourself with mean, median, and mode, and when to apply each.
- Hypothesis Testing: Focus on formulating hypotheses and understanding how to test them with appropriate methods.
- Correlation and Regression: Grasp the relationship between variables and how to model their interactions.
- Sampling Methods: Study the different techniques for collecting representative data and their applications.
By dedicating your attention to these core topics, you’ll be well-equipped to handle a wide range of problems and scenarios, laying the groundwork for long-term success in the field.
Understanding Different Types of Questions
Each test presents various question formats, and understanding how to approach them is crucial for success. By familiarizing yourself with these different types, you can develop strategies for tackling each one efficiently, ensuring you don’t miss any key points. Whether it’s solving problems or interpreting data, each question requires a distinct approach.
Multiple-choice questions are designed to test your knowledge of key concepts quickly. These require you to identify the correct option from a set of possibilities. Here, it’s important to eliminate the obviously incorrect answers and focus on the most plausible choices.
Open-ended questions assess your ability to explain concepts or work through a problem in detail. These require clear, structured responses that demonstrate your understanding of the material. Focus on presenting your reasoning logically and back up your statements with solid examples.
Problem-solving tasks often involve applying formulas and techniques to arrive at a solution. These questions assess your ability to analyze a situation and use appropriate methods to find an answer. Pay close attention to every detail in the problem, as small mistakes can lead to incorrect conclusions.
By practicing each question type, you’ll become more adept at recognizing patterns and approaching problems efficiently during the actual test.
How to Approach Multiple-Choice Questions

Multiple-choice questions are a common format designed to assess your knowledge quickly and efficiently. The key to answering these correctly lies in your ability to read carefully, eliminate wrong choices, and select the most appropriate option. Developing a systematic approach can greatly improve your performance in this section.
First, read the question thoroughly to understand what it is asking. Sometimes, key details are embedded in the wording that can help guide you to the right answer. Once you understand the question, skim through all the options before selecting one.
Next, eliminate the answers that are obviously incorrect. Look for answers that seem too extreme or unrelated to the question. This narrowing down process increases your chances of selecting the correct option even if you’re unsure.
If you’re still uncertain, try to recall relevant concepts or formulas that might help guide your choice. Trust your instincts when you feel confident, but avoid rushing; take the time to consider each option carefully.
Finally, review your selections if time permits. Double-check the wording of the question and make sure your answer aligns with what’s being asked.
Solving Descriptive Statistics Problems
Descriptive analysis involves summarizing and interpreting data in a way that reveals key trends and patterns. To solve problems in this area, it’s important to understand how to extract meaningful insights from raw data using central measures and variability indicators. This section will guide you through the essential steps to solve these types of problems effectively.
Understanding Key Measures

The first step in solving descriptive problems is identifying the relevant measures. These include the mean, median, and mode, which describe the center of your data. Knowing when to use each measure is crucial: the mean is useful for symmetric data, while the median is more appropriate for skewed distributions. The mode helps identify the most frequent value in a dataset.
Assessing Variability
Next, it’s essential to evaluate the spread or dispersion of the data. Common measures of variability include the range, variance, and standard deviation. The range gives a quick idea of how spread out the data is, but variance and standard deviation provide more detailed insight into the overall variability. Understanding these measures allows you to interpret how much individual values deviate from the average.
By mastering these steps, you can solve descriptive problems with greater ease and clarity, ensuring a thorough understanding of the data at hand.
Interpreting Probability Questions Effectively
Probability-related questions require careful analysis to understand the likelihood of different outcomes. These types of problems often involve calculating chances based on given data or scenarios, and being able to break down the question into manageable parts is crucial. The key to success lies in recognizing the type of probability problem you are dealing with and applying the right approach.
Breaking Down the Problem

Start by identifying the key components of the problem, such as the total number of possible outcomes and the specific outcomes you’re interested in. This helps you focus on the relevant data and avoid confusion when working through the calculations. Organizing the information clearly is essential for understanding how to solve the problem.
Using Probability Tables
In some cases, using tables to organize the possible outcomes can be extremely helpful. These tables provide a clear overview of the various possibilities and help you visualize the problem more effectively. Below is an example of a probability table for a simple event:
| Outcome | Probability |
|---|---|
| Heads | 0.5 |
| Tails | 0.5 |
This simple table shows the possible outcomes of a coin toss and their respective probabilities. By organizing your data in a similar way, you can more easily calculate combined probabilities or analyze complex situations.
By breaking down the problem and organizing the information in a structured way, you can approach probability questions with greater confidence and accuracy.
Tips for Working with Hypothesis Testing
Hypothesis testing is a fundamental process in decision-making that involves evaluating claims or assumptions based on sample data. This procedure can seem complex, but breaking it down into clear steps makes it more manageable. Understanding the logic behind testing, choosing the right test, and interpreting results correctly are essential for success in this area.
Step-by-Step Approach
Start by clearly defining the null hypothesis (H₀) and the alternative hypothesis (H₁). These two statements represent opposing views, and your task is to assess which one is more supported by the evidence. The null hypothesis typically suggests that there is no effect or difference, while the alternative hypothesis proposes that there is a significant effect.
Once the hypotheses are defined, choose an appropriate test based on the data and the question at hand. This could involve comparing means, proportions, or relationships between variables. Ensure you understand the assumptions of the test and select the correct method for your data.
Interpreting Results

After conducting the test, you’ll obtain a p-value, which helps you determine whether to reject the null hypothesis. A low p-value (typically less than 0.05) indicates strong evidence against the null hypothesis, suggesting that the alternative hypothesis may be true. However, always consider the context and the effect size, as a statistically significant result does not always imply practical significance.
Additionally, understanding the power of the test is important. A test with low power may fail to detect a true effect, while a test with high power provides more reliable results. Be sure to check both the p-value and the context to draw meaningful conclusions.
Time Management Strategies for Exams
Effective time management during a test is crucial to ensure that you can complete all questions thoughtfully and accurately. Managing your time well allows you to stay calm under pressure and make the most of the time available. By prioritizing tasks and maintaining focus, you can increase your chances of success.
Pre-Exam Preparation
Time management starts before you even sit down for the test. Effective preparation ensures that you are ready to handle the time constraints on the day of the test.
- Create a study schedule: Break down your study material into manageable sections and allocate time for each topic. This will prevent last-minute cramming and allow for better retention of information.
- Practice under timed conditions: Simulate test conditions by practicing with a timer. This will help you get used to the time pressure and develop a sense of how much time to allocate to each section.
- Organize materials: Ensure that all the materials you need for the test are prepared in advance, so you don’t waste time looking for items on the day of the test.
During the Test
Once the test begins, it’s essential to stay organized and efficient. Here are some strategies to keep you on track:
- Read instructions carefully: Before starting, read all instructions thoroughly to avoid mistakes that can cost valuable time later.
- Prioritize questions: Quickly skim through the entire test to identify easy questions that you can answer immediately. This will build confidence and save time for more difficult questions later.
- Allocate time per section: Set a time limit for each section or set of questions. If you’re spending too long on a question, move on and come back to it later if time allows.
- Stay calm: If you feel yourself getting stuck or stressed, take a deep breath. Maintaining a calm mindset will help you think clearly and make better decisions under time pressure.
By implementing these strategies, you can ensure that you manage your time effectively, giving you the best chance to perform well and answer all questions accurately within the available time.
Common Mistakes in Statistics Exams
During assessments that test analytical skills and data interpretation, students often make a few critical errors that can negatively impact their performance. These mistakes can be attributed to various factors, such as misinterpreting the problem, rushing through calculations, or misunderstanding key concepts. By identifying and avoiding these common pitfalls, you can improve your chances of success and approach each question more confidently.
One frequent mistake is failing to read questions carefully. It’s easy to overlook crucial details in the problem statement, such as specific conditions or requirements. Skipping steps or not fully understanding what’s being asked can lead to incorrect answers, even if the underlying concepts are clear.
Another common issue is misapplying formulas or methods. When solving problems, it’s important to choose the correct approach based on the given data and the nature of the question. Using the wrong formula or method can lead to errors, even if the steps are followed correctly. It’s essential to review the concepts and methods thoroughly before attempting problems, and to recognize when a different approach is needed.
Lastly, students often make mistakes with rounding or handling decimal places. Incorrect rounding can throw off results, especially in calculations that involve multiple steps. Being precise and following the correct rounding rules ensures that the final answer is as accurate as possible.
By recognizing these mistakes and taking steps to avoid them, you can improve your problem-solving accuracy and perform more effectively in any assessment that involves data analysis and interpretation.
How to Review Your Answers After the Test
After completing an assessment, reviewing your responses is a crucial step that can enhance your understanding and performance in future tasks. Carefully revisiting your solutions allows you to identify errors, spot patterns in your thinking, and reinforce key concepts. This process can also help you build confidence in your abilities and prepare for upcoming challenges.
When reviewing, start by focusing on questions that seemed difficult or where you were unsure of the answer. Look for patterns in the types of mistakes you made–whether it’s misinterpreting a question, using the wrong method, or making calculation errors. Identifying these areas will help you pinpoint where further practice is needed.
Step-by-Step Review Process
Follow a structured approach when revisiting your work. Here are a few steps to ensure a thorough review:
| Step | Action |
|---|---|
| 1 | Check for obvious mistakes: Look for simple errors like incorrect calculations, missing steps, or overlooked details in the problem. |
| 2 | Analyze your reasoning: Examine how you approached each question. Were you using the correct formulas and methods? Were assumptions made that could have affected the result? |
| 3 | Cross-check answers: If possible, try solving the question again or use alternative methods to verify your solution. |
| 4 | Learn from mistakes: Identify any recurring errors and make note of areas where you need improvement or further practice. |
By taking the time to review your work, you not only correct errors but also gain deeper insights into your problem-solving approach. This reflective process helps you improve both your analytical skills and test-taking strategies for future assessments.
Improving Accuracy in Calculations
Achieving precision in mathematical computations is essential for producing correct results. Whether dealing with basic arithmetic or complex problem-solving, small errors can lead to significant mistakes. The key to improving accuracy lies in careful attention to detail, consistency, and methodical approaches. By following a few proven strategies, you can significantly reduce the chances of making computational errors.
Double-Check Your Work
One of the most effective ways to ensure accurate results is to double-check your calculations. This involves reviewing your steps and verifying that each part of the process aligns with the correct formulas or rules. When solving problems that involve multiple stages, taking a moment to go over each step can help you catch any errors before finalizing your solution.
Use a Consistent Method

Sticking to a reliable and consistent method for calculations minimizes the chances of mistakes. Whether you’re using a calculator or doing the math manually, it’s important to follow the same procedures each time. This consistency ensures that you don’t overlook any steps and that each part of the problem is handled accurately.
Moreover, when working with long calculations or multiple figures, it’s helpful to break down the problem into smaller, more manageable parts. This allows you to focus on one section at a time, reducing the likelihood of errors caused by rushing or fatigue.
By incorporating these strategies into your approach, you’ll not only improve the accuracy of your calculations but also develop better problem-solving habits that can help you succeed in any analytical task.
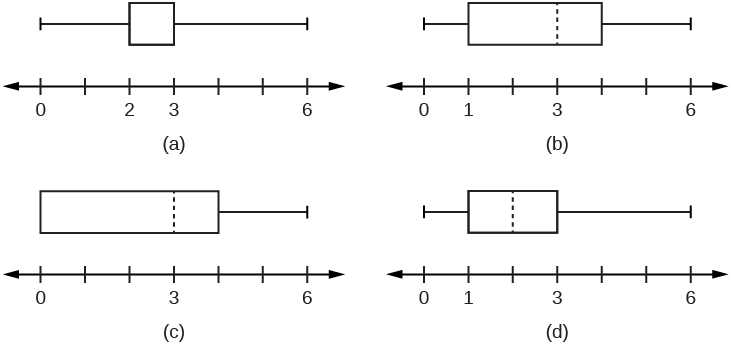
Understanding Statistical Graphs and Charts
Interpreting visual representations of data is an essential skill in many problem-solving scenarios. Graphs and charts provide a concise way to present complex information, allowing for quicker insights into trends, distributions, and relationships. To make the most of these visual tools, it is important to understand the different types of charts and how to extract meaningful information from them.
There are several common types of visual data representations, each suited for different kinds of information. Knowing which chart to use and how to interpret it is key to understanding the data at hand.
Types of Visual Representations
- Bar charts: Used to compare quantities across different categories. Each bar represents a category, with its height or length indicating the value.
- Histograms: Useful for displaying the frequency distribution of data. This chart shows how data points are distributed across intervals.
- Pie charts: Great for illustrating proportions of a whole. Each slice of the pie represents a percentage of the total data set.
- Line graphs: Ideal for showing trends over time. Each point represents a data value, and the points are connected by a line to illustrate a progression.
How to Interpret the Data
When reviewing graphs and charts, it’s important to ask the following questions:
- What is the source of the data? Ensure that the data comes from a reliable and relevant source.
- What variables are being represented? Identify the axes, labels, and what they represent.
- What trends or patterns emerge? Look for any noticeable trends, such as increases, decreases, or patterns that might indicate a correlation.
- Are there any anomalies or outliers? Pay attention to values that deviate from the expected range, as they could represent important insights or errors in the data.
Understanding how to read and analyze graphs and charts not only makes interpreting data easier but also helps in making informed decisions based on visualized information.
Effective Use of Statistical Formulas
Mastering mathematical equations is crucial for solving complex problems and deriving meaningful insights. Formulas are the building blocks of many calculations, offering a structured way to interpret and manipulate data. However, knowing when and how to apply each formula is key to achieving accurate results. Understanding the components of a formula and practicing its use in different contexts will enhance your problem-solving skills and reduce errors.
Understanding Formula Components
Each formula is made up of variables that represent specific values or quantities. Before applying any formula, it’s important to understand what each variable stands for and how it fits into the overall equation. For example, when using a formula to calculate the mean, knowing the sum of all values and the total number of data points is crucial. Similarly, in probability formulas, understanding the concepts of outcomes, events, and probabilities will guide the correct application.
Common Pitfalls to Avoid
Even the most well-known formulas can lead to mistakes if applied incorrectly. Some common errors include:
- Misinterpreting variables: Always double-check the units or measurements used in the formula to ensure they are consistent.
- Forgetting to account for all factors: In many formulas, all relevant factors must be included. Missing even one can lead to incorrect results.
- Overlooking order of operations: When applying formulas, be mindful of the correct order in which operations should be carried out, especially in more complex expressions.
By staying attentive to the structure and application of formulas, you can avoid common mistakes and improve the accuracy of your calculations.
Key Resources for Exam Preparation
Preparing for a test requires the right tools and materials to effectively review and consolidate knowledge. Accessing the right resources can make the difference between confusion and clarity. These resources range from textbooks and online platforms to practice materials and study guides, each serving a unique purpose in helping you understand concepts and hone your skills.
One of the most valuable resources is practice exercises. These provide an opportunity to apply the knowledge you’ve gained and familiarize yourself with the format of the problems you’ll encounter. Additionally, instructional videos and online courses can offer explanations and step-by-step breakdowns of challenging topics, helping to reinforce understanding.
Another key resource is group study sessions. Collaborating with peers allows you to discuss complex ideas and learn different approaches to problem-solving. Using flashcards or summary sheets can also help in memorizing formulas and important concepts, making review more efficient and manageable.
By combining various resources such as practice materials, online tutorials, peer discussions, and reference books, you can create a comprehensive study plan that boosts both your understanding and performance.
Final Thoughts on Practicing for Success
Success in any test is not just about memorizing facts; it’s about developing a deep understanding of the material and the ability to apply concepts effectively. Consistent practice plays a crucial role in reinforcing your knowledge and boosting confidence. It enables you to recognize patterns, identify common mistakes, and develop strategies for solving problems more efficiently.
While preparing for a test, it’s important to focus on both quality and consistency. Regular review sessions, combined with a variety of resources, help to solidify concepts in your mind and make you more adaptable when facing unfamiliar problems. Additionally, taking the time to reflect on past mistakes is just as important as practicing new material, as it helps you understand where improvement is needed.
Embrace the Learning Process
Remember that preparation is a continuous process. Embrace it as a journey of learning, rather than a one-time event. The more you engage with the material, the more you’ll discover nuances that can give you an edge during the test. Stay curious and keep challenging yourself to go beyond simple answers and dive into a deeper understanding of the subject.
Stay Positive and Confident
Finally, approaching the test with a positive mindset and confidence is key. Practice not only strengthens your knowledge but also builds your mental resilience. Trust in your preparation, stay focused, and remain calm. With the right strategy, consistent effort, and a positive outlook, success is within reach.