
Mastering mathematics often requires a combination of practice, guidance, and resources. For those looking to strengthen their problem-solving skills, having access to detailed solutions and explanations can make a significant difference. This resource offers insights into various exercises designed to enhance understanding and application of mathematical concepts.
By following step-by-step instructions and reviewing corrected solutions, learners can build confidence and refine their approach to complex problems. Whether you’re a student seeking to improve or an educator looking to support your classroom, these materials provide essential support to help navigate through mathematical challenges.
Structured exercises paired with comprehensive explanations allow for self-paced learning, enabling individuals to tackle difficult topics and test their understanding. This method not only reinforces key concepts but also boosts long-term retention of essential mathematical skills.
Punchline Bridge to Algebra 2nd Edition Overview
This resource provides a structured approach to learning mathematics, focusing on essential concepts and problem-solving strategies. It aims to equip students with the tools they need to successfully navigate complex exercises and develop a deeper understanding of fundamental mathematical principles.
The material is designed to guide learners through various topics, offering clear explanations and practical examples to reinforce their knowledge. By working through carefully constructed problems, students can build both their skills and confidence in tackling mathematical challenges.
- Focus on core mathematical principles
- Step-by-step exercises with clear solutions
- Designed for students at different learning levels
- Support for educators looking to enhance their teaching methods
- Flexible learning pace, suited for independent study
By utilizing this resource, learners gain a deeper insight into the application of mathematical rules, while also refining their ability to solve problems efficiently. The material encourages critical thinking and analytical skills, which are essential for mastering advanced topics in mathematics.
Understanding Key Algebra Concepts
A strong foundation in mathematics requires mastering several core principles. These foundational ideas are crucial in solving complex problems and applying mathematical rules effectively. This section focuses on the most important concepts that every student needs to grasp in order to excel in higher-level mathematical studies.
Essential Mathematical Operations
One of the most fundamental skills is the ability to manipulate numbers and symbols accurately. Understanding basic operations such as addition, subtraction, multiplication, and division is essential for more advanced topics. These operations serve as the building blocks for working with equations, expressions, and functions.
Solving Equations and Inequalities
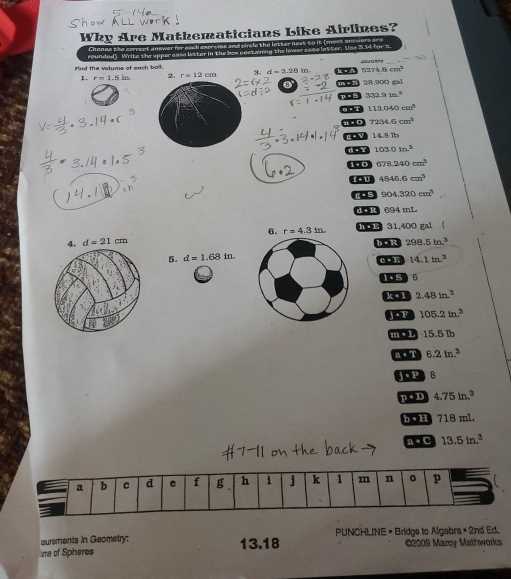
Equations and inequalities represent relationships between different quantities. Solving them involves finding values that satisfy these relationships. Grasping the concepts of variables, coefficients, and constants is key to manipulating and solving such mathematical statements. Mastery of this skill allows for the solution of more complex mathematical challenges.
How to Use the Answer Key
Utilizing a solution guide effectively can significantly enhance your understanding of mathematical concepts. It provides a way to check your work, identify errors, and ensure that you are on the right track. Knowing how to make the most of this resource is key to improving your skills and reinforcing your learning.
Step-by-Step Review
After attempting a problem, refer to the provided solutions to see the correct steps and results. This allows you to compare your method with the one outlined in the guide and identify any discrepancies in your approach. By doing this, you can pinpoint where you made mistakes and understand how to avoid them in the future.
Tips for Effective Use
Don’t just focus on the final results. Pay attention to the reasoning behind each step. Understanding the logic behind each solution will help you apply similar methods to other problems. Here are a few suggestions for getting the most out of the guide:
- Work through problems on your own before checking the solution
- Compare your steps to the guide’s method
- Identify common mistakes and learn how to correct them
- Use the explanations to reinforce your understanding
Example Table
| Problem | Your Solution | Correct Solution | Explanation |
|---|---|---|---|
| Problem 1 | 15 | 15 | Correct steps and calculations were followed. |
| Problem 2 | 20 | 18 | Check your distribution of terms in the equation. |
Benefits of Using the Learning Resource
Utilizing a structured educational tool provides numerous advantages for students aiming to strengthen their mathematical abilities. With clear explanations, step-by-step guidance, and diverse problem sets, this resource helps learners build confidence and proficiency in solving complex exercises. By engaging with these materials, students not only enhance their understanding of core concepts but also improve their problem-solving techniques.
Enhanced Problem-Solving Skills
One of the key benefits of this resource is its ability to help learners develop strong problem-solving abilities. Each problem set is designed to challenge students and encourage them to think critically. As students work through exercises, they learn to approach problems from multiple angles and refine their strategies, which is essential for mastering advanced topics.
Reinforcement of Core Concepts
Regular practice with well-organized exercises reinforces core mathematical principles and improves retention. By working through a variety of problems that cover different aspects of the subject, students deepen their understanding and gain a broader perspective on how mathematical rules apply in real-world scenarios. This reinforces both basic and advanced concepts, making it easier to tackle more complex material in the future.
Common Mistakes in Algebra Practice
While solving mathematical problems, it’s easy to make errors that can lead to incorrect results. Recognizing and understanding these common mistakes is a crucial part of the learning process. By identifying the typical pitfalls, students can avoid them in the future and improve their overall problem-solving abilities.
| Common Mistake | Possible Causes | How to Avoid |
|---|---|---|
| Incorrectly distributing terms | Forgetting to apply the distributive property | Double-check each step and carefully apply distribution |
| Sign errors in equations | Confusing positive and negative signs | Pay close attention to the signs when manipulating terms |
| Misinterpreting variables | Assuming that variables have fixed values | Remember that variables represent unknown values and must be treated accordingly |
| Not simplifying expressions | Skipping the simplification steps after solving | Always simplify the final expression to its simplest form |
Being aware of these common mistakes can help you refine your approach and reduce errors in future practice. By applying a careful and methodical approach to each problem, learners can enhance their understanding and improve their overall mathematical proficiency.
How to Improve Math Skills
Improving your mathematical abilities involves consistent practice, understanding key concepts, and applying effective strategies. By focusing on both the basics and more complex topics, you can gradually build confidence and mastery. Here are several tips to help you improve your problem-solving skills and deepen your understanding of essential mathematical principles.
Practice Regularly
The more you practice, the more familiar you become with various types of problems and solutions. Regular practice helps solidify concepts and improves recall when solving complex problems. To maximize your progress, follow these suggestions:
- Work on a variety of problems each day
- Focus on different types of exercises to build versatility
- Review incorrect answers to understand mistakes
Focus on Understanding, Not Just Memorizing
Rather than just memorizing formulas or steps, focus on understanding the logic behind them. This deeper understanding allows you to apply mathematical principles more effectively in different contexts. Here’s how to achieve this:
- Ask yourself why each step is necessary
- Break down problems into smaller parts to see how they connect
- Discuss concepts with peers or teachers to gain different perspectives
Seek Help When Needed
If you encounter difficulty with a particular concept, don’t hesitate to seek guidance. Working with others or consulting additional resources can provide clarity and help you overcome challenges. Consider:
- Asking teachers or tutors for explanations
- Participating in study groups for collaborative learning
- Using online resources to find alternative explanations or examples
By applying these strategies, you can gradually improve your skills and gain a better understanding of mathematical concepts, ultimately increasing your confidence and ability to solve a wide range of problems.
Exploring Mathematical Problem-Solving Techniques
Problem-solving is an essential skill in mathematics that requires more than just performing calculations. It involves analyzing the problem, identifying patterns, and choosing the most effective strategy to find the solution. Mastering these techniques can help students approach a wide range of challenges with confidence and precision.
Step-by-Step Approach

One of the most effective techniques for solving complex mathematical problems is breaking them down into smaller, more manageable parts. By addressing each component individually, you can gain a better understanding of the problem and avoid feeling overwhelmed. This method allows you to focus on the logic and structure of the solution without getting lost in the details.
Using Logical Reasoning
Another important technique is to use logical reasoning to guide your approach. By identifying known facts and relationships, you can eliminate unnecessary steps and simplify the process. Logical reasoning helps to establish connections between different elements of the problem, leading you toward the correct solution more efficiently.
Applying these techniques consistently can significantly improve your ability to tackle even the most challenging mathematical problems. Whether you’re working with equations, expressions, or more advanced topics, developing a systematic and logical approach is key to success.
Step-by-Step Walkthrough of Solutions
Following a structured approach to solving mathematical problems is crucial for improving both understanding and accuracy. A detailed walkthrough of the solution process can help students grasp the logic behind each step and apply these methods to similar problems in the future. By breaking down each step, learners can gain deeper insights into how solutions are reached, which is key to mastering the subject.
Breaking Down the Problem

The first step in any solution process is to carefully read and understand the problem. Identify the key information, what is being asked, and the relevant operations or concepts required to find the solution. Breaking the problem into smaller parts can make it more manageable and less intimidating. This ensures that you don’t miss important details and that you address every aspect of the question.
Step-by-Step Execution
Once the problem is understood, the next step is to apply the appropriate operations in a logical sequence. Each action taken should be explained clearly, with attention paid to the reasoning behind every step. For instance:
- Step 1: Identify the variables and constants
- Step 2: Apply mathematical rules and operations based on the problem type
- Step 3: Simplify expressions where needed
- Step 4: Double-check calculations for accuracy
By following this methodical approach, students can confidently work through similar problems in the future. Remember, understanding the “why” behind each step is just as important as getting the correct result.
How the System Supports Learning
The system provides an engaging and structured approach to mastering mathematical concepts. It is designed to break down complex ideas into manageable steps, making it easier for students to grasp challenging material. By offering a variety of practice problems and solutions, this method enhances comprehension and ensures that learners gain confidence in their skills.
One of the key features is the gradual progression from simple to more advanced topics, which allows students to build a solid foundation before tackling more difficult material. This ensures that learners are not overwhelmed and can develop their problem-solving abilities at a comfortable pace. Additionally, the clear explanations of each step provide valuable insight into the reasoning behind mathematical procedures, helping students understand the “why” behind the “how.” This promotes long-term retention and a deeper understanding of the subject.
By integrating multiple learning techniques and offering continuous support through various resources, this system creates an environment where students can thrive, refine their skills, and ultimately achieve success in mathematics.
Learning Strategies for Math Students
Effective learning strategies are essential for mastering mathematical concepts. These approaches help students understand the underlying principles and solve problems with confidence. By applying targeted methods and maintaining a consistent study routine, students can improve their skills and tackle even the most complex topics.
1. Practice Regularly: Consistent practice is key to reinforcing concepts and improving problem-solving abilities. Working on a variety of exercises ensures that students gain exposure to different types of problems and can apply what they’ve learned in various contexts. A steady practice schedule helps build confidence and familiarity with the material.
2. Break Problems into Smaller Steps: Large, complex problems can be intimidating, but breaking them down into smaller, more manageable parts makes them easier to solve. Students should focus on understanding each step of the process before moving on to the next, ensuring that no crucial details are missed.
3. Seek Additional Resources: When faced with difficulties, seeking alternative explanations or examples can provide new perspectives and enhance understanding. Online tutorials, peer discussions, and teacher support can all contribute to clarifying difficult concepts and reinforcing learning.
4. Master the Basics: A strong foundation in basic concepts is critical for success in more advanced topics. Ensuring that fundamental skills are well-understood before progressing to more challenging material will make learning smoother and less stressful.
5. Stay Organized: Keeping track of notes, assignments, and formulas can help students stay on top of their work and prevent confusion. Developing a system for reviewing and organizing material ensures that important information is easily accessible when needed.
By adopting these strategies and staying disciplined in their approach, students can significantly improve their mathematical abilities and achieve greater success in their studies.
Key Features of the Revised Version
The revised version offers a range of enhanced features designed to improve the learning experience and make mastering mathematical concepts more accessible. These updates include clearer explanations, more interactive practice opportunities, and a stronger emphasis on building foundational skills. The overall goal is to support learners at every stage of their journey, from understanding basic principles to tackling more complex problems.
Improved Structure and Content

The updated format introduces a more organized and systematic approach to teaching mathematical concepts. Each chapter is divided into smaller, focused sections, allowing students to digest information in manageable chunks. This approach helps learners better understand the material before progressing to more challenging topics.
- Clear, concise explanations for each topic
- Step-by-step breakdowns of complex problems
- Real-world examples to demonstrate practical applications
Enhanced Practice and Support Tools
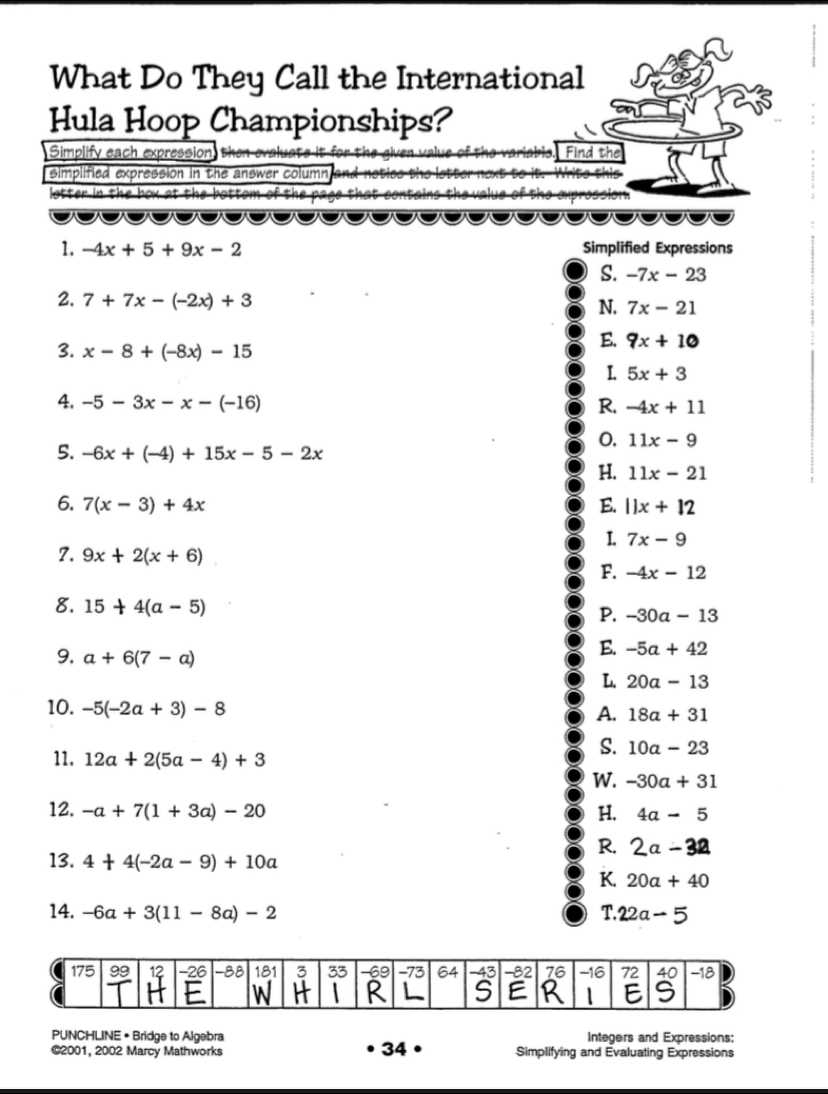
The revised version includes a variety of additional resources aimed at reinforcing learning and providing ongoing support. These tools are designed to offer personalized practice opportunities and promote active engagement with the content.
- Interactive problem sets with instant feedback
- Comprehensive solution guides for self-assessment
- Supplementary online resources for further study
With these improvements, the revised version provides a more dynamic and supportive environment for students to master essential mathematical skills.
Understanding Mathematical Equations
Mathematical equations are foundational to problem-solving and play a crucial role in many branches of mathematics. At their core, equations represent relationships between different quantities, and solving them involves finding the values that make both sides of the equation equal. Understanding how to approach and solve these relationships is essential for developing strong problem-solving skills and mastering more advanced concepts.
Key Elements of an Equation
To fully understand equations, it’s important to first recognize their key components. An equation typically consists of variables, constants, and operators. The variables represent unknown values, while constants are fixed values. Operators, such as addition, subtraction, multiplication, and division, define the relationships between the variables and constants. The goal is to manipulate these components to isolate the unknowns and solve for their values.
- Variables: Symbols that represent unknown values, often denoted by letters like x, y, or z.
- Constants: Fixed numbers that are known, such as 2, 3, or 5.
- Operators: Mathematical symbols that show how the variables and constants are related, such as +, -, *, and /.
Solving Basic Equations
Solving basic equations often requires the use of inverse operations to isolate the variable on one side. The steps typically involve simplifying both sides of the equation and applying arithmetic rules to solve for the unknown value. Understanding the order of operations and the properties of equality is crucial to ensure accurate results.
- Isolate the variable: Use inverse operations to get the variable by itself.
- Balance both sides: Ensure that both sides of the equation remain equal as you simplify.
- Check your solution: Substitute the solution back into the original equation to verify its correctness.
By mastering these basic concepts, students can build a strong foundation in solving more complex equations and advance to more challenging mathematical topics.
Why the Solution Guide is Essential
A solution guide is an invaluable resource for students working through mathematical exercises. It not only provides the correct solutions but also offers step-by-step explanations that help learners understand the processes involved in solving complex problems. Without this support, students may struggle to identify where they went wrong or fail to understand the reasoning behind each step of the solution. The ability to check one’s work and understand the methods behind it is crucial for developing strong problem-solving skills.
Clarifying the Process
One of the primary benefits of using a solution guide is its ability to clarify the steps involved in solving a problem. Often, students can reach an answer but remain unsure of how they arrived at it. A solution guide breaks down the problem-solving process, showing how to approach each stage systematically. This not only reinforces learning but also builds confidence in tackling similar problems independently in the future.
- Step-by-step explanations: Detailed breakdowns of each part of the problem-solving process.
- Illustrates logical reasoning: Demonstrates how to apply rules and properties to reach the correct solution.
- Ensures understanding: Helps students grasp concepts beyond the final result.
Enhancing Self-Assessment
A solution guide allows students to evaluate their work and spot errors. By comparing their methods and results with the guide, learners can identify areas of improvement and adjust their approach accordingly. This self-assessment process is key to reinforcing understanding and building effective study habits.
- Immediate feedback: Enables quick verification of answers and methods.
- Identifying mistakes: Helps pinpoint where mistakes were made and understand how to avoid them in the future.
- Promotes independent learning: Encourages students to become more self-reliant in their studies.
Ultimately, a solution guide is more than just a tool for checking answers–it’s a vital part of the learning process that supports understanding, self-improvement, and independent problem-solving.
Tips for Effective Practice in Mathematics

Mastering mathematical concepts requires consistent and deliberate practice. Effective practice involves not only solving problems but also understanding the underlying principles and methods. Simply working through problems repeatedly without reflecting on the process can lead to misunderstandings and confusion. To make the most out of your study time, it’s essential to adopt strategies that focus on both accuracy and comprehension.
Focus on Understanding, Not Just Results
One of the most important tips for effective practice is to prioritize understanding the reasoning behind each step rather than focusing solely on obtaining the correct answer. By understanding why certain methods work, you can apply the same principles to a variety of problems and ensure a deeper grasp of the subject. Take time to review the logic and patterns that emerge as you solve each problem.
- Break down each problem: Analyze each step and ensure you understand why it’s being performed.
- Connect concepts: Relate the current problem to previously learned material to strengthen your overall understanding.
- Ask questions: If you don’t understand a step or concept, seek clarification before continuing.
Set Clear Goals for Each Session
When practicing, it’s important to have clear goals for each study session. This can be as simple as mastering a specific technique or solving a certain number of problems within a set timeframe. Setting achievable targets allows for focused effort and helps track progress over time. Moreover, breaking down larger tasks into smaller, manageable pieces makes them less overwhelming and easier to complete.
- Start with easier problems: Warm up with simple problems to build confidence before tackling more complex ones.
- Gradually increase difficulty: Progressively challenge yourself with more difficult questions to test and expand your skills.
- Take breaks: Regular intervals of rest can help maintain focus and prevent mental fatigue.
By adopting these strategies and consistently applying them, you will improve your problem-solving abilities and gain a stronger grasp of mathematical concepts.
Advanced Topics in Mathematics
As students progress in their mathematical studies, they encounter more complex concepts that build upon foundational principles. These advanced topics often introduce new methods, more intricate equations, and a deeper understanding of mathematical relationships. Exploring these areas challenges learners to apply their existing knowledge in innovative ways, offering opportunities for intellectual growth and problem-solving mastery.
In this section, we will look at some of the key advanced topics that students may face in higher-level mathematics. These subjects are often essential for those pursuing careers in science, engineering, and other fields where analytical thinking is crucial. Understanding these topics requires a combination of practice, logical reasoning, and the ability to connect abstract ideas with real-world applications.
Systems of Equations and Inequalities

Systems of equations and inequalities are foundational to understanding how multiple variables interact. These topics allow for the analysis of more complex relationships than simple linear equations. Students learn to solve for multiple unknowns and understand how various constraints can define a system. Whether solving through substitution, elimination, or matrix methods, these systems provide tools for solving real-world problems in engineering, economics, and more.
Quadratic Functions and Their Applications

Quadratic functions represent relationships that involve squared terms. These functions are key to understanding many real-world scenarios, such as projectile motion, area optimization, and profit maximization. Mastery of quadratic equations, including methods like factoring, completing the square, and the quadratic formula, enables students to solve complex problems with ease. The graphical representation of quadratic functions, in the form of parabolas, further enhances their application in various scientific disciplines.
Exponential and Logarithmic Functions
Exponential and logarithmic functions explore the behavior of rapidly growing or decaying quantities. These functions are crucial for modeling phenomena such as population growth, radioactive decay, and financial interest rates. Understanding their properties, such as the laws of exponents and the relationship between exponents and logarithms, allows students to solve equations that involve exponential growth or decline. Mastery of these concepts is essential for fields like biology, economics, and physics.
Polynomials and Rational Expressions
Polynomials and rational expressions expand the scope of algebraic equations by introducing terms of varying degrees and allowing for more sophisticated manipulations. These topics involve operations such as polynomial division, factoring, and simplifying rational expressions. The skills developed through these problems are crucial for solving more complicated mathematical models and provide a foundation for calculus and beyond.
These advanced topics provide the mathematical tools necessary for addressing complex problems. They open doors to deeper mathematical thinking, providing students with the capability to tackle a wider array of challenges in both academic and real-world contexts.
Using Answer Guides for Self-Study
When studying independently, having access to solutions can significantly enhance the learning process. An answer guide serves as a valuable tool for self-assessment, allowing learners to verify their solutions and identify areas where they may need further practice. It also provides insights into the problem-solving process, helping students understand the steps required to reach the correct result.
Using these guides effectively involves more than just checking answers. Students can analyze the solution steps to learn the methods and strategies used to approach a problem. This reflection helps to solidify their understanding and enables them to apply the same techniques to similar questions in the future.
Benefits of Using a Solution Guide
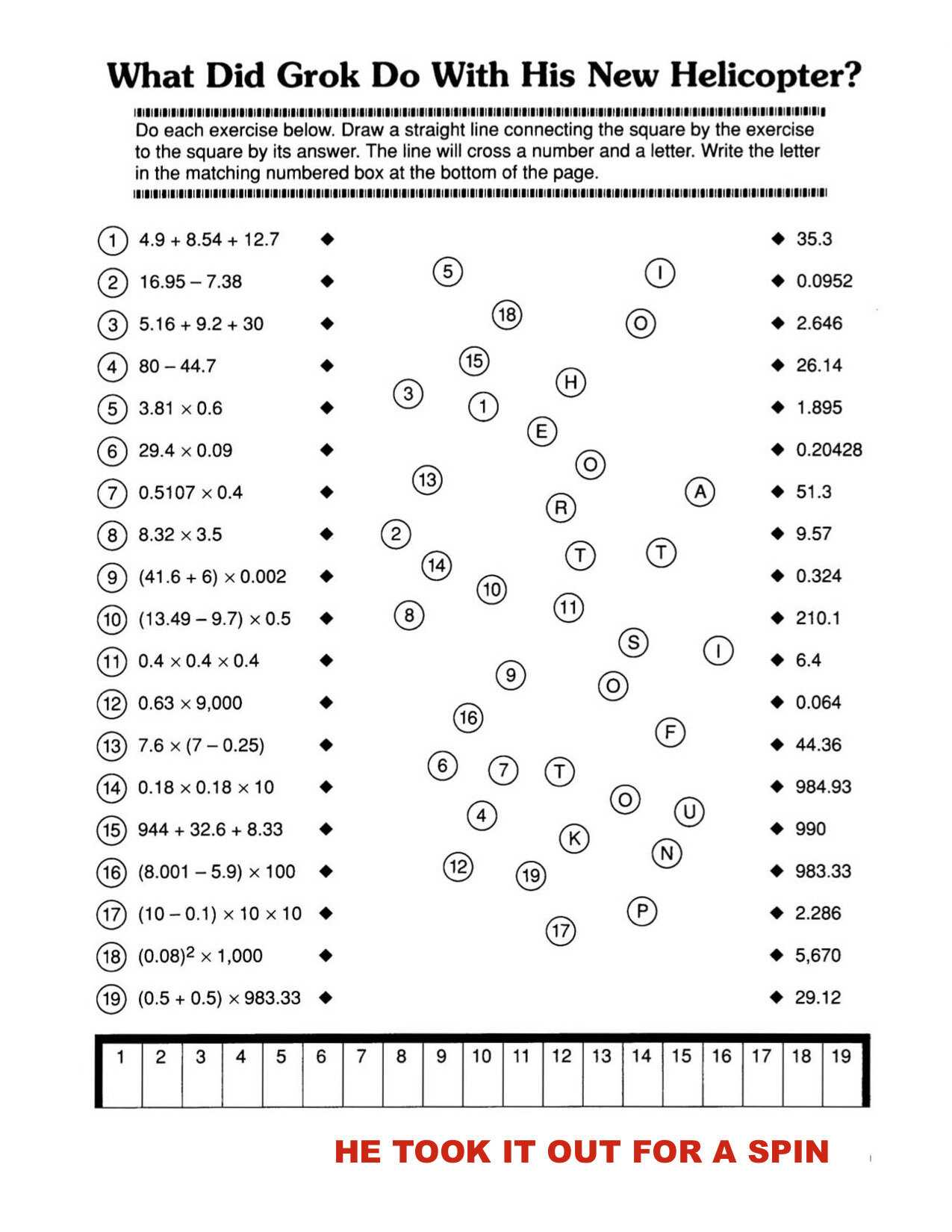
- Instant Feedback: Quickly verify if you are on the right track and identify mistakes in your work.
- Step-by-Step Learning: Understand the process behind each solution, ensuring that you grasp the underlying concepts.
- Improved Confidence: By mastering each step, students become more confident in their abilities to solve similar problems.
- Increased Retention: Reviewing correct methods and solutions reinforces learning and helps commit concepts to memory.
How to Use a Solution Guide Effectively
To make the most of a solution guide, follow these strategies:
- Attempt the problem first: Always try to solve the problem on your own before consulting the guide. This helps develop problem-solving skills and promotes deeper understanding.
- Compare step-by-step: After reviewing the solution, compare each step with your approach to identify any differences. Understand why a particular method is used.
- Analyze mistakes: If your solution differs, don’t just note the correct answer; instead, examine where you went wrong and focus on understanding the correct approach.
- Practice similar problems: Use the guide to work through similar problems to ensure you’ve fully mastered the method.
By using answer guides as a self-study tool, learners can significantly enhance their understanding, improve problem-solving techniques, and develop greater independence in their studies. This approach ensures that learners are not just memorizing answers but gaining a deeper comprehension of the subject matter.