In this section, we will provide a detailed breakdown of essential exercises and solutions found in the third unit of the curriculum. Whether you’re reviewing for a test or looking to reinforce your skills, this guide will offer a clear path to understanding and mastering key concepts.
Understanding the methods and processes behind each problem is crucial for achieving success. By exploring the solutions, you will gain a better grasp of the logical steps needed to approach similar tasks in the future. This resource is designed to help you build confidence and refine your problem-solving abilities.
Each problem has been carefully explained to ensure you can follow the reasoning step by step. The goal is not just to know the answers but to understand how to arrive at them. With practice and a solid understanding of the techniques, you will improve both your accuracy and speed in solving related challenges.
Level 1 Unit 3 Problem Solutions
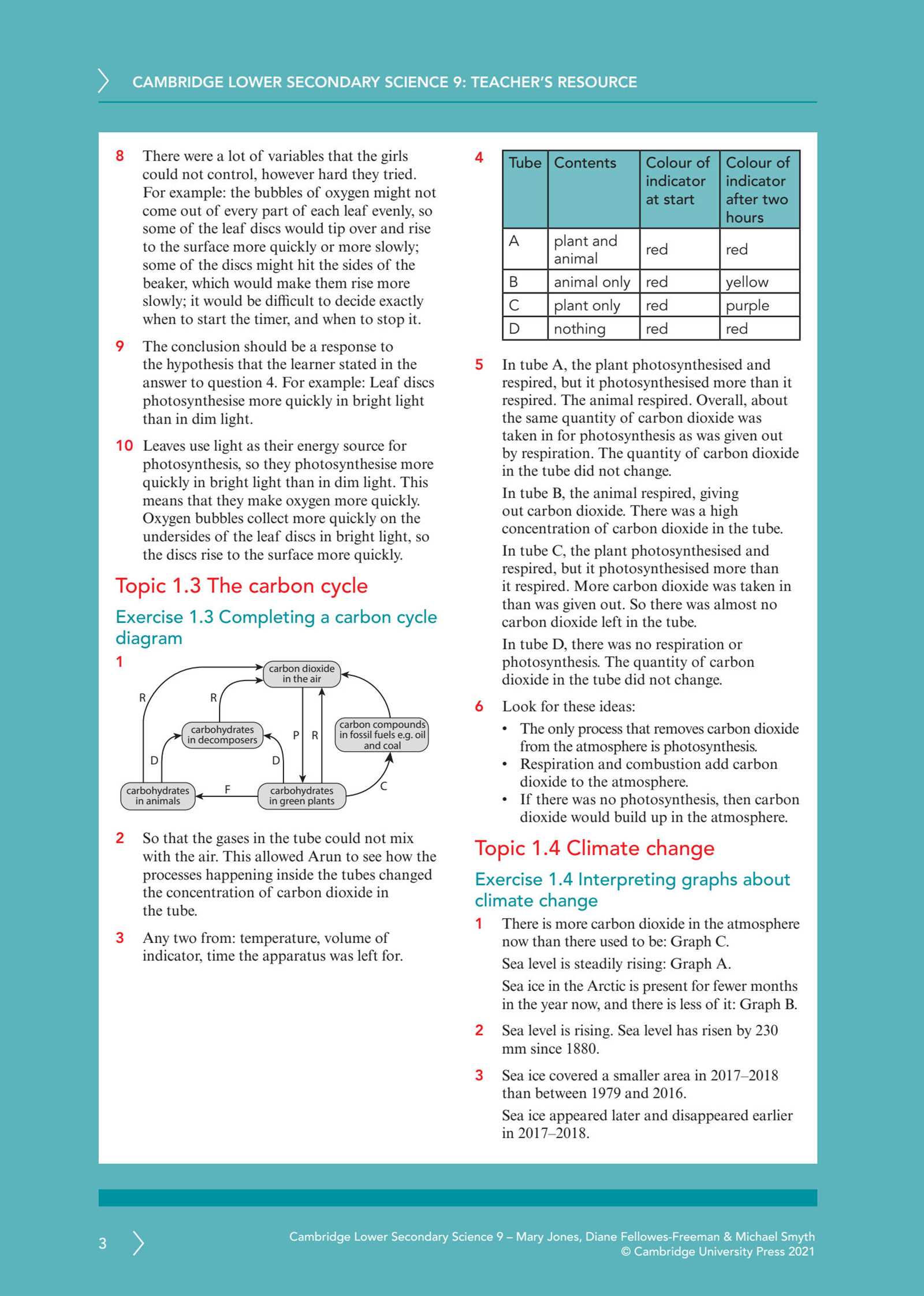
This section focuses on providing detailed solutions to the exercises in the third unit of the course. By walking through the steps of each task, you will be able to better understand the reasoning behind every solution. The explanations are designed to help you improve your problem-solving techniques and deepen your understanding of the concepts covered.
Step-by-Step Guide to Solutions
Each solution is broken down into clear, manageable steps to ensure you can follow along easily. We will explore different methods of approaching the problems, explaining the logic behind each calculation and process. By practicing these steps, you’ll be able to solve similar problems independently and more efficiently.
Common Challenges and How to Overcome Them
While working through the exercises, many learners encounter specific difficulties, such as understanding complex equations or interpreting word problems. In this section, we highlight these common challenges and provide tips and strategies to overcome them. With a deeper understanding of these key areas, you’ll feel more confident in tackling any related tasks in the future.
Understanding the Key Concepts
Grasping the fundamental principles behind each problem is essential for mastering the content in this section. Whether it’s working with equations, ratios, or geometric principles, understanding the underlying concepts allows you to approach problems with confidence and clarity. By familiarizing yourself with these core ideas, you’ll be better equipped to solve more complex tasks and apply your knowledge in real-world situations.
Each topic is interconnected, meaning that mastering one concept often paves the way for understanding others. For example, a strong grasp of basic operations is critical when tackling algebraic expressions or solving equations. In this section, we focus on breaking down each concept, highlighting the most important steps and strategies for success.
Key concepts such as fractions, proportions, and basic algebraic rules are essential building blocks in this level. By thoroughly understanding these areas, you will be able to tackle more advanced topics with ease. The goal is not only to solve problems correctly but to understand why the methods work, providing you with the tools needed to approach new challenges in the future.
Overview of Unit 3 Topics
Unit 3 covers a variety of foundational concepts that are essential for building a solid understanding of the subject. The topics explored in this section help develop both analytical thinking and problem-solving skills. Each subject area plays a critical role in advancing your knowledge, enabling you to tackle more complex challenges as you progress.
Key Areas of Focus
This unit addresses several critical topics, ranging from basic operations to more advanced techniques. Below is an overview of the main areas that are covered:
| Topic | Description |
|---|---|
| Fractions | Understanding how to work with and simplify fractions, including operations like addition, subtraction, and multiplication. |
| Proportions | Learning how to solve problems involving ratios and proportions, which are important for comparing quantities. |
| Basic Equations | Introduction to solving linear equations and understanding variables and constants in simple algebraic expressions. |
| Geometry | Exploring the basics of shapes, angles, and measurements in geometric problems. |
Progression and Application
Each topic is carefully structured to build upon the previous one, ensuring a gradual and comprehensive approach to learning. By mastering these concepts, you’ll be well-prepared for more advanced topics in later units. The skills learned here will not only enhance your mathematical ability but also improve your critical thinking and analytical skills in other subjects.
How to Use the Solution Guide
Utilizing a solution guide effectively can significantly enhance your understanding of the subject. It’s not only about checking if your results are correct but also about grasping the reasoning behind each step. This approach helps build a deeper comprehension of the methods and strategies used to solve the problems, which is key for improving your skills.
To make the most of this resource, start by attempting the problems on your own. Once you’ve worked through an exercise, refer to the guide to check your solution. Focus on the process outlined in the guide rather than just the final result. Pay attention to each step, and if there’s a part you don’t understand, review it carefully to identify where you might have gone wrong.
By analyzing the provided solutions, you’ll develop a clearer picture of the underlying principles and techniques, making it easier to tackle future problems. Over time, this practice will help you refine your problem-solving abilities and gain more confidence in your skills.
Important Formulas for Unit 3
In this section, we focus on the most essential formulas that you need to master in order to successfully tackle the problems in this unit. These formulas serve as the foundation for solving various types of exercises and are crucial for understanding the relationships between different elements within the problems.
By familiarizing yourself with these key equations, you will be able to approach tasks with greater confidence and accuracy. Below is a list of the most important formulas to keep in mind:
- Fraction Addition: a/b + c/d = (ad + bc) / bd
- Multiplication of Fractions: a/b × c/d = ac / bd
- Proportion Formula: a/b = c/d, then ad = bc
- Simple Linear Equation: ax + b = c, then x = (c – b) / a
- Pythagorean Theorem: a² + b² = c² (for right-angled triangles)
- Area of a Rectangle: Area = length × width
- Perimeter of a Rectangle: Perimeter = 2(length + width)
Make sure to memorize these formulas and understand how to apply them in different scenarios. Regular practice with these equations will help you solve problems more efficiently and with fewer mistakes. These formulas are the building blocks for solving more complex tasks in future lessons.
Step-by-Step Problem Solving
Breaking down problems into manageable steps is essential for finding the correct solution. By following a structured approach, you can simplify complex tasks and ensure that each part of the problem is tackled systematically. This method not only helps in finding the right answer but also deepens your understanding of the underlying concepts.
Understanding the Problem
Before diving into calculations, it’s important to fully understand what the problem is asking. Read through the task carefully, identify key information, and make note of what needs to be solved. If the problem involves multiple steps, think about how to organize the process before you start solving it. This initial step sets the foundation for the entire process.
Breaking Down the Steps
Once the problem is understood, the next step is to break it down into smaller, more manageable parts. This could involve simplifying equations, substituting values, or working with one aspect of the problem at a time. By doing this, you can tackle each part individually, reducing the complexity of the overall problem. After solving each step, review your work to ensure that you haven’t missed anything before moving on to the next part.
By consistently using this step-by-step approach, you will improve your ability to solve problems accurately and efficiently, developing both your skills and confidence as you progress.
Common Mistakes to Avoid
When solving problems, it’s easy to make small errors that can lead to incorrect results. These mistakes often occur due to a lack of attention to detail or misunderstanding certain concepts. Identifying and correcting these common pitfalls is crucial for improving your problem-solving skills and ensuring that your answers are accurate.
One common mistake is rushing through the steps without fully understanding the problem. Skipping important details or not reviewing the question carefully can result in incorrect interpretations. Another frequent error is misapplying formulas or forgetting to adjust them to fit the given problem. For instance, mixing up the operations in fraction addition or neglecting to simplify expressions can lead to errors.
Additionally, many learners struggle with signs and negative numbers. It’s important to double-check the use of positive and negative values in equations, especially when dealing with subtraction or division. Finally, not verifying your final solution is a common oversight. Always take a moment to review your calculations and check if the answer makes sense in the context of the problem.
Tips for Mastering Problem Solving
Becoming proficient in solving mathematical challenges requires consistent practice and a strategic approach. Mastering the process involves more than just understanding formulas; it’s about developing a clear method for tackling problems and refining your ability to work through complex tasks step by step.
One key tip is to always break down the problem into smaller, more manageable parts. This allows you to focus on solving each component individually before combining them for the final solution. Additionally, practicing regularly is crucial. The more problems you work through, the more familiar you’ll become with common patterns and techniques, which will improve both your speed and accuracy over time.
Another helpful strategy is to approach each problem with a structured mindset. Start by reviewing the question carefully, identify the key information, and determine which formulas or methods are most applicable. Also, don’t hesitate to seek out different ways of solving a problem–sometimes there’s more than one approach, and experimenting with various techniques can deepen your understanding.
Lastly, always take time to check your work. It’s easy to make simple mistakes, but reviewing your solutions helps catch errors before they become bigger issues. With consistent effort and attention to detail, you’ll build both your confidence and problem-solving ability.
How to Check Your Answers
Reviewing your work is an essential part of the problem-solving process. It ensures that you haven’t missed any crucial steps or made simple mistakes. By carefully checking your solutions, you can confirm the accuracy of your results and strengthen your understanding of the underlying concepts.
Steps for Verifying Your Work
Here’s a simple approach to ensure your solutions are correct:
- Revisit the Problem: Read the question again to make sure you fully understand what’s being asked and that you haven’t overlooked any details.
- Check Each Step: Go through your calculations and ensure that each step is logical and follows the previous one correctly. Verify the use of formulas and operations.
- Estimate the Answer: Before you look at the solution guide, try to estimate what the answer should be. This gives you a reference point to compare against your final result.
- Use Different Methods: If possible, solve the problem using a different approach and compare the results. This can help you catch errors you might have missed with the first method.
- Verify Units and Signs: Double-check any units of measurement and the signs (positive/negative) to ensure they are consistent throughout your solution.
Final Check
After reviewing your solution, take a moment to reflect on whether the result makes sense in the context of the problem. If something seems off, retrace your steps and identify where the error occurred. Taking the time to check your work thoroughly can help improve both your accuracy and problem-solving skills.
Examples of Unit 3 Problems
Understanding how to apply the concepts you’ve learned is key to mastering any subject. In this section, we will explore several examples of problems that demonstrate how to use the techniques from this unit to arrive at the correct solutions. These examples cover a variety of topics and difficulty levels, helping you practice and strengthen your problem-solving skills.
Example 1: Solving Linear Equations
Let’s consider a simple linear equation and walk through the solution step by step:
- Problem: Solve for x: 2x + 5 = 15
- Solution: Subtract 5 from both sides: 2x = 10
- Divide both sides by 2: x = 5
By following these steps, you can quickly find the value of x, which in this case is 5.
Example 2: Working with Fractions
This next problem involves adding two fractions with different denominators:
- Problem: Add: 3/4 + 2/5
- Solution: First, find the least common denominator (LCD), which is 20.
- Rewrite each fraction with the denominator 20: 15/20 + 8/20
- Now, add the numerators: 15 + 8 = 23
- The final result is 23/20, which can be written as 1 3/20.
This problem illustrates how to handle fractions with different denominators by first converting them to a common denominator.
Example 3: Solving Word Problems
Now, let’s consider a word problem that involves basic algebra:
- Problem: A car rental company charges $20 per day plus a one-time fee of $50. How much will it cost to rent a car for 5 days?
- Solution: The total cost consists of the daily charge multiplied by the number of days plus the one-time fee.
- 20 × 5 + 50 = 100 + 50 = 150
- The total cost to rent the car for 5 days is $150.
Word problems often require translating real-world situations into mathematical equations, a valuable skill to practice.
Using the Guide for Better Understanding
Accessing a solution guide can be a valuable resource for reinforcing concepts and improving problem-solving skills. By reviewing worked-out examples and detailed explanations, learners can gain clarity on where they went wrong or how to approach problems more effectively. This guide serves as both a tool for validation and an educational aid that provides deeper insights into the reasoning behind each step.
How the Guide Enhances Learning
Using the solution guide allows you to compare your method with a correct approach. This not only helps you identify any mistakes but also shows you the steps that lead to the final solution. By understanding the reasoning behind each step, you can learn new techniques and strategies to apply to future problems. Additionally, revisiting solutions after attempting problems reinforces learning and helps solidify key concepts.
Applying the Guide to Improve Skills

It’s important to actively engage with the solution guide, not just passively read through it. When you examine each solution, ask yourself why the steps are taken in a particular order and how the mathematical principles are being applied. Practicing this type of reflection will help you think critically and approach future challenges with more confidence and accuracy.
Calculating Percentages and Fractions
Understanding how to work with percentages and fractions is essential for solving a variety of problems. These mathematical concepts are commonly used in everyday situations, from calculating discounts to determining proportions. By mastering the methods for calculating both, you can easily handle problems involving parts of a whole and express them in different forms.
To convert a fraction into a percentage, you multiply the fraction by 100. Conversely, to convert a percentage into a fraction, you divide the percentage by 100 and simplify. Knowing how to switch between these two forms is helpful when dealing with problems that require either format.
Converting Between Percentages and Fractions
Here’s a step-by-step example of how to convert between percentages and fractions:
| Fraction | Percentage |
|---|---|
| 1/4 | 25% |
| 3/5 | 60% |
| 7/10 | 70% |
For example, to convert 1/4 to a percentage, multiply 1 by 100 and divide by 4, which gives 25%. To go the other way, take 60% and divide by 100, which gives 3/5.
Practical Examples of Using Percentages and Fractions
Here are a few practical examples where percentages and fractions are used:
- Calculating the price of an item after a discount: A 20% discount on a $50 item means you pay $40.
- Determining the part of a total: If a class has 30 students and 12 are absent, the fraction of absent students is 12/30, or 40%.
These types of calculations are foundational and will come up in many situations, making them vital for a clear understanding of how to work with parts of a whole in both fractional and percentage forms.
How to Approach Word Problems
Word problems often appear challenging because they require translating a real-life scenario into a mathematical equation. However, with a clear strategy and systematic approach, these problems become easier to solve. The key is to carefully read the problem, identify what is being asked, and break it down into smaller, manageable steps. By following a structured approach, you can tackle even the most complex scenarios with confidence.
Steps to Solve Word Problems
Here’s a simple guide to help you solve word problems effectively:
- Read the problem carefully – Understand the situation fully before jumping into calculations.
- Identify the question – Focus on what is being asked, such as “how much,” “how many,” or “what is the total.”
- Extract key information – Look for numbers, relationships, or operations mentioned in the problem.
- Set up an equation – Translate the words into a mathematical equation using appropriate symbols.
- Solve the equation – Use the correct methods to find the solution.
- Double-check your work – Verify the solution by re-reading the problem and ensuring your answer makes sense.
Common Tips for Word Problems
Here are some helpful tips for approaching word problems more effectively:
- Underline important information – This can help you focus on the key elements of the problem.
- Draw a diagram or picture – Visual aids can make it easier to understand the problem and spot relationships.
- Look for keywords – Words like “total,” “difference,” “product,” or “sum” often signal the type of operation you need to perform.
- Break down complex problems – If the problem is long, divide it into smaller parts and solve each one step-by-step.
By following these strategies and practicing regularly, solving word problems will become a skill you can use confidently in various scenarios.
Solving Equations Efficiently

Solving equations is a fundamental skill in algebra, but solving them efficiently requires a structured approach. Instead of performing lengthy steps or getting caught in unnecessary calculations, mastering techniques to simplify the process can save time and reduce errors. Whether the equation is linear, quadratic, or involves fractions, the key lies in applying the right strategies to isolate the variable quickly and accurately.
One of the most effective ways to solve equations is by following a systematic approach that involves simplifying both sides of the equation, applying inverse operations, and reducing complexity as much as possible. With practice, solving equations becomes faster and more intuitive.
Key Techniques for Efficient Solving
Here are some methods that can help speed up the process:
- Combine like terms: Start by simplifying both sides of the equation. Combine any terms that have the same variable or constants to make the equation simpler.
- Use inverse operations: Apply inverse operations (addition to subtraction, multiplication to division) to move terms around and isolate the variable.
- Clear fractions: If fractions are present, multiply both sides of the equation by the least common denominator to eliminate them.
- Check for special cases: Look for special scenarios, such as simple equations where the variable is already isolated, or equations that can be solved through mental math.
Common Pitfalls to Avoid
Even when using efficient strategies, it’s easy to make mistakes if you’re not careful. Here are some common errors to watch out for:
- Forgetting to apply operations to both sides: Always perform the same operation on both sides of the equation to maintain equality.
- Overcomplicating the problem: Sometimes, equations look more complicated than they are. Look for patterns or shortcuts that can simplify the problem.
- Making calculation errors: Double-check all arithmetic to avoid small mistakes that can lead to incorrect answers.
By applying these methods and remaining focused, solving equations can become a more efficient and manageable task, even with more complex problems.
Building Confidence with Practice
Developing confidence in problem-solving requires consistent practice and a willingness to tackle challenges. It’s common for students to feel unsure about their abilities when confronted with new concepts or complex tasks. However, regularly engaging with problems, breaking them down into smaller steps, and gradually increasing the level of difficulty can significantly enhance one’s skillset and self-assurance. The more problems you solve, the more familiar and approachable they become.
Building confidence is a process that goes beyond just memorizing formulas or steps; it involves understanding the logic behind the solutions and developing the resilience to face difficulties. As you practice, your problem-solving skills will improve, and you’ll notice your ability to approach new challenges with a more positive mindset.
Practical Tips for Gaining Confidence
Here are some strategies to help boost your confidence through consistent practice:
- Start with simpler problems: Begin with easier problems to build a solid foundation and gradually move on to more complex ones.
- Review mistakes: Analyze errors to understand where you went wrong. This is a key step in avoiding similar mistakes in the future and strengthening your problem-solving abilities.
- Work with others: Collaboration can provide different perspectives on solving problems, which can deepen understanding and give you new approaches to try.
- Set achievable goals: Set realistic and measurable goals for yourself, such as solving a specific number of problems per day or mastering a particular concept.
Building Resilience in Problem-Solving
It’s important to remember that challenges are part of the learning process. The more you practice, the more comfortable you become with overcoming obstacles. Resilience is built through repeated attempts and learning from both successes and setbacks. Keep practicing, stay persistent, and you’ll see your confidence grow over time.
Resources for Further Study
To deepen your understanding and refine your problem-solving skills, a variety of resources are available that can provide additional support. From textbooks and online courses to practice platforms and educational videos, these tools can help reinforce key concepts and offer new strategies for tackling problems. Leveraging multiple resources is an effective way to gain a broader perspective and improve both comprehension and application of the material.
Whether you’re looking for step-by-step tutorials, interactive exercises, or real-life applications, these resources can offer valuable insights and guidance for enhancing your knowledge and skills. It’s important to choose resources that align with your learning style and goals to maximize their impact.
Recommended Books and Guides
Books and guides are an excellent way to study at your own pace. Many of them offer detailed explanations, practice exercises, and solutions to help you understand the material better. Some of the most recommended texts include:
- Concepts in Algebra – A comprehensive guide covering essential algebraic principles with numerous examples and practice exercises.
- Practical Problem-Solving – A book that focuses on real-world applications and how to apply mathematical concepts in everyday scenarios.
- Problem-Solving Strategies for Beginners – A beginner-friendly guide aimed at helping learners build their problem-solving abilities step-by-step.
Online Learning Platforms
Online platforms provide interactive lessons and exercises that allow you to learn at your own pace. Some popular websites for further study include:
- Khan Academy – Offers free courses on a wide range of topics, including algebra and problem-solving, with video lessons and quizzes.
- Coursera – Provides access to university-level courses that cover fundamental and advanced topics in mathematics and problem-solving techniques.
- EdX – Offers high-quality online courses from top universities, focusing on strengthening mathematical reasoning and application skills.
By using these resources, you can enhance your understanding, reinforce important concepts, and improve your ability to solve problems efficiently and accurately.
How to Prepare for Upcoming Tests
Effective preparation is crucial for performing well on any exam. A well-organized approach helps reduce stress and increases the likelihood of success. By consistently reviewing material, practicing problems, and developing a strategy, you can strengthen your understanding and be ready to tackle the test with confidence.
Rather than cramming at the last minute, it’s important to start early and break down the content into manageable sections. Regular practice, reviewing past material, and focusing on weak areas can help you retain information more effectively and approach the test methodically.
Study Strategies for Success
Here are some tips for organizing your study routine and staying on track:
- Prioritize Key Concepts – Focus on the main ideas that are likely to be tested. Understanding these concepts will help you solve a variety of problems on the exam.
- Practice Consistently – Regular practice is essential for reinforcing what you’ve learned. Solve exercises, take mock tests, and review mistakes to improve your performance.
- Break Study Sessions into Blocks – Avoid long, unstructured study sessions. Break your study time into shorter, focused intervals, taking breaks in between to stay refreshed.
Time Management Techniques
Proper time management is vital to ensure that you cover all the necessary topics without feeling overwhelmed. Consider these approaches:
- Create a Study Schedule – Plan ahead and allocate time for each subject or concept. This allows you to track your progress and ensures that you don’t overlook important areas.
- Stick to a Consistent Routine – Consistency is key to reinforcing what you’ve learned. Try to study at the same time each day to make it a habit.
- Avoid Procrastination – Waiting until the last minute can lead to unnecessary stress. Stay disciplined and complete your assignments on time to avoid last-minute cramming.
By following these strategies and staying organized, you’ll be better prepared to approach your upcoming tests confidently and effectively. The goal is to review material thoroughly, focus on key areas, and stay consistent in your efforts. With the right preparation, you can perform to the best of your ability.