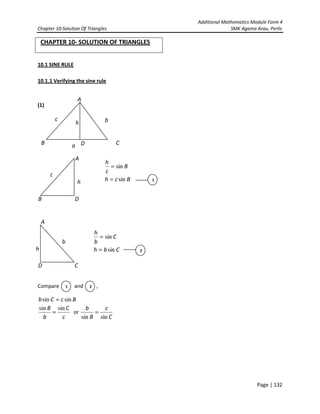
When preparing for a critical assessment in the field of mathematics, a comprehensive understanding of key concepts is crucial. Whether you’re reviewing formulas, tackling complex problems, or refining your technique for specific question types, each step contributes to improving your overall performance. This section provides valuable resources to help you navigate through these challenges with confidence.
By breaking down essential principles and offering detailed explanations, we aim to provide a structured approach to mastering the material. The solutions presented will guide you through various problem types, ensuring that you’re well-equipped to tackle any situation that arises during your evaluation.
Take your time to explore the methods and strategies outlined here. By applying these tips, you can enhance your problem-solving abilities and boost your understanding of the subject matter. With careful preparation and a clear grasp of the fundamentals, success becomes much more attainable.
Geometry Semester 1 Exam Answer Key
This section provides a comprehensive guide to the solutions for the first-half mathematics assessment. It is designed to help students understand the reasoning behind each step, offering clarity on the process of solving typical problems encountered in the test. By working through these solutions, you can gain insight into the correct approaches and deepen your understanding of the material.
Step-by-Step Solutions
Each problem is carefully broken down into smaller, manageable steps to ensure a clear explanation of the methodology. Following this structure will help reinforce your problem-solving skills and build confidence in tackling similar challenges in the future. Understanding the solution process is just as important as knowing the correct answers, as it allows you to apply these techniques to new situations.
Common Problem Types and Strategies
Throughout the test, you may encounter specific question formats, such as those requiring geometric proofs, calculations involving area and volume, or angle relationships. Familiarity with these types of problems allows you to focus on the strategy for solving rather than struggling with unfamiliar questions. Mastering these techniques is key to performing well in the assessment.
Key Concepts to Master for the Exam
Understanding the foundational ideas behind the problems you’re likely to encounter is essential for success in any assessment. Mastering these core concepts not only improves problem-solving efficiency but also builds a solid framework for tackling more complex challenges. Focusing on these key principles will help you approach the test with confidence and clarity.
Core Principles to Review
Start by revisiting the fundamental principles that form the basis of most questions. These include understanding relationships between shapes, theorems related to angles, and properties of geometric figures. Solidifying your grasp of these topics ensures you can quickly identify which methods to apply in a variety of problem scenarios.
Advanced Techniques and Strategies
Once you’re comfortable with the basics, dive into more advanced techniques such as proving theorems, working with coordinate systems, and solving complex measurement problems. Mastery of these topics will help you tackle the trickier questions that require a deeper level of reasoning and calculation.
Understanding Geometry Basics and Principles
To succeed in any mathematical evaluation, it is crucial to first establish a strong foundation in the basic principles that govern the subject. A clear understanding of these fundamental concepts allows you to approach more complex problems with ease. In this section, we will explore the core elements that form the bedrock of the subject and provide the necessary tools for tackling various challenges.
Essential Elements to Review
Familiarity with key components is essential for mastering the subject. The following topics form the core structure:
- Shapes and Figures: Recognizing different forms and their properties.
- Angles and Measurements: Understanding angle types, their relationships, and measurement techniques.
- Line and Plane Relationships: Exploring the connections between points, lines, and surfaces.
Core Theorems and Postulates
Grasping the core theorems and postulates is key to solving problems effectively. Focus on the following critical ideas:
- Pythagorean Theorem: A fundamental relationship in right-angled triangles.
- Congruence and Similarity: Understanding when shapes are identical or proportionally scaled.
- Angle Sum Property: The sum of angles in triangles and polygons.
Step-by-Step Solutions for Common Problems
Solving complex problems becomes easier when broken down into manageable steps. By following a systematic approach, you can address each component of a problem one by one, ensuring clarity and accuracy. This section will guide you through common types of problems, providing a clear path from start to finish.
Solving Triangle Problems
One of the most common problem types involves triangles. Whether you’re dealing with area, perimeter, or angles, a structured approach is key. Here’s how to approach these types of problems:
- Step 1: Identify the given information such as side lengths or angle measurements.
- Step 2: Choose the appropriate formula based on the type of triangle.
- Step 3: Apply the formula, simplifying where necessary.
- Step 4: Double-check your solution by verifying against known properties of triangles.
Working with Circles and Arcs
Problems involving circles often require a focus on radii, diameters, and angles. Here’s a typical step-by-step guide:
- Step 1: Determine the known values, such as radius or central angle.
- Step 2: Use the appropriate formula for area, circumference, or arc length.
- Step 3: Perform the necessary calculations and simplify the expression.
- Step 4: Review the result to ensure consistency with geometric properties of circles.
Important Formulas for Geometry Exams
Having a strong grasp of essential formulas is crucial for solving a wide range of problems. These equations serve as the foundation for many calculations, from determining areas and volumes to solving for unknown sides and angles. Mastering these formulas will help streamline the problem-solving process and increase your accuracy during assessments.
Here are some of the most important formulas you should commit to memory:
- Area of a Triangle: Area = 1/2 × base × height
- Perimeter of a Rectangle: Perimeter = 2 × (length + width)
- Circumference of a Circle: Circumference = 2 × π × radius
- Area of a Circle: Area = π × radius²
- Pythagorean Theorem: a² + b² = c²
These formulas are just the beginning, but they are fundamental for tackling various problem types. Understanding how to apply them in different scenarios will greatly improve your ability to solve questions quickly and efficiently.
Tips for Solving Geometry Word Problems
Word problems often present real-world scenarios that require careful analysis and logical thinking. The key to solving these challenges is breaking the problem down into smaller, more manageable steps. By focusing on the details and applying known concepts, you can solve even the most complex situations with ease.
Steps for Effective Problem Solving
Follow these steps to improve your approach to word problems:
- Read the problem carefully: Ensure you understand what is being asked and identify the given information.
- Sketch the situation: Drawing a diagram can often make it easier to visualize the relationships between different elements.
- Highlight key information: Underline or circle important numbers, terms, and clues that will help you solve the problem.
- Translate into mathematical terms: Convert the word problem into an equation or set of equations based on the relationships described.
- Double-check your solution: Verify that your answer makes sense in the context of the problem and matches the conditions given.
Common Mistakes to Avoid
Avoiding these errors can significantly improve your problem-solving accuracy:
- Misinterpreting the problem: Read carefully to ensure you are addressing the right question.
- Omitting important information: Sometimes, crucial details are hidden within the text, so pay attention to every part of the problem.
- Relying on assumptions: Base your solution strictly on the information provided, rather than making guesses.
How to Approach Proof-Based Questions
Proof-based questions require a logical and systematic approach, where each step must be supported by valid reasoning or known theorems. These types of problems test not only your knowledge of core concepts but also your ability to structure an argument and present a well-supported solution. The key is to break down the problem into smaller, logical steps and to justify each step carefully.
Step-by-Step Approach
When tackling a proof, follow these essential steps:
- Identify the given information: Carefully note the known facts and the relationships between them.
- State what needs to be proven: Understand the objective of the proof and make sure you’re clear about what you’re trying to establish.
- Choose appropriate theorems and postulates: Select known principles that are relevant to the situation and can help lead you to the solution.
- Work logically: Build the proof step by step, with each step following logically from the previous one. Provide justifications for each move.
- Conclude with the proven statement: End by explicitly stating that the desired result has been demonstrated.
Example of a Proof Structure
Here is an example of how a proof might be structured:
| Step | Explanation | Justification |
|---|---|---|
| 1 | State the given information. | Provide the facts or conditions provided in the problem. |
| 2 | Define what needs to be proven. | Clearly state the conclusion you are trying to prove. |
| 3 | Apply the appropriate theorem or property. | Cite the theorem, postulate, or definition you are using to justify your step. |
| 4 | Work through the logical steps to the conclusion. | Ensure each step logically follows from the previous one, supported by clear reasoning. |
| 5 | Conclude the proof. | Summarize that the desired result has been shown, using a concluding statement. |
Time Management Strategies for the Exam
Effective time management is essential for success during any assessment. With a limited amount of time to complete a variety of questions, it’s crucial to prioritize tasks, pace yourself, and stay focused. By developing a strategy to manage your time wisely, you can maximize your performance and reduce stress during the test.
Pre-Assessment Preparation
Preparation is key to effective time management. Before starting the assessment, consider the following:
- Familiarize yourself with the format: Understand the types of questions you will face and how much time each section should take.
- Prioritize topics: Focus on areas where you feel less confident, but don’t neglect your stronger areas entirely.
- Practice with a timer: Simulate test conditions by practicing with time limits to develop a sense of how to pace yourself.
During the Assessment
Once you begin, it’s important to keep track of time and adjust your approach accordingly:
- Read instructions carefully: Take a few moments to read through the directions for each section to avoid mistakes.
- Start with easier questions: Answer the questions you find most straightforward first to build momentum and ensure you secure those marks.
- Time yourself: Keep track of time for each section. If a question is taking too long, move on and return to it later if time permits.
- Don’t get stuck: If you encounter a particularly difficult question, don’t dwell on it for too long. Move on and come back with a fresh perspective if necessary.
Post-Assessment Review
After completing the test, take a few moments to review your work if time allows:
- Check for skipped questions: Ensure that you have answered all the questions. Double-check that you didn’t overlook anything.
- Review your answers: Quickly review your work to spot any obvious mistakes or errors, particularly in calculations or logic.
Reviewing Diagrams and Geometric Figures
Diagrams and figures are integral to solving problems in spatial reasoning. These visual representations often hold the key to understanding relationships between different elements and help simplify complex scenarios. By thoroughly analyzing the details in each diagram, you can identify patterns, relationships, and important values that will guide you toward the solution.
Key Elements to Examine in Diagrams
When reviewing a diagram, focus on the following key components:
- Labels: Pay attention to any labels, such as angles, lengths, and points, as they are crucial for applying formulas and theorems.
- Shapes and Figures: Identify the specific shapes in the diagram (e.g., triangles, circles, quadrilaterals) and note their properties and relationships.
- Marked Information: Look for any marked information such as angles, side lengths, or specific relationships between figures that can help you form equations or apply theorems.
Strategies for Analyzing Geometric Figures
To effectively analyze a figure, follow these strategies:
- Draw additional lines: If needed, draw auxiliary lines to create known shapes, such as triangles or rectangles, that can help you find missing information.
- Use symmetry: If the figure exhibits symmetry, use it to simplify the problem and identify equivalent angles or sides.
- Apply geometric properties: Use properties of shapes, such as the Pythagorean theorem, parallel lines, or angle relationships, to uncover hidden information.
Essential Theorems to Know for Geometry
Understanding fundamental principles and theorems is crucial for solving problems effectively in spatial reasoning. These theorems provide the necessary foundation for making logical deductions and solving complex problems. Mastering these key principles will not only help you in tests but also in real-world applications where geometric reasoning is required.
Core Theorems to Master
Here are some of the essential theorems that every student should be familiar with:
- Pythagorean Theorem: This fundamental theorem relates the sides of a right triangle. It states that the square of the hypotenuse equals the sum of the squares of the other two sides (a² + b² = c²).
- Properties of Parallel Lines: When a transversal cuts two parallel lines, alternate interior angles are congruent, and corresponding angles are equal.
- Angle Sum Theorem: The sum of the interior angles of any triangle is always 180 degrees.
- Congruence Theorems (SSS, SAS, ASA): These rules help determine whether two triangles are congruent based on side or angle measurements.
Advanced Theorems and Postulates
As you progress, it’s essential to also be familiar with these advanced concepts:
- Circle Theorems: Important rules about the relationships between the angles, radii, and tangents of circles.
- Angle Bisector Theorem: This theorem states that the angle bisector of a triangle divides the opposite side into segments proportional to the adjacent sides.
- Triangle Inequality Theorem: The sum of the lengths of any two sides of a triangle must be greater than the length of the third side.
Common Mistakes to Avoid During the Exam
While tackling problems that require spatial reasoning, it’s easy to make small but significant errors that can cost valuable points. Recognizing and avoiding these common pitfalls can significantly improve performance and ensure that you apply the right concepts effectively. Being aware of these mistakes allows you to focus more on the logical steps required for solving each question.
Key Mistakes to Watch Out For
Here are some of the most frequent errors made during tests in spatial reasoning:
- Misinterpreting Diagrams: Often, students overlook key details in visual representations, such as mislabeling points or missing relationships between elements. Always double-check every label and connection in the figure.
- Incorrect Application of Theorems: Applying the wrong formula or principle to a problem can lead to incorrect results. Take time to carefully read the question and ensure that you use the appropriate method for each scenario.
- Rushing Through Calculations: Hasty calculations can lead to simple arithmetic mistakes. It’s essential to slow down and perform each calculation carefully, checking your work as you go.
How to Prevent These Mistakes
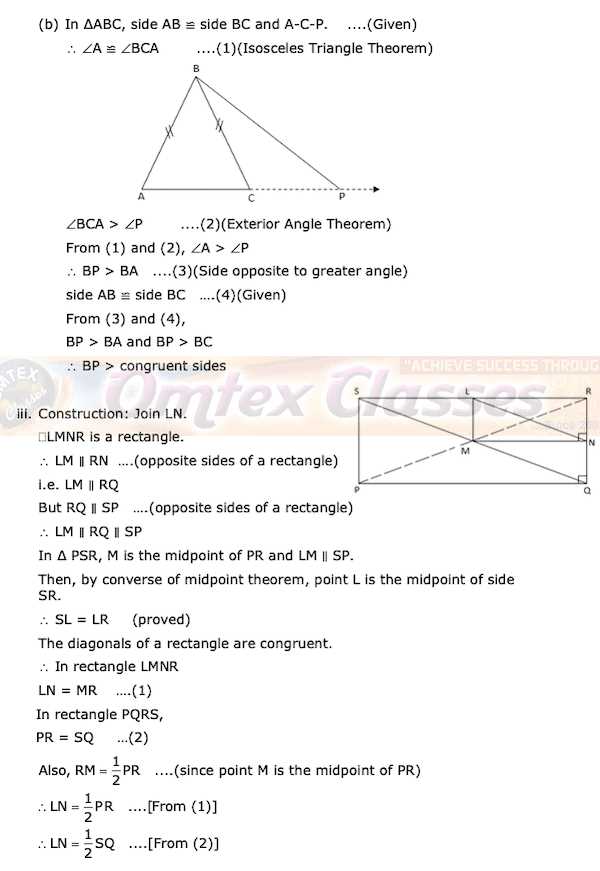
Preventing errors comes down to preparation and attentiveness:
- Review Problem Requirements: Before beginning to solve a problem, ensure that you understand all of its requirements and constraints.
- Check Units and Measurements: Ensure that all units are consistent throughout the problem, and don’t forget to convert them when necessary.
- Manage Your Time: Avoid spending too much time on a single question. If stuck, move on and come back to it later with fresh eyes.
How to Use the Answer Key Effectively

Using a solution guide properly can be a valuable tool for reinforcing your understanding and correcting mistakes. While it’s important to check your work, it’s equally crucial to use the guide not just to confirm your answers but also to learn from any errors. A thoughtful approach to using the solution set will help improve your problem-solving skills and deepen your knowledge.
Maximizing Learning Through Solutions
Here’s how to make the most of the provided solutions:
- Review Step-by-Step: Instead of just looking at the final answer, study the entire solution process. This will help you understand the reasoning behind each step and avoid future mistakes in similar problems.
- Identify Mistakes: If your solution is incorrect, analyze where the mistake occurred. Compare your work with the correct steps to pinpoint your error and make sure you understand why your method was flawed.
- Learn from Alternatives: Sometimes, the solution guide may offer a different method than the one you used. Compare both approaches and understand the reasoning behind each. This could introduce you to more efficient strategies for solving problems.
Using Solutions for Future Success
Beyond just correcting your mistakes, the solution guide can help you prepare for upcoming challenges:
- Strengthen Weak Areas: If you notice consistent mistakes in certain types of problems, focus on those areas for further practice. The solutions can give you insights into techniques and strategies to master these topics.
- Practice Independently: Once you’ve reviewed the correct solutions, attempt similar problems on your own without looking at the guide. This will help reinforce your understanding and improve retention.
Practice Questions and Solutions
Engaging with practice problems is a crucial step in reinforcing your skills and ensuring a strong understanding of key concepts. By working through problems, you can identify areas of weakness, improve problem-solving strategies, and gain confidence in applying different methods. Below, we have compiled several example problems along with their detailed solutions to guide you through the process.
Sample Problems and Solutions
Here are a few practice questions with step-by-step solutions:
| Problem | Solution |
|---|---|
| Find the perimeter of a rectangle with length 8 cm and width 5 cm. | The perimeter of a rectangle is calculated using the formula P = 2(l + w). Substituting the given values: P = 2(8 + 5) = 2 × 13 = 26 cm. |
| Calculate the area of a triangle with base 10 cm and height 6 cm. | The area of a triangle is calculated using the formula A = 1/2 × base × height. Substituting the given values: A = 1/2 × 10 × 6 = 30 cm². |
| Find the volume of a cylinder with radius 4 cm and height 10 cm. | The volume of a cylinder is calculated using the formula V = πr²h. Substituting the given values: V = π × 4² × 10 = 3.14 × 16 × 10 = 502.4 cm³. |
Tips for Practice
As you work through these questions, consider the following tips to maximize your learning:
- Break Down Each Step: When solving problems, break each question into smaller steps to make the process more manageable and avoid skipping important details.
- Use Multiple Approaches: If possible, try solving the problem using different methods to see which one is most efficient for you.
- Keep Practicing: Consistent practice will help solidify the concepts, improve your speed, and boost your overall confidence.
Understanding Different Types of Questions
When tackling problems related to shapes, figures, and spatial relationships, it is important to understand the variety of question types you may encounter. These problems can range from simple calculations to more complex proofs, each requiring a different approach and set of skills. By recognizing the type of question, you can apply the appropriate methods to solve it efficiently and accurately.
Broadly, questions can be categorized into different types such as measurement problems, reasoning questions, and conceptual inquiries. Each category demands specific strategies for success. For instance, some problems may require you to calculate areas, volumes, or perimeters, while others may involve logical deductions or require proof of a particular statement or relationship.
Below are some common types of problems you might face:
- Calculation-Based Problems: These involve solving for numerical values such as the area of a shape or the volume of a solid. They often require the use of specific formulas.
- Proof Questions: These require you to demonstrate the validity of a particular relationship or property using logical steps and theorems.
- Conceptual Understanding Questions: These may ask you to explain relationships between shapes, properties of figures, or definitions, testing your comprehension rather than just mathematical ability.
- Word Problems: These questions are framed in a real-world context, requiring you to translate the information into mathematical terms and apply the appropriate formula or method.
Being familiar with these categories will help you navigate through different types of questions and approach each one with the right mindset and tools. This will not only improve your efficiency but also your confidence in solving complex problems.
Analyzing Multiple-Choice Questions
Multiple-choice questions are commonly used to assess your understanding of mathematical concepts and problem-solving skills. These types of questions offer several possible answers, from which you must choose the correct one. While they may seem straightforward, careful analysis and strategic thinking are required to navigate through them effectively. By understanding the structure of the questions and applying logical reasoning, you can significantly improve your chances of selecting the right answer.
When facing a multiple-choice question, it’s essential to break it down into smaller parts. Start by reading the problem carefully to identify what is being asked. Then, evaluate each option methodically to see which one fits the conditions given in the question. Sometimes, you can eliminate one or two incorrect answers right away, narrowing your choices and making it easier to identify the correct one.
| Step | Action |
|---|---|
| Step 1 | Read the question thoroughly to understand what is being asked. |
| Step 2 | Identify any important clues or information in the question that can help eliminate incorrect answers. |
| Step 3 | Evaluate each answer choice to see if it matches the conditions or calculations required. |
| Step 4 | Eliminate clearly wrong answers to increase the probability of selecting the right one. |
| Step 5 | Double-check your choice by revisiting the problem and confirming your answer makes sense in context. |
By following this step-by-step approach, you can systematically analyze each multiple-choice question and confidently determine the correct answer. Remember, practice is key to improving your ability to quickly and accurately analyze these questions under time constraints.
How to Improve Your Exam Score
Improving your performance on a test requires both strategic preparation and effective study techniques. Whether you are revisiting core concepts or refining your problem-solving skills, there are several key practices you can adopt to boost your confidence and enhance your results. A well-structured approach to reviewing material and consistent practice can make a significant difference when it comes to achieving better outcomes.
Start by identifying your strengths and weaknesses. Focus on areas where you feel least confident and allocate more time to these topics. Use a variety of resources such as textbooks, online tutorials, and practice problems to reinforce your understanding. It’s also helpful to break down complex problems into smaller, more manageable steps, ensuring that you don’t overlook important details.
Additionally, make time for regular practice sessions. The more problems you solve, the more familiar you become with different types of questions. Try to simulate the testing environment by timing yourself and working through problems without assistance. This will help you improve your time management skills, so you’re better equipped to handle the real test.
Lastly, ensure that you review your mistakes. After completing practice tests or exercises, go back and carefully analyze the errors you made. Understanding why you got an answer wrong can help you avoid similar mistakes in the future and reinforce your learning.
Common Exam Formats Explained
Understanding the structure of a test is crucial for effective preparation. Different types of assessments require different strategies, so it’s important to familiarize yourself with the most common formats. Whether the focus is on problem-solving, application of concepts, or theoretical understanding, each format offers unique challenges and opportunities for demonstrating your knowledge.
One of the most common types of questions you’ll encounter involves multiple-choice items. These questions present you with a set of options, and you must select the one that best answers the question. While they can be less time-consuming, they also require careful reading and attention to detail to avoid common traps.
True/false questions are another popular format, testing your ability to recognize the accuracy of statements. These questions often challenge you to apply concepts quickly and determine their validity, requiring both knowledge and critical thinking. Pay attention to qualifiers like “always” or “never” that can change the meaning of the statement.
Another prevalent format is short-answer questions, which require you to provide a direct and concise response. These questions typically assess your ability to recall and apply formulas, definitions, or key concepts to solve problems or explain relationships.
Problem-solving questions, often seen in more complex tests, involve working through detailed steps to arrive at a solution. These questions may require you to demonstrate a thorough understanding of processes or to apply a combination of concepts. They tend to be more time-intensive, so practicing step-by-step solutions is crucial to developing confidence.
By understanding these common formats, you can tailor your study strategies to improve performance across different types of questions. Practice with each type to become familiar with the demands of each format and to strengthen your test-taking skills.
Final Tips for Exam Day Success
As the day of your assessment approaches, it’s important to focus on strategies that will help you perform at your best. A solid plan on the day of the test can make all the difference in how well you manage your time, energy, and focus. These final steps are crucial in ensuring you are prepared and confident.
Preparation the Night Before
- Review key concepts: Spend the evening before the test going over major topics, formulas, and problem types. Focus on areas where you feel less confident.
- Get a good night’s sleep: Rest is essential for concentration and memory recall. Avoid staying up late cramming, as a well-rested mind performs better.
- Prepare materials: Make sure you have all the tools you need–pencils, erasers, a calculator, or any other materials allowed for the assessment.
On Test Day
- Arrive early: Give yourself plenty of time to get settled and mentally prepared before starting the assessment.
- Read instructions carefully: Pay close attention to any instructions given at the start of the test. This can help avoid mistakes and misunderstandings.
- Manage your time: Keep an eye on the clock, and make sure to allocate time for each section of the test. If you get stuck, move on and return to difficult questions later.
- Stay calm: Keep a positive attitude, even if you encounter challenging questions. Deep breathing and staying focused can help reduce anxiety.
By following these strategies, you will enhance your chances of success and approach the test with the right mindset. Being well-prepared, rested, and confident is the key to performing at your best.