
For students aiming to succeed in high-level exams, tackling complex mathematical problems effectively is crucial. These assessments often include a section that challenges your ability to apply theoretical knowledge to practical scenarios. The key to excelling lies in understanding how to approach such tasks methodically, demonstrating your problem-solving skills in a clear and organized way.
In this guide, we will explore how to navigate and solve the challenging questions presented in these sections. By breaking down problems step-by-step, recognizing common pitfalls, and adopting the best strategies, you can maximize your performance. Success in these areas not only requires a deep understanding of core concepts but also the ability to communicate your solutions clearly and accurately.
Whether you are preparing for a similar exam or revisiting past materials, mastering these problem-solving techniques will give you the confidence needed to excel. Let’s dive into effective methods for approaching these questions and ensure you’re fully prepared for any challenge ahead.
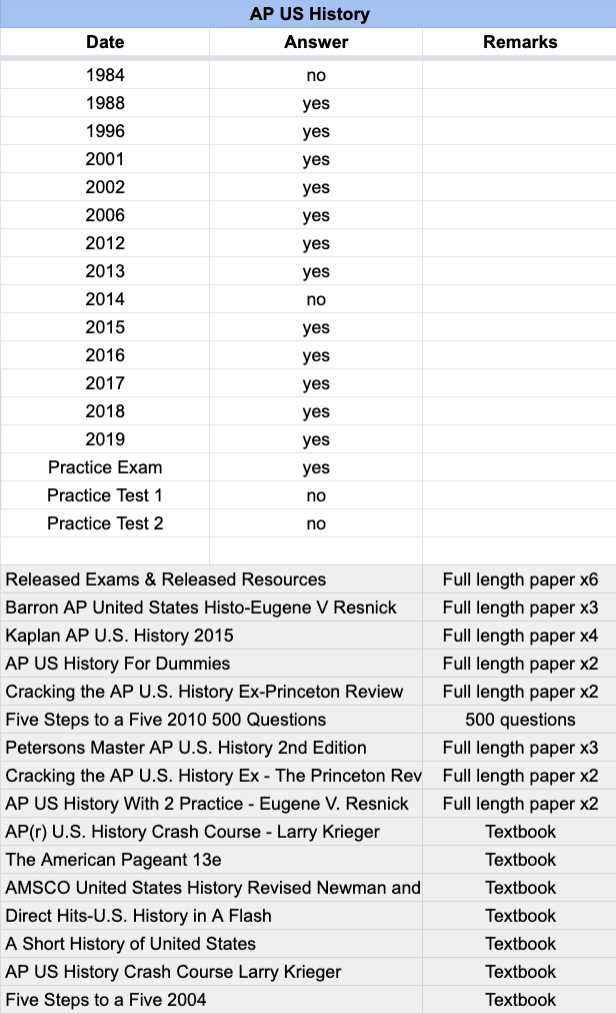
2006 AP Calculus AB Free Response Answers
When preparing for high-level math exams, it’s important to understand how to approach and solve the most demanding questions. These sections are designed to test your ability to apply mathematical principles in various contexts. Achieving high marks requires both technical proficiency and the ability to present your reasoning clearly and logically.
Looking at past exam materials can provide valuable insight into the types of problems that frequently appear. By reviewing these problems and the corresponding solutions, you can gain a better understanding of the expectations and improve your problem-solving techniques. The key is not just solving the equations, but also showing your thought process in a structured and concise manner.
This section will provide detailed guidance on how to work through challenging questions, highlighting common strategies and offering tips to help you avoid mistakes. Whether you’re studying independently or reviewing with a tutor, mastering these concepts is crucial for achieving the best results in any exam format.
Understanding AP Calculus Free Response Format
In advanced math exams, certain sections are designed to assess your ability to solve complex problems and demonstrate clear reasoning. These questions often require more than just a numerical solution; you must show your process, from the initial setup to the final result. This format tests not only your knowledge but also your ability to communicate your thought process in a structured and logical manner.
The format of these questions typically consists of multi-step problems that involve multiple mathematical concepts. It’s essential to break down each problem into smaller parts, ensuring that your work is clearly presented and each step is justified. The solutions should reflect your understanding of the subject, as well as your capacity to apply concepts effectively to new scenarios.
By understanding the format and expectations of these sections, you can approach each question with confidence. Focus on presenting your work in an organized way, paying attention to details, and making sure your answers are both correct and well-explained.
How to Approach Free Response Questions
When facing multi-step questions in an advanced math exam, it’s crucial to approach each one methodically. Rather than jumping directly to calculations, take the time to understand what the problem is asking and plan your steps carefully. This structured approach not only helps ensure accuracy but also allows you to demonstrate your full understanding of the material.
Breaking Down the Problem
The first step in solving complex questions is to carefully analyze the given information. Identify the key components, such as what is known and what needs to be found. It can be helpful to underline or highlight important values and equations. This organization will guide you through the rest of the problem-solving process, ensuring that you don’t overlook critical details.
Show Your Work Clearly
Mathematical problems often require you to present your reasoning step by step. Be sure to show each part of your work in a clear, logical order. For example, when solving equations or applying formulas, write down every step, even if it seems obvious. This will not only help avoid errors but also demonstrate your problem-solving approach. Clarity is key to ensuring that you get full credit for your effort, even if the final answer is not completely correct.
Overview of 2006 AP Exam Content
The advanced math exam is structured to assess a wide range of skills and knowledge. It includes questions that test both theoretical understanding and practical application of key concepts. The content is designed to challenge students’ abilities to solve problems, interpret complex scenarios, and demonstrate reasoning in a clear and logical way.
The exam typically covers a variety of topics, each requiring students to use different techniques and approaches. From analyzing functions and their properties to applying integration and differentiation, the questions are carefully crafted to assess how well students can apply mathematical principles to solve real-world problems. Understanding the scope and structure of the exam is crucial for effective preparation, as it allows you to focus on the most relevant topics and ensure that you are ready for every challenge that may arise.
Key Strategies for Effective Problem Solving
To succeed in solving complex mathematical problems, it’s essential to approach each challenge with a systematic strategy. This ensures that you can break down the problem into manageable steps and apply the appropriate techniques to find a solution. Effective problem-solving requires both understanding the underlying principles and using a logical process to reach the correct answer.
Understanding the Problem
The first step in any problem-solving process is to carefully read and comprehend the given information. Pay attention to what is being asked and identify any key values, variables, or constraints. A clear understanding of the problem is critical for choosing the right approach and avoiding unnecessary mistakes.
Organizing Your Approach
Once the problem is understood, organize your work. This can involve drawing diagrams, writing down equations, and identifying relationships between different parts of the problem. A clear, step-by-step approach will keep you on track and make it easier to follow your reasoning from start to finish.
| Strategy | Explanation |
|---|---|
| Break it Down | Divide complex problems into smaller, simpler parts to solve one at a time. |
| Show Your Work | Clearly write out each step to demonstrate your reasoning, even if it seems simple. |
| Double-Check Calculations | Review your math at each step to ensure no errors in arithmetic or logic. |
Breaking Down Complex Calculus Problems
When faced with complicated mathematical problems, it’s essential to break them down into smaller, more manageable steps. This approach allows you to understand the structure of the problem and apply the right techniques to solve it. By focusing on one part at a time, you can prevent feeling overwhelmed and increase your chances of reaching the correct solution.
Step-by-Step Problem Solving
One of the most effective strategies for tackling challenging questions is to divide the problem into distinct parts. This way, you can focus on solving each section systematically before moving on to the next. Here’s how to approach it:
- Identify Known Information: Begin by clearly identifying all the given values, equations, or conditions. This gives you a foundation to work from.
- Determine What is Unknown: Understand exactly what you need to find and how it relates to the given information.
- Choose the Right Method: Decide on the appropriate mathematical techniques, such as differentiation, integration, or finding limits, to solve the problem.
- Work Through Each Step: Solve each part of the problem sequentially, ensuring clarity and logical progression from one step to the next.
Common Mistakes to Avoid
While breaking down a problem is crucial, it’s just as important to stay mindful of common errors that can derail your progress. These mistakes can stem from incorrect assumptions, skipped steps, or careless calculations. To avoid these pitfalls:
- Always double-check your work after each step.
- Be sure not to overlook important values or conditions that affect the outcome.
- Don’t rush through the problem. Take your time to think through each part carefully.
Common Mistakes in Free Response Sections
When tackling detailed mathematical problems in exams, it’s easy to make small errors that can significantly affect your performance. These mistakes often stem from rushing through steps or overlooking important details, which can lead to incorrect results even if the underlying concepts are understood. Being aware of these common pitfalls can help you avoid them and improve the quality of your work.
Typical Errors to Watch For
Many students fall into the trap of making simple yet impactful mistakes while solving complex problems. Here are some of the most common issues:
- Skipping Steps: Failing to show every intermediate step can cause confusion and may lead to errors later in the problem.
- Misinterpreting the Question: Not fully understanding what the problem is asking can lead you to apply the wrong techniques or miss crucial information.
- Rounding Too Early: Rounding values too soon can reduce the accuracy of your final answer. Always try to keep full precision until the final step.
- Overlooking Units: In problems involving real-world applications, not paying attention to the units can result in incorrect calculations or meaningless answers.
- Incorrect Use of Formulas: Using the wrong formula or applying a formula in the wrong context is a common mistake, especially under time pressure.
How to Avoid These Mistakes
Here are some strategies to help reduce the risk of these errors:
- Read Carefully: Always take the time to read the problem thoroughly, making sure you understand what is being asked before starting to solve it.
- Show Your Work: Break down the problem step by step, writing out each part of the solution to ensure clarity and to catch mistakes early.
- Check Your Work: After completing the problem, review each step to ensure that no errors were made and that all conditions were met.
- Practice Time Management: Allocate enough time to each question so you don’t feel rushed, which can lead to careless errors.
Tips for Time Management During the Exam
Effective time management during an exam is crucial to ensure that you can complete all the questions with accuracy and clarity. Managing time well allows you to dedicate enough attention to each problem without rushing through them. By planning your approach and staying organized, you can maximize your performance and minimize stress during the exam.
Prioritize Your Questions
Start by quickly scanning through the entire exam to identify questions that seem more straightforward or ones that you are most confident in. Tackling easier questions first can help build momentum and save time for more complex problems later. However, make sure to allocate enough time for each question to avoid leaving any unfinished.
Allocate Time to Each Section
Once you have a sense of the overall structure of the exam, allocate time to each section based on its difficulty and point value. Set a timer for each section to help you stay on track and avoid spending too much time on a single question. If you get stuck on a difficult problem, move on and return to it later to ensure that you don’t waste precious time.
Use the Process of Elimination
When you encounter questions that seem tricky, use the process of elimination to narrow down the possible answers. Even if you’re not entirely sure of the solution, eliminating obviously incorrect choices can increase your chances of selecting the correct one, saving you time on questions that would otherwise require extensive calculations.
Stay Calm and Focused
Time pressure can sometimes lead to panic, which can cloud your judgment. Stay calm and focused throughout the exam. If you feel yourself getting anxious, take a deep breath and remind yourself that you have prepared well. Keeping a steady pace will help you maintain clarity and efficiency as you work through each question.
Essential Calculus Concepts for Success
To excel in mathematics, a strong grasp of fundamental principles is necessary. Understanding key concepts provides the foundation for solving a variety of problems with confidence. Mastery of these concepts allows you to navigate complex problems more effectively and apply your knowledge to a wide range of scenarios. Whether dealing with rates of change, accumulation, or optimization, these core ideas will serve as the building blocks for solving more advanced challenges.
Limits and Continuity
One of the most crucial ideas is the concept of limits, which allows us to understand the behavior of functions as they approach certain points. Continuity, on the other hand, examines whether a function behaves predictably at every point in its domain. Understanding how to calculate limits and determine continuity ensures that you can evaluate functions with precision, a critical skill for solving problems related to derivatives and integrals.
Derivatives and Integrals
Derivatives represent the rate of change of a function, offering insight into how values change in response to variations in the input. Mastering the rules for differentiation, such as the product, quotient, and chain rules, allows you to analyze complex functions efficiently. Similarly, integrals are essential for finding areas under curves and determining the accumulation of quantities. Understanding how to compute both definite and indefinite integrals is key to solving problems in a variety of contexts, from motion to optimization.
How to Show Work on Free Response
Presenting your process clearly is essential in any problem-solving environment, especially during exams. Showing each step in your solution not only demonstrates your understanding but also allows you to receive partial credit for your approach, even if the final answer is incorrect. By organizing your work systematically and explaining each stage, you make it easier for graders to follow your logic and award appropriate points for your effort.
Start with a Clear Problem Setup
Before diving into calculations, take a moment to set up the problem clearly. State what you are trying to find and define any variables if necessary. This helps both you and the grader keep track of your work. A well-written problem setup is especially important for complicated problems where different concepts and operations are involved.
Show Each Step Clearly
As you solve the problem, make sure to show every step of your reasoning. Whether you’re simplifying an expression, applying a formula, or using a specific method, it’s important to write down each action you take. This shows that you understand the underlying concepts and ensures that even if a mistake is made, the grader can follow your logic to award points where appropriate. If you are working through a multi-step process, break it down into smaller, manageable pieces to maintain clarity and accuracy.
Explain Your Work
Be concise but thorough in your explanations. A brief comment or note explaining why you performed a specific step can demonstrate your thought process and reassure the grader that you know why you’re making certain choices. For example, if you’re applying a rule or formula, mention why it’s applicable to the current problem. This approach can go a long way in securing partial credit.
Review and Double-Check
After completing the problem, review your work. Check for simple arithmetic errors, missing steps, or unclear explanations. This final check is essential to ensure that your solution is accurate and complete, and that the grader can easily follow the flow of your reasoning.
Mastering Graphing Techniques in Calculus
Graphing is an essential skill for understanding mathematical functions and relationships. Being able to visualize functions not only helps to interpret their behavior but also allows you to solve complex problems more efficiently. By mastering graphing techniques, you can identify key features of a function such as intercepts, asymptotes, and critical points, which are critical in solving both theoretical and applied problems.
Plotting Key Points
Start by plotting key points on the graph, such as the x- and y-intercepts, maxima, minima, and inflection points. These are often the most important features of the graph and provide valuable insight into the function’s behavior. Use substitution to find exact values and ensure accuracy. Identifying symmetry, such as even or odd functions, can also help you quickly sketch a graph and predict its overall shape.
Understanding Asymptotic Behavior
Asymptotes–whether vertical, horizontal, or oblique–are critical in understanding the end behavior of a function. Recognizing where a function approaches but never quite reaches a line can guide your graphing process and help with problem-solving. For rational functions, analyze the degrees of the numerator and denominator to determine the presence and type of asymptotes.
Analyzing Derivatives and Slopes

The derivative of a function represents the slope of the tangent line at any given point. Understanding how the derivative influences the shape of the graph is key. Where the derivative is positive, the graph is increasing; where it is negative, the graph is decreasing. Identifying critical points–where the derivative is zero or undefined–can help you locate local extrema and inflection points, which are essential for graphing more complex functions.
Using Multiple Approaches to Solve Problems
When tackling mathematical challenges, relying on a single method may not always provide the most efficient or accurate solution. Exploring different approaches allows you to find the best strategy for each problem and ensures a deeper understanding of the concepts involved. By leveraging various techniques, you can verify your results, strengthen your problem-solving skills, and enhance your ability to think critically under pressure.
Analyzing Problems from Different Angles
To effectively approach a problem, it’s important to consider multiple perspectives. For example, if solving an equation, you might begin with algebraic manipulation, but then apply graphical analysis to visualize the solution. Each method offers unique insights and can lead you to discover alternative paths to the answer.
- Algebraic Methods: Manipulating equations, factoring, or using substitution can help you simplify the problem and isolate variables.
- Graphical Methods: Plotting functions or using visual aids can provide a clearer understanding of the relationships between variables.
- Numerical Methods: Estimating solutions through approximation techniques can be especially useful when exact solutions are difficult to obtain.
- Conceptual Methods: Relying on key principles or theorems can guide you toward an efficient solution without extensive calculations.
Verifying Solutions
After arriving at a potential solution, it’s essential to verify its correctness. If possible, use a different method to confirm your results. For instance, if you’ve solved a differential equation algebraically, check your answer by graphing the solution. This validation process helps ensure that your solution is not only correct but also robust across different approaches.
By mastering multiple problem-solving techniques, you’ll become a more versatile thinker and be better equipped to handle complex challenges in mathematics and beyond.
Reviewing the 2006 Free Response Answers
Reviewing solutions to complex problems provides valuable insights into problem-solving techniques and strategies. By carefully examining step-by-step solutions, you can better understand the reasoning behind each step and learn how to approach similar problems in the future. This process of reflection helps identify common mistakes, refine your skills, and improve your overall understanding of mathematical concepts.
Key Insights from the Solutions
When reviewing the solutions, focus on the underlying strategies used to solve each problem. Pay attention to the methods chosen, how they are applied, and the logic used to transition between steps. Whether you solve the problem with algebraic manipulation, graphical methods, or numerical approximations, understanding the reasoning behind each decision is crucial for mastering the material.
| Problem Type | Method Used | Key Takeaways |
|---|---|---|
| Optimization Problems | Critical points, second derivative test | Focus on finding critical points and verifying concavity to determine maxima and minima. |
| Integration Problems | Substitution, integration by parts | Choose the appropriate method based on the form of the integrand to simplify the process. |
| Differential Equations | Separation of variables, integrating factor | Use separation or an integrating factor to solve first-order differential equations effectively. |
Identifying Common Mistakes
While reviewing the solutions, it’s important to identify common errors that may have been made. For example, misapplying a derivative rule or overlooking a boundary condition can result in incorrect solutions. By recognizing these mistakes, you can avoid them in future problems and strengthen your mathematical intuition.
Interpreting Question Requirements Clearly
Understanding the precise requirements of a question is the foundation of solving any problem efficiently. Often, mathematical problems contain multiple components that must be carefully interpreted to avoid missteps. Whether it’s identifying what the question is asking for, recognizing key constraints, or ensuring that all parts of the question are addressed, clarity in interpretation is crucial for successful problem-solving.
Key Steps to Effective Interpretation
To approach problems correctly, begin by reading the question thoroughly and highlighting critical information. This includes recognizing given values, identifying what needs to be found, and understanding the context or assumptions that influence the solution. Sometimes, questions may contain multiple subparts or require a sequence of steps to be performed in a particular order. Ensuring you fully grasp these nuances will prevent unnecessary mistakes.
| Step | Action | Why It’s Important |
|---|---|---|
| Read Carefully | Highlight key information such as given variables, constraints, and what is being asked. | Helps identify what the problem is truly asking for and avoids misunderstandings. |
| Break Down the Question | Separate the problem into manageable parts, addressing each aspect methodically. | Prevents overlooking important details or missing out on necessary steps for a solution. |
| Identify Specific Terms | Look for terms like “at this point”, “find the limit”, “maximum”, “minimize”, or “approximate”. | These guide your approach, helping you decide on methods or techniques to apply. |
Common Pitfalls in Question Interpretation
Misinterpreting a question’s requirements can lead to incorrect methods or results. One common mistake is overlooking specific constraints or requirements, such as whether a question asks for an exact answer or an approximation. Another issue is confusing what is being asked for–whether the question is requesting a derivative, integral, or other solution types. By carefully analyzing each part of the question and asking yourself what is being specifically requested, you can avoid these pitfalls.
Improving Accuracy with Practice Problems
Practicing problems is one of the most effective ways to improve your precision when solving complex mathematical challenges. Repetition not only strengthens your understanding of key concepts but also enhances your ability to apply techniques correctly under time pressure. Regular practice allows you to familiarize yourself with various problem types, recognize common patterns, and improve your overall problem-solving speed.
Why Consistent Practice Matters
By working through a variety of problems, you can develop a deeper understanding of the material and become more comfortable with different strategies. As you encounter new problems, you’ll learn how to approach each situation, assess the best method to use, and avoid common mistakes. Additionally, practicing regularly helps you build confidence in your skills, ensuring that you are prepared for any question that may arise during an exam.
Effective Techniques for Practice
To maximize the benefits of practice, it’s important to not only solve problems but to analyze the solutions thoroughly. After completing a problem, review the steps taken and check for any errors. This reflection process is essential for identifying areas that need improvement. Focus on challenging problems that test your understanding and encourage you to apply concepts in new ways.
Additionally, working with timed practice sessions can simulate exam conditions, helping you become more efficient in managing your time. By setting a specific time limit for each problem, you can develop a sense of urgency and practice thinking critically under pressure.
How Free Response Affects Your Score
The portion of the exam that requires written explanations plays a crucial role in determining your final score. These sections assess not only your ability to solve problems but also your understanding of underlying concepts and your ability to communicate solutions clearly. Effectively tackling this part of the exam can have a significant impact on your overall performance, as it often accounts for a large portion of the total score.
Factors That Influence Scoring
There are several factors that contribute to how well you perform on the written section, including:
- Accuracy of Your Solutions: The most important aspect is providing the correct answer. However, simply writing down the final result is not enough; your work must demonstrate that you understand the steps and reasoning involved.
- Clarity and Organization: Examiners look for clear, logical explanations. Properly structured responses, with each step outlined and explained, can help you score better, even if the final answer is incorrect.
- Justification of Your Approach: Being able to justify your methods shows a deep understanding of the topic. Even if you make an error along the way, if you can explain your reasoning clearly, it could still earn you partial credit.
Maximizing Your Score Potential
To maximize your score on the written portion, consider the following strategies:
- Show All Work: Always show your steps, even if the answer seems obvious. The process is often as important as the result.
- Use Proper Notation: Correct and consistent use of mathematical symbols and terminology is essential for clarity and accuracy.
- Practice Writing Solutions: Before the exam, practice writing out your solutions in a clear, organized manner. This will help you develop the skill of communicating complex ideas concisely and effectively.
By focusing on these key factors, you can ensure that your written responses will significantly boost your score and help you achieve your desired results.