
In this section, you will delve into the essential mathematical techniques that form the foundation of problem-solving skills. The focus is on strengthening your ability to handle complex equations, manipulate variables, and understand relationships between different elements. The goal is to build confidence in approaching various types of problems and enhance your overall mathematical proficiency.
Throughout this guide, we will break down important steps, clarify common misconceptions, and provide clear explanations to help you solve equations with greater ease. Each concept is presented in a logical progression, ensuring that you can follow along and grasp even the more challenging topics. By mastering the techniques in this section, you will develop a deeper understanding of how mathematics functions and be well-prepared for more advanced studies.
Mathematical Solutions and Strategies for Section 4
This section provides essential solutions and detailed explanations to help students tackle the key problems found in this part of the curriculum. It is designed to support learners in navigating through challenging equations and concepts by offering clear, step-by-step guidance. The focus is on helping students strengthen their problem-solving skills while ensuring they grasp the underlying mathematical principles.
Each solution is carefully broken down into manageable steps, allowing for better understanding of the processes involved. The problems covered in this section range from basic calculations to more complex operations, providing a well-rounded approach to mastering the material. By following these solutions, students will gain a deeper insight into how mathematical concepts work together and will be better equipped to handle similar questions in future studies.
Understanding Key Concepts in Section 4
This section introduces fundamental ideas that serve as the building blocks for more complex mathematical operations. It covers critical topics that lay the groundwork for solving problems with variables, equations, and inequalities. Gaining a clear understanding of these concepts is essential for mastering more advanced topics and developing strong problem-solving abilities.
Breaking Down Equations and Expressions
In this part, you will explore how to manipulate and simplify mathematical expressions. Key techniques such as combining like terms, distributing, and factoring are discussed in detail. These methods are crucial for solving problems efficiently and accurately.
Solving Inequalities and Graphing Solutions
Another important focus is understanding how to solve inequalities and represent their solutions graphically. By learning to graph lines and identify solution sets, you will develop a stronger sense of how algebraic concepts apply to real-world scenarios.
Step-by-Step Solutions for Section 4 Problems
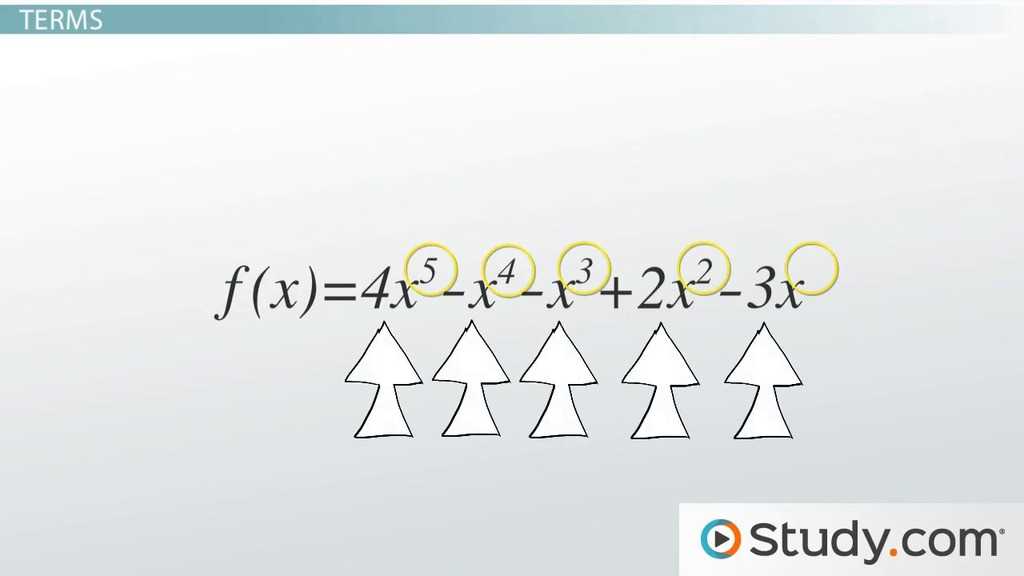
This section provides clear, detailed solutions to help you navigate through the problems encountered in this part of the curriculum. By breaking down each problem into manageable steps, you will gain a deeper understanding of the methods and reasoning needed to solve various types of mathematical challenges. These step-by-step solutions are designed to reinforce your knowledge and enhance your ability to tackle similar questions with confidence.
Each problem is carefully explained with a focus on key concepts, such as simplifying expressions, solving equations, and graphing results. The solutions aim to provide clarity on common problem types and show you how to approach them systematically. With practice, these techniques will become second nature, making it easier to solve more complex problems in the future.
Common Mistakes in Section 4
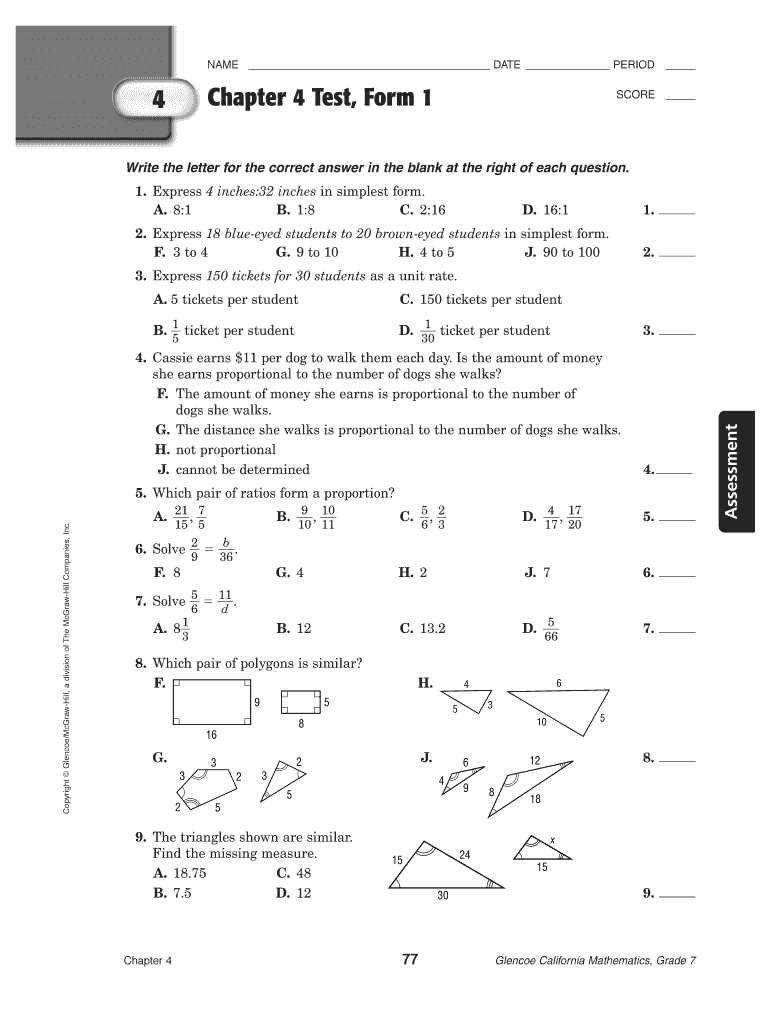
When working through problems in this section, students often make certain errors that can lead to confusion or incorrect solutions. Recognizing and avoiding these common pitfalls is crucial for developing a strong grasp of the material. Many of these mistakes stem from misunderstandings of key concepts or misapplication of techniques, but with careful attention and practice, they can be easily avoided.
Some typical errors include incorrectly simplifying expressions, making sign errors when solving equations, or failing to account for all possible solutions when dealing with inequalities. These mistakes can cause frustration, but by reviewing the steps carefully and double-checking your work, you can avoid them and improve your accuracy in solving problems.
How to Approach Word Problems
Word problems can often feel intimidating due to their narrative format, but with a systematic approach, they become much easier to solve. The key is to carefully read the problem, identify the information given, and determine what is being asked. Once you break the problem down into manageable parts, solving it becomes a logical process that involves applying mathematical principles step by step.
Start by underlining or highlighting the important details, such as numbers, variables, and relationships between quantities. Then, translate the words into mathematical expressions or equations. Pay attention to keywords like “sum,” “difference,” “product,” or “quotient” that indicate the operations you need to perform. Finally, solve the equation as you would any other, and make sure to interpret the solution in the context of the problem to ensure it makes sense.
Important Formulas in Section 4
In this section, several key formulas are essential for solving problems efficiently. Understanding how and when to apply these formulas is crucial for mastering the material. These formulas are not just theoretical; they are practical tools that will help you solve a wide range of equations and inequalities, making complex problems much simpler to handle.
Fundamental Equations and Their Uses
The following table outlines some of the most important formulas from this section, showing how each one can be used to solve specific types of problems. Familiarity with these formulas will enable you to approach equations with confidence and precision.
| Formula | Use |
|---|---|
| x = (-b ± √(b² – 4ac)) / 2a | Quadratic equation solution (quadratic formula) |
| y = mx + b | Slope-intercept form for linear equations |
| ax + by = c | Standard form for linear equations |
Application of Key Formulas
Each formula serves a specific purpose and is often applied in different contexts. For example, the quadratic formula is vital for solving second-degree equations, while the slope-intercept form is frequently used to graph straight lines and find their slopes. Mastery of these formulas is essential for solving the problems in this section and for progressing to more advanced topics.
Tips for Mastering Section 4
Mastering the concepts in this section requires a strategic approach to learning. The key to success is consistent practice, understanding the core ideas, and applying them to different types of problems. By following certain techniques and utilizing helpful strategies, you can build a strong foundation and enhance your problem-solving skills.
Effective Study Habits
To succeed in this section, focus on understanding each concept thoroughly before moving to the next. Try to break down complex problems into smaller, more manageable steps. Repetition is crucial, so make sure to solve a variety of problems to reinforce your understanding.
Key Strategies for Success
Here are some essential strategies that will help you excel in this section:
| Strategy | Benefit |
|---|---|
| Practice regularly | Helps reinforce concepts and improves problem-solving speed |
| Review mistakes | Identifies areas for improvement and prevents recurring errors |
| Work with examples | Provides insight into the application of concepts and formulas |
By using these strategies, you’ll build confidence and develop a deeper understanding of the material, making it easier to tackle more advanced topics in the future.
Solving Linear Equations in Section 4
Solving linear equations is a fundamental skill that serves as the foundation for more complex mathematical problems. In this section, we focus on understanding the methods and strategies needed to isolate variables and find their values. By mastering these techniques, you will be able to approach a wide range of mathematical problems with confidence and precision.
Steps for Solving Linear Equations
To solve a linear equation, follow these basic steps:
- Identify the variable and the constants in the equation.
- Move all terms involving the variable to one side of the equation and constants to the other side.
- Simplify both sides of the equation, combining like terms where applicable.
- Divide or multiply both sides of the equation by the necessary value to isolate the variable.
- Check the solution by substituting the value of the variable back into the original equation.
Example of Solving a Linear Equation
Consider the following equation:
2x + 5 = 15
To solve for x:
- Subtract 5 from both sides: 2x = 10
- Divide both sides by 2: x = 5
The solution to the equation is x = 5.
By practicing these steps regularly, you can become proficient in solving linear equations and apply these skills to more complex problems in the future.
Graphing Techniques for Section 4 Problems
Graphing is a powerful tool for visualizing mathematical relationships and solving equations. In this section, we will explore essential techniques for graphing linear equations and other key functions. By mastering these methods, you will be able to represent problems visually, making it easier to interpret solutions and identify patterns.
Key Steps in Graphing Linear Equations
To graph linear equations, follow these basic steps:
- Rewrite the equation in slope-intercept form y = mx + b, where m is the slope and b is the y-intercept.
- Plot the y-intercept on the graph (where the line crosses the y-axis).
- Use the slope to determine another point on the line. The slope m is the ratio of the rise (change in y) to the run (change in x).
- Draw a straight line through the two points, extending it in both directions.
Example of Graphing a Linear Equation
Consider the equation:
y = 2x + 3
For this equation:
- The y-intercept is 3, so plot the point (0, 3).
- The slope is 2, meaning for every 1 unit you move to the right on the x-axis, you move 2 units up on the y-axis. Plot another point at (1, 5).
- Draw the line through the points (0, 3) and (1, 5).
Graphing Table for Quick Reference
| Equation | Slope | Y-Intercept |
|---|---|---|
| y = 3x + 2 | 3 | 2 |
| y = -x – 1 | -1 | -1 |
| y = 1/2x + 4 | 1/2 | 4 |
By practicing these steps and using the table for reference, you will become more confident in graphing linear equations and solving related problems.
Practice Exercises for Section 4
Engaging with practice exercises is one of the best ways to reinforce the concepts learned in this section. These problems will help you sharpen your skills, test your understanding, and gain confidence in solving related equations. Whether you are tackling basic equations or more complex problems, consistent practice is key to mastering the material.
Basic Practice Problems
Start with simple exercises to ensure you understand the foundational principles:
- Solve for x: 3x + 5 = 14
- Solve for y: 4y – 7 = 21
- Solve for x: 2(x + 3) = 10
- Solve for y: 5y + 4 = 24
Advanced Practice Problems
Once you’ve mastered the basics, try solving more challenging problems:
- Solve for x: 5(x – 2) + 3 = 3x + 4
- Solve for y: 3(y + 2) – 4 = 2y + 10
- Solve for x: 2(x + 5) – 3 = 4x – 7
- Solve for y: 6(y – 3) + 2 = 5y + 8
By regularly working through these exercises, you will develop a deeper understanding of the material and improve your ability to solve various problems with ease.
Real-World Applications of Algebra 1
The concepts learned in this section extend far beyond the classroom and play a crucial role in many real-life situations. From personal finance to engineering, understanding how to manipulate variables and solve equations is essential in solving everyday problems. In this section, we explore several practical applications where the skills acquired can be applied to real-world scenarios.
Applications in Personal Finance
Mathematics is often used to manage budgets, savings, and investments. Here are some examples:
- Budgeting: Algebra can help create a budget by setting up equations to track income and expenses. For example, you can calculate how much money you have left after paying bills or how long it will take to save for a specific goal.
- Loan Repayments: When taking out a loan, the amount to be repaid can be determined by using equations involving interest rates, principal, and time.
- Investment Growth: Equations are used to determine how investments grow over time, based on different rates of return.
Applications in Engineering and Architecture
In fields like engineering and architecture, algebra is used to design structures, ensure stability, and calculate dimensions:
- Building Design: Architects use algebra to calculate the dimensions of buildings, ensuring that walls, windows, and doors fit within the planned space.
- Structural Integrity: Engineers use algebra to calculate forces, such as tension or compression, acting on materials to ensure they can withstand stress without failing.
- Project Costing: Construction projects require detailed calculations to estimate costs, materials needed, and timelines for completion.
Applications in Data Science and Technology
In data science, technology, and computing, algebra plays a critical role in analyzing data and designing algorithms:
- Data Analysis: Algebra is used to solve equations related to data trends, forecasting, and statistical analysis, helping professionals make decisions based on large datasets.
- Algorithm Development: Software developers use algebraic equations to design algorithms that power everything from search engines to social media platforms.
- Machine Learning: In the field of artificial intelligence, algebra is used to manipulate and analyze data, allowing machines to learn from experience and improve over time.
By understanding and applying these mathematical principles, you can see how vital the skills learned in this section are for solving a wide range of practical problems in our everyday lives.
How to Prepare for Chapter 4 Tests
Preparing for tests in mathematics requires a structured approach and solid understanding of the key concepts. It’s important to focus on mastering the skills that are likely to appear on the test, while also ensuring a thorough review of all the material covered. In this section, we will provide strategies and tips to help you succeed when it’s time for the exam.
Review Key Concepts and Formulas
Before tackling any practice problems, take the time to review the essential concepts and formulas. Here’s how to get started:
- Understand the Core Topics: Make sure you fully grasp the main ideas of the section, such as solving equations, graphing, and applying formulas.
- Memorize Important Formulas: Focus on key equations that are commonly used. Write them down and practice them until you can recall them quickly.
- Practice Word Problems: These often make up a significant portion of the test. Make sure you can break down the problems into smaller steps.
Practice with Sample Problems
The best way to solidify your knowledge is through practice. Try the following methods:
- Solve Practice Problems: Work through a variety of problems from the textbook or online resources. Make sure you tackle both simple and complex problems to build confidence.
- Timed Quizzes: Simulate test conditions by solving problems within a set time frame. This helps improve speed and efficiency.
- Check Your Work: After solving each problem, go back and review your solution to ensure that no mistakes were made. This will help identify weak areas.
Focus on Mistakes and Weak Areas
Don’t just focus on the problems you can solve easily; pay attention to the ones that challenge you. Here’s how to approach mistakes:
- Identify Patterns: If you’re consistently making the same mistakes, focus on understanding why and correct the process.
- Ask for Help: If you’re stuck on a particular concept, don’t hesitate to ask a teacher or classmate for assistance. Sometimes a different explanation can make things click.
- Use Online Resources: Take advantage of online tutorials, videos, or practice sites to reinforce your understanding of tricky concepts.
Stay Organized and Plan Ahead
Being well-prepared also involves staying organized and managing your study time effectively. Follow these steps:
- Make a Study Schedule: Break down your study sessions into manageable chunks and allocate specific times for reviewing each topic.
- Stay Consistent: Don’t cram at the last minute. Consistent daily practice is more effective than long, irregular study sessions.
- Get Plenty of Rest: Ensure you are well-rested before the test. A clear mind will help you think more critically and perform better.
By following these strategies, you will be well-prepared for the upcoming test and able to tackle the material with confidence.
Common Challenges in Algebra 1 Chapter 4
Many students face difficulties when working through mathematical concepts that involve solving equations, graphing, and applying various operations. These challenges often stem from misunderstandings of fundamental principles or mistakes made during problem-solving. In this section, we will explore the common issues encountered in this particular unit and offer strategies to overcome them.
Difficulty with Solving Equations

One of the most common challenges is the struggle to solve equations effectively. Many students find it difficult to manage variables and operations correctly. Here’s what typically causes confusion:
- Misunderstanding the Order of Operations: The rules for handling equations in the correct order (PEMDAS) can sometimes be overlooked, leading to errors.
- Combining Like Terms: Students often forget to combine like terms properly, resulting in incorrect expressions and solutions.
- Isolating Variables: Failing to correctly isolate the variable is a frequent mistake. It’s important to perform inverse operations in the correct sequence.
Challenges with Graphing Equations
Graphing can present its own set of challenges, especially when students are required to plot points or interpret graphs. Common issues include:
- Incorrectly Plotting Points: Sometimes students misplace points on the coordinate plane, leading to inaccurate graphs.
- Confusion with Slope and Intercept: Understanding how to identify the slope and y-intercept from an equation can be tricky for some learners.
- Graphing Linear vs. Non-linear Equations: Distinguishing between linear and non-linear equations is important for choosing the correct method of graphing.
Word Problem Difficulties
Word problems can be particularly challenging because they require translating real-life scenarios into mathematical expressions. Common obstacles include:
- Identifying Relevant Information: Many students struggle to identify the relevant data in the problem and how to use it to form an equation.
- Translating Words into Equations: The language used in word problems can be confusing, and converting it into the correct mathematical form can be a challenge.
- Setting up the Problem: Getting the initial setup wrong can make the entire solution process difficult, even if the mathematical steps themselves are correct.
Mistakes in Simplifying Expressions
Simplification of expressions is another area where students tend to make mistakes. These mistakes often stem from:
- Incorrect Distribution: Forgetting to distribute terms correctly in expressions that involve parentheses is a common error.
- Dividing by Zero: In some cases, students may not recognize when an expression leads to division by zero, which results in an undefined solution.
- Overlooking Negative Signs: Negative signs can easily be overlooked during simplification, leading to incorrect results.
By recognizing these challenges and understanding the reasons behind them, students can better prepare themselves for solving problems accurately. Addressing these areas of difficulty is essential for mastering the concepts and improving problem-solving skills.
Essential Vocabulary in Chapter 4
Understanding key terms is crucial for mastering any mathematical unit. In this section, we will discuss the most important vocabulary needed to navigate through the problems and concepts in this particular unit. These terms form the foundation for solving equations, graphing, and interpreting various mathematical expressions. Familiarity with them will help you approach each task with greater confidence and precision.
Key Terms Related to Equations
To work effectively with equations, it’s essential to grasp certain terminology. Here are some of the terms commonly encountered:
- Variable: A symbol, often a letter, used to represent an unknown value in an equation or expression.
- Coefficient: A number that multiplies a variable in an expression or equation.
- Constant: A fixed number that does not change in an equation or expression.
- Solution: The value or values that satisfy an equation.
- Equation: A mathematical statement that shows the equality of two expressions, typically involving variables and constants.
Key Terms Related to Graphing
Graphing is a visual way to represent equations and data. Understanding the following terms is essential when working with graphs:
- Coordinate Plane: A two-dimensional grid formed by two perpendicular number lines, typically labeled x and y.
- Plot: The act of marking a point on the coordinate plane based on its x and y values.
- Slope: The measure of how steep a line is, often calculated as the ratio of vertical change to horizontal change between two points on the line.
- Intercept: The point where a line crosses one of the axes on the coordinate plane (x-intercept or y-intercept).
- Linear Equation: An equation that represents a straight line when graphed, typically written in the form y = mx + b.
Mastering these essential terms will make it easier to approach problems and communicate mathematical ideas more clearly. Whether you are solving equations or interpreting graphs, understanding the language of mathematics is key to success in this unit.
How to Solve Inequalities in Chapter 4
Solving inequalities is an essential skill in mathematics that allows you to find ranges of possible solutions rather than just one specific value. In this section, we will cover the fundamental techniques for solving inequalities. These methods are similar to solving equations but require special attention when it comes to operations like multiplying or dividing by negative numbers.
Step 1: Understand the Symbols
The first step in solving inequalities is recognizing the inequality symbols and understanding their meaning:
- > means “greater than”
- < means “less than”
- ≥ means “greater than or equal to”
- ≤ means “less than or equal to”
- = means “equal to” (although this is typically used in equations, it may appear in some inequalities as well)
Step 2: Solve the Inequality
Solving an inequality is similar to solving an equation, with a few key differences. Follow these steps:
- Isolate the variable: Just like in an equation, move constants to the other side of the inequality sign by adding or subtracting them from both sides.
- Divide or multiply: If you need to divide or multiply both sides by a number, remember that if you multiply or divide by a negative number, you must reverse the inequality symbol.
- Check your solution: After solving, it’s important to test the solution to ensure that it satisfies the inequality. Often, you can do this by substituting values from the solution set back into the inequality.
Once you understand these key steps, solving inequalities will become a more straightforward process. The challenge lies in maintaining the correct inequality symbol, especially when multiplying or dividing by negative numbers.
Reviewing Key Topics Before the Exam
Preparing for an upcoming test requires a solid understanding of the main concepts covered in the material. By focusing on the key topics and practicing related problems, you can build confidence and ensure you’re ready for any question that might come your way. This section outlines important areas to review before taking the exam.
Focus on Core Concepts
Start by identifying the fundamental concepts that are essential for solving a variety of problems. These include:
- Solving equations: Be sure you can solve both simple and complex equations, including linear and quadratic forms.
- Graphing techniques: Review how to graph equations and inequalities, making sure you understand the interpretation of slopes and intercepts.
- Working with inequalities: Practice solving and graphing inequalities, paying attention to how different symbols affect the solution set.
- Operations with polynomials: Ensure you understand how to perform operations like addition, subtraction, multiplication, and factoring.
Effective Study Strategies

To maximize your study time and improve retention, try these strategies:
- Practice problems: Consistent practice with problems from each topic will help reinforce your understanding and identify areas of weakness.
- Review notes and examples: Go over class notes, textbooks, and any example problems provided. Pay special attention to any worked-out solutions.
- Take practice tests: Simulate test conditions by working through practice exams. This helps you get comfortable with the time constraints and the types of questions.
- Ask questions: If you’re unsure about any topic, don’t hesitate to ask a teacher or tutor for clarification before the test.
By reviewing these critical areas and using these strategies, you can ensure that you’re well-prepared for your exam. Understanding the key topics will not only help you perform better but also deepen your overall grasp of the subject. Stay focused, and your hard work will pay off.
Improving Your Problem-Solving Speed
Being able to solve mathematical problems efficiently is a crucial skill, especially when working under time constraints. To improve your problem-solving speed, it is essential to develop strategies that enhance both accuracy and efficiency. This section provides practical tips and techniques to help you solve problems faster while maintaining precision.
Strategies for Speeding Up Problem Solving
There are several approaches that can help you increase your problem-solving speed:
- Practice regularly: The more problems you solve, the quicker you become. Frequent practice builds familiarity with different types of problems and allows you to recognize patterns.
- Memorize key formulas: Knowing important formulas and rules off by heart will save you time during problem-solving. This reduces the need to refer back to notes or textbooks.
- Work in stages: Break problems into smaller, manageable steps. Focus on one part at a time, which will help you avoid feeling overwhelmed and maintain a steady pace.
- Eliminate unnecessary steps: Once you are familiar with the methods, practice simplifying your solutions. Often, there are multiple ways to approach a problem; try to find the most direct route.
Techniques for Managing Time Effectively
Along with problem-solving strategies, effective time management is key to improving your speed. Here are some techniques to help you manage your time during tests or practice sessions:
- Set time limits: Challenge yourself by setting a time limit for each problem. This can help you work faster and make decisions under pressure.
- Prioritize easier problems: Start with the problems you find easiest. This will build confidence and allow you to save more time for difficult questions.
- Eliminate distractions: When practicing, make sure you are focused solely on the problem at hand. Minimize distractions to prevent unnecessary delays.
- Use estimation: In some cases, an approximation can be just as useful as an exact answer. Learn to recognize when estimation will save you time without compromising accuracy.
By incorporating these strategies and techniques into your study routine, you will gradually improve your ability to solve problems quickly and efficiently. Remember, speed comes with practice, so continue working on strengthening your skills and applying these methods regularly.
Resources for Extra Practice in Algebra 1
Mastering mathematical concepts requires consistent practice, especially when tackling more complex topics. Fortunately, there are a variety of resources available to help you improve your skills and reinforce your understanding. These tools can provide additional exercises, explanations, and guidance to strengthen your problem-solving abilities.
Online Platforms and Websites
There are several websites that offer free practice problems and tutorials to help you improve your skills:
- Khan Academy: This platform provides instructional videos and practice exercises for a wide range of topics, including linear equations, inequalities, and graphing techniques.
- IXL: IXL offers personalized practice exercises and skill-building activities. It adapts to your level of understanding and helps you master specific areas.
- Wolfram Alpha: Known for its computational power, Wolfram Alpha can solve equations step-by-step, providing detailed explanations for each solution.
- Mathway: This tool can help you solve problems and show the process behind the solution. It is an excellent resource for checking your work and understanding each step.
Books and Printable Worksheets
Books and printable worksheets are also valuable resources for extra practice:
- Practice Books: Many publishers offer practice books with solutions that cover various math topics. These books often include detailed examples and answer keys.
- Workbooks: Workbooks can be found online or at local bookstores. They offer a large collection of problems and are organized by topic, allowing you to focus on areas where you need more help.
- Printable Worksheets: Websites like Math-Drills.com and Education.com provide free printable worksheets that cover a variety of topics. These worksheets are great for additional practice outside of your regular coursework.
Using a combination of online tools and traditional resources will give you the opportunity to reinforce your knowledge, clarify doubts, and practice solving problems on your own. The more you engage with different practice materials, the more confident you’ll become in your ability to tackle even the most challenging problems.