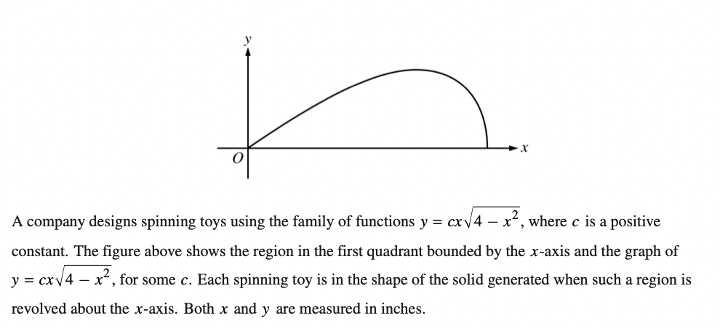
Successfully tackling advanced mathematics challenges requires not only understanding core concepts but also mastering the techniques used to solve complex problems. With a structured approach, it becomes easier to navigate through a series of intricate topics, from limits and derivatives to integrals and their real-world applications.
In this guide, we will explore the most common problem types found in higher-level mathematical evaluations. By practicing various problem formats and learning effective strategies for each, students can boost their confidence and readiness for any upcoming assessment. Time management and critical thinking are essential skills to refine, helping individuals excel under pressure while demonstrating their grasp of key mathematical ideas.
AP Calculus Exam Overview

The assessment for advanced mathematics in high school is designed to test a student’s ability to apply mathematical principles to a range of problems. It evaluates both theoretical knowledge and practical problem-solving skills. The format challenges individuals to think critically and analyze complex situations in various mathematical contexts.
Structure and Components
This assessment consists of two main parts: one focused on multiple-choice tasks, and the other on free-response scenarios. The multiple-choice section tests speed and accuracy in recognizing the correct method, while the free-response part evaluates a deeper understanding of concepts and the ability to work through problems methodically.
Scoring and Grading
Each section is scored separately, with a cumulative result determining the final grade. The grading scale is designed to measure proficiency in solving problems, not just answering questions. The goal is to assess the application of learned principles in real-world situations, ensuring that students are equipped to handle advanced mathematical challenges beyond the classroom.
What to Expect on the Exam
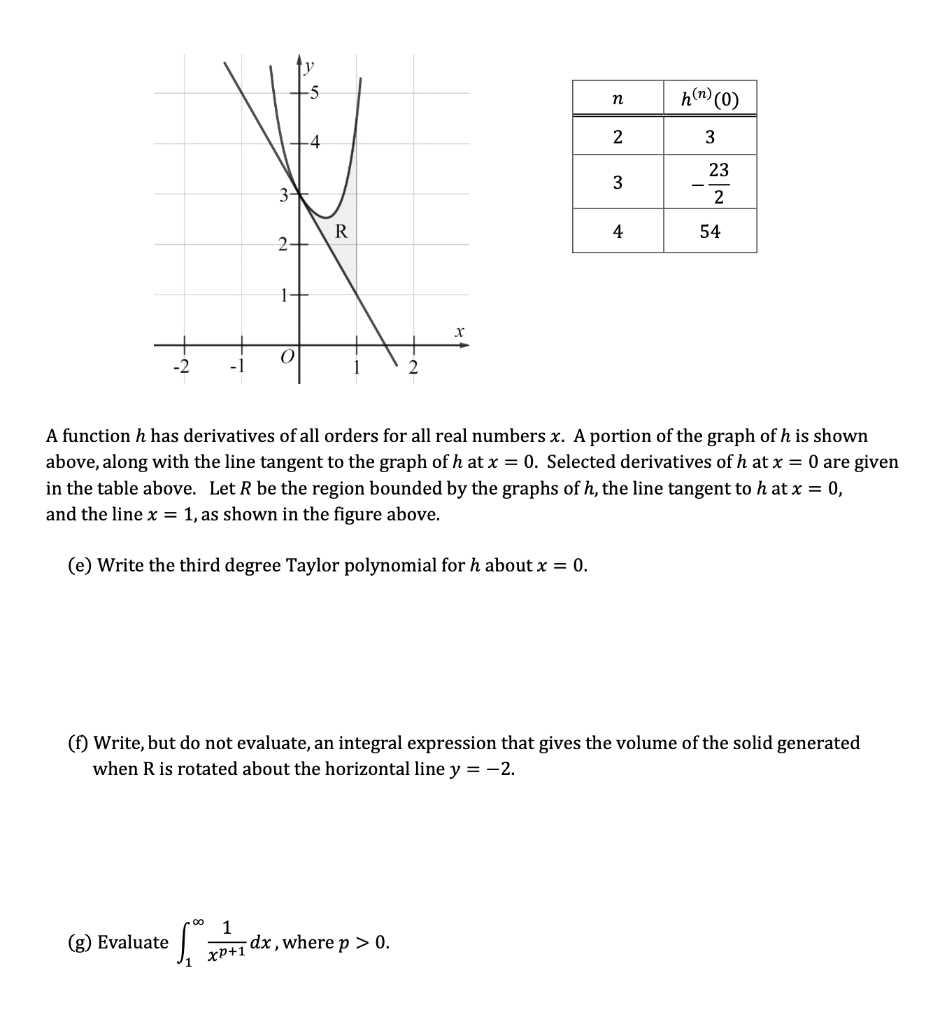
The assessment is structured to evaluate a student’s understanding of key mathematical concepts through a variety of problem types. You will encounter tasks that test both fundamental principles and the application of more advanced techniques. Being prepared for the format and timing is crucial to navigating through each section effectively.
Task Format
The assessment is divided into two distinct parts: a series of multiple-choice items designed to assess quick reasoning and selection of correct methods, followed by open-response scenarios that require detailed solutions. Each section will push your ability to not only recall formulas but also implement them accurately in different contexts.
Time Management
With limited time for each section, it’s important to pace yourself. Prioritize tasks that you find easier to complete first, leaving more challenging problems for later. Efficient time management can help alleviate pressure and ensure you complete all sections with attention to detail.
Key Topics in AP Calculus
Understanding the foundational concepts is essential for success in advanced mathematics. The material is diverse, covering everything from rates of change to the areas under curves. Each topic builds upon the previous one, and mastering these concepts provides the necessary tools for solving more complex problems effectively.
Core Concepts to Focus On
The following table outlines the primary areas to review. These topics are critical for solving the majority of problems that appear in the assessment, providing a solid foundation for understanding the broader subject.
| Topic | Description |
|---|---|
| Limits | Understanding the behavior of functions as they approach specific points or infinity. |
| Derivatives | Analyzing the rate of change of functions and solving for slopes of tangents. |
| Integrals | Calculating areas under curves and solving problems involving accumulation. |
| Series | Exploring the behavior of infinite sums and understanding convergence. |
Additional Concepts to Explore
In addition to the key topics listed, there are advanced areas that may appear in more complex scenarios. Gaining proficiency in these areas allows for a deeper understanding and improves problem-solving skills in less straightforward tasks.
Multiple Choice Question Strategies
Mastering multiple-choice tasks requires a strategic approach that maximizes efficiency and accuracy. These types of tasks often test a student’s ability to identify correct methods quickly, requiring both knowledge of concepts and the ability to make well-informed choices under time pressure. A thoughtful strategy can make a significant difference in how well you perform.
Approach Each Task Systematically
Before selecting an option, carefully read the problem to understand what is being asked. Eliminate clearly incorrect choices first. This reduces the options and increases the probability of selecting the correct answer. Afterward, review the remaining choices with attention to detail to ensure the answer you pick is the best possible solution.
Time Management Tips
Multiple-choice tasks are time-sensitive, so it is important to pace yourself. Don’t get stuck on one difficult task for too long. If you’re unsure about an answer, make your best guess and move on, returning to tougher problems if time allows. This approach ensures you don’t waste time on questions that might take too long to solve.
How to Tackle Free Response Questions
Approaching open-ended tasks requires a clear strategy and methodical thinking. These types of tasks often demand more detailed reasoning and the ability to showcase your understanding of mathematical concepts step by step. The key to handling them successfully is breaking down each part of the problem and working through it logically.
Step-by-Step Problem Solving
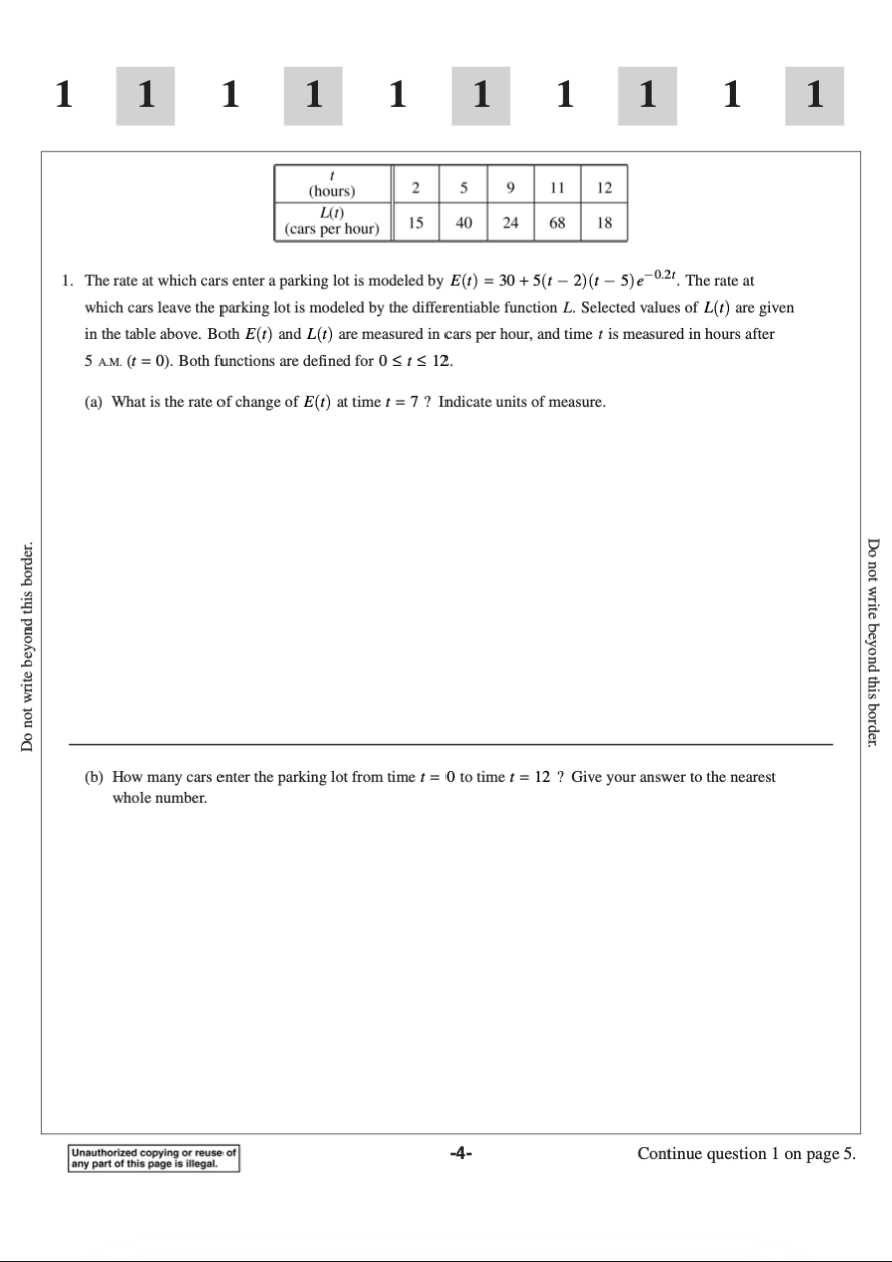
Start by reading the task carefully to understand the problem fully. Identify what information is given and what needs to be found. Organize your solution by tackling each part in sequence. Present your work clearly and show all steps to ensure that your reasoning is easy to follow. This will not only help you stay organized but also maximize your chances of receiving full credit for your solution.
Check Your Work
Once you’ve completed a response, it’s important to review your solution. Double-check your calculations and ensure that all steps are logically consistent. If time permits, re-read the problem to make sure your final answer directly addresses the original prompt. Small errors can lead to incorrect results, so a final check is essential for achieving the best possible outcome.
Understanding Limits and Continuity
The concepts of limits and continuity are foundational to understanding how functions behave. Limits describe how a function approaches a particular value as its input nears a specified point. Continuity, on the other hand, ensures that a function behaves predictably without abrupt jumps or breaks. Together, these principles are essential for analyzing more complex behaviors in mathematics.
Key Concepts to Master
To fully grasp these ideas, it’s important to understand the specific conditions under which limits exist and when functions are considered continuous. Below is a table outlining the key concepts and their characteristics.
| Concept | Description |
|---|---|
| Limits | Describes the value a function approaches as the input gets closer to a certain point, from either the left or right. |
| Continuity | A function is continuous if its graph has no breaks, jumps, or holes at the given point. |
| Left-Hand Limit | The value a function approaches as the input approaches a point from the left side. |
| Right-Hand Limit | The value a function approaches as the input approaches a point from the right side. |
Applications in Problem Solving
Understanding how limits and continuity work together allows for a deeper analysis of functions. These concepts are used to determine the behavior of complex equations, especially when investigating asymptotes, discontinuities, or points of change. With practice, these principles become invaluable tools in solving more intricate problems in higher-level mathematics.
Mastering Derivatives and Their Applications
Understanding how to find rates of change and applying this knowledge to real-world situations is essential in advanced mathematics. Derivatives provide a powerful tool for analyzing functions, helping to determine how quantities change with respect to each other. By mastering the basic rules and methods for calculating derivatives, you can solve a wide range of problems and apply them to various practical scenarios.
Key Derivative Concepts
Here are the essential topics you need to focus on when mastering derivatives:
- Power Rule: A straightforward method for differentiating polynomials.
- Product and Quotient Rules: Techniques for finding derivatives of products and ratios of functions.
- Chain Rule: A method for differentiating composite functions.
- Implicit Differentiation: Used when functions are not explicitly solved for one variable.
Applications in Problem Solving
Derivatives are not just useful for solving abstract problems; they have many real-world applications, such as:
- Optimization: Finding maximum or minimum values in functions to solve practical problems such as cost reduction or profit maximization.
- Related Rates: Solving problems where two or more variables change with respect to time, like calculating the speed of a moving object.
- Motion Analysis: Derivatives are used to find velocity, acceleration, and other key aspects of an object’s motion in physics.
- Curve Sketching: Analyzing the behavior of functions by finding critical points, concavity, and inflection points.
By practicing these techniques, you will gain the skills needed to apply derivatives effectively in both theoretical and real-world situations. Understanding these principles thoroughly will greatly enhance your problem-solving abilities in advanced mathematics.
Integral Calculus: Techniques and Examples
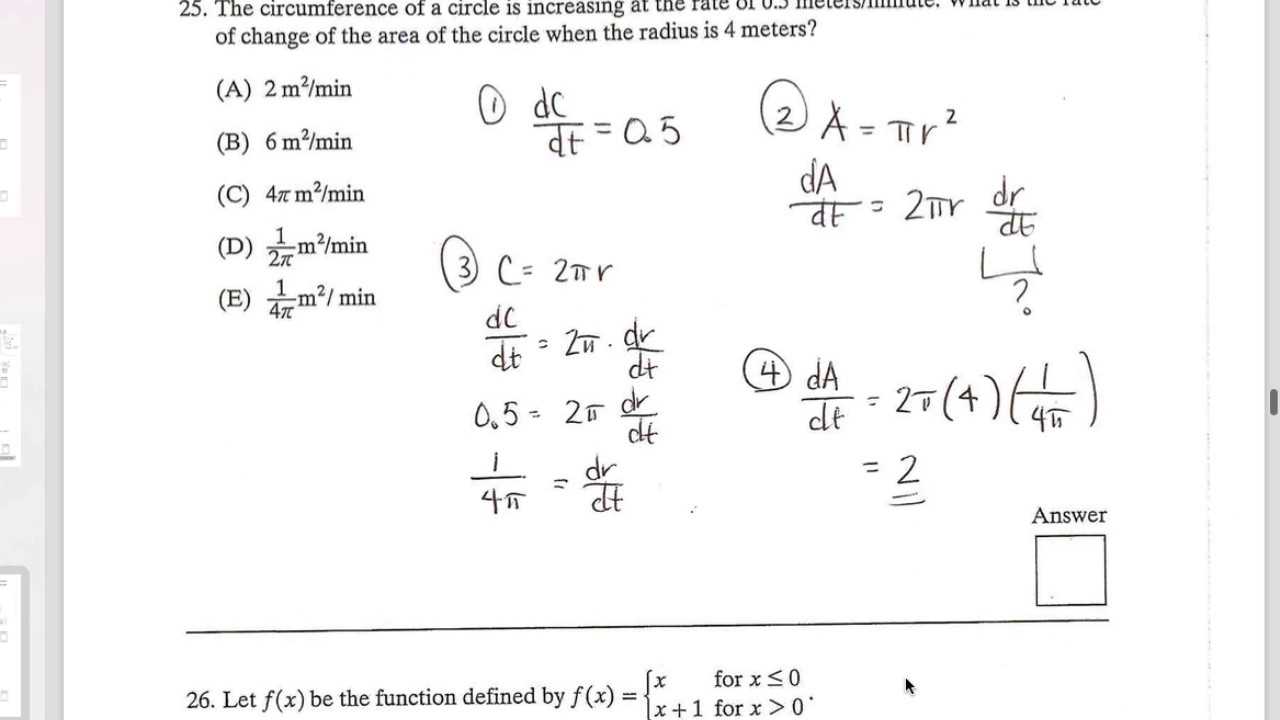
Integral mathematics is a key area in advanced problem solving, focusing on determining quantities such as areas under curves and accumulation over intervals. Mastery of these techniques allows you to tackle a variety of problems, from finding the area of irregular shapes to analyzing the total accumulation of a quantity over time. Understanding the foundational methods for computing integrals is essential for applying them effectively in both theoretical and practical contexts.
Key Techniques in Integration
There are several important techniques that help simplify the process of solving integrals. Each method applies to specific types of functions and situations:
- Substitution: A method used when an integral contains a composition of functions, simplifying the problem by changing variables.
- Integration by Parts: This technique is useful when dealing with products of functions, based on the product rule for differentiation.
- Partial Fractions: Applied to rational functions, breaking them into simpler terms for easier integration.
- Trigonometric Integrals: A method for integrating functions involving trigonometric expressions, simplifying them for calculation.
Examples of Applications
Integrals are used in various fields to solve real-world problems. Here are a few examples:
- Area Under a Curve: By integrating a function, you can find the area between the curve and the x-axis over a given interval.
- Volume of Solids: Using integration, you can compute the volume of 3D objects generated by rotating a curve around an axis.
- Accumulated Quantities: Integrals help calculate the total amount of a changing quantity over a specific time period, such as distance traveled or total accumulated profit.
By understanding these techniques and practicing their application, you can solve a broad range of problems efficiently and accurately.
AP Calculus Practice Problems
Practicing with problems is essential to solidifying your understanding of advanced mathematical concepts. By working through various types of exercises, you can sharpen your problem-solving skills, identify areas for improvement, and build confidence. These exercises can range from basic calculations to more complex real-world applications, helping to reinforce the core principles required for success.
Common Types of Problems
There are several common types of problems that appear in advanced mathematics, and each requires a different approach. Familiarizing yourself with these will allow you to handle any challenge effectively:
- Rate of Change: These problems focus on determining how a quantity changes over time or with respect to another variable.
- Area Calculations: Find the area under curves, between curves, or between a curve and the axis, using integration techniques.
- Optimization: Problems that involve finding the maximum or minimum values of a function within a given interval.
- Function Behavior: Analyzing how a function behaves by examining its derivatives, critical points, and concavity.
Example Practice Problems
Here are some practice problems that can help test your understanding of key concepts:
- Problem 1: Find the rate of change of the function at a specific point.
- Problem 2: Determine the area between the curve and the x-axis over a given interval.
- Problem 3: Solve an optimization problem to find the minimum or maximum value of a function.
- Problem 4: Analyze the behavior of a function by identifying its critical points and inflection points.
By regularly practicing problems like these, you will develop the skills necessary to approach any challenge with ease and precision.
Time Management During the Exam
Effective time management is crucial for maximizing performance in any challenging assessment. When facing a series of problems, it’s important to allocate your time wisely, ensuring that you can complete all sections while maintaining accuracy. Planning ahead allows you to approach each task with a clear strategy, reducing stress and helping you avoid rushing through difficult parts.
Here are some strategies to help manage your time effectively:
| Strategy | Description |
|---|---|
| Prioritize Easier Tasks | Start with the questions you find most straightforward to build confidence and gain momentum. |
| Set Time Limits | Allocate a specific amount of time to each section and stick to it, avoiding spending too long on any one part. |
| Skip and Return | If a question is too challenging, move on and come back to it later after completing easier ones. |
| Review Your Work | Reserve some time at the end to go over your answers and correct any errors. |
By following these strategies, you can ensure that you approach the assessment with a clear mind, completing each section on time and maximizing your chances of success.
Common Mistakes to Avoid
While tackling challenging mathematical problems, it’s easy to make mistakes that can affect the accuracy of your solutions. Recognizing and understanding these common errors can help you avoid them and approach each problem more carefully. Being mindful of these pitfalls ensures that your answers reflect a strong grasp of the material, rather than oversights or missteps.
Common Calculation Errors
One of the most frequent sources of mistakes is simple calculation errors. These can easily happen when you’re under pressure or rushing. Here are a few to watch out for:
- Sign Mistakes: Be careful with negative signs, especially when performing operations like subtraction or distribution.
- Arithmetic Missteps: Double-check simple operations, such as multiplication and division, as they often lead to errors in more complex problems.
- Skipping Steps: Rushing through a problem and skipping intermediate steps can lead to mistakes. Always show your work clearly.
Conceptual Mistakes
In addition to calculation errors, misunderstandings of key concepts can result in incorrect solutions. Here are a few common conceptual pitfalls:
- Incorrect Use of Formulas: Applying the wrong formula or misinterpreting the given conditions can lead to inaccurate answers.
- Misunderstanding Function Behavior: Errors can occur when misinterpreting the behavior of a function, such as its domain, range, or asymptotic behavior.
- Failure to Analyze Limits: Forgetting to analyze limits properly or incorrectly applying limit laws can affect the correctness of your solution.
By staying alert to these common errors, you can enhance your accuracy and avoid unnecessary mistakes that could impact your results.
Effective Study Tips for AP Calculus
Studying for advanced mathematical challenges requires a focused and strategic approach. The right study techniques can make a significant difference in understanding complex concepts and performing well. By organizing your time, using the right resources, and practicing regularly, you can ensure that you’re well-prepared for the test ahead.
Key Study Strategies
Here are some tried-and-true methods to help you prepare effectively:
- Break Down the Material: Don’t try to learn everything at once. Break the content into manageable sections and tackle one topic at a time.
- Focus on Problem Solving: Practice solving a variety of problems to reinforce concepts and identify areas of weakness.
- Create a Study Schedule: Plan your study time well in advance. Consistent, short study sessions are more effective than cramming at the last minute.
- Utilize Practice Resources: Use practice problems and mock tests to familiarize yourself with the format and types of challenges you will encounter.
Tips for Retaining Information
Retention is key to mastering complex mathematical concepts. These tips can help you retain what you’ve learned:
- Teach What You Learn: Teaching someone else the material is one of the best ways to reinforce your own understanding.
- Summarize Key Points: After studying a topic, write down a brief summary of key concepts to help reinforce the material.
- Use Visual Aids: Diagrams, graphs, and charts can be extremely helpful for visualizing concepts and understanding their relationships.
- Stay Consistent: Regular review of previously studied material will help keep it fresh in your mind.
By adopting these study strategies, you’ll increase your ability to tackle challenges with confidence and clarity, improving your performance over time.
Resources for AP Calculus Preparation
Preparing for a challenging mathematical assessment can be made easier with the right resources. A variety of materials are available to help you strengthen your understanding and enhance your skills. From textbooks and online platforms to interactive tools, using a range of study aids ensures you have the support you need to excel.
Books and Textbooks
Having the right textbooks can make a significant difference in your preparation. These resources provide structured content and practice problems:
- Review Books: Comprehensive guides that cover all key topics with practice tests and explanations. They are an excellent tool for self-study.
- Textbooks: Standard textbooks used in high school and college courses, offering in-depth explanations and worked-out examples.
- Solution Manuals: These manuals provide solutions to problems from textbooks, offering detailed steps to help you understand the process.
Online Platforms and Tools
Several online platforms offer interactive lessons, quizzes, and forums to help you prepare more effectively. Here are some of the best options:
- Interactive Websites: Websites like Khan Academy and Coursera offer free courses and tutorials, walking you through complex concepts with video lessons and quizzes.
- Practice Apps: Mobile apps like Wolfram Alpha can assist with solving problems step by step, helping you verify solutions and explore different approaches.
- Online Study Groups: Join online communities or forums where students collaborate, discuss problems, and share tips for preparation.
Other Helpful Resources
In addition to textbooks and online tools, other materials can be helpful:
- Flashcards: Use flashcards to memorize formulas, definitions, and important concepts, reinforcing your knowledge.
- YouTube Channels: Educational channels provide video tutorials on specific topics, offering different perspectives and explanations.
- Tutoring Services: If you’re struggling with specific areas, consider hiring a tutor for personalized instruction and guidance.
By utilizing a combination of these resources, you will be well-equipped to tackle the material with confidence and improve your performance. Whether you prefer structured study or interactive learning, these resources offer valuable support throughout your preparation journey.
Using Graphing Calculators in the Exam
Graphing calculators are powerful tools that can assist in solving complex problems, providing a visual representation of mathematical concepts. When allowed during a test, these devices can help streamline calculations and improve accuracy. However, it’s essential to understand their proper usage to avoid relying on them too heavily and to maximize their potential during your assessment.
Benefits of Using a Graphing Calculator

Graphing calculators offer several advantages that can make solving problems more efficient and effective:
- Visual Representation: These devices allow you to plot functions and equations, helping you visualize relationships between variables and identify key points like maxima, minima, and intercepts.
- Speed and Accuracy: With built-in algorithms, graphing calculators can quickly compute complicated operations, reducing the risk of arithmetic errors.
- Complex Problem-Solving: Graphing calculators can handle intricate integrals, derivatives, and other advanced operations that may be time-consuming to perform by hand.
Strategies for Effective Use
While graphing calculators can significantly aid in solving problems, it’s crucial to use them strategically:
- Familiarize Yourself with Functions: Ensure you know how to input various types of problems, such as plotting functions, solving equations, or calculating derivatives, on your specific calculator model.
- Use Graphs to Understand Problems: Visualizing equations and data sets through graphs can help clarify the problem, making it easier to spot trends or identify errors.
- Double-Check Results: While graphing calculators are powerful, always verify your results with manual calculations when possible. This ensures you truly understand the problem and aren’t just relying on the device.
Common Pitfalls to Avoid
Even though graphing calculators are helpful, over-reliance or improper use can lead to mistakes:
- Incorrect Settings: Ensure that your calculator is set to the correct mode (e.g., radians vs. degrees) to avoid miscalculations.
- Overuse of Technology: Don’t become dependent on the graphing calculator for every calculation; use it as a tool to assist your understanding, not as a crutch.
- Ignoring Key Steps: Relying on the calculator without understanding the underlying math may result in missing essential problem-solving steps.
Graphing calculators can significantly enhance your performance during assessments when used properly. By mastering the features of your device and employing them strategically, you can boost efficiency and accuracy, helping you approach even the most challenging problems with confidence.
How to Approach Word Problems
Word problems can often seem daunting, especially when they involve multiple steps or unfamiliar contexts. However, breaking down the problem systematically and focusing on understanding the core concepts can simplify the process. The key to solving these types of problems is clear, logical thinking and a structured approach.
Step-by-Step Strategy for Solving Word Problems
To approach word problems effectively, follow these steps:
- Read Carefully: Begin by reading the problem multiple times to ensure that you understand what is being asked. Pay close attention to the details, as they often contain important clues.
- Identify Key Information: Highlight or underline the key facts, figures, and relationships presented in the problem. This will help you focus on what is relevant.
- Translate into Mathematical Terms: Once you have identified the important information, convert the problem into a mathematical format. This might involve creating equations or diagrams based on the given data.
- Set Up the Problem: Write out the relevant formulas or equations and define any variables you need to solve for. Organize the information logically to make the problem easier to tackle.
- Solve Step by Step: Work through the problem methodically, solving one part at a time. If you get stuck, go back to your setup and see if there is a different way to approach it.
- Check Your Work: Once you’ve arrived at an answer, review your solution. Check that it makes sense in the context of the problem and verify your calculations.
Tips for Success
Here are some additional strategies that can help you solve word problems more effectively:
- Draw Diagrams: Visualizing the problem can help you understand the relationships between different elements. A diagram or graph can make complex scenarios much easier to interpret.
- Use Logical Reasoning: Think critically about the information presented. Sometimes, making logical assumptions or approximations can simplify the problem-solving process.
- Practice Regularly: The more you practice word problems, the more confident you will become in recognizing patterns and applying the correct techniques.
- Stay Organized: Keeping your work neat and clearly organized will help you avoid mistakes and make it easier to follow your thought process when checking your solution.
By following these strategies, you can approach word problems with confidence and efficiency, ensuring that you understand each step of the process and arrive at accurate solutions.
How Grading Works on the AP Exam
The grading system for advanced placement assessments is designed to reflect a student’s overall performance across a range of tasks. The scoring process takes into account both the accuracy and depth of responses, as well as the approach to problem-solving. Understanding how scores are determined can help students focus their preparation on the areas that matter most.
Grading Components
The grading for these assessments is divided into two main components: multiple-choice questions and free-response sections. Each part contributes a specific percentage to the final score, with each requiring different strategies and approaches to ensure success. Below is a breakdown of the typical grading distribution:
| Section | Weight |
|---|---|
| Multiple-Choice | 50% |
| Free-Response | 50% |
Scoring Scale
Each section is graded independently, and the final score is determined by combining both parts. The overall score is typically reported on a scale from 1 to 5, with each score indicating a level of proficiency:
- 5: Extremely well qualified
- 4: Well qualified
- 3: Qualified
- 2: Possibly qualified
- 1: No recommendation
The process of assigning scores involves detailed evaluation by trained graders, ensuring consistency and fairness. Although multiple-choice questions are graded automatically, free-response questions are carefully reviewed to evaluate reasoning and explanation along with the final solution.
Understanding the grading system can help students tailor their study strategies to maximize their performance, ensuring a well-rounded approach to both sections of the assessment.
What to Do After the Exam
Once you’ve completed the assessment, it’s important to focus on managing your next steps. The period after such an evaluation can be a mix of relief and uncertainty. Instead of worrying about what you may or may not have done, it’s best to adopt a proactive mindset and prepare for the next stages in your academic journey.
Stay Calm and Reflect
First and foremost, give yourself some time to unwind and relax. It’s natural to feel anxious about the results, but stressing over the outcome won’t change anything. Reflect on the preparation process and consider what worked well and what could have been improved. This self-reflection can be a valuable tool for future assessments, allowing you to identify strengths and areas for improvement.
Understand the Grading Timeline
After completing the task, understanding the timeline for when the results will be available can help manage expectations. Most institutions have specific periods during which they release scores. Use this time to continue with your studies or focus on other important commitments. By staying productive, you prevent unnecessary stress and keep your academic routine on track.
In the meantime, you can:
- Engage in relaxing activities, like hobbies or spending time with friends and family.
- Focus on upcoming assignments or exams in other subjects.
- Start planning for next steps in your education, such as college applications or additional learning opportunities.
Remember, the period after the assessment is just as important as the preparation itself. Take time to recharge, but also stay focused on your goals moving forward.