Mathematics can be challenging, but mastering essential principles is key to excelling in more complex topics. Whether you’re preparing for an upcoming test or simply looking to improve your skills, it’s crucial to focus on understanding core ideas and their practical applications. Through consistent practice and strategic problem-solving, anyone can strengthen their grasp on these fundamental concepts.
Problem-solving techniques are vital in approaching a variety of questions. It’s not just about memorizing formulas, but also about recognizing patterns and applying logic. With the right approach, even the most difficult problems can become manageable.
Preparation is crucial to success. The more you familiarize yourself with different types of problems and their solutions, the more confident you’ll become. This process helps build a solid foundation, enabling you to tackle even more advanced mathematical challenges with ease.
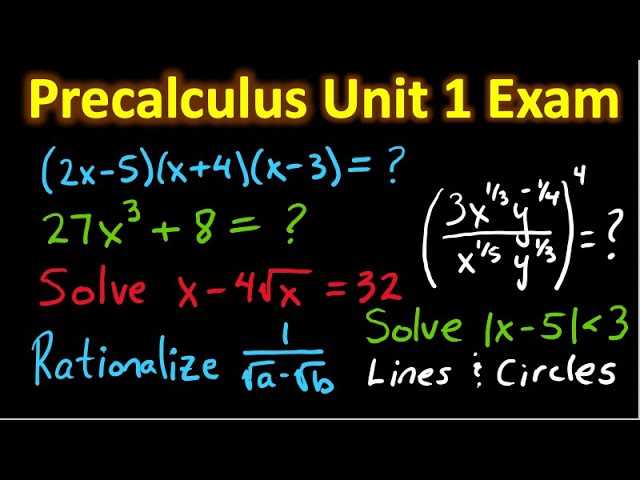
Precalculus Exam with Answers
To succeed in mastering higher-level mathematics, it’s essential to practice solving problems that challenge your understanding of various concepts. A key component of learning is not just theory, but the ability to apply knowledge to real-world scenarios. This section provides a set of practice questions, each designed to help you reinforce your understanding of important mathematical principles.
Key Problem-Solving Strategies
Each problem tests your ability to manipulate mathematical expressions and think critically about the relationships between different variables. Focus on identifying patterns and connections within each question. Take time to break down complex problems into smaller, more manageable parts. Developing this analytical approach will boost your confidence and precision.
Building a Solid Foundation
Achieving proficiency in mathematics is not about memorizing answers but about understanding why and how certain methods work. As you work through practice problems, pay attention to common patterns in solutions. This helps to internalize essential methods, making them easier to recall under test conditions. A strong foundation in these basic principles will serve you well in future mathematical endeavors.
Understanding Key Concepts in Precalculus
Grasping the fundamental ideas of advanced mathematics is crucial for solving complex problems. These core concepts lay the foundation for more intricate topics and provide the tools necessary for analyzing relationships between variables. Understanding these building blocks allows you to approach difficult challenges with confidence and clarity.
Key concepts include a range of mathematical areas, each contributing to your overall proficiency. Here are some essential topics to focus on:
- Functions: Understanding the different types of functions and their behaviors is essential. This includes linear, quadratic, and trigonometric functions, each with its own unique properties.
- Graphing: Visualizing equations and interpreting graphs is an important skill. Being able to recognize patterns and shifts in the graph allows for deeper insights into the function’s behavior.
- Polynomials: These expressions often appear in many areas of mathematics. Mastering their operations, such as addition, subtraction, and factoring, is fundamental.
- Rational Expressions: Handling fractions that involve variables requires knowledge of simplification, factoring, and solving equations involving these expressions.
- Trigonometry: Understanding the relationships between angles and sides in triangles is key. Trigonometric identities and functions like sine, cosine, and tangent play a significant role in problem-solving.
Each of these topics is interconnected, building on previous knowledge. A strong understanding of these principles makes it easier to approach more advanced topics and solve increasingly complex mathematical challenges.
Common Types of Precalculus Questions
Mathematical problem sets often focus on a variety of topics, each testing a different aspect of your understanding. These questions are designed to evaluate how well you can apply key concepts to solve specific problems. Understanding the types of questions commonly asked can help you prepare more effectively and focus on the areas that need the most attention.
Types of Questions
Questions can cover a broad range of topics, from simple calculations to complex functions and equations. Here are some of the most common types:
| Question Type | Description |
|---|---|
| Function Evaluations | These involve substituting values into given functions to find the output. They test your ability to work with different types of functions like linear, quadratic, or trigonometric. |
| Graph Interpretation | These questions ask you to analyze graphs and identify key features such as intercepts, slopes, and asymptotes. They test your understanding of how equations relate to visual representations. |
| Solving Equations | Questions in this category require solving equations, which may involve algebraic manipulations, factoring, or applying the quadratic formula. |
| Identities and Formulas | These problems often ask you to simplify or prove trigonometric identities, or to apply known formulas to solve problems involving polynomials or rational expressions. |
| Word Problems | These test your ability to translate real-world situations into mathematical expressions. They require a deeper understanding of the practical application of functions and equations. |
Common Techniques for Solving
To solve these types of problems effectively, it’s important to follow structured methods, such as:
- Substitute known values correctly and check for extraneous solutions.
- Use graphing tools to visualize problems when necessary.
- Apply algebraic properties and identities to simplify complex equations.
- Carefully read word problems to ensure the correct mathematical model is used.
By familiarizing yourself with the most common problem types, you’ll be better prepared to tackle any challenge that comes your way.
How to Solve Polynomial Equations
Solving equations that involve polynomials requires a systematic approach. These equations can have multiple solutions and involve various operations, such as factoring, applying the quadratic formula, or using synthetic division. Understanding the structure of the equation is key to choosing the right method for solving it.
Here are several strategies you can use when solving polynomial equations:
- Factoring: One of the most common methods is factoring the polynomial expression. Look for common factors or try to apply special factoring formulas, such as difference of squares or trinomials.
- Setting the equation to zero: Before solving, always set the equation equal to zero. This allows you to solve for the variable by factoring or using other methods.
- Using the quadratic formula: If the polynomial can be simplified to a quadratic form, apply the quadratic formula to find the roots. This is especially helpful when the polynomial has no easily factorable terms.
- Synthetic division: This method is used for dividing polynomials by linear factors. It simplifies the process and is particularly useful for larger polynomials.
- Graphing: Sometimes, plotting the equation on a graph can help visualize the solutions. The points where the graph crosses the x-axis are the solutions to the equation.
By mastering these techniques and practicing with different polynomial equations, you will become more efficient at solving them and understanding the underlying principles behind each method.
Trigonometric Functions and Their Applications
Trigonometric functions are a cornerstone of advanced mathematics, used to model and solve problems involving angles, distances, and periodic behaviors. These functions help describe relationships in both theoretical and real-world contexts, from simple triangles to complex waveforms. Mastering these functions is essential for solving many practical problems in fields such as physics, engineering, and even economics.
Key Trigonometric Functions
The primary trigonometric functions are based on the ratios of the sides of a right triangle. The most common ones are:
- Sine (sin): Represents the ratio of the opposite side to the hypotenuse.
- Cosine (cos): Represents the ratio of the adjacent side to the hypotenuse.
- Tangent (tan): Represents the ratio of the opposite side to the adjacent side.
- Cosecant (csc): The reciprocal of sine.
- Secant (sec): The reciprocal of cosine.
- Cotangent (cot): The reciprocal of tangent.
Applications of Trigonometry
Trigonometric functions have a wide range of applications across various fields. Some of the most notable uses include:
- Wave motion: Trigonometric functions are used to model periodic phenomena, such as sound waves, light waves, and tides.
- Angles of elevation and depression: These functions help calculate distances and heights in real-world scenarios, such as measuring the height of buildings or mountains.
- Circular motion: Trigonometry is used to describe the motion of objects moving in circular paths, such as the rotation of wheels or planetary orbits.
- Physics and engineering: Trigonometric functions are used extensively to solve problems related to forces, vectors, and oscillations.
By understanding the behavior and applications of trigonometric functions, you can gain valuable insights into both theoretical concepts and practical situations that arise in everyday life.
Mastering Graphs of Functions
Graphing functions is an essential skill in mathematics, enabling you to visually represent relationships between variables. Understanding how different functions behave and how their graphs are shaped allows you to solve complex problems more efficiently. By recognizing patterns and key features in graphs, you can gain valuable insights into the function’s properties and how it reacts to changes in input values.
Key Concepts in Graphing
Before you begin graphing, it’s important to understand several key concepts that will guide you in visualizing functions correctly:
- Intercepts: The points where a graph crosses the axes. The x-intercept occurs when y = 0, and the y-intercept occurs when x = 0.
- Domain and Range: The domain refers to the set of possible input values (x-values), while the range refers to the set of possible output values (y-values).
- Asymptotes: Lines that the graph approaches but never touches. These are often seen in rational functions or exponential functions.
- Symmetry: Some functions exhibit symmetry, such as even or odd symmetry, which can help simplify the graphing process.
Steps for Graphing Functions
To graph a function accurately, follow these steps:
- Identify key features: Determine the intercepts, domain, and range. If applicable, note any asymptotes or symmetries.
- Choose values: Select a range of x-values, both positive and negative, to get a broad view of the function’s behavior.
- Plot points: Calculate the corresponding y-values for the chosen x-values and plot them on the coordinate plane.
- Connect the points: Use smooth curves or straight lines to connect the points, paying attention to the overall shape and direction of the graph.
- Check for accuracy: Review the graph for consistency with the function’s known characteristics and adjust as needed.
By practicing these steps, you’ll improve your ability to graph various types of functions, from simple linear equations to more complex polynomials and trigonometric functions. This skill is crucial for solving mathematical problems and understanding the behavior of different functions in both theoretical and practical contexts.
Practice Problems for Algebra Skills
Strengthening algebraic skills is essential for solving a wide variety of mathematical problems. Regular practice helps develop a deeper understanding of key concepts such as manipulating equations, factoring expressions, and working with inequalities. By tackling diverse problems, you can reinforce your ability to apply algebraic techniques effectively in different scenarios.
Here are some practice problems that cover a range of important algebra topics:
Solving Linear Equations
These problems focus on solving simple linear equations with one variable:
- 3x + 5 = 17
- 2(x – 4) = 10
- 5(x + 3) = 2x – 1
- 4x – 7 = 3x + 8
Factoring Expressions
Next, practice factoring polynomials and quadratic expressions:
- x² + 5x + 6
- 3x² – 7x + 4
- 6x² – 5x – 6
- 4x² – 12x + 9
Solving Quadratic Equations
These problems will help you practice solving quadratic equations using factoring, completing the square, or applying the quadratic formula:
- x² – 5x + 6 = 0
- x² + 4x – 12 = 0
- 2x² – 3x – 5 = 0
- x² + 2x – 15 = 0
Working with Inequalities
Next, solve and graph simple inequalities:
- 2x – 3
- 3(x + 2) > 12
- 4x + 7 ≤ 19
- 5 – 2x ≥ 3
Systems of Equations
Lastly, practice solving systems of equations by substitution or elimination:
- x + y = 6, 2x – y = 4
- 3x + 2y = 7, x – y = 1
- 4x – 3y = 5, 2x + y = 3
- 5x + 4y = 9, x – 3y = 2
By consistently working through these types of problems, you’ll build confidence in your algebraic abilities and improve your problem-solving skills. The more practice you do, the easier it becomes to recognize patterns and apply the appropriate techniques quickly and accurately.
Important Tips for Trigonometric Identities
Trigonometric identities are fundamental tools for simplifying expressions and solving equations in advanced mathematics. Mastering these identities allows you to manipulate and transform complex trigonometric expressions with ease. Recognizing key patterns and applying the right identities at the right time is essential for efficient problem-solving.
Here are some important tips to keep in mind when working with trigonometric identities:
- Know the basic identities: Start by familiarizing yourself with the core trigonometric identities, such as the Pythagorean identities, reciprocal identities, and quotient identities. These form the foundation for simplifying more complex expressions.
- Practice simplifying both sides: When proving an identity, always simplify both sides of the equation separately before comparing them. Often, one side will simplify into a recognizable form of the other side.
- Use substitutions effectively: In some cases, substituting one trigonometric function for another (e.g., using sin²(x) + cos²(x) = 1) can help reduce the complexity of the expression.
- Look for common denominators: When working with sums or differences of trigonometric functions, combine them by finding a common denominator. This often makes it easier to simplify the expression.
- Factor when possible: If you see a common factor in a trigonometric expression, factor it out to simplify the problem. This is especially helpful in identities involving squares or higher powers.
- Be mindful of angles: Remember that many trigonometric identities involve specific angle relationships. Pay attention to angle sum and difference identities, as well as double angle and half-angle formulas.
- Work systematically: Approach each identity step-by-step, using logical reasoning and careful algebraic manipulation. Trying to skip steps can often lead to mistakes or confusion.
By keeping these tips in mind and regularly practicing, you’ll become more proficient in recognizing and applying trigonometric identities, ultimately making problem-solving faster and more efficient.
Using the Unit Circle in Trigonometry
The unit circle is a powerful tool for understanding the relationships between angles and their corresponding trigonometric functions. By representing these relationships on a circle with a radius of one unit, the unit circle provides a visual way to explore sine, cosine, and other functions. It allows you to easily determine values for various angles and solve problems involving trigonometric functions.
The key idea behind using the unit circle is that the coordinates of any point on the circle correspond to the values of the sine and cosine functions for the associated angle. The angle is measured from the positive x-axis, and as the angle increases, the point moves around the circle. Understanding how to read these coordinates and apply them to trigonometric functions is essential for solving many types of problems.
Unit Circle Overview
Below is a table showing key angles on the unit circle and their corresponding sine and cosine values:
| Angle (in degrees) | Angle (in radians) | Sine Value | Cosine Value |
|---|---|---|---|
| 0° | 0 | 0 | 1 |
| 30° | π/6 | 1/2 | √3/2 |
| 45° | π/4 | √2/2 | √2/2 |
| 60° | π/3 | √3/2 | 1/2 |
| 90° | π/2 | 1 | 0 |
| 180° | π | 0 | -1 |
| 270° | 3π/2 | -1 | 0 |
| 360° | 2π | 0 | 1 |
This table is essential for solving many trigonometric problems, especially when working with angles in standard positions. By memorizing key values from the unit circle, you can quickly find the sine and cosine of any angle and apply them in calculations.
Applications of the Unit Circle
The unit circle is used in many areas of trigonometry, including:
- Solving trigonometric equations: The unit circle allows you to solve equations by finding the corresponding angles for given trigonometric values.
- Identifying symmetry: The unit circle helps identify periodic behavior and symmetry in trigonometric functions, such as the repeating nature of sine and cosine functions.
- Graphing trigonometric functions: Understanding the unit circle can help you graph sine, cosine, and tangent functions, as well as other derived functions.
By mastering the unit circle, you gain a deeper understanding of trigonometric functions and their properties, which is essential for tackling more advanced topics in mathematics.
How to Approach Rational Expressions
Rational expressions, which involve fractions with polynomials in the numerator and denominator, can often seem complex at first glance. However, breaking down these expressions into simpler parts and applying systematic steps can help simplify and solve them more effectively. Understanding how to manipulate these expressions is essential for tackling more advanced algebraic problems.
Here are key steps to follow when working with rational expressions:
- Simplify the expression: Before proceeding with any calculations, check if the numerator or denominator can be factored. Factoring helps simplify the expression, making it easier to work with.
- Identify restrictions: Determine any values of the variable that would make the denominator equal to zero. These values must be excluded from the solution as division by zero is undefined.
- Reduce common factors: After factoring both the numerator and denominator, look for common factors. Cancel out any common factors between the numerator and denominator to simplify the expression.
- Find the least common denominator (LCD): When adding or subtracting rational expressions, identify the least common denominator to combine the terms. The LCD helps you combine fractions with different denominators into a single expression.
- Perform arithmetic operations: Once the rational expressions have the same denominator, you can add or subtract them. For multiplication and division, multiply or divide the numerators and denominators accordingly, then simplify the result.
- Check for any further simplifications: After performing the required operations, ensure that the expression is fully simplified. Factor if needed and cancel any additional common factors.
By following these steps and practicing regularly, you will become more comfortable with rational expressions and their manipulation. Mastering these techniques is essential for solving a variety of algebraic problems involving fractions and polynomials.
Solving Logarithmic and Exponential Equations
Solving equations that involve logarithms and exponents can initially seem daunting, but with a clear understanding of their properties, you can approach these types of problems with confidence. These equations often require transforming one form to another to isolate the variable, and once this is done, solving becomes straightforward. A systematic approach can help simplify these complex expressions and lead to accurate solutions.
Solving Logarithmic Equations
Logarithmic equations are equations where the unknown appears inside a logarithmic function. To solve these equations, follow these key steps:
- Rewrite the equation in exponential form: Use the property that the logarithmic equation
logb(x) = yis equivalent toby = x. This can help eliminate the logarithm and solve for the variable. - Isolate the logarithmic term: Before applying the inverse operation, make sure the logarithmic expression is isolated on one side of the equation.
- Check for extraneous solutions: After solving, substitute the solution back into the original equation to ensure it satisfies the equation, as logarithmic functions can sometimes introduce extraneous solutions.
Solving Exponential Equations
Exponential equations, where the unknown appears in the exponent, are solved by isolating the exponential expression and then applying logarithms. Here’s how:
- Isolate the exponential expression: If possible, rewrite the equation so that the exponential expression is isolated on one side of the equation.
- Take the logarithm of both sides: Apply a logarithm (common logarithm or natural logarithm) to both sides of the equation. This will allow you to bring down the exponent, making it easier to solve for the variable.
- Simplify the resulting expression: Use logarithmic properties to simplify the equation and solve for the unknown variable.
- Verify the solution: As with logarithmic equations, always substitute the solution back into the original equation to check for extraneous solutions.
Both types of equations require a solid understanding of logarithmic and exponential properties. With practice, you will become proficient at recognizing when to apply these techniques and solving equations efficiently.
Sequences and Series Explained
Understanding sequences and series is essential for solving a variety of mathematical problems. A sequence is simply a list of numbers arranged in a specific order, while a series is the sum of the terms in a sequence. The study of these concepts is vital for understanding patterns and solving problems related to growth, decay, and other real-world phenomena.
There are different types of sequences, such as arithmetic and geometric, each with its own defining properties. An arithmetic sequence has a constant difference between consecutive terms, while a geometric sequence has a constant ratio between consecutive terms. Recognizing these patterns allows you to apply formulas to find missing terms or calculate sums.
When working with series, the goal is often to find the sum of the terms. The sum of the terms in an arithmetic or geometric sequence can be computed using specific formulas, which are designed to simplify the process of adding large numbers of terms. In some cases, infinite series are studied, where the number of terms approaches infinity. Special techniques, such as limits, are used to determine the sum of these series.
By mastering the concepts of sequences and series, you will be able to solve complex mathematical problems involving patterns, sums, and sequences of numbers, all of which are crucial for higher-level studies in mathematics and its applications.
Limits and Continuity Explained
Understanding the concepts of limits and continuity is fundamental to analyzing the behavior of functions as they approach certain points. These concepts are crucial for understanding how functions behave near specific values, whether they approach infinity, zero, or a particular number. They also play a key role in determining whether a function is smooth and predictable, without any sudden jumps or breaks.
Limits and Their Importance
A limit refers to the value a function approaches as the input gets closer to a specific point. The limit helps to describe the behavior of functions at points where they may not be directly defined or when they exhibit extreme behavior. For example, when approaching infinity, the function might grow without bound, or it might approach a finite value.
To compute limits, there are several techniques, including direct substitution, factoring, or applying special limit laws. Understanding these methods allows for the determination of the behavior of a function at specific points, even when the function itself may not be directly evaluable at that point.
Continuity and Smoothness of Functions
Continuity, on the other hand, describes whether a function is “smooth” at a given point. A function is continuous at a point if there is no sudden jump or break in its value at that point. For a function to be continuous over an interval, it must be continuous at every point within that interval. This smoothness ensures that the function behaves predictably and that small changes in the input lead to small, corresponding changes in the output.
In more formal terms, for a function to be continuous at a point, three conditions must be met: the function must be defined at that point, the limit of the function as it approaches that point must exist, and the value of the function at that point must equal the limit. If any of these conditions fail, the function is considered discontinuous at that point.
Grasping these concepts allows for a deeper understanding of functions and is foundational for further study in calculus and other areas of advanced mathematics.
Word Problems and Solutions
Mathematical word problems present real-life scenarios that require the application of various mathematical principles to solve. These problems often involve translating a written description into an equation or formula that can be solved step by step. By practicing these problems, you can strengthen your ability to analyze situations and apply mathematical concepts effectively.
Steps for Solving Word Problems
The key to solving any word problem is breaking it down into manageable steps. First, identify the unknowns and assign variables to represent them. Then, translate the given information into mathematical expressions or equations. After forming the equation, solve for the unknowns using appropriate methods, such as algebraic manipulation, substitution, or elimination.
In some cases, it may be helpful to draw a diagram or make a table to better visualize the situation. This can simplify the process, especially for problems involving geometry, rates, or mixtures. Finally, always check the solution in the context of the problem to ensure it makes sense and meets the requirements of the question.
Examples of Common Word Problems
Word problems can cover a wide range of topics, including geometry, motion, finance, and mixtures. Here are a few examples:
- Distance Problems: Problems that involve calculating the distance traveled based on speed and time.
- Work Problems: Problems that involve determining how long it will take to complete a task when working together or at different rates.
- Mixture Problems: Problems that involve mixing two or more substances and calculating the resulting concentration.
Each type of word problem requires different strategies and techniques to solve, but by practicing regularly, you’ll become more confident in your ability to approach and solve these types of questions effectively.
Complex Numbers in Mathematics
Complex numbers are a fundamental concept that extends the real number system to solve equations that don’t have solutions in the real number set. These numbers consist of two parts: a real component and an imaginary component. The imaginary unit, denoted as i, is defined as the square root of -1. This allows mathematicians to work with numbers that cannot be represented on the real number line.
Understanding complex numbers is crucial for solving problems in fields such as engineering, physics, and computer science. They are used in various applications, including signal processing, control theory, and quantum mechanics. A complex number is typically written in the form a + bi, where a is the real part and bi is the imaginary part.
Basic Operations with Complex Numbers
When working with complex numbers, it’s important to know how to perform basic operations such as addition, subtraction, multiplication, and division. These operations are similar to those with real numbers but involve the manipulation of both the real and imaginary parts.
| Operation | Formula | Example |
|---|---|---|
| Addition | (a + bi) + (c + di) = (a + c) + (b + d)i | (3 + 2i) + (1 + 4i) = 4 + 6i |
| Subtraction | (a + bi) – (c + di) = (a – c) + (b – d)i | (3 + 2i) – (1 + 4i) = 2 – 2i |
| Multiplication | (a + bi) * (c + di) = (ac – bd) + (ad + bc)i | (3 + 2i) * (1 + 4i) = 11 + 10i |
| Division | (a + bi) / (c + di) = ((a + bi) * (c – di)) / (c^2 + d^2) | (3 + 2i) / (1 + 4i) = 0.5 – 0.5i |
Each operation follows specific rules that help simplify expressions and provide meaningful results. Mastering these operations is key to solving equations and applying complex numbers in various scenarios.
Strategies for Time Management During Exams
Effective time management is essential for success in any assessment. It ensures that you can answer all questions, manage stress, and use your allotted time wisely. Having a structured plan and knowing how to pace yourself during a test can make a significant difference in your performance. In this section, we’ll explore key strategies to maximize efficiency and minimize the risk of running out of time.
Planning Your Approach
Before diving into the questions, take a few moments to survey the entire test. Skim through the questions to get a sense of their difficulty and the time you might need for each. This can help you prioritize sections, tackle the easier questions first, and save more time for the more challenging ones. It’s also helpful to note any questions that require more time so you can allocate your resources accordingly.
- Start with the easiest questions: Answering the questions you’re most confident in first can boost your morale and help you manage your time effectively.
- Set time limits: For each section or set of questions, set a timer to ensure you don’t linger too long on any one part. A common rule is to spend no more than 1-2 minutes per point value of a question.
- Leave no questions blank: Even if you’re unsure about a question, make an educated guess. In many cases, it’s better to try something than leave it unanswered.
Managing Stress and Avoiding Panic
Staying calm during the test is just as important as managing your time. Stress can lead to mistakes and waste valuable minutes. One effective way to prevent stress from overwhelming you is to practice deep breathing techniques or take short breaks between sections to clear your mind. If you find yourself stuck on a difficult question, move on to the next one and return to it later. This will help you avoid wasting time and ensure that you complete the test with confidence.
By planning ahead, keeping track of time, and staying calm, you’ll be better equipped to handle any challenge that comes your way during the assessment. Time management is a skill that, when practiced, can greatly improve your efficiency and overall performance.
How to Review Effectively
Reviewing concepts thoroughly before any assessment requires a systematic approach. It’s not just about reading notes or redoing problems; it involves reinforcing understanding, identifying weak spots, and ensuring that all necessary topics are covered. A well-structured study plan can help solidify knowledge, making it easier to apply when needed. In this section, we’ll discuss key strategies for reviewing mathematical concepts effectively.
1. Identify Core Topics
Start by identifying the core areas that are most likely to be tested. Whether it’s functions, equations, or graphs, focusing on the fundamental topics will give you a strong foundation. Create a list of these key areas and ensure you understand the core principles behind them. Once you’ve pinpointed the areas to focus on, break them down into manageable sections to avoid feeling overwhelmed.
2. Practice Consistently
Practice is essential to mastering any mathematical concept. Begin by solving a variety of problems that cover all difficulty levels. Focus on the problems that challenge you the most, as they will help improve your problem-solving skills. It’s crucial to go beyond rote memorization and understand the reasoning behind each solution. Doing so will improve your ability to tackle unfamiliar problems with confidence.
3. Use Study Aids
Utilize various resources such as online tutorials, textbooks, and study groups. Sometimes a different explanation or method of teaching can make complex concepts easier to grasp. Online resources and video lessons can provide visual demonstrations of tricky topics, allowing for better comprehension. Group study sessions can also be beneficial for discussing concepts and solving problems together.
4. Review Mistakes
Take time to review the mistakes you make during practice. Understanding why an answer was incorrect is a powerful learning tool. It will help you identify patterns in your errors and correct them before the assessment. Don’t just move on after getting an answer wrong–analyzing your mistakes ensures that you avoid repeating them in the future.
5. Test Yourself Under Timed Conditions
Simulating the conditions of the actual assessment is one of the most effective ways to prepare. Set a timer and complete practice problems within the allocated time. This will help you manage time effectively during the actual test and identify any areas where you need to improve your speed and accuracy. Practicing under timed conditions will also help reduce anxiety and boost confidence.
By following a structured review plan, practicing regularly, and actively learning from your mistakes, you can approach any mathematical challenge with confidence and preparedness. Remember, consistent review and understanding the underlying principles are key to success.
Key Formulas to Memorize for Success
Memorizing essential formulas is critical to mastering mathematical concepts and performing well in assessments. Knowing the right equations and understanding their applications can simplify problem-solving, making it easier to tackle complex tasks. In this section, we’ll focus on some key formulas that are widely applicable and essential for success in a variety of mathematical challenges.
Essential Algebraic Formulas
Algebraic formulas form the foundation for many advanced mathematical topics. Familiarity with these can significantly improve problem-solving skills. Here are some of the most important formulas to remember:
| Formula | Description |
|---|---|
| Quadratic Formula | x = (-b ± √(b² – 4ac)) / 2a |
| Difference of Squares | a² – b² = (a + b)(a – b) |
| Factorization of a² + 2ab + b² | (a + b)² |
| Sum of Cubes | a³ + b³ = (a + b)(a² – ab + b²) |
Key Trigonometric Formulas
Trigonometric equations and identities are crucial for solving problems related to angles, circles, and periodic functions. Below are some of the most frequently used formulas:
| Formula | Description |
|---|---|
| Pythagorean Identity | sin²θ + cos²θ = 1 |
| Sum of Angles (Sine) | sin(A + B) = sinA cosB + cosA sinB |
| Double Angle (Cosine) | cos(2A) = cos²A – sin²A |
| Law of Sines | (sinA / a) = (sinB / b) = (sinC / c) |
Mastering these formulas can help you approach problems more efficiently and reduce the time spent on solving them. It’s important to not just memorize them but also understand their applications and how they relate to each other. With consistent practice, these formulas will become second nature, enabling you to handle a wide variety of tasks with confidence.