
Achieving success in mathematics requires both practice and understanding. To excel, it’s important to focus on grasping the core principles and applying them to various problems. With the right guidance, students can navigate through challenges with confidence and build a solid foundation for more advanced topics.
Effective problem-solving is the key to mastering any subject, and having access to the right resources can make all the difference. This guide provides a comprehensive look at how you can approach practice exercises and evaluate your progress. By following a structured approach, learners can easily track their improvement and pinpoint areas that need further attention.
Practicing consistently with well-designed materials helps reinforce learning and ensures long-term retention of concepts. With each step, students gain the tools they need to tackle even the most complex topics. The resources covered here are designed to support learners in achieving their full potential, making math more approachable and less intimidating.
Big Ideas Math Red Assessment Overview
Understanding key learning tools is essential for students aiming to improve their mathematical skills. These resources are designed to guide learners through a series of carefully structured challenges, reinforcing critical concepts and providing valuable feedback. The following section outlines how these materials are organized and how they can be utilized to maximize learning outcomes.
Structure and Purpose
The resources in question focus on helping students consolidate their understanding of core mathematical principles. They provide a systematic approach to reviewing and practicing topics covered throughout the curriculum. With each task carefully designed to test various problem-solving techniques, students can engage with the material at their own pace while reinforcing their knowledge.
Key Features
- Comprehensive exercises: Each task is designed to target specific concepts, allowing students to address areas where they may need more practice.
- Step-by-step solutions: The provided solutions offer clear guidance, breaking down complex problems into manageable steps for better comprehension.
- Progress tracking: Learners can monitor their improvement, helping them identify strengths and areas for growth.
- Reinforcement of concepts: Repeated exposure to similar problems ensures that students solidify their understanding over time.
By utilizing these tools effectively, students can make significant strides in mastering essential mathematical skills, preparing them for more advanced topics in the future.
Understanding the Assessment Book Structure
The structure of this learning resource is designed to guide students through a logical progression of mathematical concepts. Each section is carefully arranged to provide a balance of theory, practice, and evaluation, ensuring that learners can reinforce their understanding while applying new skills. The organization is intended to offer a step-by-step approach that encourages mastery of each concept before moving on to more complex topics.
At the heart of this structure are various sections that focus on different aspects of learning. The content is divided into units, each targeting a specific skill or group of related skills. Within each unit, students will find a series of problems and exercises that range from basic to more challenging, helping them build confidence while also encouraging deeper problem-solving abilities.
As students progress, they will encounter assessments designed to evaluate their understanding. These assessments provide valuable feedback, allowing learners to identify areas where they may need additional practice. This structured approach helps ensure that students are not only absorbing information but also applying it effectively to solve problems.
How to Use the Answer Key Effectively
The answer key is an essential tool for reinforcing learning and ensuring that students understand both the correct solutions and the process behind them. Using it properly can significantly enhance learning, helping students identify their mistakes and correct them. However, it’s important to use this resource strategically to maximize its value.
To make the most of the provided solutions, consider the following guidelines:
- Attempt problems first: Always try to solve the exercises on your own before consulting the key. This allows you to identify areas of confusion and challenges you to think critically.
- Check your work: After attempting each problem, compare your solution with the provided answer. If your solution is correct, review your approach to reinforce your understanding. If incorrect, analyze the answer key carefully to see where you went wrong.
- Understand the process: Focus not just on the final result but also on the steps involved in solving each problem. Understanding the reasoning behind each solution helps you apply similar techniques to other problems.
- Avoid over-reliance: While the key is a valuable resource, avoid using it too early in the process. It should be used as a tool for confirmation, not a crutch to rely on before making an effort.
By following these steps, students can ensure that they are using the solution guide as an effective learning tool that encourages self-reliance, critical thinking, and continuous improvement.
Key Concepts in Big Ideas Math
At the core of any effective learning material are the fundamental principles that help students understand and solve problems. These core concepts form the foundation upon which more advanced topics are built. A strong grasp of these basic principles allows learners to tackle increasingly complex challenges with confidence.
Each unit in the curriculum is designed to introduce and reinforce essential mathematical concepts that are central to problem-solving. These include:
- Problem-solving strategies: Techniques that guide students in breaking down complex problems into simpler, more manageable steps.
- Conceptual understanding: Developing a deep understanding of core principles, such as operations, equations, and functions, rather than just memorizing formulas.
- Critical thinking: Encouraging students to analyze problems from different angles and explore various methods for finding solutions.
- Application of knowledge: Learning how to apply mathematical concepts in real-world situations, making the subject more relevant and engaging.
By focusing on these foundational ideas, learners can build a robust understanding of mathematics, equipping them with the tools they need to succeed in more advanced topics and everyday problem-solving.
Step-by-Step Solutions for Practice Problems
One of the most effective ways to master new concepts is by working through practice exercises in a systematic and structured manner. Step-by-step solutions allow students to follow along with the logical progression of problem-solving, ensuring that they understand each stage of the process. This method not only helps clarify difficult concepts but also builds confidence in applying knowledge independently.
Each practice problem is paired with a detailed, sequential solution that breaks down the steps involved. By following these steps carefully, students can:
- Understand the process: Step-by-step guidance ensures that learners comprehend how each solution is reached, rather than simply memorizing the final answer.
- Build problem-solving skills: By repeating similar problems with varying difficulty levels, students develop the ability to approach challenges with a structured mindset.
- Identify areas of improvement: Breaking problems down into smaller steps allows learners to pinpoint where they made mistakes and correct them.
- Strengthen conceptual understanding: Detailed solutions help reinforce the theoretical concepts behind each problem, making abstract ideas more tangible and easier to grasp.
By working through problems with a focus on the process, students can gain a deeper understanding of the material and improve their ability to solve similar problems on their own in the future.
Common Mistakes to Avoid in Assessments
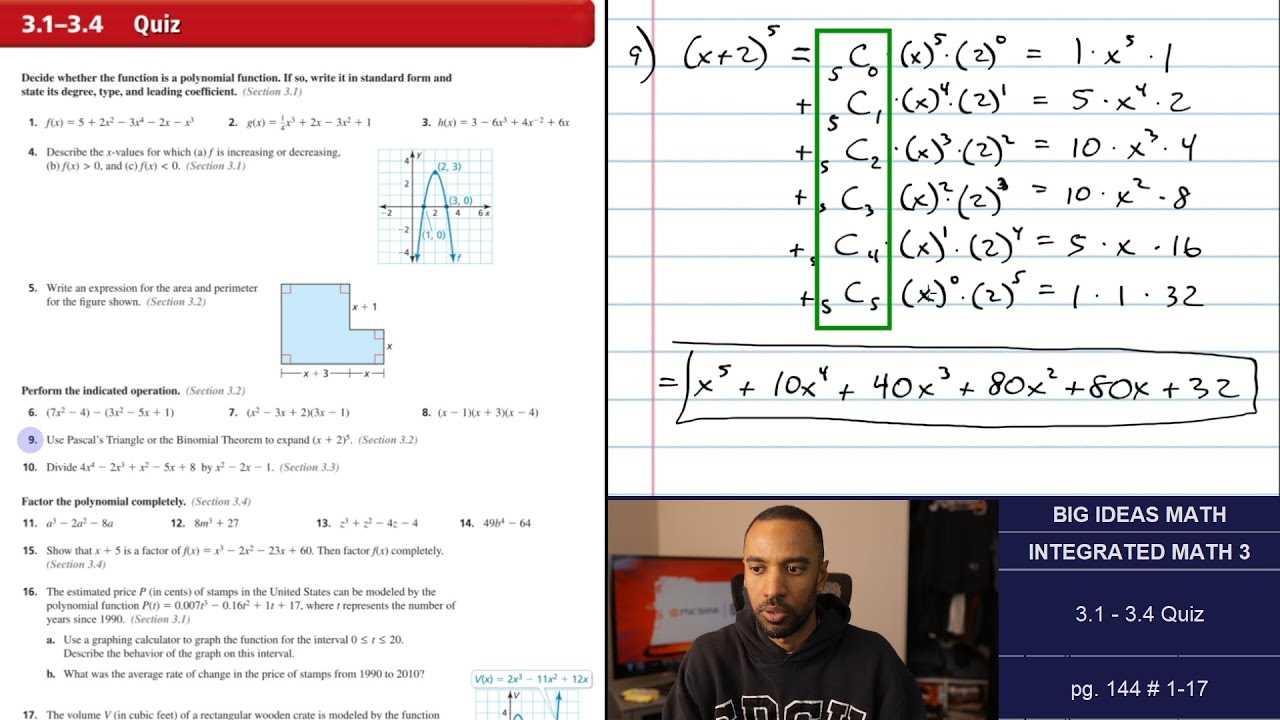
When working through practice exercises or tests, it’s easy to make mistakes that can undermine your progress. Often, these errors are not due to a lack of understanding, but rather due to careless mistakes, misinterpretation of questions, or failure to follow the correct steps. Recognizing and avoiding these common pitfalls can greatly improve your performance and deepen your grasp of the material.
Overlooking Instructions and Details
One of the most frequent mistakes is neglecting to carefully read the instructions. Simple oversights, such as missing a key condition or misinterpreting a question, can lead to incorrect answers. Always take a moment to review the instructions before diving into the problem.
- Misreading questions: Make sure you understand what is being asked. Pay attention to keywords such as “simplify,” “solve,” or “explain.”
- Skipping steps: Rushing through a problem can result in missed steps that are crucial to finding the correct answer. Always follow the procedure and check each part of your work.
Failing to Check Work
Another common mistake is not reviewing your answers before submitting or finalizing your work. Small calculation errors or overlooked details can easily go unnoticed without a careful review. A quick second look can often reveal mistakes that would otherwise be missed.
- Not verifying solutions: After solving a problem, take the time to go back and check your work, especially for complex or multi-step problems.
- Ignoring units or formats: Double-check the units of measurement and the format required for the answer, ensuring they align with the question’s specifications.
By being mindful of these mistakes, students can avoid common traps and approach problems with greater accuracy and confidence.
How to Improve Math Problem-Solving Skills
Developing strong problem-solving abilities is crucial for tackling mathematical challenges effectively. It involves not only understanding concepts but also learning how to approach problems with a strategic mindset. By refining your techniques and practicing regularly, you can enhance your ability to break down complex problems and find solutions more efficiently.
Approach Problems Step by Step
One of the most effective ways to improve problem-solving is to follow a systematic approach. By tackling each problem in stages, you reduce the chance of errors and ensure that you cover all aspects of the question.
- Understand the problem: Take a moment to fully read the problem and identify what is being asked before attempting a solution.
- Break it down: Divide the problem into smaller, more manageable parts. Solve each part separately and then combine the results.
- Choose the right method: Select the most appropriate technique for the problem. Whether it’s algebra, geometry, or another approach, use the method that best fits the situation.
Practice and Review Regularly
Consistent practice is key to improving your problem-solving skills. The more problems you work through, the more familiar you become with different types of challenges and techniques.
- Work on varied problems: Practice problems of different types and difficulty levels to expose yourself to a wide range of scenarios.
- Review mistakes: Analyze your errors and understand where you went wrong. This reflection will help you avoid similar mistakes in the future.
- Seek different perspectives: When stuck, consider alternative methods or consult resources to find new approaches to solving problems.
With consistent practice, critical thinking, and a methodical approach, you can significantly improve your problem-solving skills and tackle even the most challenging exercises with confidence.
Tips for Students Using the Assessment Book
When working with practice materials, it’s essential to use them efficiently to maximize learning outcomes. The key to success lies in how you approach the exercises, how you review your work, and how you apply the concepts to real-life problems. Below are several helpful strategies for students to make the most of these resources.
| Tip | Description |
|---|---|
| Start with a clear plan | Before diving into exercises, set a goal for what you wish to achieve in each session. This will help you stay focused and organized. |
| Read instructions carefully | Ensure that you understand each question before attempting to solve it. Carefully reading the prompt can prevent costly mistakes. |
| Work step by step | Break problems down into manageable parts. Tackling one section at a time helps prevent feeling overwhelmed and ensures accuracy. |
| Review your work | After completing each exercise, take the time to go back and check your solutions. Look for simple errors or overlooked details. |
| Use the solution guide wisely | Only refer to the solution key once you’ve given your best effort. Understand the steps involved and use the answers to confirm your approach. |
| Practice regularly | Consistent practice is essential for mastering concepts. Make sure to work through a variety of problems to reinforce your skills. |
| Track your progress | Keep track of which areas you’ve mastered and which need further attention. This will help you focus your efforts where they’re most needed. |
By implementing these strategies, students can enhance their problem-solving abilities, build stronger understanding, and make the most of their learning materials.
Strategies for Mastering Math Concepts
Mastering challenging topics requires more than just memorizing formulas or solving problems repeatedly. It involves developing a deep understanding of the underlying principles and applying them in various contexts. By adopting effective strategies, students can improve their comprehension, problem-solving skills, and overall confidence in the subject.
Understand the Fundamentals
The foundation of any subject is crucial to understanding more complex concepts. Begin by thoroughly learning the basic principles before advancing to more difficult topics. This solid base allows you to approach new material with greater ease.
- Build a strong foundation: Review key concepts regularly to ensure a deep understanding. Weak fundamentals will make more complex topics harder to grasp.
- Ask questions: If something isn’t clear, seek clarification. Don’t hesitate to ask your instructor or consult additional resources.
- Use real-world examples: Applying abstract concepts to everyday situations can make them more relatable and easier to understand.
Practice Consistently
Repetition is key to mastery. The more problems you solve, the better your understanding of different methods and techniques becomes. Practice not only reinforces learning but also helps develop speed and accuracy.
- Work on a variety of problems: Don’t limit yourself to one type of exercise. Try different problem types to broaden your knowledge and skills.
- Challenge yourself: Push your boundaries by attempting more difficult problems. This helps improve your critical thinking and adaptability.
- Review mistakes: Analyze errors to understand where you went wrong. This reflection is an important step in preventing future mistakes.
By combining a deep understanding of the fundamentals with consistent practice and thoughtful reflection, students can master complex concepts and approach any problem with confidence and skill.
Reviewing Answers for Better Understanding
Reviewing your work thoroughly is an essential part of the learning process. It allows you to identify mistakes, reinforce correct methods, and deepen your understanding of the concepts. Simply checking the final solution is not enough; understanding the steps that led to the answer is equally important.
| Reviewing Strategy | Description |
|---|---|
| Break down the steps | Don’t just focus on the final result. Review each step you took to reach the solution. Understanding the process helps you see where mistakes may have occurred. |
| Verify the method | Check if the method you used was the most efficient or appropriate one for the problem. Sometimes alternative methods may yield faster or clearer results. |
| Understand the concept | Make sure you grasp the underlying concept that the question is testing. If you don’t understand the principle behind the problem, you may struggle with similar problems in the future. |
| Compare with other resources | Cross-check your solution with other materials, such as notes or reference books, to ensure that you have followed the correct procedure and obtained the correct answer. |
| Ask for feedback | If possible, discuss your solution with a peer or teacher to get additional insights. Feedback can help clarify any misunderstandings or gaps in knowledge. |
By incorporating these strategies into your review process, you can ensure a deeper understanding of the material and improve your ability to solve similar problems in the future.
What Makes This Learning System Unique
What sets this approach to learning apart is its focus on fostering a deep understanding of fundamental principles while simultaneously encouraging the application of those principles in real-world scenarios. It blends structured practice with innovative techniques, creating an environment where learners are not only guided through exercises but also empowered to explore and discover new concepts on their own.
Key Features of the System
- Interactive Learning: Students are actively engaged in the learning process, with opportunities to experiment with different problem-solving methods and strategies.
- Real-World Applications: The curriculum emphasizes the relevance of concepts by relating them to practical, everyday situations, making the material more relatable and memorable.
- Step-by-Step Progression: Complex ideas are introduced gradually, allowing learners to build a solid foundation before moving on to more advanced topics.
- Visual and Collaborative Tools: Visual aids and collaborative activities enhance comprehension, making learning more dynamic and less abstract.
Benefits of the Approach
- Improved Retention: By combining theory with hands-on practice, students are more likely to retain and apply what they learn.
- Increased Engagement: The use of interactive methods keeps students engaged and motivated to learn, reducing the likelihood of boredom or disengagement.
- Critical Thinking Development: The system encourages students to analyze and think critically about problems, fostering stronger problem-solving skills.
- Flexible Learning Pace: This method allows students to progress at their own pace, ensuring that no one is left behind while also allowing for advanced learners to accelerate their progress.
Ultimately, this unique system not only improves students’ skills but also helps them develop a deeper appreciation for the subject, making learning an enriching and rewarding experience.
Understanding Common Misconceptions in Math

In the process of learning, students often develop misunderstandings that can hinder their progress. These misconceptions are typically rooted in oversimplified or incorrect interpretations of key concepts. Identifying and addressing these errors is essential to building a strong foundation and advancing in the subject. By recognizing the most frequent mistakes, learners can correct their approach and enhance their problem-solving skills.
One common issue is misapplying rules or formulas in situations where they don’t fit. This can lead to incorrect answers, even when the logic seems sound. For example, assuming that multiplication always results in a larger number, or that similar-looking problems can be solved using the same method, can create confusion. These misunderstandings often arise when learners fail to grasp the underlying principles that govern the operations and their relationships to each other.
Another frequent misconception involves confusing terminology. Words or phrases that appear similar but have distinct meanings in a mathematical context can mislead students. For instance, confusing the concepts of “perimeter” and “area” or treating fractions as though they are always larger or smaller than whole numbers can lead to errors in reasoning. Understanding the precise definitions and contexts of these terms is critical for avoiding such mistakes.
Furthermore, many learners struggle with abstract thinking, which is often required to solve more complex problems. Without a clear grasp of fundamental principles, students may resort to memorizing steps or using trial and error, which can only take them so far. To overcome this, it is important to approach the subject with an open mind, focusing on the “why” behind the solution process rather than just the “how.”
By addressing these misconceptions early on and encouraging a deeper understanding of the material, students can avoid common pitfalls and build a more accurate and comprehensive knowledge base.
How to Prepare for Math Assessments
Preparing for evaluations in any subject requires a structured approach, but in particular, it is essential to understand the concepts thoroughly. Adequate preparation involves more than just reviewing notes; it requires applying the learned material in various contexts, practicing problem-solving techniques, and reinforcing foundational knowledge. A well-rounded study routine can help minimize stress and maximize performance.
Key Preparation Steps
- Review Past Work: Go through previous exercises, homework, and quizzes to identify patterns in the types of problems and the concepts that were tested. This will help focus your efforts on the most important areas.
- Practice Regularly: Consistent practice is essential for reinforcing concepts. Make time each day to solve problems, especially in areas where you feel less confident. This repetition builds familiarity and confidence.
- Understand Core Concepts: Make sure you understand the underlying principles behind each problem type. Don’t just memorize procedures–understanding the reasoning behind them will help you approach problems from different angles.
Effective Study Techniques
- Active Learning: Instead of passively reading through notes, engage in active learning by solving problems, explaining concepts aloud, or teaching someone else what you’ve learned.
- Use Practice Tests: Take mock tests to simulate the actual exam environment. This will help you manage time effectively and identify areas of weakness before the real test.
- Ask for Help: If there are concepts or problems that remain unclear, seek guidance from a teacher or peer. Don’t wait until the last minute to ask questions.
With the right mindset and preparation strategy, you can approach evaluations with confidence and the skills needed to perform well. Consistency, understanding, and practice are key to success in any challenging subject.
Utilizing the Big Ideas Math Curriculum
The approach to learning in mathematics is shaped by the structure and content of the curriculum. By integrating key concepts and progressively building skills, students can develop a deeper understanding of the subject. A well-organized curriculum ensures that learners are guided step-by-step through complex topics, while offering plenty of opportunities for practice and application. Whether you are a student or an educator, utilizing this framework effectively can lead to greater success in mastering concepts.
Structured Learning Approach
The curriculum is designed with a clear progression in mind, moving from foundational concepts to more advanced material. This structure helps learners gradually build their knowledge, making each new topic easier to understand. To make the most of this framework:
- Follow the Sequence: Stick to the order of topics as presented. Each lesson builds on the previous one, and skipping steps can lead to gaps in understanding.
- Focus on Key Concepts: Make sure to grasp the essential ideas before moving on. These core concepts are crucial for understanding more complex topics later on.
- Apply What You Learn: After learning a new concept, apply it to solve problems. This helps reinforce the material and improves retention.
Interactive Tools and Resources
In addition to traditional lessons, the curriculum offers various resources that can be highly beneficial for deepening understanding. These tools include interactive exercises, practice tests, and step-by-step solutions that guide students through each problem. To maximize the benefits:
- Make Use of Online Tools: Many online platforms provide interactive exercises that offer immediate feedback, helping to identify areas for improvement.
- Engage with Additional Resources: Don’t rely solely on textbook examples. Use supplementary materials like video lessons or practice apps to further explore topics.
- Work with a Study Group: Collaborating with peers can help clarify difficult concepts. Teaching others what you’ve learned also reinforces your own understanding.
By engaging with the curriculum’s resources and adhering to its structured approach, learners can make steady progress and develop a strong foundation in the subject.
Effective Study Techniques for Math Success
Mastering complex topics in the field of numbers and logic requires consistent practice and the right approach to studying. It’s not enough to simply review concepts once and hope to retain them; effective study techniques can significantly improve understanding and problem-solving abilities. By adopting specific strategies, students can optimize their study sessions and retain information more effectively.
Key Strategies for Efficient Study
To achieve success in this area, a well-organized study routine and focus on active learning are essential. Below are some proven techniques that can help strengthen your grasp of important concepts:
| Study Technique | Description |
|---|---|
| Practice Regularly | Consistency is crucial. Make sure to set aside time each day to work through problems and apply what you’ve learned. |
| Work Through Examples | Go through sample problems step by step. This will help you understand the problem-solving process and improve accuracy. |
| Break Down Complex Problems | When facing a challenging problem, break it down into smaller, more manageable steps. This approach reduces overwhelm and improves comprehension. |
| Review Mistakes | Learn from your errors. Go back over incorrect answers to identify where you went wrong and how to correct it in the future. |
| Use Active Recall | Test yourself on the material regularly instead of passively reading through notes. This reinforces memory and comprehension. |
Creating a Productive Study Environment
A positive study environment plays a significant role in maximizing focus and retention. To ensure the best study experience, consider the following tips:
- Minimize Distractions: Find a quiet, well-lit space to study, free from interruptions like phone notifications or background noise.
- Stay Organized: Keep your study materials in order, including notes, textbooks, and any supplementary resources. An organized space promotes focus.
- Take Regular Breaks: Avoid burnout by incorporating short breaks into your study sessions. This helps to maintain concentration over long periods of time.
By applying these techniques and creating the right environment, you can significantly improve your ability to solve problems and understand key concepts. With regular practice and a focused approach, success is well within reach.
Why the Red Assessment Book Matters
Understanding and mastering essential concepts in various disciplines requires regular practice and structured review. Certain resources provide valuable opportunities for students to reinforce their knowledge and track their progress. One such resource plays a crucial role in evaluating comprehension, identifying gaps, and offering targeted practice exercises. It serves not only as a tool for learning but also as an indicator of areas needing further attention.
Benefits of Using this Learning Tool
This resource is indispensable for building confidence in problem-solving skills. Here are several reasons why it is a critical component of a student’s learning journey:
- Comprehensive Coverage: It offers a wide range of topics, ensuring that students review and reinforce the full spectrum of material they’ve learned.
- Progress Tracking: Regular use allows learners to monitor their progress, helping them recognize areas where they need improvement.
- Practice and Reinforcement: Through repetition and continued exercises, the material becomes more familiar, leading to better retention and application of concepts.
- Targeted Feedback: By evaluating completed exercises, students can gain insights into specific areas that require further attention or revision.
How It Enhances Learning Outcomes
Beyond just a set of questions, this resource provides the foundation for active learning. When used effectively, it encourages students to think critically, troubleshoot problems, and apply theoretical concepts in practical ways. This approach fosters deeper understanding, making learning more engaging and effective. The feedback provided through such resources is vital in shaping future study plans and ensuring steady improvement.
Assessing Your Progress and Growth
Tracking your development and improvement in any subject is essential for long-term success. Regular evaluations allow you to identify strengths, areas needing improvement, and patterns in your learning. Whether you are preparing for upcoming challenges or reviewing past material, it is crucial to understand how well you are progressing and what changes or adjustments can help enhance your overall performance.
Effective progress tracking involves more than just checking answers. It requires a comprehensive approach, considering various aspects such as problem-solving skills, conceptual understanding, and the ability to apply learned material in real-world scenarios. This ongoing reflection and analysis enable students to take ownership of their learning and ensure consistent growth.
Key Methods for Tracking Development
- Regular Self-Reflection: Take time after each exercise or practice session to reflect on what you’ve learned. Consider what worked well and where you faced challenges.
- Setting Specific Goals: Establish clear, achievable targets for each topic. This provides direction and motivates you to improve in focused areas.
- Reviewing Mistakes: Rather than simply looking at correct answers, focus on understanding why errors occurred. This will help you avoid repeating them and deepen your understanding.
- Using Feedback: Take note of any feedback provided by instructors or peers. Constructive criticism is invaluable in helping you refine your approach.
How to Measure Improvement
Measuring growth is not just about getting the right answers but about increasing efficiency, accuracy, and the ability to approach problems with confidence. Compare your performance over time, noting improvements in speed, precision, and the complexity of problems you can handle. As you consistently track your progress, you will notice patterns in your development, helping you to identify which areas still require attention and which ones you have mastered.
Further Resources for Improvement
To truly excel in any field, ongoing learning and practice are key. While in-class instruction and personal practice are invaluable, there are a variety of external tools that can significantly enhance your understanding and proficiency. These resources offer diverse approaches to support your growth, from interactive exercises to detailed explanations of complex concepts. Using these additional materials can help fill gaps, reinforce learned skills, and present new methods for solving problems effectively.
Whether you are a beginner or looking to master advanced topics, there are numerous options available to supplement your current learning path. Explore various resources to find what suits your learning style and objectives best.
Online Platforms and Tools
- Khan Academy: Provides free video lessons and practice exercises on a wide range of subjects, making it an excellent resource for both review and deeper exploration.
- Coursera: Offers specialized courses from universities and organizations, allowing you to learn at your own pace while earning certificates.
- Brilliant: Focuses on problem-solving and critical thinking, offering interactive lessons that challenge learners to apply concepts in creative ways.
- Wolfram Alpha: A powerful computational engine that helps with solving complex problems, providing step-by-step solutions and explanations.
Books and Print Materials
- Problem-Solving Guides: Books that offer step-by-step guides for tackling challenging problems can help build essential skills.
- Practice Workbooks: These often contain a variety of practice problems with detailed solutions, allowing you to hone your skills at your own pace.
- Conceptual Reference Books: Books dedicated to explaining core concepts in depth can provide a more thorough understanding, making complex topics easier to grasp.
Study Groups and Forums
- Online Forums: Communities like StackExchange or Reddit offer platforms where learners can ask questions, share knowledge, and discuss problems with others.
- Local Study Groups: Joining or forming study groups can provide the opportunity for collaborative learning, where you can tackle challenges together with peers.
Incorporating these additional resources into your routine can significantly improve your skills. Whether you prefer interactive tools, structured courses, or peer collaboration, there are abundant options available to complement your learning journey.