
Preparing for an advanced math assessment requires a strategic approach to solving complex problems efficiently. One of the best ways to get ready is by reviewing past test questions and familiarizing yourself with the format and structure of the questions. By practicing with these questions, you can sharpen your skills and improve your ability to manage time during the real assessment.
In this section, we will explore various ways to tackle a selection of sample questions from a past high-level math test. Understanding how to approach each question can help you increase both your accuracy and speed. Focusing on key techniques and learning from past mistakes will allow you to maximize your performance when it matters most.
2008 AP Calculus BC Practice Exam Answers
Understanding the correct responses to challenging mathematical problems is key to improving problem-solving abilities. By analyzing the results from a previous test, you can gain insight into the reasoning behind each solution and strengthen your approach for future assessments. This section highlights the essential steps in reviewing sample questions and ensuring you grasp the underlying principles involved.
Reviewing Problem Solving Techniques
One of the most effective ways to learn from past test material is by carefully going over the reasoning behind each correct selection. By identifying common patterns, you can adapt your problem-solving strategies to become more efficient and accurate. It’s important to examine each question individually and explore why specific choices are correct while others are not.
Improving Performance with Key Insights
Analyzing these solutions will also provide valuable insight into time management and question prioritization. Recognizing which types of problems tend to be more time-consuming or challenging can help you allocate your time wisely during an actual test. Focusing on strengths and addressing weaknesses will further boost your confidence and readiness.
Overview of the AP Calculus BC Exam
The Advanced Placement math assessment for advanced learners evaluates a broad range of mathematical concepts and skills. This high-level test challenges students to apply their knowledge and problem-solving abilities across various topics, including functions, derivatives, integrals, and series. It is designed to assess not only computational accuracy but also the depth of understanding and the ability to reason mathematically.
Understanding the structure of the assessment is key to effectively preparing. The format typically consists of two sections: one focused on multiple-choice questions and the other on free-response items. Both sections test the same core topics but differ in how students must engage with the material. Below is a breakdown of the test components:
| Section | Content | Time | Percentage of Total Score |
|---|---|---|---|
| Multiple Choice | Various questions covering all major topics | 90 minutes | 50% |
| Free Response | Open-ended problems requiring detailed solutions | 90 minutes | 50% |
By familiarizing yourself with this structure, you can prioritize study efforts and practice in areas that are both challenging and crucial for the test. Successful performance depends on both conceptual understanding and the ability to efficiently manage time while solving problems.
How to Approach Multiple Choice Questions
When faced with a set of predetermined answers, the key is to analyze each problem systematically and strategically. The goal is not only to identify the correct option but to also understand the underlying principles behind each question. This approach allows you to approach the problem with confidence and reduces the likelihood of errors.
Start by carefully reading the question and ensuring you fully understand what is being asked. It is crucial to avoid rushing through the text and missing vital details. Pay attention to key words and terms that define the specific mathematical concept at play. After identifying the core idea, evaluate each available option and eliminate those that are clearly incorrect. This process narrows down your choices, allowing you to focus on the most plausible answers.
If you are uncertain between two options, consider whether one answer fits the problem better than the other based on your understanding of the topic. Sometimes, even small details can indicate the best solution. Additionally, if you have time, double-check your calculations or logic before finalizing your response.
Importance of the 2008 Practice Exam
Reviewing material from previous years plays a significant role in preparing for future assessments. By analyzing questions and solutions from a past test, you can better understand the types of problems that are likely to appear and develop a more focused study approach. This specific set of sample problems provides a valuable opportunity to gauge your skills and identify areas that require more attention.
Gaining Insight into Test Format
One of the main benefits of working with past questions is becoming familiar with the format and structure of the assessment. Knowing how questions are framed and understanding the typical problem-solving approach expected will help you manage time and reduce anxiety during the actual test. This preparation gives you an edge when facing similar challenges.
Identifying Key Areas for Improvement
By evaluating the solutions from the previous test, you can pinpoint specific topics where your understanding may be lacking. Whether it’s a particular concept or a type of problem, knowing what needs further review helps optimize your study efforts. Focused practice on these weak areas will increase both your confidence and performance.
Strategies for Solving AP Calculus Questions

Approaching advanced math problems requires a systematic method that blends conceptual understanding with efficient problem-solving techniques. Whether the question involves derivatives, integrals, or series, using a structured approach can help you tackle even the most challenging problems with confidence. In this section, we’ll explore effective strategies to improve your performance on complex math questions.
Step-by-Step Problem Solving
Breaking down each question into smaller steps is key to solving it accurately. Here are a few strategies to keep in mind:
- Identify key concepts: Start by recognizing the main topic being tested, whether it’s a rate of change, an area, or an approximation.
- Write out known information: Organize the information given in the problem to avoid confusion and ensure clarity in your calculations.
- Choose the right method: Decide if you need to apply a specific theorem, formula, or technique based on the type of problem at hand.
- Check your work: Always double-check the steps you’ve taken and the answer choices to ensure they match the question’s requirements.
Time Management and Pacing
Managing your time effectively during a math test is crucial to completing all the questions within the allotted time. Consider the following tips:
- Prioritize easier questions: Start with the problems that seem less time-consuming or more familiar to boost your confidence early.
- Don’t dwell on difficult questions: If you find yourself stuck, move on to the next question and return to the challenging ones later if time permits.
- Allocate time for review: Leave a few minutes at the end of the test to go over your answers and check for any mistakes.
By implementing these strategies, you can improve both the accuracy and efficiency of your problem-solving, which will help you achieve a stronger performance in any assessment.
Understanding the Exam Format and Structure
Familiarity with the layout and organization of an advanced math assessment is essential for effective preparation. Knowing how the questions are distributed and how much time is allocated for each section allows you to develop strategies that align with the structure of the test. By understanding the format, you can manage your time efficiently and increase your chances of success.
Test Sections and Their Focus
The test is typically divided into two main sections: one consisting of questions that require selecting the correct option, and the other requiring open-ended, detailed solutions. Each section evaluates the same key concepts but requires different types of engagement. Here’s a breakdown of each section:
- First Section: This part consists of objective questions, where you choose the correct response from several options. It tests your ability to quickly identify the correct approach and apply your knowledge accurately.
- Second Section: Here, you are required to provide full solutions to more complex problems. These questions assess your understanding of concepts and your ability to communicate your reasoning clearly.
Timing and Pacing Tips
Time management is crucial for completing the test within the given period. Each section has a fixed time limit, and balancing speed with accuracy is key to performing well. Consider these tips:
- Start with easier questions: Quickly work through the simpler questions first to gain confidence and ensure you don’t run out of time.
- Save time for longer problems: Allocate enough time for the more complex problems in the second section, as these require more detailed work.
- Review your work: Leave time at the end to go over your answers and check for any mistakes or missed steps.
Common Mistakes on Multiple Choice Tests
Even the most prepared students can fall into traps when answering questions with predefined options. Understanding the common pitfalls can help you avoid these mistakes and improve your performance. Many errors are not due to a lack of knowledge but rather due to misunderstanding the question, rushing through, or making careless assumptions.
Misinterpreting the Question
One of the most frequent mistakes is failing to fully comprehend what the question is asking. It’s easy to assume you know what’s being asked based on familiarity with the topic, but the wording of the problem can sometimes lead to confusion. Always read the question carefully and identify the key elements before selecting an answer.
- Overlooking negative signs: Small details like minus signs can drastically change the meaning of a problem.
- Ignoring qualifiers: Words such as “always”, “sometimes”, or “never” can alter the interpretation of a question.
Rushing Through the Answer Choices
Another common mistake is rushing through the available options without thoroughly analyzing each one. When faced with multiple choices, it’s important to evaluate each option carefully. Even if an answer seems correct at first glance, ensure that it aligns with the question’s requirements.
- Eliminating options too quickly: Don’t dismiss choices prematurely. Often, the wrong options are designed to be plausible, making careful elimination necessary.
- Second-guessing: Overthinking or changing your initial answer without a solid reason can sometimes lead to choosing the wrong option.
How to Use Practice Tests Effectively
Practice tests are one of the most powerful tools for reinforcing knowledge and improving test-taking skills. When used correctly, they provide valuable insight into your strengths and weaknesses, as well as an opportunity to familiarize yourself with the format of the assessment. However, simply completing a practice test is not enough; it’s essential to approach it with a clear strategy to maximize its benefits.
Simulating Real Test Conditions
To get the most out of a practice test, try to replicate the actual testing environment. This will help you become accustomed to the time constraints and pressure of the real test. Follow these steps to simulate authentic conditions:
- Set a timer: Allocate the same amount of time you would have in the actual test to each section.
- Eliminate distractions: Find a quiet, comfortable space to take the test, ensuring you’re not interrupted during the process.
- Follow the rules: Avoid using notes, books, or any other resources unless it’s specifically allowed in your practice session.
Review and Analyze Your Performance
Simply completing a practice test is only part of the process. Afterward, thoroughly review your results to understand what went well and where improvements are needed. Focus on the following:
- Identify mistakes: Carefully examine incorrect answers and pinpoint the reasoning behind each mistake.
- Understand the correct solutions: Go beyond memorization–make sure you understand why the correct answers are right and how you can apply that reasoning in future questions.
- Track progress: Keep track of your performance over multiple practice sessions to see how you’re improving and which areas require more focus.
By analyzing your practice test results and refining your approach based on your findings, you’ll build both confidence and mastery over the material, leading to better performance in the real assessment.
Key Concepts Tested in 2008 Exam
In any advanced mathematics assessment, certain core principles and topics are consistently evaluated to measure a student’s proficiency. These concepts are essential for not only completing the questions but also for gaining a deeper understanding of the subject as a whole. In this section, we’ll explore the main ideas that were emphasized in the 2008 assessment and explain how mastering them can lead to better performance.
Fundamental Theorems and Properties
One of the most important areas covered in the assessment is the application of key theorems and properties. These fundamental principles serve as the foundation for many problems, and being comfortable with their use is critical for success.
- Integral Theorem: Understanding the relationship between differentiation and integration is vital for solving many problems, particularly those involving areas and rates of change.
- Limit Properties: Limits form the backbone of much of the analysis, and recognizing how to apply them to functions, sequences, and series is essential.
- Mean Value Theorem: A clear grasp of this theorem can be crucial for solving problems involving average rates of change over intervals.
Advanced Problem Solving Techniques

Another key aspect of the test is the ability to solve more complex problems that require higher-order thinking. These problems often combine multiple concepts and test your ability to integrate knowledge and apply it in varied contexts.
- Optimization Problems: These questions require identifying and solving for maximum or minimum values under specific constraints.
- Series and Sequences: Knowledge of convergence tests, series expansion, and their properties is essential for tackling these types of questions.
- Applications of Derivatives: Being able to apply the concepts of rates of change and slope to real-world problems is a key skill that is frequently tested.
How to Check Your Answers
Verifying your responses is a crucial step in any assessment process. It’s easy to overlook small errors, but taking the time to carefully review your work can make a significant difference in your overall performance. By employing a systematic approach to checking your work, you can increase the likelihood of identifying mistakes and ensuring the accuracy of your solutions.
Step-by-Step Review
One of the best ways to verify your solutions is by reviewing each question methodically. Start by reading the question again and confirming that your solution addresses all parts of the problem. This will help you avoid misinterpreting any details.
- Recheck calculations: Go over each mathematical operation carefully to ensure there are no arithmetic mistakes.
- Verify units and values: Double-check that your final results are consistent with the units and the scale of the problem.
- Revisit assumptions: Ensure that any assumptions you made while solving are correct and applicable to the specific situation.
Use Alternative Methods
If possible, use a different method to approach the problem and see if you arrive at the same conclusion. This can be a powerful tool for confirming the accuracy of your solutions.
- Substitution method: If applicable, substitute your solution back into the original equation to verify it works.
- Graphical verification: For certain problems, plotting a graph of your solution can provide a visual confirmation of its validity.
- Compare with benchmarks: If you have reference values or known results, compare your solutions with them to see if they are reasonable.
Tips for Time Management During the Exam
Managing your time effectively is crucial during any high-pressure assessment. The ability to allocate time wisely can determine your success in completing the test within the allotted time. By following a few strategic approaches, you can ensure that you address each question thoroughly without rushing at the end.
Prioritize and Plan
Before diving into the questions, take a moment to skim through the entire test. Identify the sections that you are most confident about and those that might require more time. This will help you plan your approach and avoid spending too much time on difficult questions early on.
- Start with easier questions: Tackle the questions you find most straightforward first to build confidence and save time for more challenging ones.
- Time allocation: Set a rough time limit for each section or question based on its difficulty level. Stick to it to avoid spending too much time on any one part.
- Leave time for review: Reserve the last few minutes to review your work and make sure everything is in order.
Stay Focused and Avoid Distractions
Distractions can significantly impact your efficiency. Stay focused and try not to dwell on questions that are taking longer than expected. If you encounter a challenging problem, move on to the next one and return to it later if time permits.
- Keep a steady pace: Work at a consistent speed, avoiding both rushing through questions and spending too much time on any single problem.
- Stay calm: If you feel stressed, take a few deep breaths and remind yourself that you have planned for this. Stay calm and continue working through the material systematically.
- Avoid overthinking: If you’re uncertain about a question, trust your first instinct and move on. Overanalyzing can waste precious time.
Using Calculators for AP Calculus BC
Calculators can be powerful tools in solving complex problems during the assessment, helping to speed up calculations and verify results. However, it’s important to use them wisely and efficiently, as they can also lead to mistakes if relied on too heavily. Understanding when and how to use a calculator is a key aspect of performing well.
When to Use the Calculator
There are certain types of problems where a calculator is not only helpful but necessary to obtain the correct solution. These may include complex integrals, derivatives, and graphing tasks that would be tedious to solve by hand. Knowing when to use the tool can save you valuable time.
- Graphing: For problems that involve plotting functions or finding intersections, a graphing calculator can quickly provide the necessary information.
- Complex calculations: Use the calculator to check your work when dealing with complicated algebraic expressions or lengthy calculations.
- Numerical solutions: In cases where exact solutions are difficult to find, a calculator can provide an accurate approximation.
Effective Use of the Calculator
While the calculator is a valuable tool, it is important to know how to use it effectively without over-depending on it. Relying on a calculator for every step can make you forget basic problem-solving techniques and reduce your ability to perform well when a calculator isn’t available.
- Know the functions: Familiarize yourself with the calculator’s functions and features before the test. This includes being able to quickly access integral and derivative functions, graphing capabilities, and solving equations.
- Double-check results: Always verify the results given by the calculator. It is easy to make input mistakes, and it’s essential to make sure the answer makes sense in the context of the problem.
- Use for verification: After manually solving a problem, use the calculator to confirm the correctness of your solution. This helps identify any errors you may have made during the process.
How to Improve Problem-Solving Speed
Improving your speed in solving mathematical problems is crucial, especially during time-limited assessments. Developing efficient strategies can help you work through questions more quickly and accurately. With practice, you can learn how to streamline your thought process and avoid unnecessary steps.
Strategies for Faster Solutions

There are several techniques that can boost your problem-solving efficiency. These methods help reduce the time spent on complex tasks while ensuring that you arrive at the correct solution.
| Strategy | How it Helps |
|---|---|
| Familiarity with Key Concepts | Mastering essential formulas, theorems, and methods ensures that you can apply them quickly without hesitation. |
| Breaking Down Problems | Divide complex problems into smaller, more manageable steps to prevent feeling overwhelmed and make it easier to spot solutions. |
| Time Management Practice | Regularly practicing under timed conditions helps you get used to working efficiently and staying on track during the test. |
| Using Approximation | When exact values are not required, approximate solutions can speed up calculations and allow you to move on faster. |
Building Speed through Practice
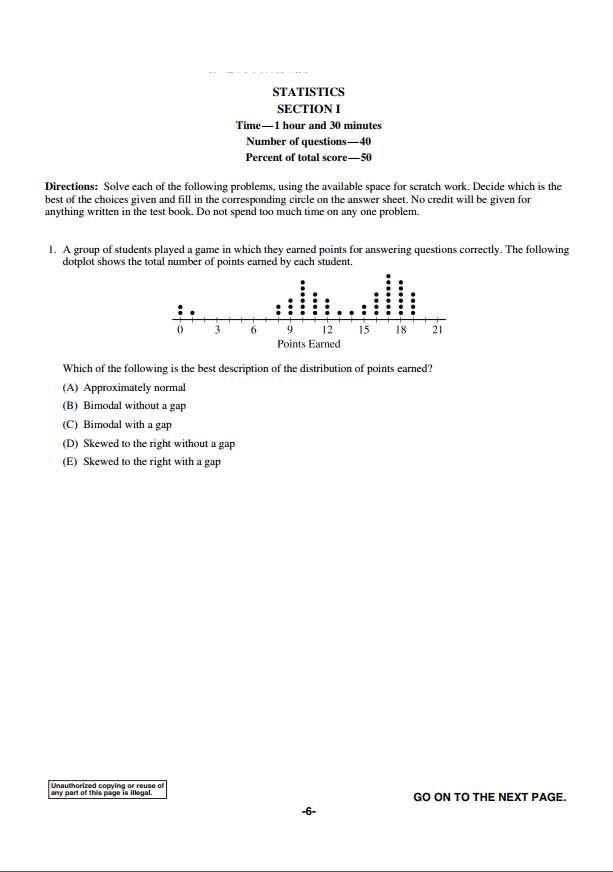
The more you practice solving problems, the faster you’ll become. Regular exercises and mock tests simulate the test environment, helping you refine your speed and accuracy. Aim for consistency in your practice routine to ensure continuous improvement.
- Start with easier problems: Begin with simpler questions to build confidence and speed before tackling more difficult ones.
- Limit unnecessary checks: While checking work is important, excessive verification can slow you down. Trust your process and check strategically.
- Increase problem difficulty gradually: As you improve, challenge yourself with more difficult problems to further enhance your speed and ability to handle pressure.
Understanding the Answer Explanations
Being able to fully comprehend the explanations behind each solution is a critical part of improving your problem-solving skills. It’s not enough to just get the correct answer–understanding why a particular approach works or why a mistake was made helps deepen your understanding and enhances your ability to apply similar strategies in future challenges.
Key Elements in Answer Explanations
Answer explanations often contain important insights into the methods and reasoning used to solve a problem. Recognizing these key elements can help you learn more effectively and apply these strategies in your own practice.
- Step-by-step breakdown: A thorough explanation often begins with the first step and progresses logically through to the final answer. Reviewing this sequence helps you see the rationale behind each decision.
- Identifying common mistakes: Many explanations highlight common errors and show how to avoid them. This is valuable in preventing these mistakes from occurring in your own work.
- Alternative methods: Some solutions provide different ways to approach the same problem. Understanding these alternatives broadens your problem-solving toolkit.
- Key concepts and formulas: Good explanations often reference important principles or formulas. Familiarizing yourself with these helps you recognize when and how to use them in different contexts.
How to Make the Most of Explanations
To truly benefit from answer explanations, it’s important to actively engage with them rather than just passively read through them. Here are some ways to make the most of the explanations:
- Review after solving: Once you’ve attempted a problem, compare your method with the explanation. This helps you identify areas for improvement and solidify your understanding.
- Ask questions: If something in the explanation is unclear, make a note of it and try to resolve your confusion. Seek help from resources or instructors if necessary.
- Practice similar problems: After understanding the explanation, work through similar problems to reinforce the method and build confidence in your ability to apply it independently.
How the 2008 Exam Prepares You
Preparation for a challenging assessment requires more than just memorizing facts and formulas. It involves developing a deep understanding of the material and honing critical thinking skills. The 2008 assessment serves as a comprehensive tool for students, helping them strengthen both their theoretical knowledge and practical problem-solving abilities.
One of the key aspects of this assessment is its ability to simulate the types of questions you are likely to face in future academic and professional situations. The structure and content of the problems help you become familiar with the format and level of complexity that you will encounter, ensuring that you are fully equipped to handle similar challenges in real-world contexts.
Building a Strong Foundation
By engaging with the problems, you strengthen your foundational knowledge. This preparation helps you understand the core concepts at a deeper level, making it easier to apply them in new and unfamiliar situations. The focus is not solely on rote learning but on developing a comprehensive grasp of underlying principles.
Boosting Test-Taking Confidence
Through repeated exposure to practice questions, students build confidence in their ability to perform under time constraints and pressure. By practicing effective strategies, such as time management and pacing, you become better equipped to tackle each question with a clear and organized approach, minimizing the likelihood of errors caused by stress or rush.
Furthermore, this preparation process fosters an adaptive mindset. By reflecting on mistakes and identifying areas for improvement, you learn to approach new problems with a growth-oriented attitude, which is invaluable for future learning experiences.
Practice Question Breakdown and Review
When preparing for a challenging assessment, it’s not enough to simply answer questions; a thorough breakdown and review of each problem is crucial for understanding both the methodology and the underlying concepts. By dissecting each practice problem, students can gain valuable insights into their thought processes, identify mistakes, and refine their problem-solving strategies.
Breaking down practice problems provides an opportunity to understand the reasoning behind correct solutions and spot common pitfalls. This method not only improves performance but also enhances long-term retention of material. Let’s explore how to approach this review process effectively:
Steps for Analyzing Each Question
- Read Carefully: Start by carefully reading the question to ensure that you understand what is being asked. Pay attention to specific terms and conditions in the problem that may influence your approach.
- Identify Key Concepts: Highlight the concepts that are being tested. Is it asking about a specific principle, formula, or method? Pinpointing the core concept allows you to focus on the right approach.
- Work Through the Solution: Break the problem into smaller steps. Work through each step systematically to find the solution, paying attention to intermediate results to avoid errors.
- Check Your Answer: After solving the problem, double-check your solution to ensure that it makes sense and aligns with the original question. If possible, try using a different method to verify the answer.
Reviewing Mistakes for Future Success
After completing the problem, it’s crucial to reflect on any mistakes made during the process. Here’s how to review them effectively:
- Identify Missteps: Was the mistake due to a misunderstanding of the question, a misapplication of a formula, or a simple computational error?
- Understand the Correct Method: Once you’ve identified the error, go back to the correct solution and carefully review the steps that lead to it. Make sure you understand why the solution is correct and how to apply the method in future questions.
- Practice Similar Questions: To reinforce your understanding and solidify your skills, try solving similar problems. This will help you become more confident and accurate in your approach.
By systematically breaking down and reviewing each practice question, you can maximize your learning and ensure that you’re fully prepared for the actual assessment.
Improving Your Accuracy on MCQs
To excel in assessments involving questions with predefined options, improving accuracy is key. The goal is not just to answer correctly but to minimize mistakes and consistently select the right option with confidence. Achieving this level of precision requires practice, attention to detail, and effective strategies for eliminating incorrect choices.
Here are a few strategies that can help increase your accuracy and reduce errors when tackling such questions:
1. Understand the Question Thoroughly
One of the main reasons for incorrect responses is misinterpreting the question. It’s essential to read each prompt carefully and make sure you fully understand what is being asked before considering the options. Look for keywords and specific instructions that can guide your response.
2. Eliminate Clearly Wrong Options
When faced with several potential answers, begin by eliminating the options that are obviously incorrect. This strategy increases your chances of selecting the right answer by narrowing down your choices. Even if you’re unsure about the exact solution, reducing the number of possible options significantly boosts your accuracy.
Additionally, look for patterns or specific details in the choices that might give clues about which options are incorrect. Common distractors often have slight variations or errors that can be easily spotted.
3. Double-Check Calculations and Assumptions
Many errors come from simple calculation mistakes or assumptions that aren’t explicitly stated in the question. Double-check any formulas you use and ensure you are applying them correctly. If the question involves numerical computations, take extra care to verify your calculations step by step.
4. Practice Under Timed Conditions

Accuracy often improves with practice, especially under time pressure. By practicing questions in a simulated environment with a set time limit, you can become more efficient and reduce the likelihood of errors due to rushing. As you get more comfortable with timing, you can focus more on the quality of your answers and less on completing the task quickly.
By applying these strategies and honing your skills, you can improve your ability to consistently answer questions accurately, even in high-pressure situations.
Next Steps After Completing the Practice Test
After completing a series of simulated questions, the next step is to analyze your performance carefully. Reviewing your results allows you to identify areas of strength and areas that require more focus. This critical evaluation will guide your future study sessions and help you refine your skills before tackling real assessments.
Here are some effective strategies for what to do next:
1. Review Incorrect Responses
Begin by carefully reviewing any incorrect responses. Understanding why you made a mistake is the first step in avoiding it in the future. Look for patterns in your errors–are they due to simple mistakes, misinterpretations, or a lack of understanding? Addressing these issues will directly impact your performance.
2. Focus on Weak Areas
After identifying which questions you struggled with, dedicate additional study time to the concepts that caused difficulty. If you encountered particular types of problems that were challenging, find similar problems to practice, or review any relevant theories or techniques that you may not have fully grasped.
3. Analyze Time Management
Time management is just as important as content knowledge. Reflect on how well you managed your time during the test. Did you spend too much time on difficult questions? Did you rush through simpler ones? Adjust your approach to ensure that you’re spending an appropriate amount of time on each question in future sessions.
4. Take a Break and Come Back Later
After a thorough review, it’s essential to step away for a moment. This break helps refresh your mind and allows you to return to the material with a clearer perspective. Coming back to reattempt questions after some time has passed can help reinforce the learning process.
5. Track Your Progress Over Time
As you continue practicing, keep track of your progress by recording which areas have improved and which still need work. This will allow you to measure your growth and fine-tune your study plan based on your evolving needs.
| Action | Purpose |
|---|---|
| Review Incorrect Responses | Identify mistakes and learn from them. |
| Focus on Weak Areas | Strengthen understanding of difficult concepts. |
| Analyze Time Management | Ensure efficient use of time in future practice. |
| Take a Break | Return with a fresh perspective to reinforce learning. |
| Track Progress | Monitor improvement and adjust study plan accordingly. |
By taking these steps, you’ll continue to build confidence and ensure that your preparation is thorough and effective as you move toward mastering the material.