Cracking the AP Calculus AB Exam Answers

Achieving top results in advanced mathematics assessments requires a strategic approach and strong understanding of key concepts. By developing a clear study plan and utilizing effective techniques, students can maximize their performance. Success hinges on mastering both theory and application under time constraints.

Familiarity with core topics, such as derivatives, integrals, and limits, is crucial for navigating through questions efficiently. Knowing how to approach various problem types with precision is just as important as understanding fundamental principles. Effective preparation is essential for building confidence and overcoming challenges.

By focusing on time management, practicing past problems, and staying calm during the assessment, students can ensure they are well-prepared. Every aspect of preparation plays a vital role in securing high marks, from knowing essential formulas to analyzing questions with a critical mindset.

Cracking the AP Calculus AB Exam Answers

Successfully navigating advanced mathematical assessments involves a combination of in-depth knowledge and strategic problem-solving. By focusing on key areas and practicing under timed conditions, students can enhance their chances of achieving top results. Precision in answering questions, combined with effective time management, plays a crucial role in excelling at these tests.

It’s essential to approach each section with a methodical mindset. This includes understanding the various question types and knowing how to quickly identify the most efficient solution strategies. Below is a helpful breakdown of common sections and their respective approaches:

Section	Approach
Multiple Choice	Focus on eliminating wrong answers and using process of elimination to boost accuracy.
Free Response	Organize answers step-by-step, showing all work clearly to earn partial credit.
Graph Interpretation	Pay attention to key features like slopes, intercepts, and areas under curves.
Application Problems	Translate real-world scenarios into mathematical models to simplify the problem-solving process.

Adopting this structured approach not only reduces anxiety but also increases efficiency during the test. Practicing regularly with sample problems and focusing on understanding key concepts rather than memorization will help solidify your grasp on difficult topics. Confidence built through consistent effort is one of the best strategies for success.

Understanding the Exam Structure

Grasping the layout of an advanced mathematics test is crucial for maximizing performance. A solid understanding of each section’s format allows for better time management and targeted preparation. Familiarizing oneself with the different types of questions and their requirements is key to navigating the assessment effectively.

General Breakdown

Typically, the test is divided into two main sections: multiple choice and free response. Each of these areas assesses different skills and requires different approaches. Here’s an overview of how each section works:

• Multiple Choice: This part usually contains a variety of questions, each with several possible answers. Speed and accuracy are essential for success here.
• Free Response: In this section, students must provide detailed solutions, often showing work step-by-step. Attention to clarity and completeness is key.

Time Allocation and Strategy

Effective time management is crucial when taking a high-stakes test. Knowing how long to spend on each section and question helps maintain focus and ensures that no part of the test is rushed.

1. Prioritize easier questions to build confidence and secure quick points.
2. Allocate time to review and refine free-response solutions.
3. Don’t spend too long on difficult multiple-choice items; move on and come back later if time allows.

By approaching the assessment with a clear strategy and understanding the test’s structure, students can improve their efficiency and ensure they are well-prepared for each section.

Key Topics to Focus On

Focusing on the core concepts covered in any high-level mathematics assessment is critical to achieving success. Understanding which areas carry the most weight can guide your study efforts and ensure you are well-prepared for the challenge. It is essential to concentrate on fundamental topics that frequently appear and form the foundation of more complex problems.

Core Concepts and Theorems

Grasping the fundamental principles is crucial for tackling a wide range of problems. These topics often require both conceptual understanding and practical application:

• Limits: Know how to evaluate limits, both analytically and graphically. Understand one-sided limits, infinite limits, and continuity.
• Derivatives: Master techniques for finding derivatives of various functions, including product, quotient, and chain rules.
• Integrals: Be comfortable with both definite and indefinite integrals. Practice integration techniques such as substitution and parts.

Problem Types to Emphasize

In addition to the core concepts, certain problem types tend to appear frequently and can be particularly challenging:

• Related Rates: These problems require a good understanding of how variables change with respect to one another and often involve derivatives.
• Area Under Curves: Both definite integrals and geometric interpretation of integrals are vital for solving these problems.
• Optimization: Problems that require maximizing or minimizing a given quantity are commonly featured, often involving critical points and first/second derivative tests.

By concentrating on these critical topics, you will have a solid foundation to approach a wide variety of questions and be better equipped for the test.

How to Manage Your Time

Effective time management is one of the most critical factors in ensuring success during high-level assessments. With multiple sections to complete and a limited time frame, knowing how to allocate your time efficiently can make the difference between finishing strong and leaving questions unanswered. Proper preparation and strategic decision-making are essential when time is of the essence.

Here are some key strategies to help you manage your time effectively:

Plan Ahead

• Know the Total Time Available: Be aware of how long you have for the entire assessment and break it down by section.
• Set Time Limits for Each Section: Divide your time according to the complexity and length of each part. For example, spend less time on multiple-choice questions and more on free-response sections.
• Account for Review Time: Set aside some time at the end to double-check your work and revisit difficult questions.

During the Assessment

• Start with Easier Questions: Quickly tackle simpler problems to build momentum and secure easy points.
• Move Quickly Through Multiple-Choice: If unsure, make an educated guess and move on. Don’t get stuck on any single question for too long.
• Don’t Rush Free-Response: Though time is limited, ensure you provide clear, organized answers to maximize scoring potential.

Final Tips

1. Stay calm and composed to avoid unnecessary stress, which can drain your time and energy.
2. Consider using a watch or clock to keep track of your pace and make adjustments as needed.
3. Remember, a balanced approach to time management not only boosts your confidence but also helps you perform at your best.

By managing your time strategically, you can tackle all sections confidently and ensure you have enough time to review and refine your responses.

Effective Study Strategies

Successful preparation for an advanced assessment requires more than just memorization; it demands a strategic approach to mastering key concepts and honing problem-solving skills. By incorporating a variety of effective study methods, students can ensure they are not only familiar with the material but also capable of applying their knowledge under timed conditions. The right study plan can make a significant difference in performance.

Active Learning Techniques

Passive reading or note-taking alone is not enough to fully prepare for complex questions. Active engagement with the material leads to deeper understanding and retention. Here are some methods to enhance your learning:

• Practice Problems: Regularly solve a variety of problems to test your understanding and reinforce key techniques.
• Teach Someone Else: Explaining concepts to others forces you to clarify your own understanding and identify gaps in your knowledge.
• Utilize Flashcards: Create flashcards for formulas, key concepts, and definitions to help with memorization and quick recall.

Study Planning and Consistency

Developing a structured study plan and sticking to it is essential for long-term retention and avoiding last-minute cramming. Consistency and time management are key:

• Set Realistic Goals: Break down your study material into manageable chunks, focusing on one topic at a time.
• Create a Study Schedule: Allocate specific time slots for each subject area, ensuring regular reviews to reinforce what you’ve learned.
• Prioritize Weak Areas: Identify your weakest topics and dedicate extra time to improving them, rather than just reviewing familiar concepts.

By employing these study strategies, students can approach the material with confidence, ensuring they are fully prepared for any challenges they may face during the assessment.

Common Mistakes to Avoid

During any advanced mathematics assessment, certain errors are commonly made that can negatively impact performance. Recognizing and avoiding these pitfalls is crucial for improving accuracy and efficiency. Many of these mistakes stem from oversights or rushed decisions, which can be prevented through careful preparation and attention to detail.

Here are some common errors that students should be mindful of:

• Misreading Questions: Skipping over important details in the wording of problems can lead to incorrect interpretations. Always read each question carefully to understand exactly what is being asked.
• Neglecting Units: Forgetting to include or convert units, especially in application-based problems, can result in incomplete or incorrect solutions. Always check your units as you work through problems.
• Rushing Through Solutions: Speed is important, but rushing through steps without carefully considering each one can lead to simple calculation errors. Take the time to review your work as you go.
• Forgetting to Show Work: In many cases, partial credit is awarded for the process, even if the final answer is incorrect. Always write out each step, showing how you arrived at your conclusions.
• Not Managing Time Properly: Spending too much time on one difficult question can cause you to run out of time for others. Keep an eye on the clock and move on if needed, returning to challenging questions later.

Avoiding these mistakes can significantly improve your chances of success. Practicing with past problems and refining your approach can help you become more aware of potential errors and eliminate them in the future.

Mastering the Multiple Choice Section

Excelling in the multiple-choice portion of an advanced mathematics assessment requires more than just familiarity with the material–it demands strategic thinking and a solid approach. The goal is not only to answer questions correctly but also to do so efficiently, without wasting valuable time on difficult problems that can be tackled later. With the right techniques, this section can be completed quickly and accurately.

Here are some effective strategies for mastering this section:

• Understand Question Format: Multiple-choice questions often present tricky alternatives designed to test deeper understanding. Read each option carefully and eliminate obviously incorrect answers first.
• Use Process of Elimination: Even if you’re unsure about the answer, narrowing down the choices can increase your odds. Dismiss clearly wrong answers to improve your chances of selecting the right one.
• Don’t Overthink: Often, the first answer that comes to mind is correct. Don’t overcomplicate problems by second-guessing yourself, unless you have a strong reason to do so.
• Manage Your Time: If a question feels too time-consuming, move on and return to it later. Completing easier questions first can help build momentum and secure points.
• Double-Check Your Calculations: If you have time, quickly verify your math before finalizing your answer. Simple calculation errors can lead to incorrect responses, even if you know the concept well.

By applying these strategies, you’ll be better prepared to tackle the multiple-choice questions efficiently and effectively, ensuring you maximize your score in this section.

Approaching Free Response Questions

Free-response questions present an opportunity to demonstrate a deeper understanding of mathematical concepts. Unlike multiple-choice problems, these require a well-thought-out approach, as you need to show your process clearly and provide a detailed solution. The key to excelling in this section lies in breaking down the problem step by step and ensuring that each part of the solution is logically connected.

Here are some strategies to help you approach these questions effectively:

• Read Carefully: Before beginning, make sure you understand what the question is asking. Highlight important information and be clear on what needs to be solved.
• Organize Your Work: Present your steps in a clear, logical order. This not only helps you stay focused but also allows graders to easily follow your thought process.
• Show All Steps: Even if you know the answer, it’s crucial to write down every step of your reasoning. Partial credit is often awarded based on the process, so be sure to demonstrate your work.
• Use Proper Notation: Ensure that you’re using correct mathematical symbols and terminology. Proper notation makes your work easier to understand and more likely to be awarded full credit.
• Double-Check Your Work: After finishing a problem, take a moment to go over your solution. Look for any small errors, such as sign mistakes or incorrect calculations, that could affect your final answer.

In free-response sections, you are often awarded points for the methodology you use to solve a problem, not just for the correct answer. Taking the time to show a complete solution can make a big difference in your score.

Utilizing Practice Exams Effectively

Practice exams are one of the most valuable tools in preparing for any rigorous assessment. They not only help familiarize you with the format and timing of the test but also provide insight into the types of questions that may appear. Taking practice tests allows you to identify weak areas, manage time effectively, and build confidence before the actual assessment day.

Simulating Real Test Conditions

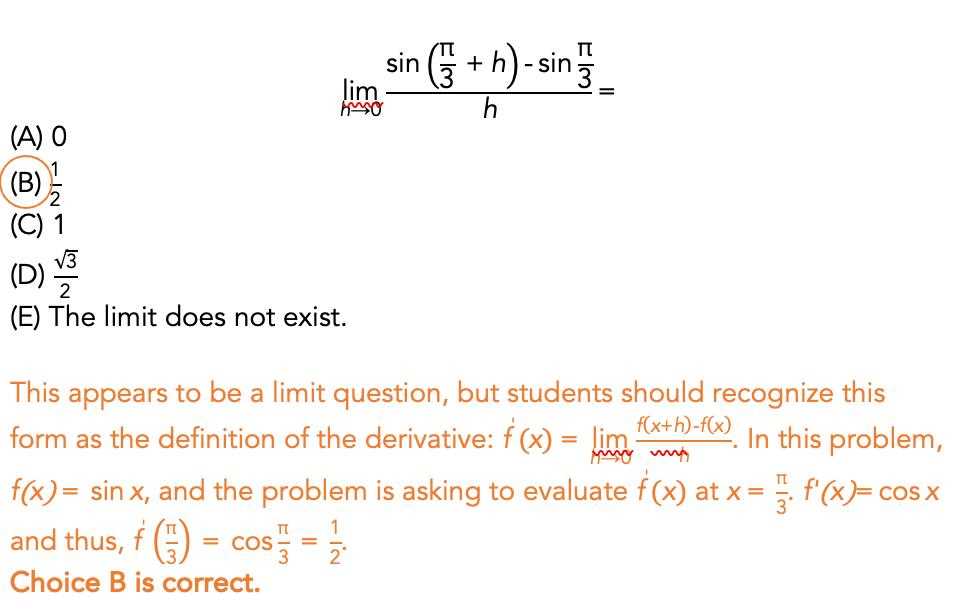
To get the most out of practice exams, it’s essential to simulate the actual testing environment as closely as possible. This means adhering to the same time limits and minimizing distractions. By practicing under test conditions, you can get a better sense of pacing and improve your ability to stay focused throughout the entire assessment.

• Set a Timer: Stick to the time limits for each section of the practice test, just as you would in the actual assessment. This helps train your brain to work efficiently under time constraints.
• Eliminate Distractions: Find a quiet space to take the practice test. Turn off your phone and avoid any interruptions to simulate the concentration required on test day.
• Take Breaks: Schedule breaks between sections to rest your mind, but be mindful of the overall time limit to ensure you’re staying on track.

Analyzing Your Performance

Once you’ve completed a practice test, it’s crucial to analyze your results carefully. This step is often overlooked but is key to improving. Focus on understanding why you missed certain questions and what mistakes you made. This will help you target specific areas for improvement and refine your problem-solving skills.

• Review Incorrect Answers: Go through each wrong answer, trying to understand the mistake. Was it a calculation error, a misinterpretation of the question, or a gap in knowledge?
• Identify Patterns: If you consistently struggle with a particular type of problem or topic, allocate more study time to that area.
• Track Progress: Keep a record of your practice test scores and track your improvement over time. This will provide motivation and give you a sense of how much you’ve grown.

Incorporating practice exams into your study routine is a highly effective way to boost performance. Not only do they help you familiarize yourself with the test structure, but they also enable you to pinpoint weaknesses and practice strategies for managing time efficiently. With consistent effort and careful analysis of your results, practice tests will be an essential tool in your preparation process.

Understanding Key Formulas and Theorems

Mastering fundamental mathematical formulas and theorems is essential for success in any advanced assessment. These core principles serve as the building blocks for solving a wide range of problems, and having a deep understanding of them allows you to apply them effectively under timed conditions. Whether dealing with derivatives, integrals, or limits, knowing when and how to use these formulas is crucial for tackling complex questions efficiently.

Here are some of the most important concepts you should focus on:

• Fundamental Theorem of Calculus: This theorem connects the concepts of differentiation and integration. Understanding it will help you simplify many problems that involve areas under curves or the rate of change.
• Chain Rule: A key rule for differentiating composite functions, the chain rule is crucial when dealing with more complex functions.
• Product and Quotient Rules: These two rules are indispensable when differentiating products or quotients of functions. Knowing how to apply these rules will save time during the test.
• Integration by Parts: This method is used to integrate products of functions. Familiarity with this technique is essential for solving a wide variety of problems that involve integration.
• Trigonometric Identities: Many problems will require you to simplify expressions involving trigonometric functions. Mastering key identities will make these problems much easier to approach.

Familiarizing yourself with these key formulas and theorems will give you the foundation needed to solve problems accurately and confidently. Regular practice will ensure that you can recall them quickly when needed and apply them efficiently under test conditions.

Tips for Staying Calm During the Exam

Test anxiety is common, but learning how to stay calm during high-pressure situations can significantly improve your performance. A clear and focused mind is essential for problem-solving, and stress can often hinder your ability to think logically. By incorporating a few techniques into your preparation, you can stay composed and approach each question with confidence.

• Practice Deep Breathing: Taking slow, deep breaths can help calm your nerves and restore focus. Try inhaling for a count of four, holding for four, and exhaling for four to relax your body and mind.
• Stay Positive: Replace negative thoughts with affirmations. Remind yourself that you’ve prepared well, and that you have the skills needed to succeed.
• Use Time Wisely: If you encounter a difficult question, don’t panic. Move on to the next one and return to it later. Time management will reduce feelings of being overwhelmed.
• Take Short Breaks: If permitted, take a brief pause during the test to stretch or close your eyes for a moment. These breaks can help refresh your mind and alleviate stress.
• Visualize Success: Before starting, close your eyes for a few seconds and picture yourself working through the problems with ease. Positive visualization can improve your mindset and reduce anxiety.

By staying calm and focused, you will be better equipped to solve problems effectively and perform at your best. Remember, taking care of your mental and emotional well-being is just as important as knowing the material itself.

Using Graphing Calculators Wisely

Graphing calculators can be powerful tools when used properly, offering quick solutions to complex mathematical problems. However, relying on them too much can hinder your understanding of key concepts. The key is knowing when to use the calculator to enhance your problem-solving process, and when to rely on your own knowledge and skills. By striking the right balance, you can make the most of this valuable resource without over-dependence.

• Understand Calculator Functions: Familiarize yourself with the basic and advanced functions of your calculator. Know how to graph functions, find derivatives, and solve equations efficiently. This will save time and prevent confusion during the test.
• Use for Verification: Use the calculator to verify your manual calculations rather than to perform all of them. For example, after solving a problem, check your results on the graphing tool to ensure accuracy.
• Practice Without It: While calculators are allowed, it’s essential to practice problems without relying on them. This helps reinforce your understanding of core concepts and ensures you’re not overly reliant on technology.
• Avoid Calculator Overuse: Use the graphing calculator as a supplement, not a crutch. When tackling simpler problems, try to solve them manually first. Only use the calculator when necessary for more complex calculations or when asked to graph a function.
• Be Aware of Restrictions: Ensure you’re familiar with any limitations on the calculator, such as which functions are prohibited or restricted. For example, certain features may be disabled during the test to maintain fairness.

When used wisely, a graphing calculator can significantly improve efficiency and accuracy. But it should be viewed as a tool to support your understanding, not replace it. Mastering the use of your calculator will help you approach questions with confidence and precision.

How to Prepare in the Final Weeks

The last few weeks before a major test can be crucial in solidifying your knowledge and boosting your confidence. During this time, it’s important to prioritize both review and practice, focusing on areas where you feel less confident while reinforcing your strengths. A well-structured study plan can help you manage your time effectively and ensure that you are fully prepared when test day arrives.

• Review Key Concepts: Go over fundamental principles and formulas that you know will appear. Make sure you have a solid understanding of topics that are frequently tested, such as limits, derivatives, and integrals.
• Focus on Weak Areas: Identify areas where you struggle and allocate extra time to them. Whether it’s graphing, application problems, or solving differential equations, give yourself time to practice these types of questions.
• Practice Under Timed Conditions: Start taking practice tests in a timed setting. This will help you manage your time effectively during the actual assessment and build stamina for longer sessions.
• Review Past Mistakes: Go through your previous practice problems and analyze where you went wrong. Understanding your mistakes is key to preventing them in the future.
• Stay Consistent: In the final weeks, it’s better to study regularly than to cram all at once. Break your study sessions into manageable chunks and ensure you’re consistently reviewing each topic.

As the test approaches, maintain a balanced approach, giving yourself time to relax and recharge. Your goal should be to feel prepared and confident, but not overwhelmed. Trust your preparation and stay focused on doing your best when the day arrives.

How to Interpret AP Scoring Rubrics

Understanding scoring rubrics is crucial to effectively approaching assessments and improving your performance. These rubrics provide a clear framework for what is expected in each section and help you understand how your responses will be evaluated. By familiarizing yourself with the structure and criteria of the scoring system, you can better tailor your approach to maximize your potential score.

Understanding Scoring Criteria

Scoring rubrics break down responses into specific categories, such as accuracy, completeness, and clarity. Each section typically has a range of points based on how well you meet these criteria. For example, in a problem-solving task, you may earn points for setting up the correct equation, applying appropriate methods, and providing a correct final answer. The key to scoring well is ensuring that each step is clearly shown and logically sound.

Breaking Down Point Allocation

Different types of questions are often weighted differently in terms of point value. For instance, a multiple-step problem might carry more points than a simple one-step calculation. Understanding how points are allocated allows you to prioritize your efforts. Focus on questions that offer the highest point value but do not neglect the smaller questions, as every point counts towards your overall score.

• Accuracy: Correct answers are essential, but remember that partial credit can be awarded if you show a logical approach, even if the final answer is incorrect.
• Clarity: Your responses should be neat and organized. Clearly label steps and provide explanations when needed, especially in free-response sections.
• Methodology: Be sure to show your work. In many cases, demonstrating your thought process is just as important as the correct final result.

By reviewing rubrics and practicing according to the scoring guidelines, you can align your preparation with the expectations set by the test. This strategy not only boosts your performance but also helps you manage your time and effort efficiently during the assessment.

Building Confidence Before the Test

Confidence plays a critical role in performing well during assessments. Mental preparedness and a positive mindset can significantly affect your approach to each question, helping you stay calm and focused. In the weeks leading up to the test, developing confidence is not just about mastering content but also about boosting self-belief through effective preparation and practice.

Positive Self-Talk and Visualization

One of the most powerful tools for building confidence is mental conditioning. Before the test, spend some time practicing positive self-talk and visualization techniques. Imagine yourself successfully solving problems and staying composed under pressure. This type of mental rehearsal can reduce anxiety and make you feel more capable when faced with challenging questions.

Mastering Content and Practice

Another essential aspect of confidence-building is thorough preparation. By consistently reviewing key concepts and practicing a variety of problems, you will strengthen your understanding and reduce uncertainty. As you become more familiar with the material, you will feel more secure in your abilities to handle different types of questions.

• Review Key Concepts: Ensure you have a solid grasp of important principles, such as derivatives, integrals, and limits.
• Simulate Test Conditions: Take practice tests under timed conditions to replicate the pressure of the real test.
• Track Your Progress: Measure improvements by revisiting difficult problems and noticing your growing ability to solve them.

Building confidence is a gradual process that comes with preparation, practice, and a positive mindset. As you progress, remember that each challenge faced and each problem solved increases your self-assurance. When you walk into the testing room with a sense of belief in your abilities, you’re more likely to perform at your best.

Post-Exam: What’s Next

After completing any significant assessment, it’s crucial to focus on the next steps, regardless of the immediate outcome. The period following the test is an opportunity for reflection, relaxation, and planning for what comes next. Understanding how to manage this time wisely can help you maintain motivation and reduce post-test stress.

1. Take Time to Relax

Immediately after the test, it’s essential to take a break. Whether you’ve performed well or are unsure, giving yourself time to unwind will help you recharge. Engaging in activities that you enjoy, like spending time with friends or pursuing a hobby, can relieve stress and improve your overall well-being.

2. Reflect on the Experience

Once you’ve had some time to relax, it’s helpful to reflect on the entire process. Consider what strategies worked well, what could be improved, and how you felt during different sections. This self-reflection helps you identify areas for future growth and builds a more strategic approach to any upcoming assessments or challenges.

3. Focus on Future Plans

After the dust has settled, start looking ahead to your future goals. Whether you’re preparing for another challenge, applying to universities, or continuing with your current studies, it’s essential to focus on next steps. Think about what you’ve learned from this experience and how you can apply that knowledge to your next academic pursuit.

• Review Test Performance: Once the results are released, review the scoring breakdown to identify areas of improvement.
• Set New Goals: Whether it’s preparing for another test or strengthening weak areas, plan your next steps strategically.
• Celebrate Achievements: Take time to recognize your efforts and accomplishments, regardless of the outcome.

Remember, the process doesn’t end with the test. The skills, strategies, and lessons learned throughout the preparation journey can be applied to future endeavors. Keep your focus on growth and take pride in your progress, both academically and personally.