Financial Mathematics Exam Questions and Answers

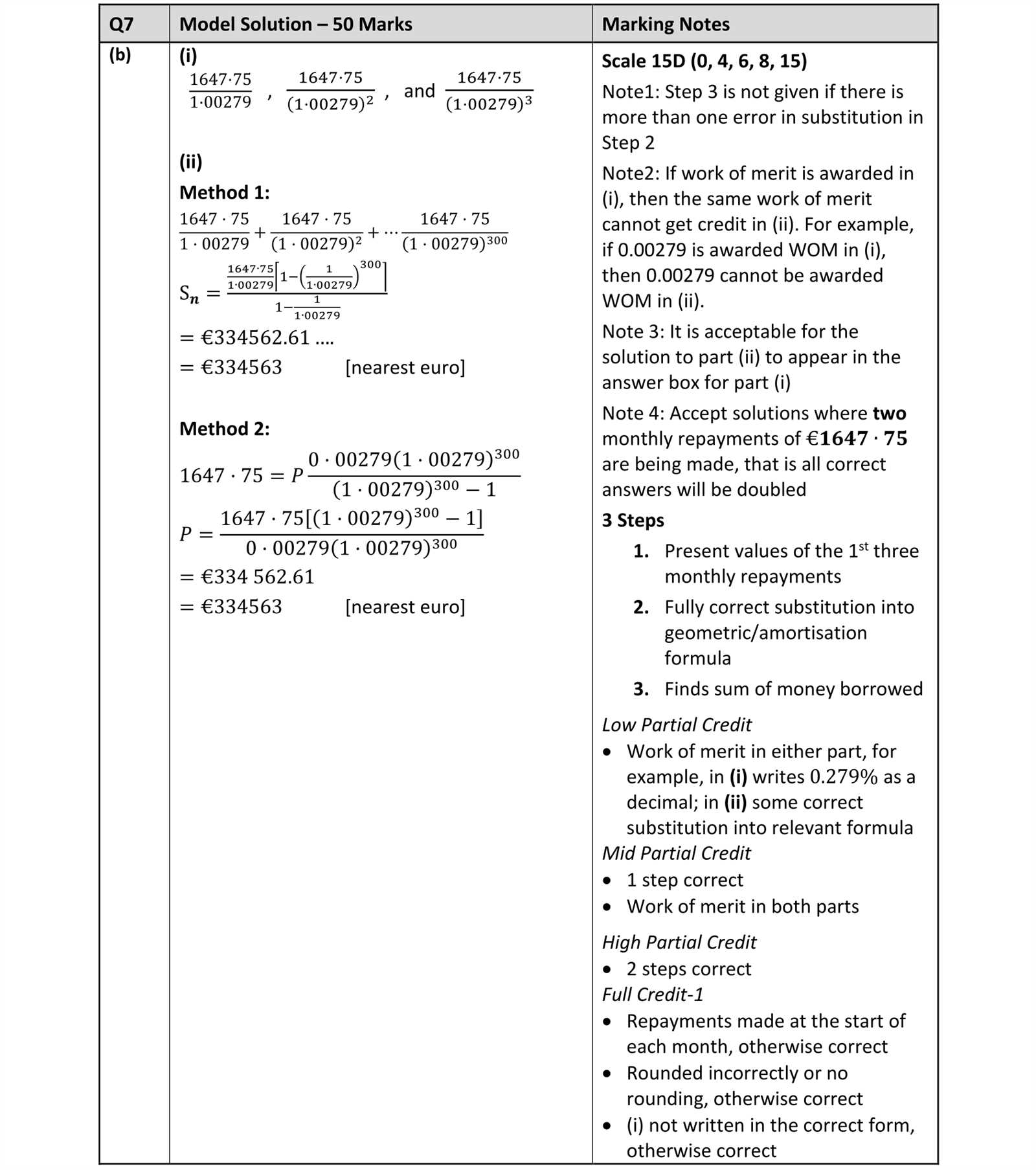
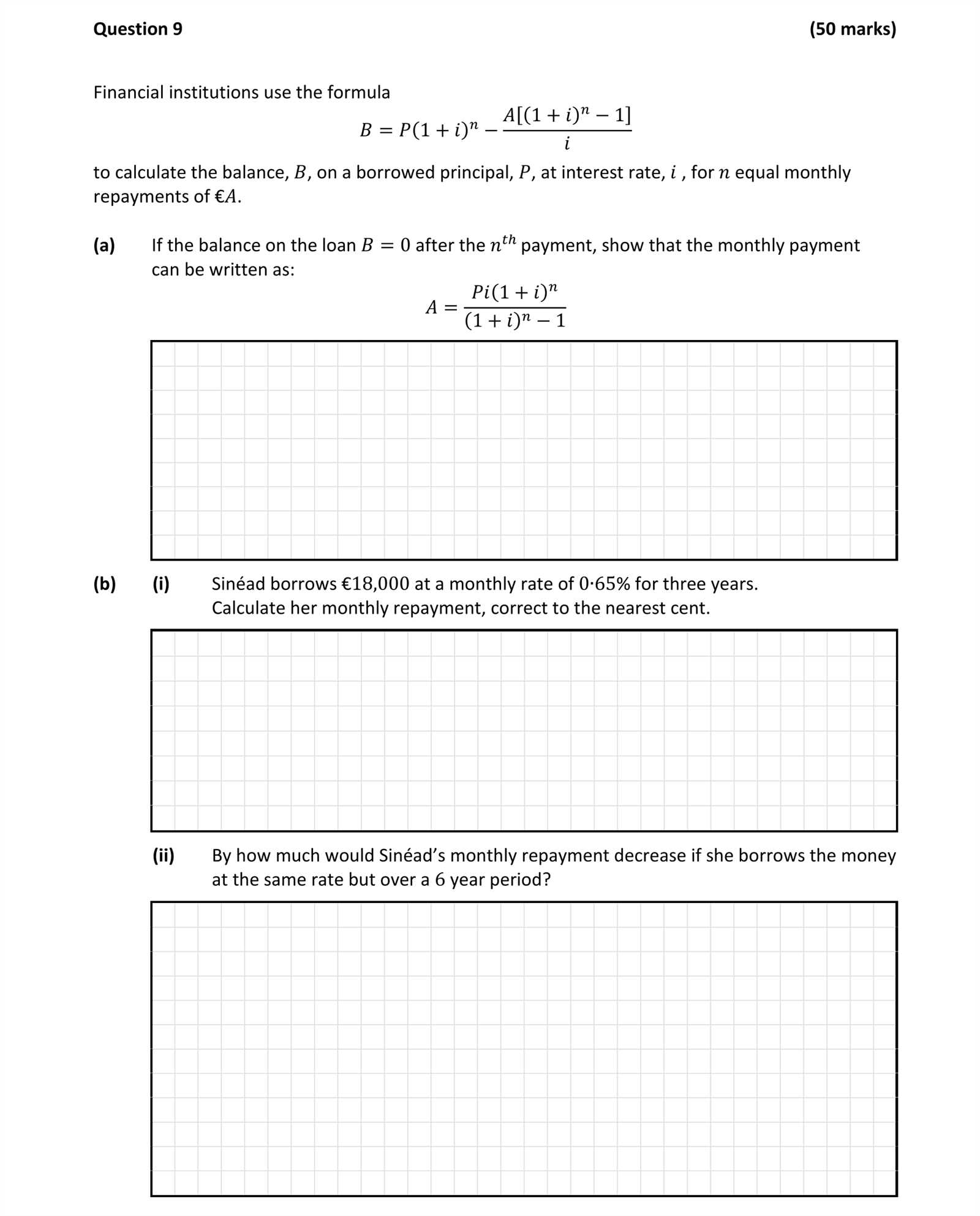
Mastering key concepts in applied finance can significantly enhance your performance during assessments. This section is designed to guide you through essential topics, providing a comprehensive understanding of the material you will encounter. By focusing on practical examples and efficient problem-solving techniques, you will be well-prepared to face challenges with confidence.

In this guide, we will explore various types of problems, offer strategies for tackling complex calculations, and discuss common approaches used by top performers. By the end, you will have the tools needed to navigate your studies and excel when faced with similar tasks.

Effective preparation involves not only understanding theoretical concepts but also honing your ability to apply them to real-world scenarios. Practical experience is key, and the more you practice, the more confident you will feel when addressing different types of problems in your assessments.

Financial Mathematics Exam Questions and Answers

This section is focused on helping you prepare for the most common challenges encountered in assessments related to applied finance. Understanding how to approach various types of problems and applying the right methods can significantly improve your chances of success. By reviewing essential concepts and practicing regularly, you’ll be better equipped to tackle the material effectively.

Common Problem Types

In this area, you will encounter several types of problems that are commonly found in assessments. These tasks generally involve applying formulas and techniques to solve real-life financial scenarios. Some of the most common categories include:

• Time value of money
• Loan amortization schedules
• Investment valuations
• Risk assessment calculations
• Interest rate analysis

Effective Approaches for Solving Problems

To improve your performance, it’s crucial to develop an efficient strategy for approaching each problem. Below are key strategies that can help you perform well:

1. Understand the problem before attempting to solve it.
2. Identify relevant formulas and decide which to use.
3. Break complex problems into smaller, manageable parts.
4. Double-check your results for accuracy.
5. Practice regularly to increase speed and confidence.

Understanding Key Financial Concepts for Exams

Grasping essential principles in the field of applied finance is fundamental for performing well in assessments. A solid understanding of the core concepts allows you to solve complex tasks with confidence and accuracy. Focus on mastering the basics, as they form the foundation for more advanced topics and ensure that you can handle a wide range of scenarios that may arise in tests.

Here are some of the key concepts you should focus on:

• Time value of money: The idea that money today is worth more than the same amount in the future due to its earning potential.
• Present and future value calculations: Understanding how to calculate the current value of future cash flows and vice versa.
• Interest rates: How rates affect investments, loans, and other financial instruments over time.
• Discounting and compounding: Techniques used to calculate the impact of interest over time on a principal amount.
• Risk assessment: Evaluating potential risks associated with investments or financial decisions.

Mastering these key concepts will help you approach different problems with the necessary tools and strategies. The more familiar you become with these ideas, the easier it will be to solve related tasks under pressure.

Common Topics in Financial Mathematics Exams

In assessments related to applied finance, several recurring themes are commonly tested. These topics form the core of many tasks and often require an in-depth understanding of mathematical techniques and their practical applications. A strong grasp of these subjects will help you confidently approach various problems and enhance your overall performance.

Key Areas to Focus On

Among the most frequently tested topics, the following are essential for mastering applied finance tasks:

• Time Value of Money: Understanding the principle that money today is more valuable than the same amount in the future due to its potential to earn.
• Loan Amortization: Calculating the breakdown of payments over the life of a loan, including principal and interest portions.
• Investment Valuation: Analyzing how to determine the value of an asset or investment based on future cash flows and market conditions.
• Risk Management: Evaluating potential risks in financial decision-making and the use of various models to minimize or mitigate these risks.
• Interest Rate Calculations: Determining how interest rates affect investment growth, loan payments, and other financial metrics over time.

Understanding the Fundamentals

Each of these topics is foundational in solving typical problems encountered in this field. Having a strong grasp of these concepts allows for efficient application during assessments, as well as in real-world financial decision-making.

How to Approach Exam Questions Effectively

To succeed in assessments, it is crucial to develop an effective approach to solving problems. Knowing how to break down complex tasks and apply the right techniques efficiently can save time and improve accuracy. A clear strategy will allow you to navigate challenges with confidence and ensure that you can handle all types of problems under time constraints.

Step-by-Step Problem Solving

When facing a new problem, take a moment to read through it carefully before diving into the calculations. Here’s a step-by-step approach to solving problems effectively:

• Read the problem thoroughly: Ensure you understand what is being asked and identify key information.
• Highlight relevant data: Focus on the numbers, variables, or conditions necessary for solving the task.
• Choose the appropriate method: Select the correct formula or technique based on the problem type.
• Break it into smaller parts: Tackle the problem step by step, addressing each element before moving to the next.
• Review your work: Double-check calculations and logic to confirm that your solution is accurate.

Managing Time Effectively

Time management is essential during an assessment. Prioritize easier problems first to build confidence and save time for more complex tasks. Ensure you allocate time for review at the end to check for any possible errors.

Important Formulas to Remember for Exams

In assessments related to applied finance, remembering key formulas is essential for solving problems quickly and accurately. These equations serve as tools for calculating various aspects of investments, loans, and other financial scenarios. Mastering the most commonly used formulas can greatly improve your performance and efficiency when tackling complex tasks.

Here are some of the essential formulas to keep in mind:

• Present Value (PV): PV = FV / (1 + r)^n – Used to calculate the current value of a future cash flow, where FV is the future value, r is the interest rate, and n is the number of periods.
• Future Value (FV): FV = PV * (1 + r)^n – Determines the future worth of an investment based on its present value, interest rate, and time period.
• Compound Interest: A = P(1 + r/n)^(nt) – Calculates the amount of money accumulated after interest is applied periodically, where A is the amount, P is the principal, r is the annual interest rate, n is the number of times interest is compounded, and t is the time in years.
• Loan Amortization: M = P * (r(1 + r)^n) / ((1 + r)^n – 1) – This formula is used to calculate the fixed monthly payment M for a loan with principal P, interest rate r, and number of periods n.
• Interest Rate Conversion: r = (1 + i/n)^n – 1 – Used for converting nominal interest rates to effective annual rates, where i is the nominal rate and n is the number of compounding periods per year.

By committing these formulas to memory, you will be well-equipped to solve problems efficiently and accurately under timed conditions.

Strategies for Solving Complex Problems

When faced with challenging tasks, having a structured approach is key to solving them efficiently. Complex problems often involve multiple steps, requiring careful analysis and the application of different techniques. By following proven strategies, you can simplify even the most intricate scenarios and reach solutions with confidence.

Breaking Down the Problem

The first step in tackling any difficult task is to break it down into smaller, more manageable parts. This process helps you focus on one aspect at a time, preventing confusion and reducing the chances of making mistakes. Follow these steps to approach problems systematically:

• Identify key elements: Highlight the critical information and data points in the problem.
• Define what needs to be calculated: Determine the exact outcome you are trying to achieve.
• Organize the data: Arrange the information in a clear, logical order to make the next steps more straightforward.

Applying the Right Techniques

Once the problem is broken down, it’s time to apply the appropriate methods. The key is to match the task at hand with the right technique or formula. Here are a few approaches to consider:

• Use known formulas: Identify if there are any established equations that directly apply to the problem.
• Work step by step: Solve the problem gradually, checking your work after each step to ensure accuracy.
• Use estimation techniques: When dealing with large numbers or complex calculations, estimation can simplify the process and save time.

By breaking down the problem and applying the correct techniques, you will increase both your speed and accuracy, helping you tackle difficult challenges more effectively.

Time Management Tips During the Exam

Effective time management is crucial for success when tackling tasks under time constraints. With multiple problems to solve, it’s important to allocate your time wisely to ensure you can complete all sections and still have time for review. By staying organized and maintaining a steady pace, you can increase your chances of achieving the best results.

Here are some strategies to help you manage your time effectively during an assessment:

Strategy	Tip
Prioritize Tasks	Start with easier problems to build confidence and gain momentum, leaving more challenging tasks for later.
Set Time Limits	Allocate specific amounts of time for each section and stick to it. Don’t get stuck on one problem for too long.
Use a Timer	Keep track of time using a clock or timer. This will help you stay on schedule and avoid rushing at the end.
Leave Room for Review	Set aside a few minutes at the end to go over your answers. Re-check for mistakes or missed details.
Don’t Overthink	If you’re unsure about a solution, move on and come back to it later. Don’t waste time on one issue.

By implementing these time management tips, you can maximize your performance and approach the assessment with confidence, ensuring you tackle each section efficiently and effectively.

Practice Questions to Boost Your Confidence

Consistent practice is one of the best ways to strengthen your problem-solving skills and build confidence. By working through various examples, you can familiarize yourself with different problem types and improve your ability to handle them under pressure. The more you practice, the more comfortable and prepared you will feel when faced with challenges.

Here are some practice problems that can help you get started and enhance your problem-solving ability:

Problem Type	Practice Example
Time Value of Money	Calculate the present value of $1,000 due in 5 years with a 6% annual interest rate.
Loan Amortization	Find the monthly payment for a $10,000 loan with an annual interest rate of 5% over 10 years.
Investment Growth	Determine the future value of an investment of $500 after 3 years, with an annual return rate of 8% compounded yearly.
Interest Rate Calculation	What is the effective annual rate (EAR) for a nominal rate of 6% compounded quarterly?
Risk and Return	Calculate the expected return of a portfolio with two assets, one having a return of 7% and the other 12%, with weights of 40% and 60%, respectively.

By regularly practicing problems like these, you can not only reinforce your understanding but also gain the confidence to approach more complex tasks with ease. Make sure to time yourself to simulate real conditions and improve your speed as well.

Analyzing Past Papers for Trends

Reviewing previous assessments is a powerful strategy to understand the most commonly tested topics and patterns. By identifying trends in past tasks, you can predict the types of problems likely to appear in future challenges. This analysis helps you focus your preparation on areas that carry the most weight, ultimately improving your efficiency and confidence.

Key Areas to Focus On

When analyzing past tasks, it’s important to look for recurring themes or concepts that frequently appear. These areas are often essential to mastering the subject and are typically given more emphasis. Pay attention to:

• Recurrent problem types: Identify the types of problems that come up regularly, such as those involving calculations, concepts, or specific techniques.
• Common formulas: Take note of formulas that consistently appear in different contexts. Familiarity with these will save time during preparation.
• Specific topics: Some topics may be tested more often than others. Prioritize studying these areas for better results.

Using Past Papers to Improve Performance

Once you’ve identified the trends, use them to guide your study plan. Practicing with past tasks will help you familiarize yourself with the question format and the level of difficulty you can expect. Additionally, solving past papers under timed conditions can improve your time management skills and help you develop a more strategic approach.

Topic	Frequency in Past Papers
Time Value of Money	Frequently tested (appears in almost every past paper)
Interest Calculations	Common (appears at least once in each assessment)
Risk and Return	Occasionally tested (appears every second or third paper)
Investment Valuation	Rarely tested (appears in about one out of five papers)

By consistently practicing with past tasks and focusing on the trends, you can enhance your preparation and approach the next assessment with confidence and skill.

Common Mistakes to Avoid in Financial Exams

While preparing for assessments, it’s easy to overlook certain details that can significantly impact your performance. Many individuals make similar errors under pressure, which can be avoided with careful attention to detail and better time management. Recognizing and addressing these common pitfalls can lead to a more successful outcome.

Key Mistakes to Watch Out For

Here are some common mistakes that many students make during evaluations:

• Skipping the Instructions: Failing to read the guidelines carefully can lead to misunderstanding what is being asked, resulting in incomplete or irrelevant responses.
• Not Managing Time Properly: Spending too much time on a single problem can leave you with insufficient time to finish others. It’s crucial to allocate time effectively for each section.
• Forgetting to Show Work: In many cases, it’s important to demonstrate the steps taken to reach a solution. Not showing your work can result in losing marks, even if the final answer is correct.
• Overcomplicating the Problem: Sometimes, simpler solutions are the best. Overthinking a problem can lead to unnecessary errors and confusion.
• Not Checking for Errors: Rushing through tasks without reviewing your work often leads to careless mistakes. Always leave time to double-check your answers for accuracy.

How to Avoid These Pitfalls

To prevent these errors, practice effective planning and problem-solving techniques:

• Carefully read each question: Understand what is being asked before attempting the solution. Break down the problem into smaller steps to avoid confusion.
• Follow a structured approach: Create a clear strategy for tackling each task, and stick to it. Don’t let difficult problems derail your progress.
• Review your work: Always check your calculations and reasoning. This extra step can help you catch errors before submission.

By avoiding these common mistakes, you can increase your chances of achieving the desired results and improve your overall performance.

How to Interpret Financial Data Accurately

Accurate interpretation of numerical data is crucial when making informed decisions. Whether it’s understanding trends, identifying patterns, or drawing conclusions, interpreting data with precision ensures that you are making the right calls based on solid evidence. Without a clear understanding of how to read and analyze the figures, you may misinterpret key insights and arrive at incorrect conclusions.

Steps to Properly Analyze Data

To effectively interpret numerical information, follow these essential steps:

• Understand the Context: Before analyzing any data, ensure that you comprehend the context in which it is presented. Consider factors such as time periods, industry standards, and any variables that could influence the data.
• Identify Key Metrics: Focus on the most relevant figures that directly impact the analysis. For example, identifying growth rates, margins, or ratios helps in understanding the performance or health of a subject.
• Look for Patterns and Trends: Data often reveals important patterns over time. Look for recurring trends or anomalies that can provide insights into the subject’s behavior or performance.
• Compare with Benchmarks: Whenever possible, compare the data with benchmarks, averages, or past results. This will help you assess whether the current data is within a normal range or deviates from expected values.
• Consider External Factors: External factors, such as market conditions, regulations, or seasonal effects, can impact data interpretation. Ensure that you account for these factors when drawing conclusions.

Common Mistakes to Avoid

When analyzing data, it’s easy to make assumptions that may lead to incorrect interpretations. Avoid these common mistakes:

• Ignoring Outliers: Outliers can have a significant impact on your analysis. Always investigate unusual data points to determine if they represent genuine trends or errors.
• Overlooking the Big Picture: Focusing too much on one piece of data can lead to tunnel vision. Make sure to look at the overall context and compare all relevant factors to form a balanced view.
• Relying Too Much on Historical Data: While historical data is important, it may not always predict future performance. Always consider current market trends or other influencing factors.

By following these guidelines and avoiding common errors, you can interpret data more accurately, leading to better decision-making and clearer conclusions.

The Role of Interest Rates in Calculations

Interest rates play a pivotal role in a variety of numerical evaluations, particularly when it comes to determining the cost of borrowing, returns on investments, and assessing future value over time. They act as a percentage applied to an amount of money, influencing how funds accumulate or increase in value. Whether calculating loan repayments or evaluating investment potential, understanding the impact of interest rates is crucial for accurate projections and informed decision-making.

Understanding Interest Rate Impacts

Interest rates can affect a range of financial models and calculations, and they are often expressed as annual percentages. Here’s how interest rates influence key financial operations:

• Loan Repayments: For loans, interest rates determine the total cost of borrowing. Higher rates increase the amount to be repaid over the loan’s lifetime, while lower rates reduce the financial burden.
• Investment Growth: In investments, interest rates dictate how much a sum of money will grow over time. Compounding interest, where interest is calculated on both the initial principal and the accumulated interest, accelerates this growth.
• Discounting Future Cash Flows: Interest rates are used to calculate the present value of future cash flows. This is essential when evaluating projects or investments that will generate revenue or costs at different points in time.

Key Formulas Involving Interest Rates

Several essential formulas rely on interest rates to determine values. Understanding these formulas can help in performing accurate calculations:

• Simple Interest: The formula for simple interest is: Interest = Principal × Rate × Time. This formula is used when interest is calculated only on the principal amount.
• Compound Interest: The formula for compound interest is: A = P (1 + r/n)^(nt), where P is the principal, r is the annual interest rate, n is the number of times the interest is compounded per year, and t is the number of years.
• Present Value of Annuities: The present value of an annuity formula uses the interest rate to calculate the current value of a series of future payments: PVA = PMT × [(1 – (1 + r)^-n) / r].

By incorporating interest rates into financial calculations, individuals can make more informed decisions and better evaluate potential returns or costs associated with different financial scenarios.

Types of Problems You Will Encounter

During your preparation or practice, you’ll face a wide variety of challenges that test your ability to apply various techniques and concepts. These problems are designed to evaluate your understanding and your capacity to solve real-world scenarios by using the appropriate formulas and methods. Below are the common types of problems you will likely come across in such evaluations.

Common Problem Categories

Each category tests different skills and focuses on distinct aspects of applied calculations. Familiarizing yourself with these types will enhance your ability to solve them efficiently:

• Time Value of Money: Problems that deal with how money changes over time, considering interest rates and the impact of inflation. These can include future value, present value, annuities, and loan amortizations.
• Investment Valuation: These problems typically involve calculating the worth of investments based on cash flows, discount rates, and risk assessments. Examples include bond pricing and stock valuation.
• Risk and Return Calculations: These questions focus on assessing the relationship between risk and the potential returns of various financial instruments, often requiring the use of formulas like standard deviation and Sharpe ratio.
• Loan Amortization: You will often encounter questions that require you to calculate the repayment schedule of loans, including the principal and interest payments over time, using amortization tables.
• Discounting and Compounding: Questions in this category ask you to calculate the effects of discounting future cash flows to present value or calculating compound interest over time, including continuous compounding problems.

Problem-Solving Techniques

While the types of problems may vary, the approach to solving them often remains the same. Here are a few general techniques that will help you navigate through these problems:

• Break Down the Problem: Start by identifying the known variables and the unknowns. Determine which formula is appropriate for the situation.
• Draw Diagrams or Use Tables: For problems like amortization schedules or cash flow analysis, creating a visual representation can help organize your approach and ensure accuracy.
• Double-Check Units: Always ensure that your units are consistent, especially when working with rates, periods, and amounts.

By practicing with these types of problems, you’ll strengthen your understanding and develop the skills needed to tackle even the most complex scenarios effectively.

Working with Present and Future Values

Understanding the relationship between present and future values is crucial when solving problems that involve time and money. These concepts are essential for evaluating how the value of money changes over time due to factors such as interest rates or inflation. Whether you’re looking to calculate how much an investment will be worth in the future or determining the present worth of a future cash flow, mastering these calculations is key to effective financial analysis.

Present value (PV) represents the current worth of a sum of money that you will receive or pay in the future, discounted at a specific interest rate. On the other hand, future value (FV) indicates what a sum of money today will be worth at a given point in the future when interest is applied over time. Both values are interconnected and serve as fundamental tools for a wide range of calculations, including investment evaluation, loan assessments, and savings growth.

Calculating Present Value

To calculate present value, you need to discount future cash flows back to their value in today’s terms. The formula for PV is:

• PV = FV / (1 + r)^n

Where:

• PV: Present value
• FV: Future value
• r: Interest rate per period
• n: Number of periods

For example, if you are expecting to receive $1,000 in 5 years, and the annual interest rate is 5%, the present value of that future amount would be calculated by discounting it over the 5 years at 5% interest.

Calculating Future Value

Future value calculations are used to determine how much a sum of money today will be worth in the future. The formula for FV is:

• FV = PV * (1 + r)^n

Where:

• FV: Future value
• PV: Present value
• r: Interest rate per period
• n: Number of periods

For instance, if you invest $1,000 today in an account that earns 5% annually, in 5 years, the future value of that investment would be determined using the formula above.

Both present and future value calculations are fundamental in making informed financial decisions, whether it’s for personal savings, investments, or evaluating the cost of future obligations. By practicing these concepts, you’ll develop the skills necessary to evaluate financial opportunities effectively and make strategic decisions regarding money management.

Advanced Topics to Prepare For

As you progress in your studies, you will encounter more complex concepts that require a deeper understanding of how different variables interact in various financial scenarios. These advanced topics are designed to challenge your ability to analyze situations with multiple factors at play and will often require you to apply a range of skills learned from foundational principles. By preparing for these advanced areas, you can strengthen your problem-solving abilities and enhance your confidence when dealing with more intricate tasks.

Advanced topics often involve multiple stages of calculation, as well as the application of sophisticated models and techniques. These subjects require a clear grasp of underlying concepts and a strong aptitude for critical thinking. While they may seem challenging at first, with focused practice, you will be able to tackle them effectively.

Here are some key advanced topics to focus on:

• Risk Management: Understanding how to assess and manage the uncertainty that comes with various financial transactions and investments.
• Options Pricing: Learning to evaluate the value of financial derivatives like options and futures using models such as the Black-Scholes model.
• Portfolio Theory: Analyzing how to construct an investment portfolio that maximizes returns for a given level of risk.
• Capital Budgeting: Understanding methods such as Net Present Value (NPV) and Internal Rate of Return (IRR) to evaluate long-term investment projects.
• Arbitrage and Market Efficiency: Learning how to identify arbitrage opportunities and understanding the principles of efficient markets.

Mastering these advanced topics will not only help you perform better in assessments but will also prepare you for real-world financial decision-making. As you prepare, it’s important to regularly practice applying these concepts through case studies and problem-solving exercises, ensuring that you’re well-equipped to handle a wide range of complex situations.

How to Review Your Exam Answers Efficiently

Reviewing your responses after completing an assessment is a crucial step that can significantly impact your final result. It’s not just about checking if you’ve answered every part of the task, but also about ensuring that your reasoning is clear and that all necessary steps have been followed accurately. Efficiently reviewing your work requires a systematic approach, focusing on key areas where errors are most likely to occur and ensuring that your solutions are both complete and precise.

The first step in an effective review is managing your time well. Allocate a set amount of time to go over your work, ensuring that you don’t rush through the process. Start by double-checking your calculations and ensuring that no steps are missing. Pay close attention to common areas where mistakes tend to occur, such as signs, units, and rounding errors. If you can, try to approach each problem from a different angle to confirm that your approach was correct.

Key Areas to Focus on During Review

• Check for Consistency: Ensure that your answers align with the given conditions and assumptions. Inconsistent results can indicate mistakes made during the calculation or interpretation stages.
• Verify Key Formulas: Double-check that you have used the correct formulas and applied them properly. Small mistakes in formulas can lead to large errors in final answers.
• Reevaluate Logical Flow: Ensure that your reasoning is clear and that you’ve addressed every part of the problem in a structured manner. Check that your logic holds throughout.
• Review Units and Conversions: Always verify that your units are consistent and that you’ve properly converted where needed. Misinterpreting units can cause significant errors in your results.

Final Steps for Efficient Review

• Take a Break: If time allows, take a short break before reviewing your work. A fresh mind can help you spot mistakes you may have missed earlier.
• Check Your Work in Reverse: Work backwards from your final answer to verify that each step is correct. This can be an effective way to spot any errors in your process.
• Ask Yourself Key Questions: Have you answered all parts of the task? Does your answer make sense? Is there a simpler way to approach the problem?

By following these steps, you can ensure that you’ve reviewed your work thoroughly and that your solutions are as accurate as possible. With practice, you will become more efficient at identifying potential mistakes and making necessary adjustments, which will improve both your confidence and your overall performance.