
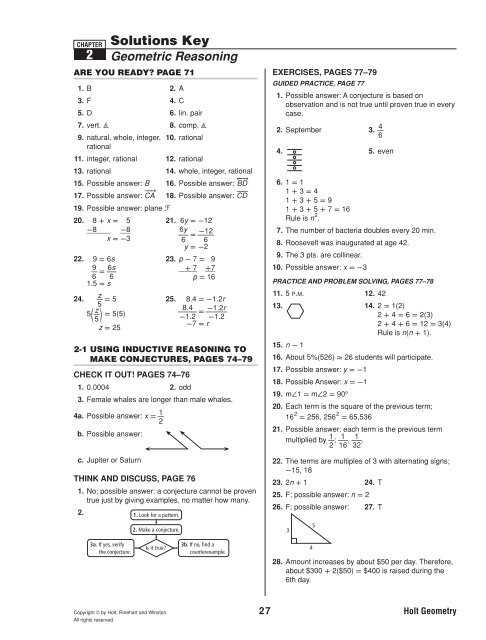
Understanding and solving mathematical challenges is essential for building a strong foundation in various fields of study. These exercises help improve problem-solving skills and the ability to think critically. Tackling such tasks requires both focus and the application of core principles to arrive at accurate results.
Effective learning comes from repeated exposure to different types of problems, along with strategic thinking that makes difficult topics more approachable. It’s important to break down each problem into manageable parts, identifying patterns and applying techniques that enhance understanding.
By reviewing problems systematically and using practical examples, learners can sharpen their skills and gain confidence. Practicing regularly allows students to see improvements in their speed and accuracy, preparing them for more advanced challenges ahead.
Lesson 2.6 Geometry Practice Overview
This section is designed to guide you through essential exercises aimed at enhancing your understanding of key mathematical concepts. The goal is to provide a comprehensive approach to solving problems that require logical thinking, spatial reasoning, and application of specific formulas. By working through these tasks, you will gain the skills needed to tackle more complex challenges with confidence.
Key Topics Covered
The exercises focus on a variety of important topics that are foundational for mastering advanced mathematical reasoning. These include identifying relationships between shapes, calculating measurements, and solving problems involving proportions and symmetry. By exploring these areas, you will develop a solid understanding of the core principles that underlie many mathematical applications.
Effective Problem-Solving Strategies
To excel in solving these problems, it’s crucial to approach each one with a clear strategy. Breaking down complex tasks into smaller, manageable steps allows for better comprehension and accuracy. By focusing on understanding the underlying concepts and practicing regularly, you’ll improve your ability to solve similar challenges in the future with greater ease.
Understanding Geometry Practice Concepts
Grasping the fundamental ideas behind solving mathematical problems is crucial for building a strong foundation in this area. This section aims to explain the core concepts that will guide you through various challenges. These concepts form the basis for effective problem-solving and allow for a deeper understanding of spatial relationships and numerical reasoning.
Core Concepts to Focus On
When approaching these tasks, it’s important to focus on several key principles that will aid in solving problems efficiently. These include:
- Identifying patterns in shapes and figures
- Applying measurement formulas accurately
- Understanding the relationships between angles, sides, and other elements
- Using logical reasoning to simplify complex scenarios
Practical Application of Concepts
Once these ideas are understood, applying them to real-world problems becomes much easier. You can enhance your skills by regularly practicing with different types of problems. The more you apply these concepts, the more intuitive the process becomes. Key techniques to focus on include:
- Breaking down problems into smaller steps for clarity
- Drawing diagrams to visualize relationships
- Checking calculations and logic for accuracy
Step-by-Step Solutions for Lesson 2.6
This section provides detailed solutions to help you understand how to approach and solve each problem methodically. By following a structured approach, you can ensure clarity in your reasoning and accuracy in your calculations. Breaking down the steps will help reinforce key concepts and build confidence in solving similar challenges.
| Step | Explanation | Result |
|---|---|---|
| 1 | Read the problem carefully and identify key information. | Key values and variables identified. |
| 2 | Determine the appropriate formula or method to use. | Formula selected based on the problem’s requirements. |
| 3 | Substitute known values into the equation. | Values placed into the formula accurately. |
| 4 | Simplify the equation step by step. | Equation simplified to the final form. |
| 5 | Check the result for accuracy and reasonableness. | Final answer verified. |
By following these steps, you will be able to approach similar problems with confidence and solve them efficiently. Each solution reinforces a methodical way of thinking that can be applied to a variety of problems within this topic.
Key Strategies for Solving Geometry Problems
When tackling mathematical challenges that involve shapes, measurements, and spatial reasoning, having a set of proven strategies can greatly improve your efficiency and accuracy. By following systematic methods, you can simplify complex problems and ensure a logical progression toward the correct solution. The following strategies are essential for mastering these types of tasks.
Effective Approaches to Problem Solving
To solve problems effectively, it is crucial to follow a structured approach:
- Understand the problem: Read the problem carefully, identifying the given information and what is being asked.
- Visualize the situation: Drawing a diagram can help clarify relationships between elements and make the problem more manageable.
- Break down the steps: Divide the task into smaller, more manageable steps to avoid confusion and ensure accuracy.
- Use relevant formulas: Apply the appropriate mathematical formulas based on the information you have.
- Double-check your work: Review each step to ensure calculations are correct and logical connections are made.
Tips for Overcoming Challenges
Sometimes, problems may seem overwhelming. Use these additional tips to make the process easier:
- Look for patterns or symmetries that can simplify the task.
- Consider using estimation when appropriate to check the reasonableness of your results.
- Review similar problems to understand how specific methods or techniques were applied.
- Don’t hesitate to break the problem into even smaller parts if it feels complex.
By applying these strategies consistently, you will improve your problem-solving skills and increase your confidence in tackling similar tasks in the future.
Common Mistakes in Geometry Practice
While working through mathematical problems involving shapes, measurements, and spatial reasoning, it’s easy to make errors that can lead to incorrect solutions. Identifying and understanding these common mistakes is key to improving your problem-solving skills. By recognizing where errors tend to occur, you can avoid them and approach tasks with greater accuracy.
Some of the most frequent mistakes include:
- Misinterpreting the problem: Not fully understanding the question or overlooking key information can result in using incorrect methods or formulas.
- Incorrect application of formulas: Using the wrong formula or applying it incorrectly is a common error that can lead to incorrect results.
- Rushed calculations: Making mistakes during arithmetic or algebraic manipulations is often due to haste, which can lead to inaccurate results.
- Overlooking units of measurement: Forgetting to include or convert units appropriately can distort the answer and cause confusion in the solution.
- Neglecting to check work: Not reviewing the solution to ensure each step makes sense is a mistake that can go unnoticed until later stages.
Avoiding these pitfalls requires attention to detail, a clear understanding of the problem, and careful execution of each step. Developing a habit of reviewing your work and taking time to understand the concepts behind the problems will help prevent these common mistakes.
How to Approach Complex Geometry Questions
Tackling intricate problems involving shapes, angles, and spatial relationships can be challenging, but with the right approach, you can break them down into manageable parts. By carefully analyzing each aspect of the problem and applying systematic methods, you can approach even the most complex questions with confidence. Understanding key strategies is essential to navigating through complicated scenarios effectively.
Key Steps for Breaking Down Complex Problems
To simplify complicated questions, follow these essential steps:
| Step | Action | Purpose |
|---|---|---|
| 1 | Identify the given information and unknowns. | Clarify what you know and what needs to be determined. |
| 2 | Draw a diagram or visual representation. | Visualize the situation to better understand the relationships between elements. |
| 3 | Break the problem into smaller, solvable parts. | Focus on one aspect at a time to simplify the process. |
| 4 | Choose and apply relevant formulas. | Use appropriate equations based on the given information. |
| 5 | Check the results for consistency and reasonableness. | Ensure the solution fits logically within the context of the problem. |
Additional Tips for Success
While following the above steps, consider the following tips for more effective problem-solving:
- Work systematically: Tackle one part of the problem at a time to avoid feeling overwhelmed.
- Look for patterns: Identifying recurring relationships or symmetries can simplify the process.
- Stay organized: Keep track of all values and intermediate steps to avoid confusion.
- Practice regularly: The more problems you solve, the easier it becomes to recognize common strategies.
By applying these strategies, you can approach even the most challenging questions with clarity and confidence.
Tips for Improving Geometry Problem-Solving Skills
Developing strong problem-solving skills in mathematical scenarios involving shapes, measurements, and spatial relationships requires consistent practice and strategic thinking. By honing key techniques and staying disciplined in your approach, you can significantly improve your ability to tackle complex tasks. Applying a structured method helps in breaking down problems and finding the most efficient solutions.
Effective Strategies for Success
Here are some strategies to enhance your problem-solving skills:
- Understand the concepts: Instead of memorizing formulas, focus on fully understanding the principles behind them. This will help you apply them more effectively in different contexts.
- Visualize the problem: Draw diagrams or use models to represent the situation. This makes abstract concepts more tangible and easier to work with.
- Break down complex problems: Divide larger problems into smaller, more manageable parts. Solve each part step by step to avoid feeling overwhelmed.
- Practice regularly: The more problems you solve, the better you’ll become at recognizing patterns and applying strategies efficiently.
Maintaining Focus and Consistency
Consistency is key when improving your skills. Here are some additional tips to stay on track:
- Work in a structured manner: Follow a logical order when solving problems, such as first identifying known values, then selecting the right methods, and finally reviewing your work.
- Learn from mistakes: Don’t be discouraged by errors. Analyze where you went wrong and understand why the mistake happened. This will prevent future missteps.
- Stay patient: Some problems take time to solve, and it’s important to maintain patience. Taking your time can lead to a more thorough and accurate solution.
By incorporating these strategies into your study routine, you will improve your problem-solving skills and be better equipped to handle challenging scenarios with ease.
Mastering Geometry Formulas and Theorems

Achieving mastery in mathematical concepts related to shapes, sizes, and spatial relationships relies heavily on a deep understanding of key formulas and theorems. These essential principles serve as tools for solving a wide variety of problems efficiently. When you are familiar with the core rules that govern these concepts, you can approach problems with confidence and precision.
Mastering these formulas requires not only memorization but also understanding how and when to apply them. By developing a strong foundation in the fundamental relationships between angles, areas, and volumes, you can build a solid problem-solving strategy that helps you navigate more complex scenarios.
To truly excel, it’s important to regularly review and practice these formulas in different contexts. This will enhance your ability to recognize patterns and apply the appropriate rules under various conditions.
Analyzing Problem Types
Understanding the different types of problems encountered in mathematical exercises is essential for efficient problem-solving. By identifying the specific nature of each question, you can apply the right approach and utilize appropriate techniques to arrive at a solution. Whether dealing with basic principles or more intricate scenarios, recognizing the structure of the problem is the first step toward success.
Problems often vary in complexity and require different strategies to solve. Some may focus on simple calculations, while others might test your ability to apply theoretical concepts in real-world contexts. The key is to break down each problem into manageable steps and address them systematically.
Basic Calculation-Based Problems
These problems typically involve straightforward numerical operations, such as calculating areas, angles, or distances. They are usually well-defined and require a clear understanding of foundational formulas. Once you are familiar with these, solving them becomes a matter of applying the correct formula in a structured manner.
Conceptual and Theoretical Problems
These types of questions focus on the application of deeper principles and theorems. They may ask for explanations or require you to solve problems using multiple steps that involve abstract reasoning. Mastery of key concepts is essential for successfully navigating these challenges.
Answer Check: Self-Evaluation Tips
Reviewing your work is a crucial step in ensuring the accuracy of your solutions. Self-evaluation helps identify any mistakes, correct them, and reinforce the understanding of concepts. By systematically reviewing your approach and results, you can develop a more confident problem-solving skillset and avoid repeating errors in the future.
While solving complex problems, it’s easy to overlook small mistakes. This is why double-checking each step is vital. Whether it’s recalculating values, reviewing your reasoning, or checking for consistency, a thorough evaluation process can significantly improve your outcomes.
Recheck Your Work Step by Step
Start by revisiting each part of the solution. Verify that every calculation was performed correctly and that each step logically follows from the previous one. Look for signs of mistakes such as incorrect signs, misapplied formulas, or skipped steps.
Compare Results with Different Approaches
If possible, try solving the problem using an alternative method. Comparing the results from different approaches can help confirm the validity of your solution. If the outcomes match, you can be more confident in the accuracy of your work.
Tip: Take your time during the review process. Rushed evaluations often miss critical errors that can affect the overall outcome.
Visual Aids in Solving Exercises
Using visual tools can significantly enhance problem-solving by providing a clearer understanding of the task at hand. These aids help break down complex concepts, making them more accessible and easier to grasp. By representing problems visually, you can better understand relationships, patterns, and geometrical properties involved in the exercise.
Diagrams, charts, and graphs are some of the most effective visual tools. They allow you to see the shapes, angles, and lines directly, making it easier to apply the appropriate formulas or strategies. Visual aids also reduce the chances of making errors by providing a concrete reference point.
Common Visual Tools

- Diagrams: Illustrating the given problem using shapes, lines, and angles helps to visualize relationships.
- Graphs: Plotting data on coordinate planes can clarify the positions of points or the relationships between them.
- Tables: Organizing information in tabular form aids in comparing variables and tracking key values.
- Color Coding: Highlighting different parts of a problem with distinct colors can help distinguish between different components of the figure.
Why Visual Tools Matter
- Improved Understanding: They provide a more intuitive grasp of abstract concepts, making them easier to understand and manipulate.
- Reduced Mistakes: A clear diagram minimizes the chances of overlooking important details or making miscalculations.
- Increased Efficiency: With visual tools, you can quickly identify the correct approach and focus your efforts on solving the problem.
Tip: Always take time to carefully draw out the problem before jumping into calculations. A clear visual foundation can simplify even the most challenging tasks.
Time Management for Problem-Solving Exercises

Effectively managing time is essential when working through challenging exercises. By organizing your approach, you can maximize productivity and reduce stress. A structured plan ensures that each step is completed thoroughly without rushing or overlooking important aspects.
Proper time management helps you stay focused, break down complex tasks, and gives ample time to review and adjust your work. It’s crucial to balance speed with accuracy, ensuring that you can solve problems efficiently without compromising on the quality of your solutions.
Strategies for Efficient Time Management
- Set Clear Goals: Break down your tasks into smaller, manageable parts. Focus on one section at a time to avoid feeling overwhelmed.
- Prioritize Difficult Problems: Start with more complex problems when your mind is fresh. This helps prevent frustration later when you’re feeling fatigued.
- Allocate Time for Each Problem: Assign a specific amount of time to work on each task. This keeps you on track and prevents spending too much time on any one problem.
- Take Breaks: Short breaks help refresh your mind and improve focus, especially during lengthy sessions.
- Review and Revise: Always leave time at the end to go back and check your work. This will help you spot any mistakes and refine your solutions.
Tools to Help Manage Time
- Timers: Use a timer to keep track of your time for each problem or section. This helps maintain momentum.
- Checklists: Organize your tasks and mark them off as you go. It provides a visual sense of progress and keeps you motivated.
- Study Schedule: Plan dedicated time for exercises each day. Consistency is key to improving efficiency over time.
By applying these time management techniques, you can not only solve exercises effectively but also build a habit of working efficiently under time constraints. This approach will help you become more confident in your problem-solving abilities and achieve better results in less time.
How to Review Problem-Solving Results

Reviewing your solutions after completing exercises is an essential step in understanding your approach and improving your skills. This process helps identify any mistakes, gaps in reasoning, or areas where your method could be more efficient. By taking time to assess your work, you can solidify your knowledge and avoid repeating errors in the future.
The review process involves a thorough examination of both the final solutions and the steps you took to reach them. Instead of simply confirming whether your answers are correct, focus on understanding why a certain approach worked or didn’t, and how you can adjust your strategy next time.
Steps to Effectively Review Your Work
- Recheck Each Step: Go through each calculation and reasoning step carefully. Verify that no steps were skipped or done hastily.
- Understand the Process: Reflect on the method used to solve each problem. Ask yourself if there’s a more efficient way to reach the same conclusion.
- Identify Patterns: Recognize recurring strategies or types of problems that seem challenging. Knowing your strengths and weaknesses allows you to focus on areas that need improvement.
- Compare with Solutions: If available, compare your work with reference solutions. This comparison will highlight any differences in approach and help you refine your methods.
Tips for Improving Review Sessions
- Break Down Complex Problems: For difficult problems, break them down into smaller parts to make sure every aspect is fully understood.
- Seek Feedback: If you’re unsure about a solution, ask a peer or mentor for feedback. They might offer insights that you missed.
- Keep a Review Log: Maintain a log of common mistakes or areas where you struggled. Over time, this log will help you recognize patterns in your mistakes and help you avoid them in future tasks.
By consistently reviewing your work with these strategies, you’ll be able to pinpoint areas of improvement, refine your approach, and enhance your problem-solving abilities for future challenges.
Preparing for Assessments
Proper preparation is key to performing well on any evaluation. It involves more than simply reviewing content; it’s about understanding concepts, practicing methods, and ensuring you are ready for a variety of problem types. By following a structured approach, you can build confidence and improve your ability to tackle questions efficiently and accurately.
Start by identifying the main topics and skills that will be assessed. Break these down into manageable parts, and focus on the areas where you feel least confident. Reviewing your past work and practicing different problem types will help reinforce your understanding and increase your chances of success.
Effective Preparation Strategies
- Review Key Concepts: Focus on the fundamental principles that form the basis of the subject. Understanding the core concepts allows you to approach any problem with confidence.
- Practice Regularly: Consistent practice is essential. Solve a variety of problems to familiarize yourself with different scenarios and reinforce your problem-solving skills.
- Test Yourself: Take practice exams or quizzes under timed conditions. This will help you manage time during the actual assessment and give you a feel for the types of questions that may appear.
- Review Mistakes: After practicing, go over your mistakes carefully. Understanding why you made an error and how to avoid it next time is crucial for improvement.
Time Management Tips
- Set a Study Schedule: Allocate specific times each day for preparation. This will help you stay organized and ensure you cover all necessary topics.
- Prioritize Difficult Areas: Spend more time on topics that are more challenging to you, but don’t neglect areas you are already comfortable with.
- Take Breaks: Don’t overwork yourself. Short, regular breaks will help maintain focus and prevent burnout.
By following these strategies and preparing systematically, you’ll feel ready to approach your assessment with confidence and perform at your best.
Improving Understanding with Practice
Mastering any subject requires consistent effort and dedication. By engaging in regular exercises and tackling a variety of problems, you reinforce your understanding and build a deeper connection with the material. This process not only enhances retention but also improves the ability to apply knowledge to new and unfamiliar situations. Through continual practice, complex concepts become more intuitive, and problem-solving skills become sharper.
To make the most of your study sessions, focus on a balanced approach. It’s important to challenge yourself with increasingly difficult problems, but also to revisit simpler tasks to ensure the basics remain solid. Over time, this will help you gain confidence and develop a deeper understanding of the key principles that drive the subject.
Additionally, reviewing mistakes after each exercise is crucial. Analyzing errors not only helps you avoid repeating them but also offers valuable insights into areas that may need further attention. Identifying patterns in your mistakes allows you to target specific weaknesses and focus your practice on the areas that will yield the most improvement.
Answering Word Problems Effectively
Succeeding in word problems requires a systematic approach that helps you break down the information and find the best way to apply your knowledge. The key is to understand the problem, identify the given details, and figure out what is being asked. By following a logical progression, you can simplify the problem and ensure that you approach it with clarity.
Step-by-Step Approach
Start by reading the problem carefully. Highlight or underline important information, such as numerical values and key relationships. Next, translate the problem into mathematical terms. Draw diagrams or shapes if necessary, as visualizing the problem can make it easier to understand. Once you have a clear picture of the situation, identify the appropriate formulas or methods to apply.
Double-Check Your Work
After solving the problem, always take a moment to review your solution. Check your calculations, make sure all units match, and verify that your answer makes sense in the context of the problem. If anything seems off, retrace your steps and try to identify where you may have made an error. A careful review ensures accuracy and reinforces your understanding.
Using Resources to Master Geometry Practice

Utilizing various tools and materials can significantly enhance your ability to solve complex problems and master new concepts. Whether you’re studying independently or preparing for assessments, having access to high-quality resources will help reinforce understanding and sharpen your skills. From textbooks to online tutorials, diverse learning aids provide valuable support during your educational journey.
Types of Resources
Different types of resources can aid in mastering mathematical challenges:
- Textbooks and Guides: These contain structured lessons, examples, and exercises designed to build foundational knowledge.
- Online Platforms: Websites and apps often provide interactive problems, video tutorials, and step-by-step solutions.
- Study Groups: Working with peers can offer fresh perspectives and enhance collaborative problem-solving skills.
- Tutors and Teachers: Personalized assistance helps clarify difficult topics and address specific areas of confusion.
How to Use Resources Effectively
To maximize the benefits of these resources, create a balanced study routine that incorporates both theory and practice. Use textbooks to understand the principles and theories, and leverage online tools for hands-on problem solving. Additionally, seek feedback from others and focus on areas where you need improvement.
| Resource Type | Benefits | When to Use |
|---|---|---|
| Textbooks | Clear explanations, structured examples | For initial learning and understanding concepts |
| Online Platforms | Interactive exercises, immediate feedback | To practice and reinforce learned concepts |
| Study Groups | Collaborative learning, diverse insights | When tackling challenging problems or preparing for assessments |
| Tutors | Personalized guidance, targeted help | When needing detailed explanations for specific topics |