Enhancing your ability to tackle challenging numerical tasks is essential for achieving strong results in various academic evaluations. Building a foundation of logical reasoning and analytical thinking ensures you can approach even the most complex scenarios with confidence.
In this guide, you will find detailed solutions and explanations that help clarify common challenges encountered during assessments. Each example is designed to improve your understanding and enable you to apply learned concepts effectively.
By practicing regularly and reviewing well-structured examples, you can strengthen your knowledge, improve your efficiency, and prepare yourself for success in any quantitative examination.
How to Ace ACT Math Test
Mastering numerical reasoning requires a blend of strategic preparation and consistent effort. Developing a systematic approach to problem-solving can significantly enhance your performance in quantitative evaluations.
Start by identifying the core topics that frequently appear in such assessments. Focus on understanding the principles behind each concept rather than memorizing steps. This deeper comprehension will enable you to adapt to different question formats effectively.
Time management is another critical factor. Allocate time wisely during your preparation and during the evaluation itself, ensuring you can tackle both straightforward and complex tasks. Practicing under timed conditions can simulate real scenarios and boost your confidence.
Finally, reviewing your progress regularly helps solidify your knowledge. Analyze any errors to understand the gaps in your learning and refine your approach. This iterative process ensures steady improvement and readiness for success.
Top Strategies for Math Success
Achieving excellence in quantitative problem-solving requires a thoughtful combination of preparation techniques and focused practice. By adopting proven strategies, you can enhance your ability to approach questions efficiently and accurately.
Build a Strong Foundation
Begin by reinforcing your understanding of fundamental concepts. Ensure you grasp the basics of calculations, formulas, and logical reasoning. A solid groundwork enables you to solve more advanced problems with confidence.
Practice with Purpose
Work on diverse problem types to familiarize yourself with a range of scenarios. Focus on both speed and precision, striving to minimize errors while maintaining a steady pace. Regular review of completed exercises helps identify areas that need improvement.
Consistent effort, combined with the right techniques, will prepare you to excel in numerical challenges and achieve outstanding results.
Common Mistakes Students Should Avoid
When preparing for numerical evaluations, certain errors can hinder progress and reduce overall performance. Recognizing these pitfalls early can help refine your approach and improve outcomes.
Overlooking Basics
- Skipping foundational topics can lead to confusion with more advanced questions.
- Neglecting to review formulas or basic principles may cause avoidable errors.
Mismanaging Time
- Spending too much time on a single question can limit your ability to complete the entire evaluation.
- Failing to allocate time for reviewing completed sections increases the risk of missed errors.
By identifying and addressing these common mistakes, students can enhance their preparation process, boost confidence, and achieve better results in numerical problem-solving tasks.
Detailed Solutions for Complex Problems
Tackling challenging numerical tasks requires a clear understanding of the underlying principles and a step-by-step approach. Breaking down intricate problems into manageable parts can simplify the process and lead to accurate solutions.
Step-by-Step Problem Breakdown

Begin by analyzing the question carefully to identify the key information provided. Highlight the relationships or patterns within the data to determine the most efficient method for solving. Each step should build logically on the previous one, ensuring clarity and accuracy.
Using Examples to Clarify Concepts
Practical examples are an excellent way to illustrate solutions. By applying techniques to real-world scenarios, you can understand how theoretical concepts translate into actionable steps. Examples also help in spotting common pitfalls and learning how to avoid them.
By practicing detailed solutions and focusing on a methodical approach, you can master even the most complex numerical challenges and improve your problem-solving skills significantly.
Understanding Key Math Concepts Easily
Grasping essential numerical principles is the foundation for solving a wide variety of problems effectively. By focusing on clarity and simplification, you can make even the most abstract ideas more approachable and easier to apply.
Breaking Down Complex Ideas
Start by dividing complicated topics into smaller, more digestible parts. Identify the core idea within each concept and build your understanding step by step. Visual aids, such as diagrams or charts, can help clarify relationships and patterns.
Connecting Theory to Real-Life Examples
Relating theoretical knowledge to everyday scenarios makes learning more intuitive. When you see how these principles function in real-world applications, it becomes easier to understand their relevance and usage in problem-solving.
With consistent effort and a focus on simplifying difficult concepts, you can develop a stronger grasp of key ideas, making problem-solving more efficient and effective.
Tips for Managing Test Time Effectively
Time management is crucial for performing well in any assessment. Properly allocating time to each section ensures that you can complete all tasks without rushing or leaving questions unanswered. Implementing a strategic approach can help you make the most of your time and improve your results.
Prioritize and Plan
- Start by quickly scanning through the entire section to identify easier questions that you can answer quickly.
- Allocate more time to challenging problems, but avoid spending too long on any single question.
- Skip questions that take too much time and come back to them later if you have time left over.
Practice Under Time Constraints
- Simulate real testing conditions by practicing with a time limit. This builds familiarity with the pacing required.
- Track your progress to ensure you’re staying on schedule and make adjustments as needed.
By managing your time effectively, you can maximize your performance and approach each question with a clear, focused mindset.
Breaking Down Challenging Math Questions
Complex problems can often feel overwhelming at first glance, but breaking them into smaller, manageable parts can make them much more approachable. By analyzing each aspect of a question systematically, you can identify patterns and apply the most effective strategies to find the solution.
Identify Key Information
Start by carefully reading the problem and highlighting essential details. Look for numbers, relationships, and key phrases that indicate what the question is asking. Understanding the core components of the problem is the first step toward finding the solution.
Break the Problem into Steps

Once you’ve identified the key information, break the problem down into logical steps. Solving a complex question often requires multiple calculations or reasoning processes. By tackling one step at a time, you avoid feeling overwhelmed and increase your chances of accuracy.
With practice, these techniques will allow you to approach even the most difficult problems with confidence and clarity.
Using Practice Tests for Better Scores

Engaging with simulated exams is one of the most effective ways to improve your performance. These exercises help you familiarize yourself with the format, practice time management, and identify areas that need more attention. With consistent effort, they can significantly boost your confidence and results.
Familiarize Yourself with the Format
By regularly completing simulated exams, you become accustomed to the structure of the questions, the pacing, and the overall flow. This familiarity reduces anxiety and helps you perform more efficiently when faced with the real challenge.
Analyze Your Mistakes
After completing each simulation, take time to review your mistakes and understand why certain answers were incorrect. Identifying weak points allows you to focus your study efforts on the areas that will most improve your performance.
Using these methods consistently not only increases your comfort with the material but also sharpens your problem-solving skills, helping you achieve better outcomes in the long run.
How to Improve Problem Solving Skills
Improving your ability to solve complex problems is a gradual process that requires practice and strategic thinking. By developing a systematic approach, you can enhance your analytical skills and tackle even the most difficult challenges with confidence. The key lies in breaking down problems into smaller, more manageable parts and using logical reasoning to find solutions.
Break Problems into Manageable Parts
Start by carefully analyzing the problem and dividing it into simpler components. Identify the given information and what the question is asking. This will help you organize your thoughts and make the process less overwhelming.
Practice Logical Reasoning and Patterns
Developing strong logical reasoning skills is essential for solving problems effectively. Look for patterns and relationships between different elements of the problem. Once you recognize these, you can apply relevant strategies more quickly and accurately.
With consistent practice, you will not only improve your problem-solving ability but also become more confident in approaching challenges of any complexity.
Key Formulas to Memorize for Success
Mastering essential formulas is a critical part of performing well in problem-solving challenges. Having these key equations readily available in your memory will save time and increase accuracy when solving complex questions. These formulas form the foundation of many problems and can make a significant difference in your overall performance.
| Formula | Description |
|---|---|
| Area of a Circle: A = πr² | Used to find the area of a circle given its radius. |
| Pythagorean Theorem: a² + b² = c² | Helps in finding the sides of a right triangle. |
| Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a | Used to solve quadratic equations of the form ax² + bx + c = 0. |
| Perimeter of a Rectangle: P = 2(l + w) | Used to calculate the perimeter when the length and width are known. |
| Slope Formula: m = (y₂ – y₁) / (x₂ – x₁) | Helps in finding the slope between two points on a line. |
By memorizing these formulas and understanding their applications, you’ll be better equipped to tackle a wide range of challenges with efficiency and confidence.
Practical Exercises for Skill Building
Engaging in focused exercises is essential for honing your problem-solving abilities. These activities help reinforce key concepts, improve accuracy, and build confidence. By practicing various types of challenges, you can solidify your understanding and speed up your performance when faced with more complex scenarios.
Effective Exercises to Enhance Skills
- Timed Drills: Complete sets of problems within a set time limit to improve both speed and accuracy.
- Step-by-Step Solutions: Break down each problem into manageable steps to ensure you understand the process behind each solution.
- Conceptual Problems: Focus on exercises that emphasize understanding core concepts and their applications, rather than just memorization.
- Real-Life Application: Solve practical, real-world problems to better understand how abstract principles relate to everyday situations.
Tips for Effective Practice
- Set specific goals for each practice session, such as mastering a particular formula or type of problem.
- Review your mistakes after completing each exercise to understand where improvements are needed.
- Incorporate a mix of problem difficulty levels to avoid frustration and ensure steady progress.
By consistently working through practical exercises, you’ll strengthen your skills and be more prepared to tackle even the most challenging problems with confidence and ease.
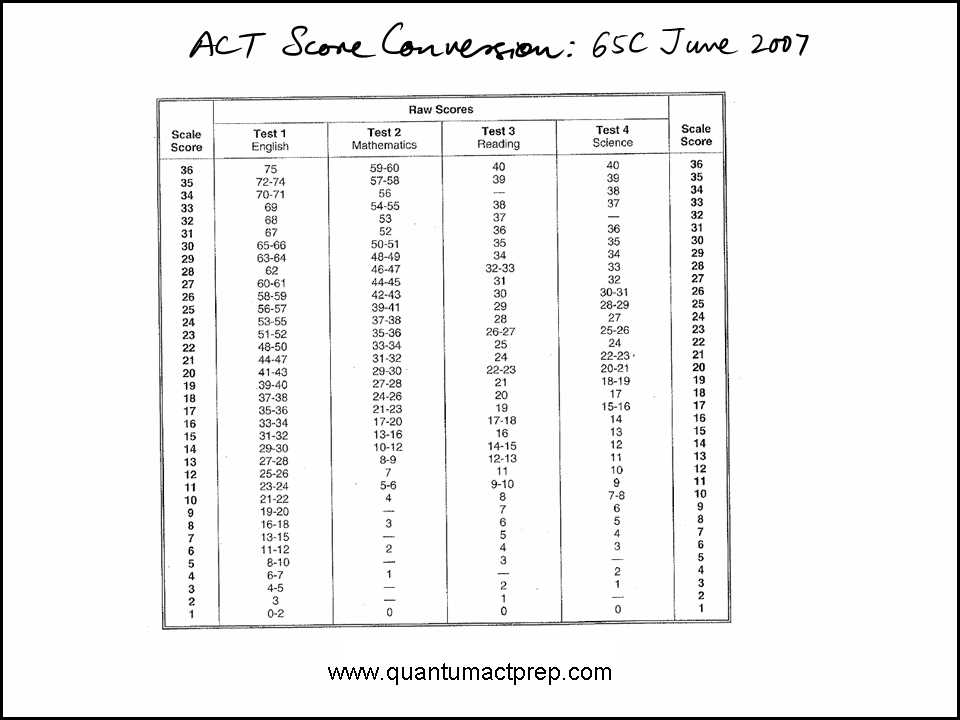
How to Interpret Math Test Results
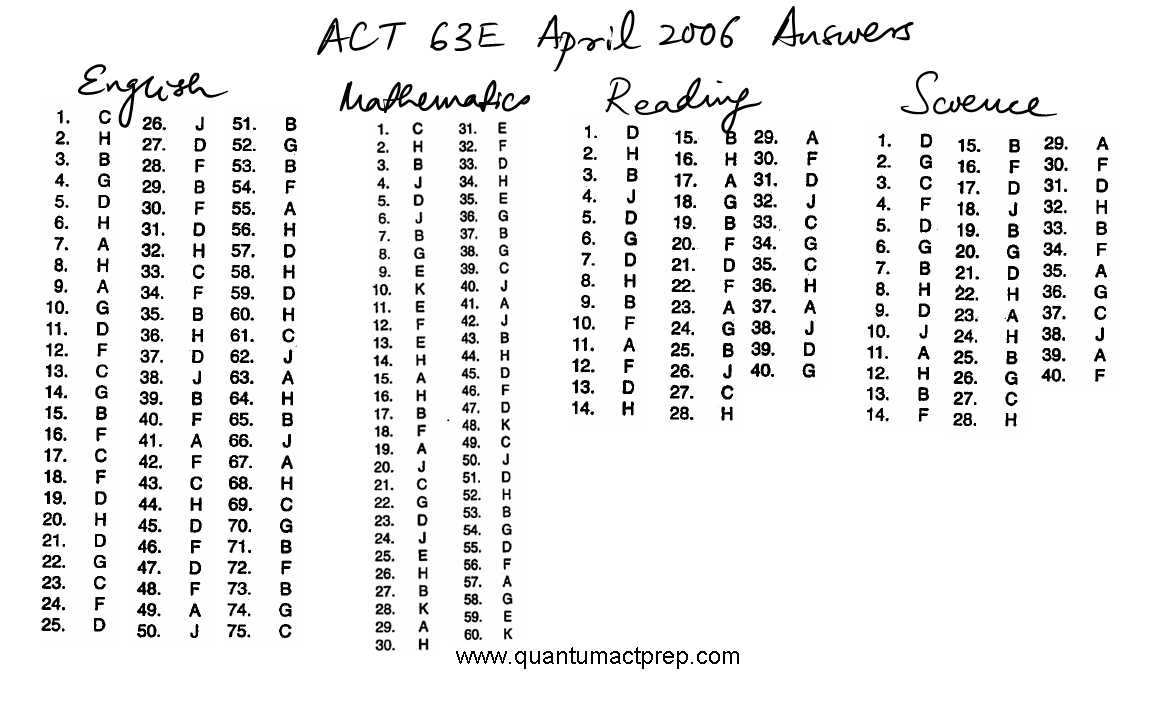
Understanding the results of your assessments is a crucial step in improving problem-solving skills. It is not just about seeing whether an answer is correct or incorrect, but also recognizing the underlying patterns, mistakes, and areas for improvement. By interpreting your results effectively, you can identify where to focus your efforts for greater success.
Start by reviewing each problem, paying close attention to both the answers you got right and the ones you missed. Look for recurring themes or concepts in the mistakes you made. This will help you pinpoint which areas need more practice. For example, if you consistently struggle with fractions, it’s a sign that you may need to revisit the fundamental concepts behind them.
It’s also important to consider the time taken to answer each question. If you missed questions due to rushing, focus on time management strategies. If you spent too much time on a problem, practice techniques that can help you solve it more efficiently. This can help you find a balance between speed and accuracy in future assessments.
Finally, reflect on the strategies you used to approach the problems. Were there faster methods you could have used? Were there alternative ways to solve the problems that you missed? This reflection is key to improving your approach and refining your problem-solving skills.
Importance of Consistent Practice Sessions
Regular and focused training plays a key role in enhancing problem-solving abilities. By engaging in consistent exercises, individuals not only strengthen their skills but also develop a deeper understanding of the core concepts involved. It’s this ongoing effort that ensures steady improvement and boosts overall confidence.
One of the primary benefits of consistent practice is that it helps solidify fundamental concepts, making them second nature. This repeated exposure allows you to recognize patterns and connections between different problems, improving your efficiency and accuracy when tackling new challenges. The more you practice, the faster you become at applying learned strategies.
Building Momentum is another critical aspect of regular exercises. As you make progress, even small victories contribute to a sense of achievement, which in turn encourages you to keep going. This positive reinforcement creates a cycle of continuous improvement, helping you remain motivated and determined.
Setting aside dedicated time for these sessions is essential. Whether you choose short, frequent sessions or longer, focused intervals, the goal is consistency. By making this a habit, you’ll not only sharpen your abilities but also build resilience and the ability to handle more complex tasks with ease.
Reviewing and Learning from Mistakes
Making errors is an inevitable part of the learning process, but it’s how we respond to them that determines progress. Instead of seeing mistakes as setbacks, viewing them as valuable learning opportunities can significantly enhance your problem-solving skills. Reflecting on where things went wrong and understanding the root causes helps to avoid similar pitfalls in the future.
When reviewing mistakes, it’s important to not just identify what went wrong but also explore why it happened. This deeper analysis allows you to pinpoint weak areas and develop strategies to overcome them. Whether it’s a misunderstanding of a concept, rushing through a problem, or overlooking key details, each mistake offers insights into how you can improve.
Tracking Patterns in your errors can also be beneficial. If certain types of mistakes occur repeatedly, it might signal a need to revisit specific concepts or practice certain techniques. By addressing these recurring issues, you can gradually turn weaknesses into strengths.
Learning from mistakes requires patience and a mindset focused on growth. Acknowledging errors, understanding the lessons they provide, and adjusting your approach can lead to greater mastery of the material. Ultimately, this process builds both skill and confidence, ensuring you are better prepared for future challenges.
Essential Resources for ACT Math Preparation
To excel in any subject, having the right tools and materials is crucial. Whether you’re preparing for an exam or simply seeking to improve your skills, the right resources can make a significant difference. By using carefully selected study materials, you can effectively build knowledge and confidence, ensuring you’re well-prepared for any challenge that comes your way.
Here is a list of key resources that can assist in enhancing your performance:
| Resource Type | Description | Recommended Use |
|---|---|---|
| Books | Comprehensive guides that cover fundamental concepts and strategies. | Use for detailed explanations and a wide range of practice questions. |
| Online Tutorials | Interactive video lessons that demonstrate various problem-solving techniques. | Ideal for visual learners and those looking to review specific topics. |
| Question Banks | A collection of past questions and practice problems. | Perfect for testing your skills and getting familiar with the format. |
| Mobile Apps | Portable applications that provide on-the-go study sessions and quizzes. | Use for quick reviews and consistent, daily practice. |
By incorporating these resources into your study plan, you can strengthen your abilities and build a deeper understanding of the material. Balancing these tools with consistent effort will contribute greatly to achieving success.