
In this section, we explore fundamental principles and methods used to solve complex problems. With a focus on practical approaches, we aim to provide clear, step-by-step guidance to help you grasp essential topics and develop a deeper understanding.
By breaking down challenging exercises and explaining the necessary techniques, we ensure that each concept is easily accessible. Whether you’re looking for tips to enhance your comprehension or solutions to specific problems, this guide will support your learning journey.
Key Solutions and Explanations
This section focuses on unraveling complex problems by presenting detailed solutions. We provide a structured approach to dissecting various topics, highlighting the essential techniques needed for tackling challenges effectively.
Each example is broken down step by step, ensuring clarity and a deeper understanding. By following the outlined methods, you will gain confidence in solving similar problems and applying these strategies in future tasks.
Understanding Key Concepts in Chapter 5
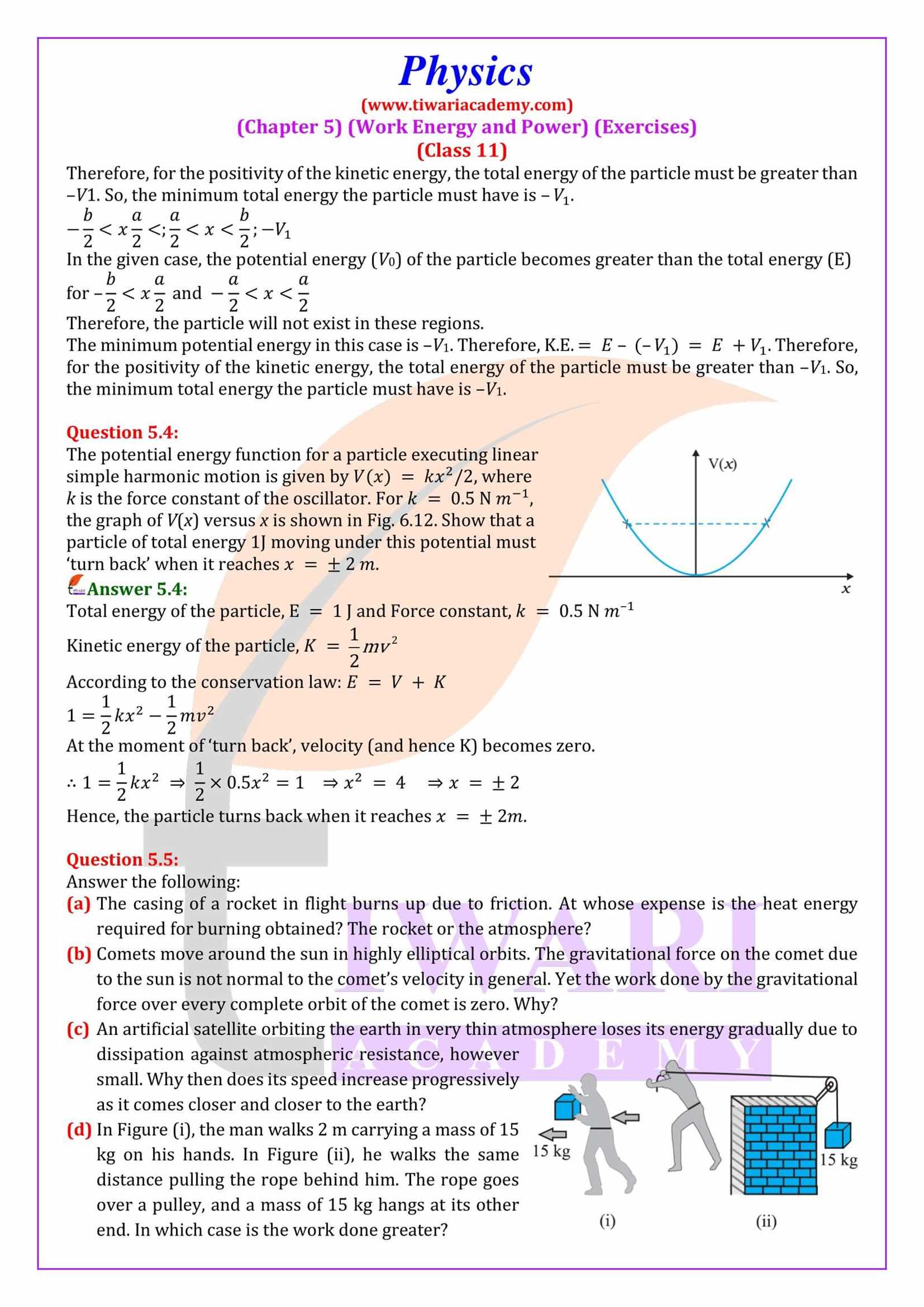
In this section, we delve into the essential ideas that form the foundation of the material. By exploring the core principles, we aim to provide a solid framework for understanding more advanced topics. Grasping these fundamental concepts is crucial for tackling more complex challenges that follow.
Core Principles and Their Applications
We begin by examining the fundamental theories that govern the subject. These core principles are not only vital for solving exercises but also serve as the basis for practical applications in real-world scenarios.
Building a Strong Conceptual Foundation
By focusing on the key ideas, we help reinforce the mental models needed to approach problems confidently. A strong understanding of these concepts will improve both theoretical knowledge and problem-solving skills.
How to Approach Problems Effectively

To tackle challenging problems with confidence, it’s essential to break them down into manageable steps. A clear strategy can help you navigate through complex scenarios and arrive at the correct solution with less frustration. This section will guide you through effective techniques for problem-solving.
Key Steps to Solve Complex Problems
- Read and Understand the Problem: Carefully analyze the question and identify the given information and what is being asked.
- Identify Relevant Concepts: Determine which principles or formulas apply to the problem.
- Organize Information: Write down the known values and make a plan of action for how to use them.
- Apply Formulas: Use appropriate equations to connect the given data and solve for the unknown.
- Check Your Work: Review the calculations and ensure the final result makes sense in the context of the problem.
Common Strategies for Success
- Practice Regularly: Frequent practice helps solidify concepts and improves problem-solving speed.
- Stay Organized: A methodical approach prevents mistakes and ensures that no important information is overlooked.
- Seek Alternative Solutions: Try solving the same problem in different ways to deepen your understanding.
Step-by-Step Guide to Solutions

This section provides a detailed, step-by-step approach to solving various problems. By breaking down each task into simple actions, we help you understand the underlying methods and apply them confidently to similar exercises.
| Step | Description |
|---|---|
| 1 | Read and Analyze the Problem: Begin by thoroughly understanding the question, identifying the key variables and what needs to be solved. |
| 2 | Gather Relevant Information: Organize the given data, noting any necessary units and constants that may be involved in the solution process. |
| 3 | Choose the Right Formula: Identify which equations or principles are applicable to the problem based on the information provided. |
| 4 | Substitute Values: Carefully substitute the known values into the equation and perform the necessary calculations. |
| 5 | Check the Solution: Once the calculations are completed, double-check the results to ensure they are logical and consistent with the problem context. |
Important Formulas from Chapter 5
This section highlights the key equations essential for solving problems in this topic. Understanding and applying these formulas will help you tackle various exercises with confidence and accuracy.
Fundamental Equations
These core formulas are widely used and form the foundation of many problem-solving techniques. Make sure to memorize them and practice using them in different scenarios.
- Formula 1: Equation for calculating speed:
v = d / t - Formula 2: Equation for calculating force:
F = m * a - Formula 3: Equation for work done:
W = F * d
Advanced Formulas
These advanced equations are crucial for tackling more complex problems. They provide deeper insight into the relationship between variables and allow for more refined calculations.
- Formula 4: Equation for kinetic energy:
KE = 1/2 * m * v² - Formula 5: Equation for potential energy:
PE = m * g * h
Common Mistakes and How to Avoid Them
When solving complex problems, it’s easy to make errors that can lead to incorrect results. Recognizing these common mistakes and understanding how to avoid them is crucial for improving your problem-solving skills and achieving accurate outcomes.
Typical Errors to Watch Out For
Several mistakes frequently occur during problem-solving. Being aware of them can help you avoid unnecessary setbacks and improve your approach.
- Misinterpreting the Question: Always ensure you fully understand the problem before beginning the solution process. Read the question carefully and highlight important details.
- Incorrect Unit Conversion: Units are essential in solving problems accurately. Double-check that all units are consistent and convert them properly if needed.
- Forgetting to Include Constants: Some problems require constants such as gravitational acceleration or specific material properties. Make sure these are included in your calculations when necessary.
How to Improve Accuracy

By adopting a more systematic approach, you can minimize mistakes and solve problems more effectively.
- Double-Check Calculations: Always review your math before finalizing an answer. A small error can lead to significantly different results.
- Use Diagrams and Visual Aids: Drawing diagrams or labeling important variables can help you better understand the problem and avoid confusion.
- Practice Regularly: The more problems you solve, the more familiar you become with potential pitfalls and how to avoid them.
Real-World Applications of Principles
The concepts covered in this section are not just theoretical; they have practical applications in many fields of everyday life. Understanding how these principles are used in real-world situations enhances your ability to apply them effectively and see their relevance beyond the classroom.
Examples of Practical Applications
The principles learned in this section are applied in a wide range of industries, from engineering to healthcare. Below are some key examples where these concepts are crucial:
| Application | Principle Used |
|---|---|
| Automobile Safety Systems | Force and Motion: The principles of force, acceleration, and deceleration are used in designing airbags and seatbelts to ensure safety during collisions. |
| Electric Power Generation | Energy Conversion: The conversion of mechanical energy to electrical energy in power plants uses concepts of energy transfer and efficiency. |
| Medical Imaging | Waves and Radiation: Techniques such as X-rays and MRIs utilize the properties of waves and radiation for diagnostic purposes. |
| Space Exploration | Gravitational Forces: Understanding gravitational fields and orbits is essential for satellite launches and space missions. |
Benefits of Understanding Applications
By applying theoretical knowledge to practical scenarios, you can not only solve real-world problems but also innovate new solutions. This understanding is a key driver of technological advancements and improvements in various industries.
Tips for Mastering Content
To truly grasp the material and excel in solving related problems, it’s important to adopt effective study techniques. By following a structured approach and focusing on key areas, you can build a solid understanding and perform well in assessments.
Effective Study Techniques
Mastering complex topics requires dedication and the right methods. Here are a few strategies that can help improve your comprehension and retention:
- Active Problem Solving: Regularly practice solving problems to reinforce your understanding of the concepts. The more problems you work through, the better you’ll grasp the underlying principles.
- Understand, Don’t Memorize: Rather than memorizing formulas, focus on understanding how and why they are used. This approach helps when you encounter variations of problems.
- Group Study: Discussing difficult topics with peers can provide new insights and clarify concepts that might be confusing when studied alone.
Additional Strategies for Success

Incorporating these additional tips can further enhance your grasp of the material and improve overall performance:
- Break Down Complex Ideas: If a concept seems overwhelming, break it into smaller parts and tackle each one individually before putting everything together.
- Use Visual Aids: Diagrams, charts, and mind maps can help visualize relationships between variables and simplify complex processes.
- Review Regularly: Regular review helps solidify the material in your memory and prevents forgetting key concepts over time.
How to Study for Better Results
Achieving excellent results in this subject requires more than just passive reading; it involves an active approach to learning. By incorporating effective study strategies, you can deepen your understanding and improve your problem-solving abilities.
Start by focusing on the key concepts, ensuring you understand the underlying principles before moving on to more complex problems. Break down difficult topics into smaller, manageable sections to make learning less overwhelming.
Regular practice is essential. The more problems you solve, the more confident you’ll become in applying theories to real-world scenarios. It’s also important to review and reinforce your knowledge frequently to retain key information long-term.
Lastly, seek support when necessary. If you encounter challenges, discuss them with peers or instructors. Collaborative learning can offer new perspectives and help clarify difficult concepts.
Detailed Explanation of Key Exercises
In this section, we will go through some essential exercises step by step to illustrate how to apply the concepts learned. By breaking down these problems, you’ll gain a better understanding of the methods used to find solutions and how to approach similar challenges effectively.
Each exercise highlights a key principle and demonstrates the process of analyzing and solving problems. Understanding the reasoning behind each step will help reinforce your learning and improve your problem-solving skills. Whether it’s applying formulas, analyzing data, or interpreting results, these exercises will provide a comprehensive guide to mastering the material.
As you work through each problem, remember that practice is key. The more familiar you become with solving different types of exercises, the more confident and efficient you will be in applying the principles to new scenarios.
Breaking Down Complex Problems
When faced with intricate problems, it can be overwhelming to know where to begin. The key to tackling these challenges is breaking them down into smaller, more manageable steps. By carefully analyzing each component of the problem, you can identify the best approach for finding the solution.
The first step is to read through the problem thoroughly, ensuring that you understand all the variables and conditions involved. Once you have a clear understanding, start by identifying the key concepts and formulas that apply. This will help you organize your thoughts and decide on the most effective method for solving the problem.
Breaking down complex tasks involves:
- Identifying known and unknown variables: Make a list of the values given and what you need to find.
- Choosing appropriate formulas: Select the equations that relate to the situation and can help solve for the unknowns.
- Solving step by step: Work through the problem systematically, ensuring each step follows logically from the previous one.
By approaching each problem in this structured way, you’ll find that even the most complex situations become much easier to navigate and solve effectively.
Understanding Units and Measurements
Accurate measurement is essential when solving problems that involve physical quantities. To analyze and interpret results correctly, it is important to understand how various units of measurement are applied and how they relate to each other. This section provides insight into the significance of units and how to work with them effectively in problem-solving.
Key Concepts of Units
Every physical quantity is associated with a unit, which represents the standard by which it is measured. Understanding these units and their relationships helps to ensure consistency in calculations and allows for meaningful comparisons. The International System of Units (SI) is the most widely used system, but it is important to recognize and convert between different systems when necessary.
Converting Units and Dimensional Analysis

In many problems, you will need to convert from one unit to another. Knowing how to convert units correctly is crucial for obtaining the right answers. Dimensional analysis, which involves checking the consistency of units in equations, is a useful tool for verifying your calculations and ensuring that you are using the correct units throughout the problem.
How to Use Graphs in Problems
Graphs are powerful tools for visualizing relationships between variables and simplifying complex problems. They allow you to interpret data quickly, identify trends, and make predictions based on graphical representations. Understanding how to read, interpret, and construct graphs is crucial for solving various types of problems effectively.
Graphs can represent many different types of relationships, such as linear or non-linear. The first step is to understand the axes and the units used on each. Once you grasp these basics, you can extract valuable information from the graph, such as the slope, intercepts, and areas under curves.
Key Steps to Using Graphs
- Label the axes: Ensure each axis is clearly labeled with the appropriate quantity and unit.
- Plot data points: Carefully plot each point according to the values given in the problem.
- Draw the curve or line: Depending on the data, either connect the points with a straight line or draw a curve to best fit the data.
- Interpret the graph: Identify key features such as slopes, intercepts, or areas under the curve that can provide answers to the problem.
By using graphs, you can visualize the problem, recognize patterns, and solve it more efficiently. Whether you are analyzing motion, forces, or other quantities, graphs will often be your guide to clearer understanding and faster solutions.
Concepts Related to Energy and Motion
Understanding how energy and motion are interconnected is fundamental in studying the dynamics of objects and systems. These concepts form the backbone of many problems, as they explain how forces and energy transfer affect movement. Grasping the relationship between work, energy, and motion enables a deeper comprehension of natural phenomena and mechanical systems.
Energy and Its Forms
Energy exists in many forms, including kinetic, potential, thermal, and mechanical. Kinetic energy refers to the energy of an object in motion, while potential energy is stored energy based on position or configuration. By understanding these two primary types of energy, we can analyze how energy is transferred or transformed during various physical processes. For instance, when an object falls, potential energy is converted into kinetic energy.
Motion and Work

Work is done when a force causes an object to move over a distance. The concept of work is crucial when studying motion, as it is directly linked to energy transfer. The equation for work–force times distance–helps in calculating the energy used or transferred when an object moves. Understanding this relationship allows for the analysis of both linear and rotational motion in various contexts.
Advanced Topics Covered in Chapter 5
In this section, we explore more sophisticated concepts that expand on fundamental principles, diving into areas that require a deeper understanding of the material. These advanced topics bridge the gap between theoretical knowledge and practical application, offering insight into complex systems and phenomena. They provide a more intricate perspective on how energy, forces, and motion interact in various scenarios.
Key Advanced Topics
- Conservation Laws: These laws explain how certain quantities, such as energy and momentum, remain constant in isolated systems, even during interactions.
- Work-Energy Theorem: This theorem connects the work done on an object with the change in its kinetic energy, offering a powerful tool for solving problems in mechanics.
- Rotational Dynamics: The study of how objects rotate and the forces involved, extending basic motion principles to angular motion.
- Energy Transformation: Understanding how energy can change forms, such as from potential to kinetic energy, and the factors that influence these transitions.
Real-World Applications
The understanding of these advanced topics is essential not only in academic settings but also in real-world applications, such as engineering, aerospace, and renewable energy technologies. By studying how energy is conserved or transformed, we can optimize systems for better performance and efficiency, whether in designing machines or understanding natural processes like weather patterns or the behavior of particles at high speeds.
Practice Problems to Reinforce Learning
To strengthen your understanding of key concepts, it’s essential to actively apply what you’ve learned through targeted exercises. Practice problems are a great way to test your knowledge, identify areas where you may need further review, and gain confidence in solving real-world problems. By regularly working through problems, you can deepen your grasp of theoretical principles and improve your problem-solving skills.
Suggested Problems
- Kinetic Energy Calculation: A car is moving at a certain velocity. Calculate its kinetic energy and determine how it changes when the speed is doubled.
- Work and Force Relationship: A person applies a constant force to lift a box. Calculate the work done, considering the displacement and direction of force.
- Energy Conservation in Collisions: Analyze a collision between two objects, using conservation laws to determine the final velocities.
- Momentum Problems: A moving object collides with a stationary one. Use the principles of momentum conservation to find the final velocities of the objects after the impact.
Approaching Practice Problems
- Step 1: Read the problem carefully and identify known variables.
- Step 2: Choose the appropriate formula or method to approach the problem.
- Step 3: Solve step-by-step, ensuring each stage is logically sound.
- Step 4: Check your final answer for consistency with the problem’s units and conditions.
By practicing a variety of problems, you can develop a systematic approach to solving complex issues, making it easier to navigate more advanced topics in the future.
How Chapter 5 Relates to Other Topics
Understanding the concepts explored in this section is essential, as they lay the groundwork for a wide range of related subjects. The principles introduced here not only provide a foundation for future studies but also connect to several broader concepts across the field. Whether you are tackling advanced topics or revisiting basic principles, this material serves as a bridge between earlier lessons and more complex subjects.
For example, the concepts explored here have strong ties to energy conservation, momentum, and the behavior of forces, which are essential for understanding mechanical interactions. In later sections, these principles become more nuanced as they apply to dynamic systems, thermodynamics, and even modern theories. The interconnectedness of topics emphasizes the importance of mastering these core ideas before advancing further in the study of the field.
Building a Strong Foundation in Physics
Establishing a solid understanding of the fundamental principles is crucial for mastering more advanced topics. By grasping the basic concepts and methods early on, you equip yourself with the tools needed to approach more complex challenges. This strong base will enable you to navigate intricate problems with greater ease and confidence, as each new concept often builds on what you’ve already learned.
A well-structured approach to studying these foundational topics ensures that the connections between various theories and laws are clear. It is important to not only understand individual concepts but also how they relate to one another within the broader framework of the subject.
| Key Concepts | Importance |
|---|---|
| Fundamental Forces | These form the basis for understanding interactions in various systems. |
| Energy and Work | These principles are crucial for explaining how systems change and evolve over time. |
| Motion and Forces | They provide the groundwork for more complex analyses of systems in motion. |