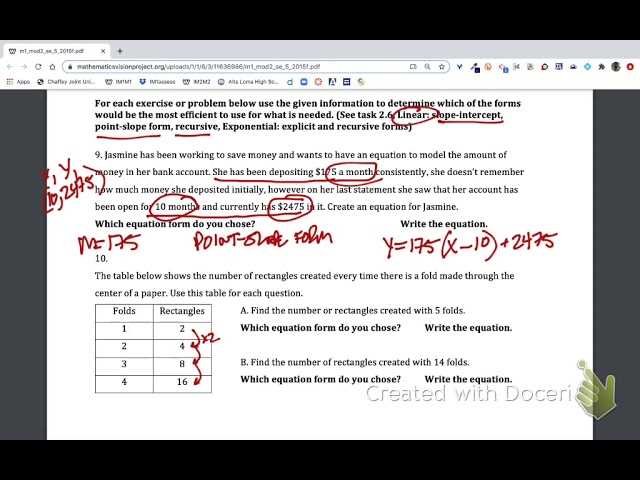
The fourth section of the introductory course covers a variety of essential concepts that are crucial for mastering the subject. This part emphasizes the application of learned principles through practice, problem-solving, and critical thinking. It aims to provide students with a strong foundation and the necessary tools to succeed in more advanced areas of study.
In this section, you will encounter different types of challenges, ranging from simple calculations to complex word problems that require a deeper understanding of theoretical ideas. By following the step-by-step solutions provided, you will be able to identify patterns and develop strategies to approach similar problems with confidence.
Comprehensive explanations and strategic tips are designed to guide you through the learning process, helping you not only solve problems but also build the problem-solving mindset necessary for further academic achievement.
Understanding Key Solutions and Techniques
This section is focused on providing clear explanations and effective methods for tackling common problems within the fourth level of the course. Here, we break down various exercises step-by-step, offering insights into how to approach and solve them efficiently. Whether you are looking to reinforce your understanding or refine your problem-solving skills, this guide will help you achieve your learning goals.
To better assist with mastering this section, we cover a range of example problems and their solutions, emphasizing critical thinking and logical approaches. By following these structured steps, you can gain confidence in your ability to solve similar challenges on your own.
- Step 1: Identify the key variables and understand the problem requirements.
- Step 2: Apply the correct formula or method based on the type of question.
- Step 3: Solve the problem systematically, ensuring each step follows logically from the previous one.
- Step 4: Double-check your work for accuracy and consistency.
By following these steps, you will not only be able to solve individual problems but also build a solid foundation for tackling more complex scenarios in future coursework. Understanding the core principles behind each solution is essential for long-term success.
For additional practice, be sure to review the provided examples and attempt similar exercises to strengthen your problem-solving techniques. The more you practice, the easier it becomes to spot patterns and develop effective strategies for various problem types.
Understanding the Key Concepts
Grasping the fundamental principles is crucial for mastering this level. The key concepts introduced in this section are designed to build a solid foundation, allowing students to apply learned skills in a variety of contexts. Understanding these concepts will not only help you solve specific problems but also develop a deeper understanding of the subject overall.
Core Ideas and Their Applications
At the heart of this section are a few central ideas that students must understand to move forward. These concepts can be applied to a wide range of exercises and often require careful thought and practice to fully internalize. Let’s explore how these core ideas play out in problem-solving scenarios.
| Concept | Description | Example |
|---|---|---|
| Variable Relationships | Understanding how different elements of a problem interact and affect each other. | Solving equations with multiple unknowns. |
| Problem Breakdown | The ability to divide complex problems into smaller, manageable parts. | Solving word problems step by step. |
| Mathematical Patterns | Identifying recurring structures that can simplify solutions. | Recognizing arithmetic sequences in number series. |
How These Concepts Relate to One Another
The concepts in this section are often interrelated. A deep understanding of each will help you see how they connect, allowing for more efficient problem solving. For example, identifying variable relationships can help you break down a problem, while recognizing patterns can speed up the process of finding a solution. The more familiar you become with these ideas, the easier it will be to apply them in different contexts.
Step-by-Step Solutions for Exercises

This section provides detailed instructions to help you work through various exercises, breaking them down into manageable steps. The goal is to guide you through each problem systematically, ensuring a clear understanding of the process and helping you build confidence in solving similar tasks on your own.
By following each step closely, you’ll develop the skills needed to approach a wide range of problems. These solutions are structured to help you focus on the most important concepts, avoiding unnecessary confusion and making each solution more approachable.
Example Exercise 1: Solving a Linear Equation
Let’s go through the process of solving a basic equation with one unknown. The steps below will help you solve the equation step by step:
- Step 1: Write down the equation clearly.
- Step 2: Simplify both sides of the equation if necessary.
- Step 3: Isolate the variable by performing operations on both sides.
- Step 4: Solve for the unknown value.
- Step 5: Check your solution by substituting it back into the original equation.
Example Exercise 2: Solving a Word Problem
Now, let’s break down a word problem and convert it into a mathematical equation:
- Step 1: Read the problem carefully and identify the key information.
- Step 2: Translate the words into a mathematical expression or equation.
- Step 3: Solve the equation using appropriate methods.
- Step 4: Interpret the solution in the context of the problem.
- Step 5: Verify your solution to ensure it makes sense with the given information.
By following these steps for each exercise, you’ll gain a deeper understanding of the problem-solving process and improve your overall performance in similar tasks.
Common Mistakes and How to Avoid Them
When solving problems in this section, it’s easy to make small errors that can lead to incorrect results. Recognizing these common pitfalls is essential to avoid them and improve your accuracy. Understanding the nature of these mistakes will help you develop better strategies for problem-solving and strengthen your overall grasp of the material.
In this section, we’ll explore the most frequent mistakes that students make and provide practical advice on how to prevent them. With careful attention to detail and a methodical approach, these errors can be minimized, allowing you to tackle each problem with confidence.
| Mistake | Cause | How to Avoid |
|---|---|---|
| Incorrectly simplifying expressions | Rushing through steps or overlooking arithmetic rules | Take your time to carefully check each simplification. |
| Forgetting to check the solution | Skipping the verification step after solving | Always substitute your answer back into the original problem. |
| Misinterpreting word problems | Not identifying key information in the text | Highlight important details and translate them into equations. |
| Skipping steps in multi-step problems | Overlooking intermediate stages to save time | Write out each step clearly to avoid missing any important calculation. |
By staying mindful of these common mistakes and applying the recommended solutions, you can enhance your problem-solving skills and avoid unnecessary setbacks. Practicing consistently and reviewing each step carefully will help you improve your precision and efficiency over time.
Important Formulas in Module 4
In this section, we will focus on the key formulas that are essential for solving problems in this level. These formulas serve as the foundation for many of the exercises and help you apply the right approach to various types of questions. Mastering these expressions will enable you to tackle challenges more effectively and efficiently.
Each formula has a specific purpose, and understanding how to use it correctly is crucial for finding the right solutions. Whether you’re solving equations, analyzing relationships, or working through more complex problems, these formulas will be your guide throughout the process.
Linear Equation Formula
The linear equation is one of the most fundamental concepts, often appearing in various forms. The general formula is:
y = mx + b
Where:
- y is the dependent variable
- m is the slope of the line
- x is the independent variable
- b is the y-intercept
Quadratic Formula
For solving quadratic equations, the following formula is essential:
x = (-b ± √(b² – 4ac)) / 2a
Where:
- a, b, and c are the coefficients of the quadratic equation ax² + bx + c = 0
- √ represents the square root
These formulas, along with others specific to the topic, are vital for understanding the mathematical principles at play and efficiently solving the exercises in this section. Ensure that you understand when and how to apply each formula for the best results.
How to Solve Word Problems Effectively
Word problems can often seem intimidating due to their complex language and abstract nature. However, breaking down the problem into smaller, manageable steps can make it much easier to solve. This section will guide you through the process of tackling word problems in a structured and systematic way, helping you develop the skills to approach them with confidence.
Step 1: Understand the Problem
The first step in solving any word problem is to carefully read and understand the situation being described. Focus on the key information and identify what the question is asking for. It’s important to highlight or underline important details such as quantities, relationships, and specific instructions.
- Identify: What is the question asking?
- Determine: What are the known values and unknowns?
- Clarify: Are there any units or conversions needed?
Step 2: Translate into Mathematical Expressions
Once you have a clear understanding of the problem, the next step is to translate the words into a mathematical form. This often involves creating equations or identifying relationships between variables. Pay attention to keywords that indicate specific operations, such as “sum,” “difference,” “product,” and “quotient.”
- Keywords: Use terms like “total,” “each,” “per,” and “difference” to identify the correct operation (addition, subtraction, multiplication, or division).
- Equations: Write the equation that represents the problem and its relationships.
- Variables: Define your unknowns and assign variables to them (e.g., x, y).
After setting up the equation, solve for the unknowns step by step. Always double-check your calculations to ensure accuracy and consistency with the problem’s context.
By following these steps, you can break down complex word problems into simpler components, making them easier to solve. With practice, this method will become second nature, helping you to solve a wide variety of word problems more efficiently.
Tips for Preparing for Module 4 Exam
Preparing for an exam requires more than just reviewing material; it involves understanding the core concepts, practicing regularly, and adopting effective strategies to ensure you’re fully ready. In this section, we will outline practical tips that will help you approach your studies efficiently and enhance your performance in the upcoming exam.
By following a structured study plan and focusing on key areas of difficulty, you can improve your retention of information and boost your confidence. Whether you’re revising through practice problems or seeking clarification on specific topics, these tips will guide you towards a successful outcome.
1. Understand the Key Topics
Start by identifying the core concepts covered in the exam. Focus on understanding the theory behind each topic and its practical applications. Make sure to:
- Review key formulas: Ensure you’re comfortable using essential formulas in different contexts.
- Practice problem-solving: Work through a variety of problems to strengthen your understanding.
- Clarify doubts: Don’t hesitate to ask questions about anything you don’t fully understand.
2. Create a Study Schedule
A well-organized study schedule is crucial for managing your time effectively. Break your study sessions into manageable chunks, allocating specific times for each topic. Consider the following:
- Prioritize difficult areas: Spend more time on topics that you find challenging.
- Review regularly: Short, frequent study sessions are often more effective than cramming at the last minute.
- Take breaks: Regular breaks can help maintain focus and prevent burnout.
By following these tips and staying consistent in your preparation, you will be well-equipped to tackle the exam with confidence. Practice, review, and stay organized to ensure success.
Examples of Complex Problem Solving
Complex problems often require a systematic approach to break them down into simpler, more manageable parts. By understanding the core principles and using the right techniques, solving intricate challenges becomes a structured process rather than a daunting task. In this section, we will explore examples of such problems and demonstrate how to tackle them efficiently.
These examples will not only highlight common strategies but also offer insight into the logic and methods used to simplify complex situations. Whether dealing with multi-step equations or real-world applications, these examples will provide a deeper understanding of problem-solving techniques.
Example 1: Solving Systems of Equations
One of the common complex problems involves solving systems of equations, where multiple variables are involved. Here’s how you can approach this type of problem:
- Step 1: Write down the system of equations.
- Step 2: Use either the substitution method or elimination method to isolate one variable.
- Step 3: Solve the resulting equation for the remaining variable.
- Step 4: Substitute the found value back into one of the original equations to solve for the second variable.
By following these steps, you can solve systems of linear equations and find the values of the unknowns.
Example 2: Word Problem Involving Rates and Proportions
Another example of a complex problem is when you’re dealing with rates and proportions. Here’s an approach to solve such a problem:
- Step 1: Identify the key quantities involved (e.g., time, distance, speed).
- Step 2: Set up the proportion or rate equation based on the relationships between the quantities.
- Step 3: Solve for the unknown by cross-multiplying or using algebraic manipulation.
- Step 4: Check your solution by substituting it back into the context of the problem to ensure it makes sense.
By following this method, you can solve problems involving rates, such as speed or work, and determine unknown quantities accurately.
Through these examples, we can see how breaking down complex problems into systematic steps can lead to clear and correct solutions. Practicing these methods will strengthen your problem-solving skills and prepare you for even more challenging tasks.
Breaking Down Difficult Problems
Complex problems often appear overwhelming at first, but breaking them down into smaller, more manageable parts can make the process of solving them much easier. Instead of trying to tackle the entire problem at once, the key is to focus on individual components, systematically simplifying each step. This approach not only makes difficult problems less intimidating but also helps in finding solutions more efficiently.
The process involves identifying the core elements of the problem, determining the relationships between them, and applying the right strategies for each part. By taking this step-by-step approach, you can ensure a more structured and logical solution to even the most challenging tasks.
Step 1: Identify Key Information
The first step is to extract all relevant information from the problem. This involves recognizing the given data and understanding what is being asked. Pay close attention to the details, such as numbers, conditions, and constraints, as they provide the foundation for solving the problem.
- Highlight known values: Mark the numbers or variables provided.
- Determine unknowns: Identify what you need to find.
- Understand relationships: Look for connections between values or variables.
Step 2: Simplify the Problem
After identifying the key information, the next step is to simplify the problem. Often, this means breaking down a complex question into simpler sub-problems that can be solved independently. You can use various techniques such as:
- Substitution: Replacing variables with known values or expressions to reduce complexity.
- Drawing diagrams: Visual representations can clarify relationships between variables or quantities.
- Approximations: When exact solutions are difficult, approximate values can be used to simplify the process.
By breaking down the problem into smaller pieces, you can solve each part one at a time, leading to a clear and accurate solution. This method not only enhances your problem-solving skills but also builds confidence in tackling even the most complex challenges.
Exploring Different Solution Strategies
When solving complex problems, there isn’t always a single path to the solution. Depending on the nature of the question and the available information, different strategies can be employed to arrive at an answer. Exploring a variety of approaches not only helps in finding the most efficient method but also deepens your understanding of the problem. In this section, we’ll examine several strategies that can be applied to different types of problems, showcasing their strengths and ideal use cases.
Each strategy has its own set of advantages depending on the complexity and requirements of the problem. Understanding these approaches will allow you to adapt and choose the best method for any given situation, ensuring that you tackle challenges with confidence.
Strategy 1: Working Backwards
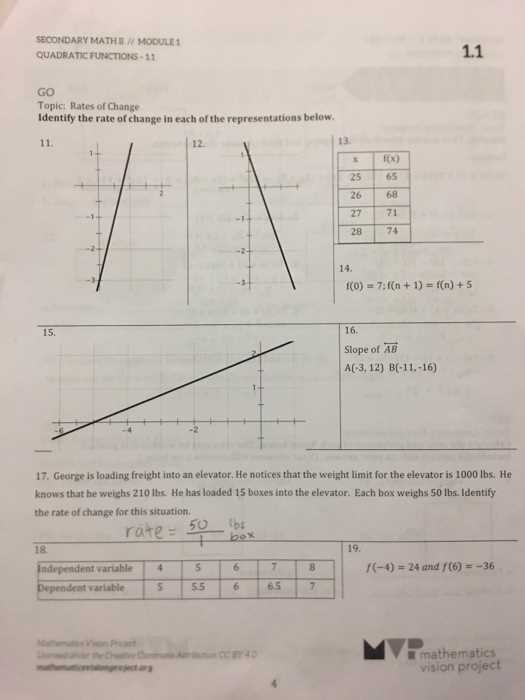
One effective method is working backwards, where you start with the desired outcome and work through the problem in reverse order. This strategy is especially useful when the final result is known but the steps leading to it are unclear. By retracing the steps, you can often uncover the solution.
- Step 1: Identify the end result or target value.
- Step 2: Work backwards by reversing the operations or steps.
- Step 3: Check each step to ensure consistency with the original conditions.
Strategy 2: Using Logical Deduction
Logical deduction involves using known facts and rules to systematically eliminate incorrect possibilities and narrow down the correct answer. This method is particularly effective for problems that require you to make inferences based on a set of conditions or relationships.
- Step 1: List all known facts and conditions.
- Step 2: Apply deductive reasoning to eliminate impossible options.
- Step 3: Continue deducing until you arrive at the solution.
| Strategy | Best For | Advantages |
|---|---|---|
| Working Backwards | When final result is known | Clear and direct path to solution |
| Logical Deduction | Problems with conditions and rules | Helps eliminate wrong answers quickly |
| Trial and Error | When other methods are difficult | Can lead to discovery through experimentation |
By employing these strategies, you can explore multiple angles to find solutions to challenging problems. Understanding when and how to use each method will ultimately enhance your problem-solving toolkit.
Commonly Asked Questions in Module 4
As students work through challenging problems, certain questions tend to arise frequently. These questions often focus on concepts, techniques, or specific steps in solving exercises. Understanding the common doubts that learners encounter can help you better prepare for assessments and improve your problem-solving skills. In this section, we’ll address some of the most frequently asked questions, providing clear explanations to guide you through the learning process.
Question 1: How do I identify the right approach for a problem?
Choosing the correct method to solve a problem can be one of the most challenging aspects of problem-solving. The key is to analyze the question carefully and look for clues that suggest the most appropriate approach. For example, if a problem provides a set of conditions or relationships between values, logical deduction may be the best strategy. If the outcome is already known, working backwards might be a more efficient choice.
- Tip: Break down the problem into smaller parts and identify the key relationships before deciding on a method.
- Tip: Look for familiar patterns in the problem that align with previously solved examples.
Question 2: What if I make a mistake during the problem-solving process?
Making mistakes is a natural part of learning. If you find that your solution doesn’t seem to be working, don’t get discouraged. Instead, step back and review the steps you’ve taken so far. Check for any errors in calculation or logic and retrace your work. Sometimes, simply rephrasing the problem or thinking about it from a different angle can help you spot mistakes and correct them.
- Tip: Double-check your calculations and ensure that all operations follow the correct order of operations.
- Tip: If possible, test your solution by substituting values back into the original equation or scenario.
Question 3: How can I improve my understanding of complex concepts?
Complex concepts often require practice and repetition to fully grasp. To improve your understanding, work through additional practice problems, seek alternative explanations, or discuss the concepts with peers or instructors. Sometimes, visualizing a problem or using real-world examples can make abstract concepts easier to understand.
- Tip: Break down complex concepts into simpler, more manageable components.
- Tip: Review related material to build a stronger foundation before tackling more advanced topics.
By addressing these common questions, you can approach problems with greater confidence and clarity. Remember, consistent practice and understanding the reasoning behind each step are key to mastering difficult concepts.
Visual Aids for Better Understanding
Visual aids are powerful tools that can enhance comprehension, especially when dealing with complex concepts. These tools help to simplify abstract ideas, making them easier to grasp by providing a clear and tangible representation. Using diagrams, charts, graphs, and other visual methods can greatly improve the way students approach problems and retain information. In this section, we’ll explore some of the most effective visual aids that can assist in understanding key concepts and solving difficult exercises.
Diagrams and Graphs
One of the most effective ways to visualize problems is through diagrams and graphs. These tools help to illustrate relationships between different elements, making patterns and trends more apparent. For example, plotting data on a graph can reveal underlying connections that may not be immediately obvious through numbers alone.
- Tip: Use graphs to identify trends, slopes, or intersections that can provide key insights into the problem.
- Tip: Diagrams can be especially helpful when dealing with geometric problems, as they allow you to see shapes, angles, and proportions more clearly.
Flowcharts and Step-by-Step Visual Guides
Flowcharts and step-by-step guides are ideal for breaking down complex processes into manageable steps. These tools can guide you through the sequence of actions required to reach a solution, ensuring that no important step is overlooked. Whether you’re solving an equation or tackling a word problem, these visual aids provide structure and clarity.
- Tip: Break down multi-step problems into smaller sub-problems, and use flowcharts to map out the sequence of operations.
- Tip: Visual guides are helpful when you need to identify the order in which steps should be performed to avoid confusion.
By incorporating these visual aids into your study routine, you can strengthen your problem-solving skills and improve your overall understanding of challenging concepts. Remember, the right visual representation can often make the difference between confusion and clarity, allowing you to approach problems with confidence.
Strategies for Time Management
Effective time management is crucial when tackling any set of problems or preparing for assessments. It involves organizing and planning how to divide your time between various tasks to maximize productivity and ensure that deadlines are met without stress. By implementing the right strategies, you can manage your workload efficiently and avoid procrastination, leading to better results and reduced anxiety during exams.
One key to successful time management is prioritization. Identify the most important or difficult tasks and allocate more time to those first. By addressing challenging problems early, you’ll have more energy and focus to handle them. Breaking down larger tasks into smaller, manageable steps also helps to prevent feeling overwhelmed and keeps progress steady.
- Plan Ahead: Set realistic goals for each study session, focusing on one concept or problem at a time.
- Set Deadlines: Create specific time limits for each task to ensure you stay on track.
- Use a Timer: Implement techniques like the Pomodoro method, where you work for 25 minutes and take a short break, to keep focused and avoid burnout.
Another important strategy is eliminating distractions. Find a quiet, comfortable place to study, and limit access to your phone or other distractions that could disrupt your focus. Establish a routine to improve your efficiency–studying at the same time each day helps to create a habit and boosts productivity.
Finally, make sure to review your progress regularly. Reflect on what you’ve accomplished and adjust your schedule if necessary. Flexibility is key to maintaining a balance between completing tasks and staying motivated.
Exploring the Module 4 Workbook
The workbook for this section serves as a comprehensive tool for reinforcing and practicing the concepts learned throughout the lessons. It is designed to guide you through a variety of exercises, helping to deepen your understanding and improve problem-solving skills. By working through each section, you can apply theoretical knowledge to practical scenarios, building confidence in your abilities.
Structure of the Workbook
The workbook is divided into clear sections, each focusing on specific topics. Each section includes a series of problems that vary in difficulty, enabling you to progress from basic concepts to more complex challenges. The questions are structured to encourage critical thinking, ensuring that you develop both your reasoning and calculation skills.
- Concept Reviews: Before starting the exercises, each section provides a brief summary of the key concepts to help refresh your memory.
- Practice Problems: A range of problems follows, designed to help you apply what you’ve learned in practical contexts.
- Check Your Understanding: After each set of exercises, a review section allows you to assess your progress and identify areas that may need more attention.
Maximizing Your Learning
To get the most out of the workbook, it’s important to approach it strategically. Begin with the easier problems to build your confidence and gradually work towards the more challenging ones. Take your time to understand each question and solution before moving on. If you encounter difficulties, review the related material and attempt similar problems until the concept becomes clear.
- Tip: Don’t rush through the problems. Patience and focus will help you grasp the material more effectively.
- Tip: Use the workbook as a self-assessment tool. Regularly test your understanding and revisit topics as needed to ensure solid retention of the material.
By actively engaging with the workbook and applying the strategies above, you will gain a deeper understanding and be better prepared for any assessment or real-world applications of the material.
Reviewing Key Practice Questions
Effective learning requires a thorough review of essential practice questions that reinforce the core concepts and techniques. By revisiting key exercises, you can test your understanding, identify weak areas, and solidify your knowledge. This section highlights some of the most important problems that will help you master the material and prepare for future challenges.
Identifying Core Problem Types

Throughout the practice section, you will encounter problems designed to cover a wide range of topics. Some problems are straightforward, while others require deeper analysis and multiple steps. The most important thing is to identify the types of problems that appear most frequently in assessments and to become proficient at solving them.
- Problem Type 1: Questions that focus on fundamental concepts and require basic calculations or simple problem-solving techniques.
- Problem Type 2: More complex problems that involve applying multiple concepts simultaneously to arrive at a solution.
- Problem Type 3: Word problems that test your ability to interpret real-world scenarios and translate them into mathematical solutions.
Maximizing Your Review Process
To make the most out of reviewing these key practice questions, focus on understanding the reasoning behind each solution. Don’t just memorize the steps–analyze why each method is used and how the problem is solved. If you make a mistake, take the time to work through it and figure out where you went wrong. This will help you avoid repeating similar mistakes in the future.
- Tip: Break down each question into smaller parts to understand the logic behind each step.
- Tip: After solving a problem, try to come up with a different approach or method to arrive at the same result.
By reviewing these key practice questions and consistently applying problem-solving techniques, you will be better prepared for both assessments and practical application of the material.
How to Double-Check Your Answers
When solving problems, it’s crucial to ensure your solutions are accurate. Double-checking your work not only helps you catch errors but also reinforces your understanding of the steps involved. This section explores effective strategies to verify your results and build confidence in your work.
Reviewing the Process
Start by reviewing the steps you took to solve the problem. Check each stage to ensure you followed the correct method. Ask yourself whether you made any assumptions, skipped any necessary steps, or misapplied a concept. Ensuring each step is logically sound can help you spot mistakes early.
- Tip: Work through the problem again from the beginning, focusing on each part carefully.
- Tip: Cross-check with different methods or approaches, if possible, to see if the outcome aligns.
Using Estimations
Another effective technique is using estimation to quickly check if your answer seems reasonable. If the problem involves numbers, approximate the solution and see if it aligns with your final answer. For example, if you’re solving a large multiplication, rounding the numbers can help you quickly spot discrepancies.
- Tip: If your answer is significantly different from your estimate, review your calculations.
- Tip: Use estimation to identify potential mistakes in your logic or computational steps.
By employing these strategies, you can increase your accuracy and avoid errors, ensuring that your solutions are both correct and well-understood.
Building Confidence with Practice
Mastering any subject requires consistent practice and repetition. By tackling various problems, learners can strengthen their skills, reduce anxiety, and build confidence in their abilities. This section focuses on how regular practice can lead to improved performance and a better understanding of concepts.
Start with Simple Exercises
Begin with straightforward problems to build a solid foundation. These exercises help reinforce basic concepts and set the stage for more challenging tasks. Gradually increasing the difficulty level allows you to tackle harder problems with greater confidence as your skills improve.
- Tip: Focus on mastering the fundamentals before moving on to advanced topics.
- Tip: Repeat simpler problems multiple times to internalize key concepts.
Track Your Progress
Keeping track of your practice sessions allows you to monitor your growth over time. By noting your strengths and areas for improvement, you can focus your efforts on challenging areas. Tracking progress also provides motivation, as you see how far you’ve come.
- Tip: Set small, achievable goals for each practice session.
- Tip: Reflect on your mistakes to learn from them and avoid repeating them in the future.
With continued practice, you’ll become more proficient, making it easier to tackle complex problems and perform well in assessments. Confidence grows as you experience success with each exercise, making learning a more rewarding experience.
Resources for Additional Study
For students looking to deepen their understanding and gain further mastery over the subject, supplementary resources can be a great help. Whether you prefer online tutorials, textbooks, or interactive platforms, there are various tools available to support continued learning. This section highlights some useful options to consider in your study journey.
Online Platforms and Websites
Numerous websites provide interactive exercises, video tutorials, and forums to discuss complex problems. These resources can complement what is taught in class and offer explanations from different perspectives.
- Khan Academy: Offers free video lessons on a wide range of topics, with quizzes and practice problems.
- Coursera: Provides online courses from universities, often with practical assignments.
- Wolfram Alpha: A powerful computational tool for solving equations and visualizing problems.
Books and Study Guides
Books and study guides can be a great option for those who prefer traditional learning methods. Many study guides offer detailed explanations and step-by-step solutions that can be helpful for revising key concepts and preparing for exams.
- Textbooks: Often provide in-depth theory and examples with practice problems at the end of each chapter.
- Study Guides: Condense important concepts into easy-to-understand summaries and include review questions.
- Workbooks: Offer a hands-on approach with a variety of problems to practice, designed to reinforce learning.
By using these resources, you can supplement your learning, clarify doubts, and further hone your skills. The variety of options available ensures that there’s something for every learning style and preference.