Big Ideas Math Integrated Mathematics 2 Answers

In this section, we will explore various techniques for solving complex problems found in advanced educational courses. Students often face challenges when tackling exercises that require critical thinking and a solid understanding of foundational principles. To help with this, we will guide you through the essential methods and approaches to succeed in these tasks.

By breaking down each problem into manageable steps, you can gain a deeper insight into how to approach and solve even the most difficult questions. With the right tools and mindset, every problem becomes an opportunity to refine your skills and build confidence. This guide will not only support you in finding solutions but will also enhance your overall problem-solving abilities.

Big Ideas Math Integrated Mathematics 2 Answers

In this section, we will examine the essential problem-solving techniques and step-by-step methods for tackling exercises in advanced courses. By exploring practical solutions and approaches, students can improve their ability to solve challenging problems effectively and efficiently. Whether you are preparing for tests or looking to deepen your understanding, mastering these strategies is key to success.

Understanding the Approach

When faced with complex questions, it’s crucial to break them down into simpler, more manageable parts. By focusing on individual concepts and applying proven strategies, students can develop a deeper understanding of the material. The key lies in recognizing patterns and applying logical reasoning to find the correct solutions.

Tips for Effective Problem Solving

One of the most important steps is practicing regularly to reinforce key principles. Through repeated exposure, you will begin to notice familiar patterns, making it easier to navigate future problems. Additionally, seeking out resources and tools that explain the reasoning behind each solution will ensure a stronger grasp of the concepts, leading to greater success in solving similar challenges.

Overview of Integrated Mathematics 2

This section provides an in-depth look into the structure and content of a comprehensive educational course that blends various mathematical topics. Students will encounter a range of concepts, each building upon previous knowledge to create a unified understanding. The course aims to strengthen problem-solving skills while offering a broad perspective on key ideas.

Core Topics Covered

The curriculum focuses on a mix of algebra, geometry, and data analysis, providing students with a well-rounded mathematical foundation. As they progress, learners will explore more advanced concepts, ensuring they have the tools to approach increasingly complex problems with confidence. Below is a breakdown of the primary areas covered in this course:

Topic	Description
Algebra	Exploring expressions, equations, and inequalities to understand relationships between variables.
Geometry	Studying shapes, angles, and the properties of space to solve geometric problems.
Data Analysis	Learning to interpret data sets, calculate probabilities, and make informed conclusions.
Functions	Understanding the behavior of functions and their applications in real-world scenarios.

Learning Goals and Progression

The course is designed to help students master foundational skills before moving on to more advanced topics. Each unit builds logically on the previous one, ensuring a clear progression. As learners advance, they gain confidence in their ability to apply mathematical principles in practical situations.

Key Concepts in Integrated Math 2

This section highlights the essential principles that form the foundation of the course. These core concepts are designed to build a strong mathematical understanding that will enable students to tackle various challenges. By mastering these ideas, learners will enhance their ability to solve problems and apply their knowledge in different contexts.

Among the critical topics are the relationships between variables, the study of geometric shapes and their properties, and the analysis of data. Understanding functions and their behavior is also a central theme, as it connects multiple areas of study. By exploring these ideas in depth, students develop a comprehensive skill set for approaching complex problems.

Additionally, the ability to work with equations, inequalities, and various algebraic expressions is fundamental. These concepts serve as the building blocks for more advanced topics, ensuring that learners have the necessary tools to succeed in future studies.

How to Use the Answer Key Effectively

Knowing how to make the most of a solution guide is essential for reinforcing understanding and improving problem-solving skills. Simply checking your work against the provided solutions isn’t enough; it’s important to analyze the reasoning behind each step to fully grasp the material. By carefully reviewing solutions, students can identify mistakes, understand the correct approach, and build confidence in their abilities.

Steps to Follow When Using the Guide

Here are some strategies for using the solution key in a way that enhances your learning:

• Work through the problems first: Always attempt the exercises before consulting the solutions. This allows you to test your understanding and identify areas where you may need improvement.
• Review step-by-step: Go through each solution in detail, making sure to understand the logic behind every step. If something is unclear, try working through it on your own or seek additional resources.
• Compare different methods: If there are multiple ways to approach a problem, compare your method to the one in the solution guide. This can expose you to new strategies and improve your problem-solving flexibility.
• Take notes: Write down key points or alternative techniques you come across while reviewing the solutions. This reinforces learning and creates a useful reference for future exercises.

Common Pitfalls to Avoid

While the solution guide is a valuable tool, there are several things to be cautious about:

• Relying too heavily on the guide: Using the guide as a crutch can hinder the development of independent problem-solving skills. Use it as a supplementary tool, not a replacement for effort.
• Skipping over explanations: Don’t just look at the final answer–take the time to understand how it was derived. Skipping this step can lead to a shallow understanding of the material.
• Ignoring mistakes: If you make a mistake, analyze where you went wrong and why. Understanding your errors is crucial for improvement.

Step-by-Step Guide to Solving Problems

Approaching problems methodically is key to mastering complex concepts. By breaking down each task into manageable steps, students can reduce the likelihood of mistakes and enhance their understanding of the subject matter. This systematic approach ensures clarity and allows for consistent progress as you work through different types of exercises.

Steps to Approach Each Problem

Follow these steps for effective problem-solving:

1. Understand the question: Carefully read the problem to ensure you know exactly what is being asked. Pay attention to any details that may influence your approach.
2. Identify key information: Highlight or underline important numbers, variables, and conditions that are crucial for solving the problem.
3. Choose an approach: Think about the methods you have learned that could be applied to this problem. Decide whether it’s an algebraic, geometric, or logical approach, depending on the task at hand.
4. Set up the problem: Write down any equations, formulas, or relationships that apply. Organizing the information visually can make it easier to work through the problem.
5. Work through the steps: Solve the problem step by step, checking each stage carefully. Ensure that each step logically follows from the previous one.
6. Double-check your solution: After reaching a solution, go back through the problem and verify that your calculations are correct and that the solution addresses the original question.

Common Strategies for Success

In addition to following a structured approach, these strategies can further improve your problem-solving skills:

• Draw diagrams or graphs: Visualizing the problem can help you better understand relationships between different elements.
• Look for patterns: Identify any recurring trends or relationships that can simplify the problem-solving process.
• Break the problem into smaller parts: If the problem feels overwhelming, divide it into smaller, more manageable sections to tackle one by one.
• Stay organized: Keep your work neat and ordered. This will make it easier to spot mistakes and maintain focus.

Common Challenges in Integrated Math 2

As students progress through their studies, they often encounter obstacles that can make certain concepts more difficult to grasp. These challenges may arise from misunderstandings of fundamental principles or from the complexity of more advanced topics. Recognizing and addressing these common difficulties early on can help students build confidence and improve their problem-solving abilities.

Key Areas of Difficulty

There are several aspects of this course that students often find particularly challenging:

• Algebraic Manipulations: Many students struggle with simplifying expressions, solving equations, and working with inequalities. Mastery of these foundational skills is critical for tackling more complex problems.
• Understanding Functions: The concept of functions–how inputs relate to outputs–can be tricky. Identifying the correct function type and applying it correctly requires practice and a solid understanding of their behavior.
• Geometric Proofs: Constructing and understanding geometric proofs can be confusing, especially when students are required to connect various properties of shapes and theorems in logical sequences.
• Word Problems: Translating real-world scenarios into mathematical expressions or equations is often difficult. Students may struggle with identifying the right information and forming the correct relationships.
• Data Analysis and Interpretation: Working with data sets, calculating averages, and interpreting results can be overwhelming for some students. The key is to develop strong analytical skills and a clear understanding of statistical concepts.

Strategies for Overcoming Challenges

Here are some helpful strategies for overcoming these common difficulties:

• Practice Regularly: Consistent practice is essential for mastering complex concepts. By working through different types of problems, students can reinforce their skills and improve their understanding.
• Seek Help When Needed: Don’t hesitate to ask for clarification when a concept is unclear. Whether from a teacher, tutor, or online resources, additional explanations can make a significant difference.
• Break Down Problems: When faced with a challenging problem, break it into smaller, more manageable parts. Solve each part step by step to avoid feeling overwhelmed.
• Use Visual Aids: Diagrams, graphs, and charts can help clarify complex relationships and make abstract concepts more tangible.
• Review Mistakes: Go over any errors to understand what went wrong. Learning from mistakes is crucial for improving your overall performance.

Tips for Mastering Math Problems

To truly excel at solving problems, it’s essential to approach each challenge with both strategy and understanding. Building proficiency requires consistent practice, developing a deep understanding of the principles involved, and honing problem-solving techniques. By following certain tips and guidelines, students can significantly improve their ability to tackle a wide range of exercises with confidence.

Effective Strategies for Success

Here are several key tips for mastering complex tasks:

• Focus on Understanding Concepts: Rather than memorizing formulas or steps, strive to understand the underlying concepts. This approach will allow you to apply knowledge in various situations and adapt to new problems.
• Work Through Examples: Go through solved examples step by step to understand the logic behind each solution. This will provide a clear model for approaching similar problems on your own.
• Practice Regularly: Consistent practice is one of the most effective ways to build skills. The more problems you solve, the more familiar you will become with the various strategies and techniques needed.
• Break Problems into Smaller Steps: When faced with a difficult problem, divide it into smaller, more manageable parts. Solve each part individually before combining them to reach the final solution.
• Ask Questions: Don’t hesitate to ask for help if something is unclear. Whether from a teacher, tutor, or online resource, asking questions helps clarify confusion and leads to better understanding.

Common Pitfalls to Avoid

While mastering problem-solving, be aware of these common mistakes:

• Rushing Through Problems: Take your time to carefully read and understand each question. Rushing can lead to overlooked details and incorrect answers.
• Skipping Steps: Ensure you follow each step of the process. Skipping steps can result in errors, especially in complex problems that require careful calculations.
• Not Reviewing Work: Always double-check your solutions. Even small errors can lead to incorrect results, so reviewing your work can prevent mistakes from going unnoticed.

Understanding the Problem-Solving Process

Effectively solving problems requires more than just applying formulas or memorized steps. It involves a deep understanding of the task at hand, the ability to think critically, and the skill to break down complex situations into manageable parts. By following a structured process, individuals can approach problems more confidently and systematically, leading to more accurate and efficient solutions.

Key Steps in Problem-Solving

The problem-solving process can be broken down into a series of essential steps:

• Identify the Problem: Begin by clearly understanding what the problem is asking. Read the question carefully and ensure you know exactly what needs to be solved.
• Gather Relevant Information: Collect any data, variables, or conditions that are provided within the problem. Identifying key pieces of information is crucial for formulating the right approach.
• Choose a Strategy: Based on the problem’s nature, select the appropriate method or approach. This might involve algebraic manipulation, logical reasoning, or visual representation like graphs or diagrams.
• Work Through the Steps: Solve the problem step by step, ensuring each action is logical and accurate. Keep track of any intermediate steps and check your calculations as you go.
• Verify the Solution: Once you reach a solution, review the problem and your steps to confirm that the solution answers the original question. Double-checking helps to avoid errors and ensures accuracy.

Effective Strategies for Improving Problem-Solving Skills

To further enhance your ability to solve problems, consider the following strategies:

• Practice Regularly: The more you practice solving problems, the more comfortable you will become with identifying patterns and applying the right techniques.
• Learn from Mistakes: Mistakes are an inevitable part of the learning process. Take time to understand why an error occurred and how to avoid it in the future.
• Stay Organized: Keeping your work organized helps maintain clarity and reduces the likelihood of making mistakes. Write down every step and keep calculations neat and clear.
• Work with Others: Collaborating with peers can provide fresh perspectives on solving problems and help reinforce your understanding through discussion.

Breaking Down Complex Math Questions

When faced with a challenging problem, it can often feel overwhelming. However, the key to solving these complex questions lies in breaking them down into smaller, more manageable parts. By deconstructing the question and addressing each element individually, you can simplify the process and make progress step by step. This approach not only helps in finding solutions more efficiently but also builds a deeper understanding of the concepts involved.

Steps for Decomposing Complex Problems

To effectively tackle difficult questions, follow these steps:

• Analyze the Question: Carefully read through the problem to understand what is being asked. Highlight important information and identify what needs to be solved.
• Identify Key Components: Break down the question into smaller, more digestible pieces. Look for equations, relationships, or patterns that can help guide you toward the solution.
• Separate Different Topics: If the question involves multiple concepts (such as algebra, geometry, or data analysis), focus on each one individually. Solve the components before integrating them for a final solution.
• Use Logical Steps: Work through the problem logically and step-by-step. Avoid jumping ahead or skipping steps, as this can lead to errors.

Example: Breaking Down a Complex Problem

Let’s look at an example of how to break down a complex problem. The following table shows the breakdown of a word problem into smaller steps:

Step	Action
Step 1	Read the problem carefully to understand what is being asked.
Step 2	Identify key values and variables in the problem.
Step 3	Formulate an equation or relationship based on the information provided.
Step 4	Solve the equation or relationship, step by step.
Step 5	Verify the solution by checking if it satisfies the original question.

By following these steps, you can transform a complex question into a series of more manageable tasks, ultimately making the problem easier to solve.

How to Approach Word Problems

Word problems often seem intimidating at first, but they can be tackled successfully by applying a methodical approach. The key is to break the problem down into manageable steps, identify the relevant information, and translate it into mathematical expressions or logical reasoning. By focusing on understanding the context and systematically working through each part of the problem, you can develop a clear path to the solution.

Here are some steps to help approach word problems effectively:

• Read Carefully: Begin by reading the problem in its entirety. Pay close attention to the details, as they often contain crucial information needed to solve the problem.
• Identify the Question: Determine exactly what the problem is asking. Highlight or underline the question to keep it in focus as you work through the solution.
• Extract Key Information: Identify the important numbers, values, or relationships mentioned in the problem. Look for clues that can be used to form equations or find unknowns.
• Plan the Solution: Think about how to approach the problem. Consider which methods or strategies will be most effective–whether it’s setting up an equation, drawing a diagram, or using logical reasoning.
• Solve the Problem: Use the method you’ve chosen to solve the problem. Work through each step carefully, checking for accuracy as you go.
• Check Your Answer: Once you arrive at a solution, review the problem and your work to ensure that the answer makes sense and answers the original question.

By following these steps and practicing regularly, you’ll become more comfortable with word problems and develop the skills needed to solve them with confidence and accuracy.

Important Formulas to Remember

When solving problems, having a set of key formulas at your disposal can make a significant difference in efficiency and accuracy. These formulas serve as essential tools that help to simplify complex calculations and provide quick solutions to common types of problems. By understanding and memorizing these formulas, you can improve your problem-solving abilities and approach various topics with greater confidence.

Basic Algebraic Formulas

Algebra is fundamental to many types of problems. Some basic formulas include:

• Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a (Used to solve quadratic equations)
• Slope Formula: m = (y₂ – y₁) / (x₂ – x₁) (Used to find the slope of a line between two points)
• Point-Slope Form of a Line: y – y₁ = m(x – x₁) (Used to express the equation of a line when the slope and a point are known)

Geometry and Measurement Formulas

Geometry often requires understanding basic area and volume formulas. These are key for working with shapes and solids:

• Area of a Triangle: A = 1/2 * base * height
• Area of a Circle: A = π * r² (Where r is the radius)
• Volume of a Cylinder: V = π * r² * h (Where r is the radius and h is the height)

These formulas are only a small sample, but they cover some of the most common situations you’ll encounter. By mastering these and others, you’ll be well-equipped to approach a variety of problems across different topics with ease.

How to Verify Your Answers

Verifying your solution is a crucial step in the problem-solving process. After completing a problem, it’s important to confirm that the solution is correct and meets the conditions set by the question. This ensures accuracy and prevents mistakes that can go unnoticed in complex problems. Verification can be done in several ways, depending on the type of problem you are solving.

Check by Substitution

One of the most effective ways to verify your answer is to substitute the solution back into the original equation or problem statement. If the solution satisfies all conditions, it is likely correct. For example, if you solved an equation, substitute the value of the variable back to check if both sides of the equation are equal.

• For algebraic problems: Substitute the value of the variable into the original equation and check if both sides are equal.
• For geometry problems: Check if the calculated area or volume matches the expected result for a given shape.

Use Reverse Operations

Another way to verify your work is by using reverse operations. This means retracing your steps but in the opposite direction. For example, if you solved for a variable by multiplying both sides of an equation, try dividing both sides by the same factor to see if you return to the original equation.

• For algebraic operations: Apply reverse operations, such as solving for x by isolating terms, and check if the original equation is restored.
• For word problems: Reread the problem and substitute your answer back to ensure it satisfies the conditions given in the context of the problem.

By applying these verification methods, you can feel more confident in the accuracy of your solutions and avoid unnecessary errors. It’s also a helpful practice for reinforcing concepts and improving problem-solving skills.

Using Online Resources for Assistance

When tackling challenging problems, online resources can be a valuable aid in gaining clarity and reinforcing understanding. These resources provide explanations, examples, and tools that help break down complex concepts into manageable steps. By utilizing trusted websites, tutorials, and forums, learners can receive support and additional perspectives to guide them through difficult tasks.

Online Tutorials and Videos

One of the most effective ways to supplement your learning is through video tutorials and step-by-step guides. Platforms like YouTube, educational websites, and math-specific sites offer visual explanations that make difficult concepts easier to grasp. Videos can walk you through problems at your own pace, offering both visual and auditory cues to deepen your comprehension.

• Look for visual guides: These can help demonstrate processes such as graphing, solving equations, or simplifying expressions.
• Pause and replay: Videos allow you to revisit specific steps or concepts that may require further clarification.

Interactive Tools and Forums

Interactive online tools, such as graphing calculators, equation solvers, and problem generators, offer practical help in solving problems. These tools let you experiment with different values and see the outcomes instantly, enhancing your understanding of how formulas and operations work in real-time.

• Graphing tools: Websites like Desmos allow you to visualize equations and explore solutions graphically.
• Community forums: Joining online discussion platforms like Stack Exchange or Reddit can connect you with peers and experts who can offer guidance or explain tricky concepts.

By integrating online resources into your study routine, you can access a wealth of information and support that enhances your learning experience and improves problem-solving efficiency. These tools can provide both immediate assistance and long-term knowledge, making them an indispensable part of any student’s toolkit.

Strategies for Test Preparation

Preparing for assessments requires a strategic approach that emphasizes understanding key concepts, practicing problems, and managing time effectively. A well-organized study plan, combined with focused review sessions, can significantly improve performance. By using proven techniques, you can not only retain essential information but also gain confidence in applying it during the test.

One of the most effective ways to prepare is to break down your study sessions into manageable segments. This approach ensures that you cover all topics without feeling overwhelmed, while allowing time for review and practice. Additionally, incorporating active recall techniques–such as testing yourself on various problems–helps reinforce learning and enhances memory retention.

Focus on Problem Areas

Identify and dedicate extra time to the topics or types of problems you find most challenging. This focused approach helps ensure that you are not just reviewing familiar material but actively addressing your weaker areas. Regular practice of these difficult concepts will improve your problem-solving skills and increase your ability to handle similar questions on the test.

• Review class notes: Go through your notes and textbooks, paying special attention to topics that have been consistently difficult for you.
• Seek clarification: If there are still concepts that confuse you, don’t hesitate to ask a teacher, tutor, or use online resources for further explanation.

Simulate Test Conditions

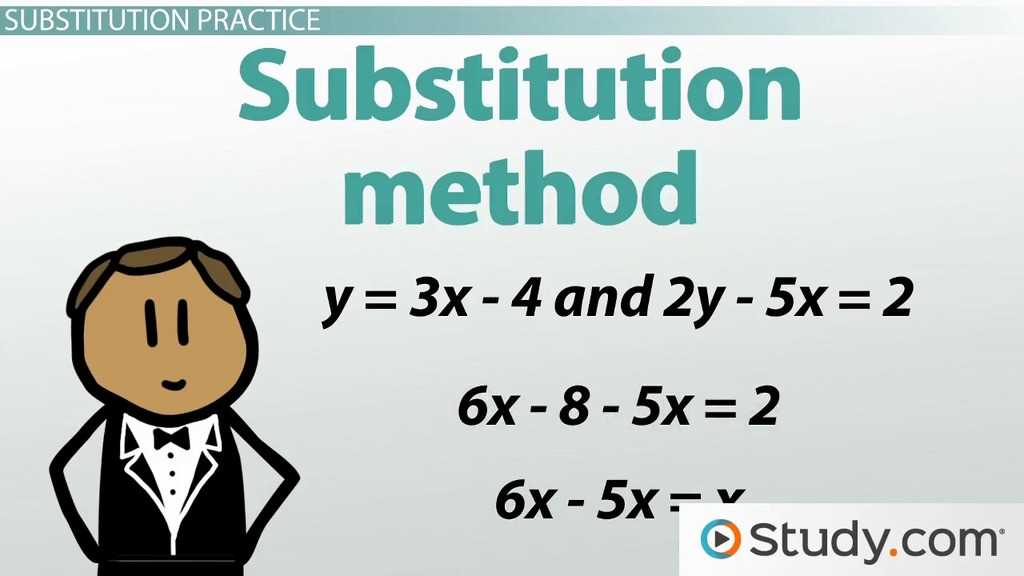
Simulating real test conditions can help you become accustomed to the time constraints and pressure of an actual exam. Set a timer and work through practice problems or past test papers, paying attention to the time you spend on each question. This practice can help improve your time management skills and reduce anxiety on test day.

• Use past exams: Completing past assessments allows you to become familiar with the format and types of questions likely to appear.
• Take timed practice tests: Mimic exam conditions by setting a time limit for each section or problem set, which will improve your pacing.

By implementing these strategies, you can approach the test with a clearer understanding of the material, increased confidence, and a better ability to manage your time. Consistent preparation, along with targeted practice and problem-solving, is key to achieving success.

Benefits of Practice and Review

Regular practice and thorough review are essential components of mastering any subject. By consistently engaging with the material, learners strengthen their understanding and retain crucial concepts. This approach not only enhances knowledge but also boosts confidence and problem-solving skills, enabling individuals to perform better under pressure.

When you make a habit of revisiting problems, whether through exercises or assessments, you begin to recognize patterns and improve your ability to apply concepts in various contexts. Repetition is key to building long-term retention, as it helps transfer information from short-term to long-term memory. Furthermore, reviewing past work enables you to identify areas that require additional focus, allowing for more effective study sessions.

Improves Retention and Understanding

Engaging with problems over time helps reinforce previously learned material, ensuring that the information stays fresh in your mind. Whether revisiting solved problems or attempting new ones, practice reinforces your ability to recall concepts quickly and accurately. It also enables a deeper understanding, as repetition encourages you to approach problems from different angles and grasp underlying principles more clearly.

• Revisit difficult topics: Take the time to go over areas where you struggled in the past to improve your comprehension.
• Practice consistently: Make practice a regular part of your routine to solidify concepts and increase your speed in solving problems.

Builds Confidence and Reduces Anxiety

As you practice more, you become more familiar with the material, which reduces anxiety during tests or exams. Knowing that you’ve already tackled similar problems gives you the confidence to approach the test with a clearer mindset. Regular practice allows you to handle challenges effectively and improves your ability to think critically under time constraints.

• Familiarize yourself with the format: By solving practice problems that mimic test conditions, you gain confidence in handling the actual exam.
• Track your progress: Reviewing your past work helps you see improvement over time, reinforcing your belief in your capabilities.

Incorporating regular practice and review into your study routine is a proven strategy for mastering difficult concepts and excelling in any assessment. With patience and consistency, you will find yourself better prepared, more confident, and equipped to tackle even the most challenging problems.

Advanced Topics in Integrated Math 2

As students progress through their studies, they encounter more complex concepts that require a deeper understanding of earlier principles. These advanced topics are designed to challenge learners, pushing them to apply what they’ve learned in new and more sophisticated ways. By mastering these concepts, students can develop critical thinking skills and gain the tools needed for success in higher-level courses.

Advanced concepts build on fundamental knowledge but require the student to approach problems with greater depth and sophistication. From analyzing data to understanding complex functions and geometric relationships, these topics demand both creativity and analytical thinking. Mastering these areas is crucial for anyone wishing to pursue further studies in related fields such as engineering, economics, or advanced science.

Complex Functions and Their Applications

One of the core advanced topics involves understanding complex functions and their uses. These include functions with more intricate transformations and applications that may not be immediately obvious at first glance. Students will explore various types of functions such as quadratic, exponential, and logarithmic, learning to analyze their behavior and how they apply in real-world scenarios.

• Transformations of functions: Learn to apply shifts, stretches, and reflections to functions to better understand their behavior.
• Exponential and logarithmic relationships: These functions are foundational for solving real-life problems involving growth and decay, such as population studies and finance.

Advanced Geometry and Trigonometry

Another key area of advanced study is geometry and trigonometry, particularly as it relates to analyzing complex shapes, solving for unknown angles, and understanding spatial relationships. These topics explore the intricacies of triangles, circles, and polygons, and are essential for fields such as architecture, physics, and engineering.

1. Trigonometric identities and equations: Students will delve into the unit circle, sine, cosine, and other trigonometric functions, learning how to manipulate and apply these identities.
2. Geometric proofs and reasoning: Advanced geometry involves constructing rigorous proofs to establish the properties and relationships between geometric figures.

Mastering these advanced topics requires a strong grasp of both the basics and the ability to think critically and analytically. With practice and continued learning, students will be equipped to tackle complex problems and prepare for future academic challenges.