
When preparing for a comprehensive assessment in mathematics, having a clear understanding of the concepts covered is essential. This section will guide you through essential problem-solving techniques, offering detailed insights and solutions to commonly encountered questions. Whether you are reviewing for a school test or reinforcing your knowledge, this material provides valuable support in navigating key topics.
By working through various exercises and examples, students can gain a deeper grasp of the core principles. The following content will help break down complex problems into simpler steps, making it easier to approach similar challenges with confidence. Clear explanations and step-by-step guidance aim to improve both understanding and performance in mathematics assessments.
Mathematics Assessment Solution Guide
This section offers a comprehensive guide to the solutions for a typical mathematics assessment. Here, we provide well-organized solutions to problems commonly found in such tests. The goal is to give you the tools to understand the process behind each solution, ensuring that you not only get the correct result but also understand the reasoning behind it.
Step-by-Step Problem Breakdown
Each solution is broken down into manageable steps, making it easier to follow and replicate. By reviewing these steps, you can better prepare for similar tasks in the future. This method also helps identify the logical connections between different mathematical concepts, reinforcing your overall understanding.
Key Strategies for Effective Problem Solving
In addition to providing the solutions, this section highlights key strategies for approaching common challenges. These strategies aim to improve your problem-solving efficiency and accuracy, allowing you to tackle complex questions with confidence.
Overview of Mathematics Assessment
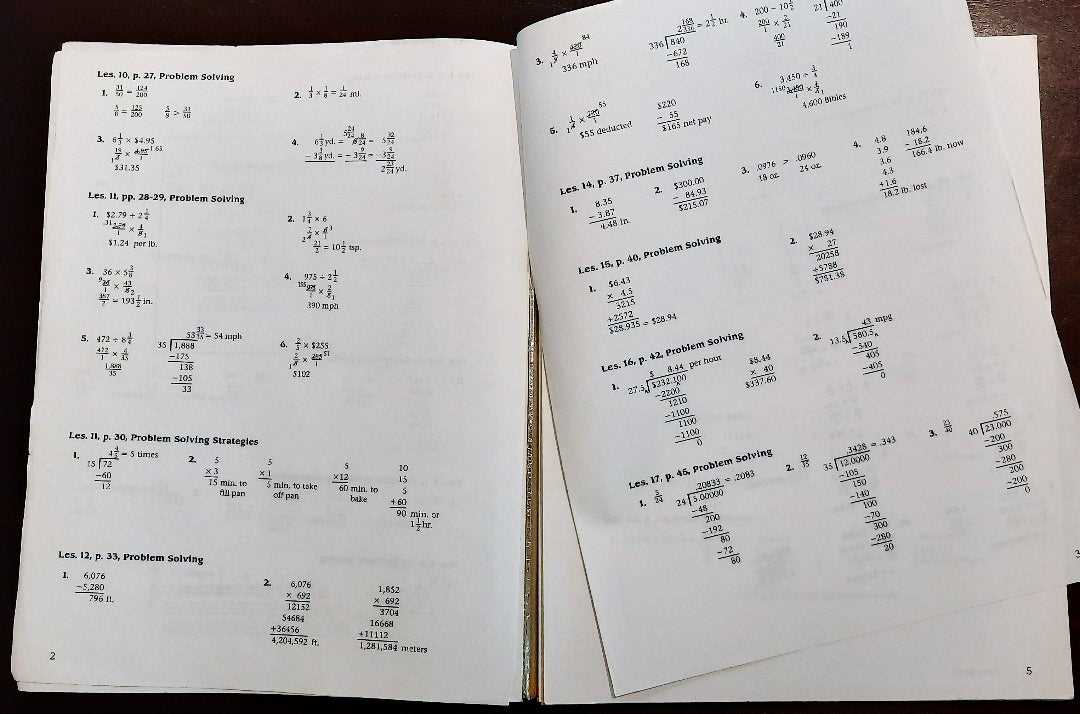
This section provides a broad understanding of the structure and content typically covered in a mathematics assessment. It aims to help students grasp the types of questions they may encounter, the areas of focus, and how to approach them for optimal performance. The focus is on foundational concepts that serve as the basis for higher-level studies.
Core Topics Covered
In most assessments, the questions are designed to test knowledge across several key areas. Below are some of the primary concepts typically included:
- Basic operations with numbers
- Solving simple equations
- Understanding patterns and sequences
- Working with fractions and decimals
- Interpreting word problems
Preparation Tips for Success
Effective preparation for this type of assessment involves focusing on understanding core principles and practicing a variety of problems. Consider the following tips to enhance your readiness:
- Review key concepts regularly
- Practice with a variety of sample problems
- Work through problems without relying on calculators
- Understand the reasoning behind solutions, not just the results
- Manage time effectively during practice sessions
How to Use the Solution Guide
This section will walk you through how to effectively utilize the provided solutions for your practice problems. The purpose of this guide is not only to provide the correct answers but to help you understand the methods used to reach them. By studying these solutions, you can gain insight into the steps required to solve similar problems independently.
Step-by-Step Review
Each solution includes a detailed breakdown of the steps involved. Begin by reviewing the problem, then follow the logical sequence outlined in the solution. This process will help you grasp how each step contributes to the final result and how you can apply the same techniques to different questions.
Learn from Mistakes

As you compare your solutions to those provided, take note of any differences. If you made an error, carefully analyze where the mistake occurred and understand why the correct approach works. This reflective process helps solidify your understanding and prevents future errors in similar problems.
Understanding Key Mathematical Concepts
This section aims to help you grasp the foundational principles that are essential for solving a wide range of mathematical problems. By focusing on the core ideas, you can build a strong understanding that will enable you to tackle more complex challenges. These fundamental concepts form the backbone of many types of problems you may encounter.
Fundamental Operations

At the heart of most mathematical problems lies the ability to work with basic operations such as addition, subtraction, multiplication, and division. Mastering these operations is crucial for solving more advanced problems. Understanding the relationship between these operations and how to manipulate them in different contexts will help you become more proficient.
Equations and Relationships
Another key concept involves understanding how to work with relationships between different quantities. This includes solving for unknowns in simple expressions and recognizing how to isolate variables. Knowing how to balance equations and apply logical steps to both sides of an equation is vital for ensuring accuracy in your solutions.
Common Mistakes in Mathematics
Even experienced learners can make mistakes while solving mathematical problems. These errors often arise from misunderstanding the concepts, overlooking details, or rushing through steps. Identifying common pitfalls can help you avoid them and improve your accuracy in solving problems.
Typical Errors to Watch For
Here are some of the most frequent mistakes made during problem-solving:
- Misapplying basic operations, especially with negative numbers
- Forgetting to distribute terms properly in expressions
- Confusing the order of operations when multiple steps are involved
- Incorrectly simplifying fractions or decimals
- Making calculation errors due to lack of attention to detail
How to Avoid These Mistakes
To reduce errors, take the following steps:
- Double-check each calculation and operation before moving on
- Review the rules for each mathematical operation carefully
- Break complex problems into smaller, more manageable parts
- Use a methodical approach, ensuring all steps are followed in order
- Practice regularly to become more familiar with common problem types
Step-by-Step Solution Examples
This section provides detailed examples of how to solve common mathematical problems. Each example is broken down into individual steps, allowing you to follow along and understand the process behind each solution. By carefully studying these examples, you can learn to approach similar problems with confidence and accuracy.
For each problem, the solution will be presented in a logical sequence, showing how to manipulate the given information and arrive at the correct result. This method helps clarify the reasoning behind each step and ensures you are prepared to solve a variety of questions effectively.
How to Prepare for the Assessment
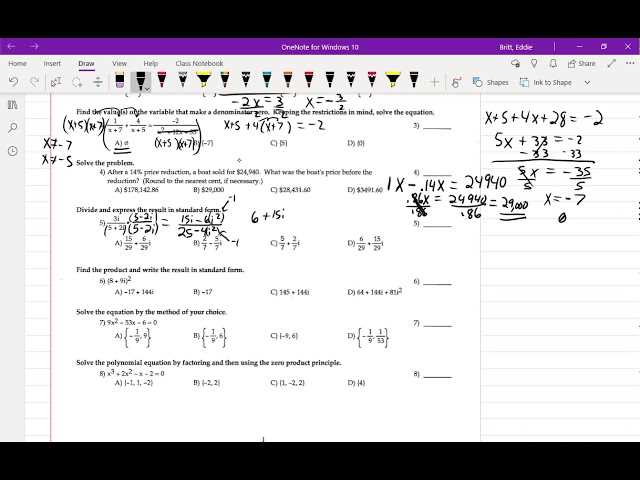
Effective preparation is crucial to achieving success in any mathematical assessment. By developing a structured study plan, reviewing core concepts, and practicing problem-solving techniques, you can strengthen your skills and approach the test with confidence. In this section, we will explore methods to maximize your preparation and ensure you are fully ready.
Study Plan and Time Management
One of the most effective ways to prepare is to create a study plan that focuses on key areas where you need improvement. Prioritize topics based on their importance and your current understanding. Allocate enough time to review each concept, and set small, achievable goals to track your progress.
| Day | Focus Area | Time Spent |
|---|---|---|
| Day 1 | Basic Operations and Fractions | 2 hours |
| Day 2 | Solving Equations | 2 hours |
| Day 3 | Word Problems and Sequences | 2 hours |
| Day 4 | Review and Practice | 3 hours |
Practice and Review Techniques
Once you’ve outlined your study schedule, the next step is to actively engage with practice problems. Focus on problems that challenge you the most, and ensure that you fully understand the solutions. Regular review sessions will help reinforce key concepts and improve your retention.
Reviewing Basic Mathematical Operations
Mastering fundamental operations is essential for solving a wide range of problems. These basic skills serve as the foundation for more complex tasks, so it’s crucial to practice them until they become second nature. In this section, we’ll focus on the core operations that you will frequently encounter in mathematical assessments, providing a solid grounding in each.
Understanding Addition and Subtraction
At the heart of arithmetic are addition and subtraction. These operations are the building blocks for many mathematical tasks. Understanding how to add and subtract efficiently is crucial, as these skills are used in everything from balancing equations to solving word problems.
Multiplication and Division
Once you’ve mastered addition and subtraction, the next logical step is to understand multiplication and division. These operations are essential for working with larger numbers and for performing tasks that involve scaling or breaking things into equal parts. Mastery of these operations will make more advanced mathematical work much easier.
Algebraic Expressions Explained
Understanding how to work with mathematical expressions is a fundamental skill in solving a variety of problems. These expressions often combine numbers, variables, and operations to represent relationships between different quantities. By learning how to simplify, evaluate, and manipulate these expressions, you gain the ability to tackle a wide range of mathematical challenges.
Each expression can be thought of as a formula or a shorthand for a set of operations. Mastering the components of these expressions–such as constants, variables, and operators–helps you recognize how they can be simplified or manipulated to solve problems more effectively. Whether dealing with simple or complex expressions, this knowledge forms the basis for working with equations and inequalities.
Common Questions on the Assessment
When preparing for a mathematical assessment, students often encounter recurring types of questions. These questions typically test the understanding of core concepts and the ability to apply mathematical operations in various contexts. By familiarizing yourself with the most common question types, you can better prepare for the challenges of the test.
Frequently Asked Question Types
Here are some of the most common types of problems that tend to appear in assessments:
- Basic operations with numbers, including addition, subtraction, multiplication, and division
- Simplifying expressions and evaluating them with given values
- Solving simple equations involving one or more variables
- Word problems that require the application of formulas or equations
- Identifying patterns and completing number sequences
Strategies for Answering These Questions
To approach these types of questions effectively, consider the following tips:
- Read the question carefully to ensure you understand the requirements
- Break down the problem into smaller, manageable steps
- Apply the appropriate mathematical operation for each part of the problem
- Double-check your calculations to avoid simple errors
- Use the process of elimination when working with multiple-choice questions
Importance of Practice Problems
Consistent practice is essential for mastering mathematical concepts and improving problem-solving skills. By working through a variety of practice problems, you reinforce your understanding and build the confidence needed to approach different types of tasks. Practicing regularly helps you recognize patterns, identify common challenges, and develop strategies to solve problems more efficiently.
In this section, we will explore the key benefits of solving practice problems and how they contribute to better preparation and performance. The more problems you tackle, the more you become accustomed to different problem structures and the more proficient you become in applying the right techniques.
| Benefit | Explanation |
|---|---|
| Improved Accuracy | Regular practice helps reduce errors by making you familiar with the correct steps and procedures. |
| Faster Problem Solving | Repeatedly working on similar problems allows you to develop speed and efficiency in finding solutions. |
| Better Understanding of Concepts | Hands-on practice reinforces theoretical knowledge and aids in translating concepts into real-world applications. |
| Confidence Boost | Successfully completing practice problems boosts self-assurance, making you more prepared for assessments. |
Strategies for Solving Word Problems
Word problems can often seem intimidating due to the complexity of translating a written scenario into a mathematical equation. However, with the right approach, you can simplify these challenges and solve them with ease. The key to tackling word problems is understanding the information provided and identifying the mathematical operations required to find the solution.
By following a structured method, you can break down the problem into manageable parts and systematically work through it. This will not only help you find the correct solution but also improve your ability to approach similar problems in the future.
Step-by-Step Approach
To solve word problems effectively, follow these steps:
- Read the problem carefully: Take time to understand all the details, ensuring you don’t miss any key information.
- Identify the question: Focus on what the problem is asking you to find and highlight the important data.
- Translate the words into math: Convert the text into a mathematical equation by identifying operations such as addition, subtraction, multiplication, or division.
- Solve the equation: Apply the necessary steps to find the solution.
- Double-check your work: Ensure that the answer makes sense in the context of the problem.
Common Pitfalls and How to Avoid Them
Even when following a structured approach, certain mistakes can occur. Common pitfalls include:
- Missing key details: Sometimes, essential information is hidden within the text or presented in a tricky way. Take your time to identify all variables.
- Using incorrect operations: Carefully consider which mathematical operation fits the situation. For example, using addition when subtraction is needed can lead to an incorrect result.
- Rushing through the steps: Word problems require thoughtful attention. Avoid skipping steps, as it may lead to errors.
Tips for Time Management on the Exam
Efficiently managing your time during a test is crucial for maximizing your performance. Proper time allocation ensures that you have enough opportunity to answer each question, check your work, and avoid unnecessary stress. By planning ahead and staying focused, you can handle even the most challenging sections with confidence.
The key to effective time management is preparation and strategy. Prioritize tasks, pace yourself, and remain calm to ensure you make the most of your time in the testing environment.
Here are some practical tips to help you manage your time effectively:
- Know the test format: Familiarize yourself with the structure and types of questions you will face. This will allow you to anticipate how much time to allocate for each section.
- Start with the easy questions: Begin by answering the questions you find easiest. This boosts your confidence and ensures you score points quickly.
- Set time limits: Allocate a specific amount of time for each question or section. Stick to these limits to prevent spending too much time on one problem.
- Move on when stuck: If you encounter a question that seems particularly difficult, move on to the next one. You can always come back to it later with fresh perspective.
- Leave time for review: Set aside a few minutes at the end to review your work. This can help you catch any mistakes or overlooked details.
By following these strategies, you can effectively manage your time, reduce anxiety, and ensure a more successful testing experience. Remember, time management is a skill that improves with practice and experience.
Understanding Equations and Inequalities
Equations and inequalities are fundamental concepts in mathematics that form the basis for solving a wide range of problems. Both involve relationships between variables, but they express different types of conditions. Equations represent statements of equality, where two expressions are equal, while inequalities represent statements of comparison, where one expression is greater or less than another.
To effectively solve problems involving these concepts, it’s important to understand the key differences and the steps required to work with them. Each type requires a specific approach to find the solution and interpret the results correctly.
Key Differences Between Equations and Inequalities
| Equations | Inequalities |
|---|---|
| Represent equality between two expressions | Represent a comparison between two expressions |
| Use an equal sign (=) | Use inequality symbols (>, |
| Solutions are specific values | Solutions are ranges of values |
| Can be solved by isolating the variable | Can be solved by finding the range of possible solutions |
Solving Equations and Inequalities
When solving equations, the goal is to find the value of the unknown variable that makes the equation true. This typically involves performing operations on both sides of the equation to isolate the variable.
In contrast, solving inequalities requires finding the range of values that satisfy the inequality. While the process is similar to solving equations, the main difference is that inequalities often result in a range of solutions, and the direction of the inequality symbol may change when multiplying or dividing by negative numbers.
Understanding these concepts and knowing when to apply each method is crucial for solving a variety of mathematical problems. With practice, you’ll gain the ability to interpret and solve both equations and inequalities accurately.
Solving Linear Equations
Linear equations are fundamental to understanding how to manipulate expressions involving variables. These types of equations have variables raised to the first power, and the goal is to find the value of the variable that makes the equation true. Solving them involves applying a series of logical steps to isolate the variable on one side of the equation.
When working with linear equations, the main objective is to balance both sides of the equation. This means whatever operation you perform on one side must be done on the other side to maintain equality.
Steps for Solving Linear Equations
- Start by simplifying both sides of the equation if necessary. Combine like terms and eliminate any parentheses.
- Move all the terms containing the variable to one side of the equation, and constant terms to the other side.
- Isolate the variable by performing operations (such as addition, subtraction, multiplication, or division) to both sides of the equation.
- Once the variable is isolated, simplify the equation to find the solution.
- Check your solution by substituting it back into the original equation to ensure it satisfies the equation.
Example of Solving a Linear Equation
Consider the equation: 2x + 5 = 13. To solve this, follow these steps:
- Start by isolating the term with the variable. Subtract 5 from both sides:
- 2x = 8
- x = 4
- 2(4) + 5 = 13, which simplifies to 13 = 13. The solution is correct.
By following these steps, you can solve linear equations efficiently and accurately. With practice, this process becomes quicker and more intuitive, laying a strong foundation for tackling more complex problems.
Importance of Checking Your Work
Verifying your calculations and solutions is a crucial step in ensuring accuracy. Mistakes, whether small or large, can occur during problem-solving, and they may not always be immediately obvious. By taking the time to review your work, you reduce the likelihood of errors and increase your confidence in the final results.
Checking your work allows you to catch common mistakes, such as miscalculations, sign errors, or incorrect steps. It also helps to ensure that you have followed the correct method and that the solution aligns with the question’s requirements.
Steps to Effectively Check Your Work
- Revisit the Original Problem: Ensure that you understand the question and what is being asked before reviewing your solution.
- Retrace Your Steps: Go through the solution process step-by-step to confirm that each operation was performed correctly.
- Use a Different Method: If possible, try solving the problem in a different way and compare the results.
- Double-Check Calculations: Carefully review all arithmetic and algebraic operations to identify any mistakes.
- Substitute the Solution: If appropriate, substitute your solution back into the original problem to check if it satisfies the equation or condition.
Why It Matters
Reviewing your work not only helps you spot mistakes but also solidifies your understanding of the concepts. It ensures that your solution is reliable and minimizes the risk of missing out on important details. Moreover, this habit fosters good problem-solving skills, which are valuable for future challenges.
By taking a moment to verify your answers, you can confidently submit your work knowing that you have done your best to ensure its accuracy.
Resources for Further Study
To continue improving your skills and deepen your understanding, there are numerous resources available that can guide you through more complex topics and provide extra practice. These materials range from online platforms to books and videos, all designed to enhance your learning experience.
By utilizing these resources, you can build a stronger foundation, reinforce concepts you may find challenging, and practice various problem-solving strategies at your own pace. Whether you prefer interactive exercises, video explanations, or textbooks, the following options will help you advance your knowledge and skills.
Online Learning Platforms
- Khan Academy: A comprehensive platform offering free lessons in various subjects, including a vast range of topics in mathematics, from basic to advanced concepts.
- Coursera: Provides access to courses from universities around the world, offering in-depth lessons and quizzes to reinforce learning.
- EdX: Similar to Coursera, EdX offers free courses with the option to pay for certificates. Many courses focus on mathematical reasoning and problem-solving techniques.
Books and Practice Workbooks
- “Mathematical Thinking” by John D. Cook: A book that challenges students to think critically about numbers, equations, and mathematical relationships.
- “The Art of Problem Solving” by Richard Rusczyk: A series that provides a deep dive into problem-solving strategies, ideal for students who want to hone their skills for more advanced challenges.
- Practice Workbooks: There are many workbooks available at bookstores or online retailers that provide exercises specifically designed to reinforce key concepts, such as solving equations, inequalities, and more.
Video Tutorials and Channels
- YouTube – MathHelp: This channel provides clear, step-by-step video lessons on a variety of topics, including solving equations and understanding mathematical principles.
- PatrickJMT: Known for concise explanations and visual aids, PatrickJMT offers tutorials that cover everything from basic arithmetic to more advanced topics.
By combining these resources, you can further enhance your knowledge and develop a stronger grasp of the topics you may encounter in future studies. Stay curious, practice consistently, and continue exploring new learning materials to build your mathematical foundation.
Next Steps After the Assessment
After completing a major assessment, it’s important to reflect on the experience and plan your next steps to continue progressing. Whether you performed well or faced challenges, there are always opportunities for growth. This is the perfect time to assess your understanding, identify areas for improvement, and determine how to build on the knowledge you’ve gained.
The following actions can help you stay on track and ensure you’re ready for the next stage in your learning journey:
- Review Your Performance: Take the time to thoroughly review your results. Understand what you did well and where mistakes were made. This reflection will help you identify patterns and areas that need more attention.
- Seek Additional Support: If you encountered difficulties during the assessment, consider reaching out to a teacher or tutor for further clarification. Engaging with others can provide different perspectives and strategies for understanding difficult concepts.
- Set New Learning Goals: Based on your performance and self-reflection, set specific goals for the next phase of your studies. These goals should focus on areas where you need improvement, as well as advancing topics you’re already comfortable with.
- Practice Regularly: Continued practice is key to mastering new skills. Make a habit of working on practice problems, reviewing challenging concepts, and testing yourself regularly to reinforce your learning.
- Explore Advanced Topics: If you feel confident in your current knowledge, start exploring more advanced topics to expand your skills. This will prepare you for future challenges and help you stay ahead.
Taking these steps will ensure that you maintain a strong learning trajectory, build confidence in your abilities, and prepare you for more complex subjects in the future.