
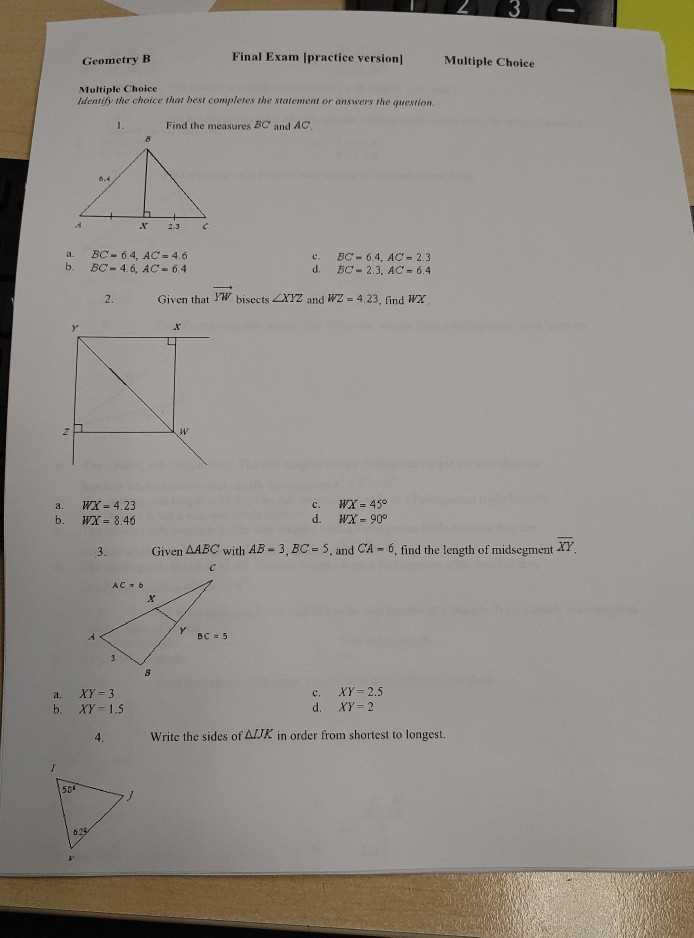
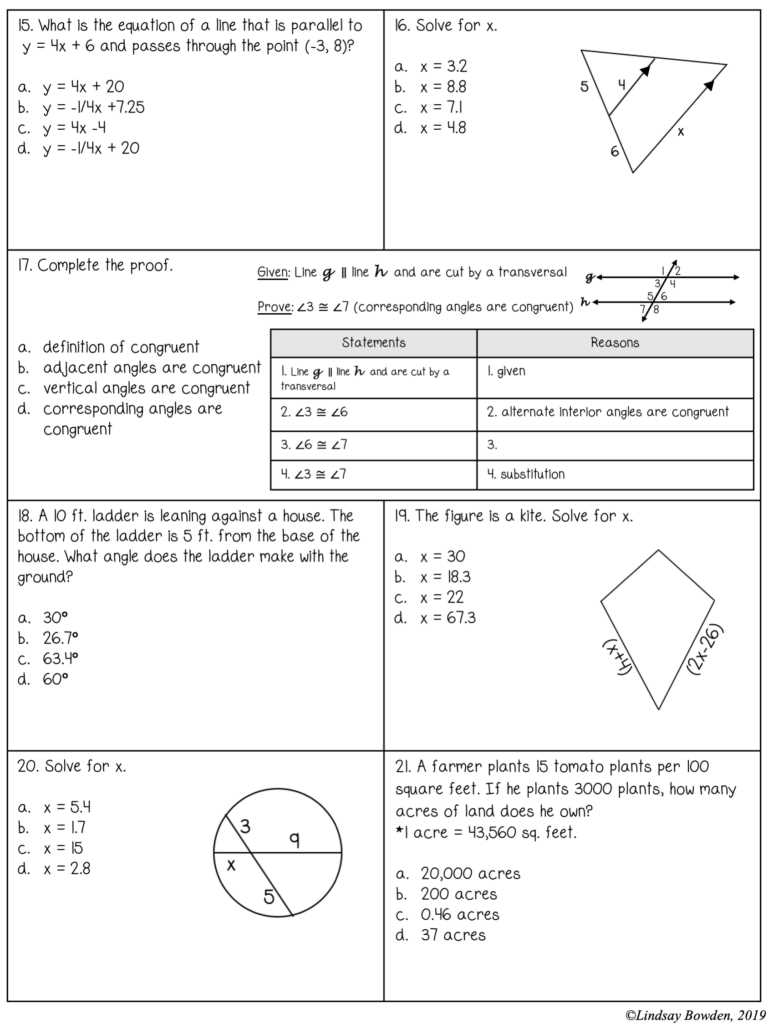
As students approach their mid-course evaluations, it’s essential to revisit key concepts and strengthen problem-solving skills. A well-rounded understanding of the material is crucial for success, especially when facing complex questions that test both knowledge and application. This section is designed to support your preparation, offering insights into common challenges and effective strategies for tackling them.
By focusing on foundational principles and carefully analyzing problem types, you can approach each section with confidence. Detailed explanations and step-by-step breakdowns are provided to clarify concepts, helping you better grasp essential techniques. Whether you are reviewing formulas, solving equations, or interpreting diagrams, mastering these core elements will empower you to perform at your best.
Comprehensive Solutions for Mid-Course Evaluation
This section provides detailed explanations and solutions to help you navigate through various questions typically found in assessments. Understanding how to approach each problem type is key to mastering the material and ensuring accuracy in your responses. With the right guidance, complex tasks become easier to solve, and common mistakes can be avoided.
Below, you will find step-by-step solutions that cover a range of topics. Each solution is broken down to highlight the reasoning behind each step, offering clarity and insight into how to correctly tackle similar questions in the future. The breakdown of formulas and techniques allows for a deeper understanding of their applications.
Key Topics Covered
- Shape Properties – Understanding different geometric forms and their key characteristics.
- Equation Manipulation – Steps for solving expressions and finding unknown variables.
- Angle Relationships – How angles interact and their significance in problem-solving.
- Trigonometric Applications – Using trigonometric ratios to solve real-world scenarios.
- Coordinate Analysis – How to interpret and manipulate coordinates in a plane.
By examining each part carefully, you will gain not only the correct results but also the reasoning behind them. This ensures that you can confidently apply similar techniques when faced with new challenges.
Step-by-Step Breakdown
- Start with Key Definitions: Make sure to clearly understand terms such as angle, vertex, and side length before diving into calculations.
- Use Visual Aids: Diagrams can provide insight into how elements are related. Label the figures and identify known values.
- Apply the Right Formula: Match the problem to the appropriate equation or theorem, ensuring all variables are correctly substituted.
- Double-Check Calculations: After obtaining results, recheck each step for accuracy to avoid simple mistakes.
With this approach, solving problems becomes a systematic process, allowing you to develop a strong foundation for any future assessments.
Understanding the Assessment Format
Familiarity with the structure and layout of an evaluation is crucial for effective preparation. Knowing the types of questions and the time allotted helps reduce anxiety and enables more efficient study sessions. By understanding the format, students can anticipate what to expect and approach each section with a clear strategy.
Typically, these evaluations consist of a variety of question types, each assessing different skills. Some sections focus on theoretical knowledge, requiring you to recall definitions, theorems, and principles, while others test your ability to apply concepts to solve problems. It’s important to recognize the format in order to allocate time appropriately for each type of task.
Question Types
- Multiple Choice: Questions that offer several answer choices, where only one is correct. These test your understanding of key concepts.
- Short Answer: Aimed at assessing your ability to quickly recall facts and provide concise responses.
- Problem Solving: These problems require step-by-step calculations, often involving formulas or geometric reasoning.
- Diagram Interpretation: Questions that involve reading and analyzing diagrams, requiring you to identify relationships between different parts.
Time Management and Strategy
Time management is essential for successfully completing each section. Prioritize questions based on difficulty and value, and ensure you have enough time to review your answers. If you encounter challenging problems, it’s often best to move on and come back to them later, preventing you from getting stuck.
Key Concepts for Assessment Preparation
To achieve success in any evaluation, it is essential to understand the foundational principles that will be tested. Mastery of key concepts not only helps you recall important information but also equips you with the skills necessary to apply that knowledge in problem-solving scenarios. By focusing on these core topics, you can streamline your study process and improve your performance.
Familiarity with important terms, theorems, and formulas is crucial. In addition, practicing with different types of problems will reinforce your understanding and develop your ability to think critically and efficiently. Below are some of the most important concepts to focus on when preparing for the assessment.
Essential Principles to Master
- Properties of Shapes: Understand the key characteristics of various geometric forms, such as angles, sides, and symmetry. This knowledge is the foundation for solving problems related to area, perimeter, and volume.
- Theorems and Postulates: Familiarize yourself with essential theorems like the Pythagorean Theorem, as well as postulates that govern relationships between lines, angles, and shapes.
- Formulas and Equations: Memorize the most frequently used formulas for calculating areas, volumes, surface areas, and other important geometric properties.
- Transformations and Symmetry: Understand how figures can be translated, rotated, reflected, and scaled, and how these transformations affect their properties.
Problem-Solving Techniques
- Step-by-Step Solutions: Break down complex problems into smaller, manageable parts. Apply the relevant formulas and rules systematically to avoid confusion.
- Visualizing the Problem: Drawing diagrams or visual aids can often clarify relationships and make abstract concepts more concrete.
- Practice with Varied Problems: The more problems you solve, the more familiar you will become with different scenarios. Practice with both theoretical and applied questions.
By mastering these core topics and practicing regularly, you will be well-prepared to tackle a wide range of questions with confidence and precision.
Reviewing Essential Geometry Terms
A strong understanding of basic terminology is vital for solving problems effectively and accurately. The language of this field is built on key terms that define relationships, properties, and characteristics of different figures and concepts. Familiarity with these terms allows you to quickly grasp the nature of a problem and select the appropriate methods for finding solutions.
Below are some of the fundamental terms that are commonly encountered. Mastering these will not only help you understand the material better but also enable you to approach each problem with confidence and clarity.
- Angle: The space between two intersecting lines measured in degrees. Common types include acute, obtuse, and right angles.
- Vertex: The point where two lines or segments meet to form an angle or corner.
- Edge: A line segment where two faces of a polyhedron meet.
- Perpendicular: When two lines intersect at a right angle (90 degrees).
- Parallel: Lines that never intersect and remain equidistant from each other.
- Radius: The distance from the center of a circle to any point on its circumference.
- Diameter: A straight line passing through the center of a circle, connecting two points on its edge.
- Congruent: Figures or objects that have the same size and shape, often used to compare angles or sides of polygons.
- Symmetry: A property where one half of a figure is a mirror image of the other half.
By reinforcing these definitions and understanding how they apply to different shapes and problems, you can greatly enhance your problem-solving abilities and accuracy in assessments.
Strategies for Solving Geometry Problems
Approaching complex problems requires a structured and methodical strategy. Whether working with shapes, angles, or measurements, having a clear plan can greatly improve your accuracy and efficiency. A systematic approach not only ensures you don’t overlook important details but also helps you stay organized and focused throughout the problem-solving process.
The following strategies will guide you through different types of challenges, making it easier to identify key relationships, apply the correct methods, and arrive at the correct solution.
Step-by-Step Problem Solving
- Read Carefully: Always start by carefully reading the problem statement to ensure you understand what is being asked. Pay attention to any given information and identify what needs to be determined.
- Draw a Diagram: Visualizing the problem by sketching a diagram can help clarify the relationships between different elements. Labeling all known values and unknowns is key to keeping track of important information.
- List Known and Unknown Values: Organize the information by separating what is given and what you need to find. This can often help you identify the most appropriate formula or theorem to use.
- Break Down the Problem: Complex questions may involve multiple steps. Break the problem into smaller, manageable parts, solving one step at a time.
Applying Theorems and Formulas

- Use Relevant Theorems: Identify which theorems or principles apply to the problem, such as the Pythagorean Theorem, angle sum property, or area formulas.
- Substitute Correctly: When applying formulas, ensure that all variables are correctly substituted with their corresponding values, taking care with units of measurement.
- Check for Special Properties: Recognize special properties, like parallel lines or congruent angles, that can simplify calculations or lead to shortcuts in solving the problem.
By following these strategies, you’ll develop a clearer understanding of each problem and enhance your ability to find solutions efficiently and accurately.
Common Mistakes to Avoid on the Assessment
While preparing for any type of evaluation, it’s just as important to recognize potential pitfalls as it is to master the material. Even well-prepared students can fall into common traps that lead to mistakes. Being aware of these errors ahead of time can help you avoid them and improve your overall performance.
In the following section, we will discuss some of the most frequent mistakes made during assessments and offer advice on how to steer clear of them. By understanding these missteps, you’ll be better equipped to approach each question with confidence and accuracy.
- Misreading the Question: One of the most common mistakes is failing to fully understand what the question is asking. Take time to carefully read each problem, making sure you know exactly what is being requested.
- Skipping Over Details: Often, small details are key to solving a problem. Pay attention to every piece of information provided in the problem statement, as missing even one piece can lead to incorrect solutions.
- Forgetting to Label Diagrams: Failing to label important values or parts of a diagram can cause confusion later in the process. Always ensure that all known values are clearly marked, and leave space for unknowns that you’ll calculate.
- Incorrect Use of Formulas: Misapplying formulas or using the wrong one for the situation is a frequent mistake. Be sure to double-check that the formula matches the type of problem you’re solving.
- Rushing Through Calculations: Speed is important, but rushing through calculations often leads to careless errors. Take your time with each step and double-check your work to ensure accuracy.
- Not Reviewing Your Work: Failing to review your answers before submitting can result in missing small mistakes or oversights. If time allows, always take a moment to review your work and ensure all steps are clearly shown and correctly calculated.
By being mindful of these common mistakes and applying a careful, methodical approach, you can minimize errors and maximize your performance on the assessment.
How to Use the Answer Key Effectively
An answer key can be a valuable tool in the learning process when used correctly. It serves not only as a source of correct solutions but also as a guide for understanding the methods and reasoning behind each answer. By analyzing the provided solutions, you can deepen your understanding of the material and identify areas for improvement.
To make the most of an answer key, it’s essential to go beyond simply checking your final answer. Here are some strategies for using this resource to enhance your learning and performance.
- Understand the Steps: Instead of just looking at the final solution, take time to review each step of the solution process. Understand the logic and methods used, and see how each step connects to the next.
- Identify Mistakes: If your solution differs from the answer key, compare your process to identify where you went wrong. This can help you pinpoint misunderstandings or gaps in your approach.
- Learn the Method: Even if you get a correct result, review the methods used in the solution to ensure you are applying the correct strategies. This will reinforce your problem-solving skills for future challenges.
- Practice Independently: After reviewing the solution, try solving a similar problem on your own without referring to the answer key. This will help solidify your understanding and improve your confidence.
- Focus on Weak Areas: Use the answer key to identify concepts or steps you struggled with. Spend extra time practicing those areas to ensure you can apply the methods correctly in future problems.
By using the answer key in this thoughtful and proactive manner, you can improve both your understanding of the material and your overall problem-solving abilities.
Geometry Proofs and Logical Reasoning
Logical reasoning plays a crucial role in the study of shapes and figures, particularly when it comes to proving relationships and properties. To demonstrate the truth of a statement, one must follow a clear and methodical sequence of steps, using established principles and logical deductions. Understanding how to construct valid arguments is essential for successfully solving problems and showing how one piece of knowledge leads to another.
In this section, we will explore how to approach proofs and use reasoning effectively to establish the validity of geometric propositions. Below is a table that outlines key logical steps often used in constructing proofs, along with their meanings.
| Step | Description |
|---|---|
| Given | The information provided in the problem or the assumptions you can use as starting points. |
| To Prove | The statement or relationship that you need to demonstrate is true based on the given information. |
| Reasoning | The logical steps that connect your given information to the statement you are trying to prove. This can include applying definitions, postulates, or previously proven theorems. |
| Conclusion | The final result of your proof, where you’ve shown the truth of the statement based on your reasoning. |
By following these steps and ensuring each part of the argument is logically sound, you can effectively prove geometric relationships and solve related problems. Remember, each proof should flow naturally from one statement to the next, with no gaps in the reasoning. Logical consistency is key to mastering this skill.
Exploring Geometric Shapes and Properties
Understanding the characteristics and features of different shapes is fundamental to solving many types of problems. Each shape has its own set of properties, such as angles, sides, and symmetries, which can be used to solve puzzles, prove theorems, and find unknown values. By studying these properties, we can gain insights into how shapes relate to one another and how their characteristics can be applied in various contexts.
In this section, we will explore some of the most common shapes and their key properties. Knowing these properties will help you recognize patterns and relationships, making it easier to approach problems involving these figures.
- Triangles: Triangles are defined by three sides and three angles. Their properties vary depending on the type of triangle, such as equilateral, isosceles, or scalene. The sum of the interior angles of any triangle is always 180°.
- Quadrilaterals: These four-sided shapes come in various forms, including squares, rectangles, parallelograms, and trapezoids. Each type has unique properties, such as parallel sides or right angles, which influence how we calculate area and perimeter.
- Circles: Circles are defined by their center and radius. They have several important properties, such as the constant ratio of the circumference to the diameter (π), and formulas for calculating area and circumference.
- Polygons: Polygons are closed figures with straight sides. The number of sides determines the type of polygon, such as pentagons (5 sides), hexagons (6 sides), and so on. The sum of the interior angles of a polygon can be calculated using the formula (n – 2) × 180°, where n is the number of sides.
By familiarizing yourself with these shapes and their properties, you will be better equipped to solve problems that require you to apply geometric principles, whether in practical or theoretical contexts.
Coordinate Geometry Review Tips
Understanding the relationships between points, lines, and shapes in a coordinate plane is essential for solving many problems in mathematics. In this section, we will discuss practical strategies for reviewing the concepts and techniques used in this area. These tips will help you strengthen your skills and gain confidence in applying coordinate principles to different types of problems.
Mastering Key Formulas
One of the most important aspects of working with coordinates is knowing the essential formulas. Here are a few key formulas to remember:
- Distance Formula: Used to calculate the distance between two points, d = √((x2 – x1)² + (y2 – y1)²).
- Midpoint Formula: Used to find the midpoint of a line segment, M = ((x1 + x2)/2, (y1 + y2)/2).
- Slope Formula: Used to find the slope of a line, m = (y2 – y1) / (x2 – x1).
Visualizing the Problem
Another helpful approach is to visualize the given points and lines on a coordinate plane. This will give you a better sense of the relationships between them and help you solve the problem more easily. Plotting points on graph paper or using graphing software can help you see the connections clearly and reduce the chances of making calculation errors.
By focusing on these strategies, you can improve your ability to solve problems involving coordinates and relationships between geometric objects on a plane. Consistent practice will ensure that you can apply these techniques with accuracy and speed when needed.
Trigonometry Basics for Geometry Exams
Trigonometry plays a crucial role in solving various problems involving angles and lengths in triangles. Understanding the basic concepts and functions of trigonometry is essential for tackling these types of questions effectively. This section will introduce the fundamental principles of trigonometry and provide useful tips for applying them in geometric contexts.
Key Trigonometric Ratios
There are three primary trigonometric ratios used to relate the angles of a right triangle to the lengths of its sides. Memorizing these ratios will help you quickly solve for unknown values:
- Sine (sin): The ratio of the length of the opposite side to the hypotenuse. sin(θ) = opposite / hypotenuse.
- Cosine (cos): The ratio of the length of the adjacent side to the hypotenuse. cos(θ) = adjacent / hypotenuse.
- Tangent (tan): The ratio of the length of the opposite side to the adjacent side. tan(θ) = opposite / adjacent.
Using the Pythagorean Theorem
In addition to the trigonometric ratios, the Pythagorean Theorem is another essential tool for solving problems involving right triangles. This theorem relates the lengths of the sides of a right triangle:
- Theorem: a² + b² = c², where a and b are the lengths of the two legs and c is the length of the hypotenuse.
By applying these basic principles, you can confidently approach problems involving angles and side lengths in right triangles. Consistent practice with these concepts will improve your ability to solve trigonometric problems quickly and accurately.
Understanding Geometry Word Problems
Word problems often present challenges as they require translating a real-world situation into mathematical expressions and formulas. The key to solving these problems is understanding the underlying relationships between the given information and how to apply relevant concepts. This section will provide strategies for approaching and solving word problems effectively.
Breaking Down the Problem
The first step in solving any word problem is carefully reading the question and identifying the given data. Breaking the problem down into smaller, manageable parts will make it easier to understand and solve. Here are some tips to consider:
- Identify key information: Highlight or underline important numbers, shapes, and relationships mentioned in the problem.
- Draw a diagram: Sketching a visual representation of the situation can help you better understand the problem and how the pieces fit together.
- Define variables: Assign variables to unknown values and note the relationships between them.
Choosing the Right Formula
Once the problem is broken down, it’s important to select the appropriate formula or strategy for solving it. The choice of method depends on the type of information given and what you need to find. For example:
- For area or perimeter: Use the respective formulas for shapes like rectangles, circles, or triangles.
- For angles and relationships: Use trigonometric functions or angle sum properties for polygons and circles.
- For volume or surface area: Use the formulas for three-dimensional shapes like cubes, spheres, or cones.
By breaking the problem down into parts, choosing the right strategy, and applying the relevant formulas, you can efficiently solve word problems and gain a better understanding of the concepts at play.
Reviewing the Pythagorean Theorem
The Pythagorean Theorem is one of the most fundamental principles in mathematics, particularly when dealing with right-angled triangles. It establishes a relationship between the lengths of the sides of a right triangle, providing a method for calculating the length of one side when the other two are known. Understanding this theorem is essential for solving a wide range of problems involving triangles and distances.
The theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This can be expressed algebraically as:
| a² + b² = c² |
| a and b are the lengths of the two legs, and c is the length of the hypotenuse. |
This relationship allows you to solve for any missing side when the other two sides are known, making it a powerful tool for both theoretical and practical applications, such as navigation, construction, and physics. Below are some steps to follow when applying the Pythagorean Theorem:
- Step 1: Identify the sides of the triangle. The hypotenuse is always opposite the right angle.
- Step 2: Apply the formula a² + b² = c² using the known side lengths.
- Step 3: Solve for the unknown side by rearranging the formula.
By mastering this theorem, you can approach many problems involving right-angled triangles with confidence, whether they relate to distance calculations, area determinations, or other real-world applications.
Angles and Their Relationships in Geometry
Understanding the different types of angles and their relationships is crucial in solving various mathematical problems. Angles play a key role in understanding shapes, lines, and their interactions. They are fundamental to a wide range of geometric concepts, from basic constructions to complex proofs.
Angles can be classified into various types based on their measure. These classifications allow us to establish relationships between angles, such as complementary, supplementary, and vertical angles. Recognizing these relationships helps in simplifying problems and finding unknown values.
| Type of Angle | Definition | Example |
|---|---|---|
| Acute Angle | Any angle less than 90° | 45° |
| Right Angle | An angle exactly equal to 90° | 90° |
| Obtuse Angle | An angle greater than 90° but less than 180° | 120° |
| Complementary Angles | Two angles that add up to 90° | 40° and 50° |
| Supplementary Angles | Two angles that add up to 180° | 110° and 70° |
| Vertical Angles | Angles that are opposite each other when two lines intersect | 120° and 120° |
By mastering the properties and relationships between angles, you can solve a variety of problems more effectively. Whether dealing with parallel lines, polygons, or circles, an understanding of these angle types will help clarify the relationships within different geometric structures.
Understanding Circle Theorems and Properties

Circles are fundamental shapes in mathematics, and understanding their theorems and properties is essential for solving many geometric problems. These properties govern the relationships between various elements of a circle, including its radius, diameter, and the angles formed by intersecting lines or chords. By mastering these concepts, you can tackle a wide range of problems involving circular structures and their various attributes.
Key theorems related to circles help explain how different parts of the circle interact. For example, the relationship between the radius and the tangent line, or the behavior of angles subtended by chords, are just a few of the key ideas that arise in circle theorems. Understanding these relationships can make solving complex problems more intuitive and manageable.
Common Circle Theorems
- Angle at the Center Theorem: The angle at the center of the circle is twice any angle at the circumference subtended by the same arc.
- Tangent-Secant Theorem: A tangent to a circle is perpendicular to the radius at the point of contact.
- Chord Theorem: If two chords in a circle intersect, the products of the lengths of the segments of one chord equal the product of the lengths of the segments of the other chord.
Key Properties of Circles
- Radius: The distance from the center to any point on the circle is constant.
- Diameter: The longest possible chord of the circle, passing through the center, is twice the length of the radius.
- Circumference: The perimeter of a circle, calculated as 2πr, where r is the radius.
By understanding these core properties and theorems, solving problems involving circles becomes much more straightforward. With practice, recognizing when and how to apply these concepts will lead to quicker and more accurate solutions in various geometric contexts.
Working with Geometric Constructions
Constructing geometric shapes and figures using precise tools is a key skill in solving various mathematical problems. These constructions help visualize and understand the relationships between different elements in a figure, whether they are angles, lines, or shapes. By mastering these techniques, you can create accurate diagrams and improve your problem-solving abilities. Constructions also serve as the foundation for proving various geometric properties and theorems.
Geometric constructions are often performed with basic tools such as a compass, straightedge, and protractor. These tools allow for the creation of points, lines, circles, and other shapes with specific properties. Some common constructions include bisecting angles, drawing perpendicular lines, and creating parallel lines. Through these techniques, you can establish the foundation for more complex geometric problems.
Basic Construction Techniques
- Bisecting an Angle: Using a compass and straightedge, divide an angle into two equal parts by constructing an arc that intersects both rays of the angle, followed by drawing a line from the vertex through the intersection point.
- Constructing Perpendicular Lines: To construct a perpendicular line to a given line from a point not on the line, create a pair of arcs from the point, and draw a line through their intersection to form the right angle.
- Constructing Parallel Lines: Draw a line through a point parallel to an existing line by creating two equal angles with the given line and using the angle bisector.
Using Constructions for Proofs
Geometric constructions also play a critical role in proofs, where accuracy and precision are essential. By constructing specific shapes or elements, you can demonstrate relationships and prove various properties. For instance, constructing the perpendicular bisector of a line segment can help prove congruence of triangles. Similarly, the construction of an angle bisector is often used to demonstrate the equality of angles in certain geometric figures.
By consistently practicing geometric constructions, you can improve both your understanding and your ability to solve geometric problems effectively. Each construction technique serves as a building block for understanding more advanced geometric concepts.
Preparing for Test Time Management
Effectively managing your time while preparing for a mathematical assessment is crucial for success. Balancing study sessions, practice problems, and review materials can be challenging, but with the right strategy, you can maximize your efficiency and ensure that you’re fully prepared. Time management not only helps you cover all necessary topics but also reduces stress, giving you a sense of control over your preparation process.
To start, it’s important to set clear goals for each study session. Determine which topics or concepts need the most attention and allocate more time to those areas. Break your study time into manageable blocks, ensuring regular breaks to maintain focus and avoid burnout. Prioritize areas where you feel least confident and gradually work through the material to build your understanding. Consistent, focused study sessions are far more effective than cramming at the last minute.
Here are some practical tips for managing your study time:
- Create a Study Schedule: Develop a daily or weekly study plan that breaks down the material you need to cover. Make sure to balance difficult topics with easier ones to avoid feeling overwhelmed.
- Use Practice Problems Wisely: Practice problems are one of the best ways to prepare, but make sure to pace yourself. Start with easier questions and gradually increase the difficulty as you feel more confident.
- Simulate Test Conditions: Try practicing under timed conditions to simulate the test environment. This helps you get comfortable with time constraints and improve your speed in solving problems.
- Review Mistakes: After completing practice problems, spend time reviewing your mistakes. Understanding where you went wrong and correcting those errors can lead to better performance on the actual test.
- Stay Consistent: Consistency is key. Studying for a short amount of time every day is far more effective than trying to study everything in one long session.
By managing your time wisely, you’ll ensure that you approach the assessment with confidence and a clear understanding of the material. Proper planning allows you to cover all topics, stay focused, and give yourself the best chance to perform at your highest level.
Final Review and Last-Minute Tips
As the day of the assessment approaches, it’s essential to focus on reinforcing key concepts and reviewing any challenging material. The final review should consolidate what you’ve already learned and ensure that you’re prepared to tackle any problem that might come your way. This period is all about optimizing your study efforts and sharpening your skills, so you can perform confidently under pressure.
In the final hours leading up to the test, there are a few strategies to help maximize your preparation and avoid feeling overwhelmed:
1. Focus on Key Areas
Identify the topics that carry the most weight or that you’ve struggled with the most. Spend your remaining time revisiting these areas to solidify your understanding. Prioritize practice problems that test your knowledge in these key areas, as they are likely to appear more frequently in the assessment.
2. Avoid Cramming
Avoid trying to learn new material at the last minute. At this stage, it’s better to reinforce what you already know. Cramming can increase stress and lead to confusion, especially when it comes to complex concepts. Instead, use your time for reviewing notes and practicing problems that are representative of the test content.
3. Quick Refresh on Formulas and Theorems
Take a few minutes to quickly go over important formulas and theorems. Make sure you know where to apply each one and have them fresh in your mind. This quick recap can help reduce the chances of forgetting key concepts during the test.
4. Stay Calm and Confident
Stress can impair your ability to think clearly, so take time to relax. Deep breaths and positive self-talk can help you maintain composure. Remember, you’ve put in the work, and now it’s about executing what you know with confidence.
By focusing on these strategies and maintaining a calm mindset, you’ll maximize your effectiveness during your final preparation. These last-minute tips will help you approach the test with clarity and ensure you’re ready to perform your best.