Mathematics can often seem daunting, especially when faced with complex problems that require a deeper understanding of concepts. Whether you’re working through challenging assignments or revisiting topics for review, having the right support can make all the difference in your success. This section focuses on offering essential tools and methods to navigate through intricate exercises effectively, providing clarity on how to approach and solve various mathematical challenges.
By breaking down each problem and offering insightful explanations, students can gain confidence in their ability to tackle even the most difficult tasks. The guidance offered here emphasizes not only finding the correct solutions but also understanding the reasoning behind each step. With consistent practice and the right approach, mathematical concepts can become clearer and more manageable, paving the way for academic growth and achievement.
Understanding Cpm Core Connections Course 3
The third level of this mathematical program is designed to guide students through advanced concepts, promoting a deep understanding of fundamental principles. It emphasizes critical thinking and problem-solving skills, encouraging learners to not just find answers but to grasp the underlying reasoning behind each solution. The focus is on developing a strong foundation that will support future learning in higher-level mathematics.
Key Features of the Program

This level covers a broad range of topics, with a particular focus on algebraic reasoning, geometric relationships, and data analysis. The lessons are structured to build progressively, ensuring that each concept is thoroughly explored before moving on to more complex material. Students are challenged to apply their knowledge in practical scenarios, reinforcing their learning through exercises and problem sets.
Why It Matters
By mastering the material at this stage, learners can gain a strong mathematical toolkit that will serve them well in both academic and real-world settings. The ability to analyze and solve problems systematically is an invaluable skill that extends beyond the classroom. This approach not only prepares students for future academic success but also helps them develop a logical and structured way of thinking that can be applied to a variety of disciplines.
Overview of the Cpm Core Connections Curriculum
This educational program is structured to help students develop a strong understanding of mathematical concepts through progressive learning. The curriculum is designed to ensure that each topic is covered comprehensively, building from foundational principles to more complex theories. The goal is to cultivate critical thinking and problem-solving abilities that students can apply in a variety of academic and real-world scenarios.
The material is organized in a way that encourages active learning and engagement. Lessons focus on real-life applications, helping students see the practical value of what they are studying. Each unit introduces new challenges that build on previously learned concepts, fostering continuous growth and mastery of the subject matter. By the end of the program, students will have gained both the skills and confidence necessary to tackle advanced mathematical problems.
Key Concepts in Course 3 Mathematics
This level of mathematics introduces a variety of essential ideas that are fundamental to developing a deeper understanding of the subject. Students will explore a range of topics that reinforce their problem-solving abilities and encourage logical thinking. The concepts presented in this stage provide a solid foundation for more advanced mathematical study.
Core Areas of Focus
The following areas are key components of the curriculum:
- Algebraic Expressions: Students learn how to manipulate variables, solve equations, and understand functions.
- Geometry: The focus is on shapes, angles, and geometric properties, as well as understanding their real-world applications.
- Statistics and Probability: Emphasis on data analysis, probability calculations, and interpreting results.
- Ratios and Proportions: This concept helps students understand relationships between quantities and apply them to real-life situations.
- Linear Relationships: The study of graphs and equations that represent relationships between variables.
How These Concepts Build Skills
Each topic is interconnected and designed to build on previous lessons. Students will develop strong analytical skills and the ability to approach complex problems systematically. These concepts not only enhance mathematical reasoning but also equip students with valuable skills applicable in other subjects and real-world situations.
How the Answer Key Supports Learning
Providing students with solutions helps to reinforce their understanding of mathematical concepts and ensures that they can apply the techniques they’ve learned effectively. By reviewing the correct steps and results, learners can identify where they may have gone wrong and correct any misunderstandings. This resource serves not only as a way to verify answers but also as a tool to deepen comprehension and promote critical thinking.
With clear explanations and step-by-step breakdowns, learners can gain insights into the problem-solving process. This approach encourages them to think critically about each step, fostering a better grasp of the underlying principles. Moreover, it enables students to track their progress, pinpoint areas for improvement, and develop the skills needed to solve more complex problems independently.
Breaking Down the Course 3 Problems
To truly master the material, it’s essential to break down each problem into manageable parts. This approach allows students to understand the structure of the question and apply the correct methods to find the solution. Rather than being overwhelmed by the complexity, learners can tackle the problem step by step, gaining confidence along the way.
Steps for Analyzing Problems
- Read Carefully: Understand t
Using the Answer Key Effectively
To make the most of a solution guide, it’s important to approach it not just as a tool for verification, but as a learning resource. The true value lies in understanding the process behind each solution, not just confirming the result. By closely examining the steps and reasoning provided, students can gain a deeper insight into the problem-solving methods and apply them to similar challenges in the future.
Here’s a method for using a solution guide in a way that enhances learning:
Step How to Use the Solution 1. Review the Answer Check your result against the provided solution to ensure you’re on the right track. 2. Analyze the Steps Carefully follow each step of the solution, noting how each part of the problem is addressed. 3. Identify Mistakes If your answer differs, identify where your approach went wrong and compare it to the correct process. 4. Practice Independently After understanding the solution, try similar problems without looking at the guide to reinforce your skills. Using a solution guide in this structured way not only helps to correct errors but also deepens your understanding and strengthens your mathematical abilities for the future.
Common Challenges in Course 3
As students advance in their studies, they often encounter more complex concepts and problems that require a deeper level of understanding and application. In this level, learners may face several common challenges that can make the material seem overwhelming at first. However, recognizing these challenges and addressing them strategically can help students navigate through the difficulties and achieve mastery.
One frequent obstacle is the difficulty in applying abstract concepts to real-world problems. Many students struggle to see the practical relevance of certain mathematical principles, which can lead to confusion and frustration. Another challenge arises when multiple steps are required to solve a problem, often leaving students unsure of where they went wrong when an answer doesn’t match. These hurdles can hinder progress if not addressed with proper strategies.
Key Challenges Include:
- Understanding Complex Word Problems: Interpreting and translating real-world situations into mathematical equations can be difficult for many learners.
- Solving Multi-Step Problems: As questions require more steps, it becomes easy to lose track of the process or make simple mistakes.
- Mastering New Theories: New mathematical concepts, such as algebraic expressions or advanced geometry, can be overwhelming when first introduced.
- Time Management: Students may feel rushed to complete assignments, which can affect their ability to thoroughly understand each problem.
By recognizing these common issues early on, students can develop the necessary skills and strategies to overcome them and continue progressing confidently through the material.
Strategies for Solving Complex Problems
When faced with challenging problems, it’s essential to approach them with a clear strategy that breaks down the task into manageable steps. Tackling complex questions requires more than just applying formulas; it demands a methodical approach to ensure each part of the problem is understood and addressed. By using proven strategies, students can improve their problem-solving skills and gain confidence in their ability to tackle even the most difficult tasks.
Step-by-Step Breakdown
One effective strategy is to break the problem into smaller, simpler parts. This method allows you to focus on one component at a time, preventing feelings of overwhelm. By addressing each part systematically, you can ensure that all aspects of the problem are covered without missing crucial steps. For example, in algebraic problems, start by isolating variables, and in geometry, focus on identifying key shapes and angles first.
Look for Patterns and Connections
Another powerful approach is to search for patterns and relationships within the problem. Recognizing similarities to previously solved problems can help you apply familiar techniques in new contexts. Identifying repeating patterns often leads to shortcuts or simpler methods for finding solutions, especially in algebra and probability. Developing this ability to spot connections can make solving complex problems faster and more intuitive.
By combining these strategies–breaking down problems and identifying patterns–students can develop a more structured and efficient approach to problem-solving, allowing them to tackle even the most complex questions with greater ease.
What to Expect in Course 3 Assignments
As you progress through your studies, the assignments will become more challenging, requiring you to apply a deeper understanding of the material. These tasks are designed to test your ability to solve complex problems, think critically, and use multiple steps to reach a solution. Rather than just focusing on memorization, these assignments aim to enhance your problem-solving skills and conceptual knowledge, preparing you for more advanced topics.
Types of Problems
The assignments in this level will include a variety of problem types, each designed to test specific skills. You can expect to encounter:
Problem Type Description Word Problems These problems require translating real-world scenarios into mathematical equations and solving them step by step. Multi-Step Calculations Questions that involve solving several parts of a problem in a sequence, requiring critical thinking at each stage. Conceptual Questions These assess your understanding of underlying principles, rather than just applying formulas or methods. Approach to Completing Assignments
To succeed in these assignments, it is important to follow a systematic approach. Start by carefully reading through each problem, making sure you understand the question before proceeding. Break down each task into smaller steps and solve them individually. Don’t rush; take the time to review your work and make sure every part is correct. Practice regularly and seek help when needed, as consistency and persistence are key to mastering the material.
How Course 3 Builds on Previous Knowledge
As students progress in their studies, each level is designed to deepen and expand upon the foundational concepts learned in earlier stages. In this level, the material introduces more complex ideas that rely heavily on the skills and knowledge acquired previously. By building on earlier concepts, learners are able to tackle more challenging problems with a stronger understanding and a more refined approach.
The new material isn’t introduced in isolation; it often connects directly to previously learned concepts, encouraging students to see how different areas of study overlap and complement one another. For example, algebraic skills developed in earlier levels become crucial for solving problems involving geometry or advanced functions. This continuity helps reinforce learning and allows students to apply their growing understanding to a wider variety of problems.
By reviewing and revisiting key concepts while adding new layers of complexity, this level ensures that students can apply both old and new knowledge seamlessly, leading to a more cohesive learning experience. This approach not only improves retention but also prepares students for future topics that will build even further on the skills they are currently mastering.
Important Mathematical Tools in Course 3
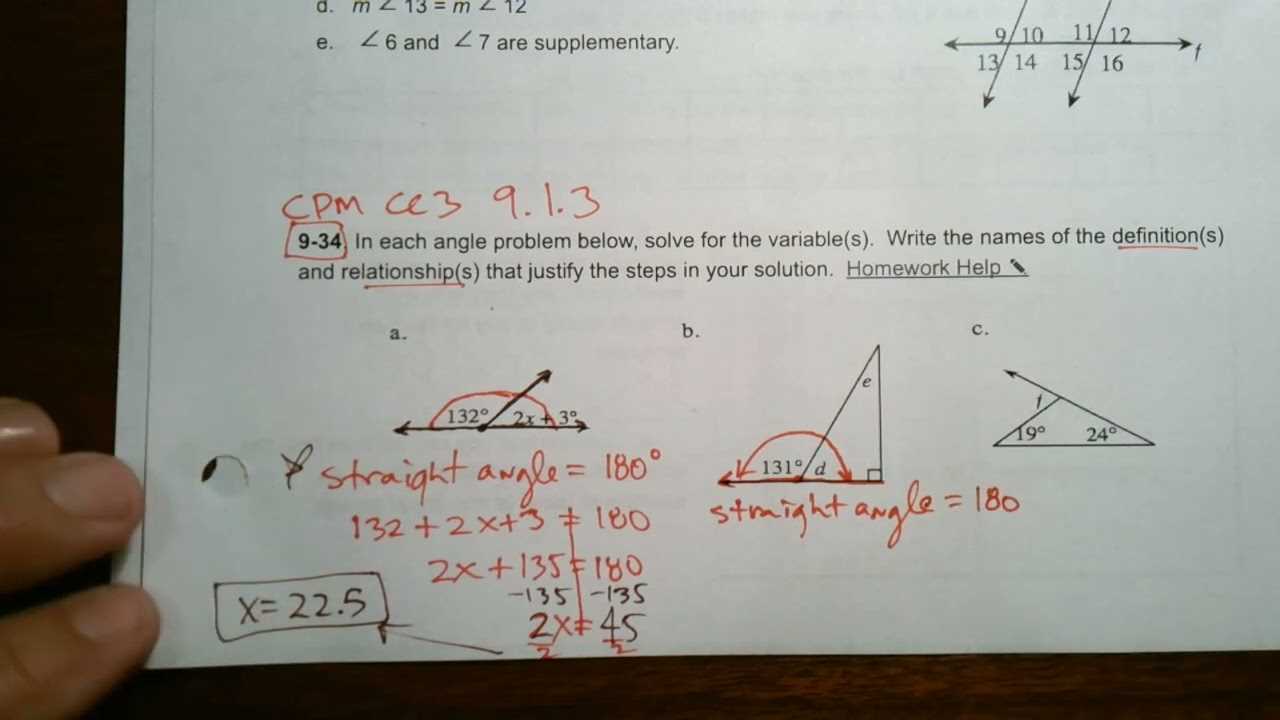
As students move forward in their studies, certain mathematical tools become essential for solving complex problems. These tools not only help to streamline the process of finding solutions but also enable a deeper understanding of the concepts being taught. Mastery of these tools allows students to tackle a wide range of topics with greater ease and efficiency. In this level, several key methods and strategies are introduced that will serve as building blocks for future learning.
Among the tools covered, some are fundamental for algebraic manipulations, while others are designed to aid in visualizing geometric concepts or analyzing data. By understanding when and how to use each tool, students can enhance their problem-solving abilities and strengthen their overall mathematical proficiency.
Tool Use Graphing Calculators Used to plot equations and visualize functions, helping to understand relationships between variables. Algebraic Manipulation Techniques like factoring and simplifying expressions are used to solve equations and inequalities. Geometric Constructions Tools such as compasses and rulers are used to draw precise shapes and measure angles in geometry problems. Systems of Equations Used to solve problems that involve multiple unknowns, such as in linear algebra or optimization. Probability Tools Techniques for analyzing chance events, including counting methods and basic probability formulas. Each of these tools is introduced gradually, with an emphasis on practical application. As students become comfortable with them, they will be able to apply these methods across a variety of mathematical challenges, ultimately improving their problem-solving skills and enhancing their overall understanding of the subject matter.
How the Answer Key Enhances Understanding
Providing solutions to problems is just one part of the learning process. What truly enhances comprehension is understanding the reasoning behind each step. Access to correct solutions, accompanied by detailed explanations, allows students to see the thought process involved, helping them identify any gaps in their own approach. This guidance fosters a deeper understanding of the material, offering valuable insights into both the methods used and the logic applied to reach a conclusion.
Key Benefits of Solutions for Learning

- Clarification of Concepts: When students review correct answers alongside explanations, they can better grasp difficult concepts that might have been unclear during initial learning.
- Step-by-Step Guidance: Solutions provide a clear breakdown of the problem-solving process, allowing students to follow along and understand the reasoning behind each decision.
- Identifying Mistakes: By comparing their work to the provided solutions, students can identify where they may have gone wrong and correct their mistakes.
- Building Confidence: Seeing correct solutions boosts students’ confidence, reassuring them that they are on the right track when solving similar problems in the future.
Effective Use of Provided Solutions
Rather than just copying the final answer, students should focus on understanding each step in the process. By carefully reviewing the solution methods, learners can recognize patterns and strategies they can apply to future problems. Additionally, understanding how to approach different types of questions equips students with the flexibility to tackle a wide range of challenges, enhancing their problem-solving skills and overall mathematical ability.
Improving Problem-Solving Skills
Enhancing problem-solving abilities is essential for mastering any subject, particularly mathematics. By developing a structured approach to tackling challenges, students can improve their critical thinking and analytical skills. Practicing various problem types and understanding the strategies used to solve them allows individuals to build confidence and competence in their abilities. With time, these enhanced skills extend beyond academics and can be applied to real-life situations as well.
Techniques to Strengthen Problem-Solving

- Break Down the Problem: Start by identifying what is being asked. Isolate the known information and the unknowns to structure your solution approach more effectively.
- Look for Patterns: Recognize repeating elements or structures within problems. Identifying patterns can often lead to a quicker and more efficient solution.
- Work Backwards: In some cases, reversing the process can help find a solution. By working backwards from the desired result, you may uncover useful steps that might otherwise be missed.
- Use Visual Aids: Diagrams, charts, and graphs can often clarify complex problems. Visualizing the problem allows for a more intuitive approach to finding solutions.
- Test Different Methods: If one approach doesn’t work, try another. Flexibility in problem-solving is essential, as different problems may require different strategies.
Building Consistency Through Practice
Consistency is key when it comes to developing strong problem-solving skills. Regular practice not only reinforces the techniques mentioned above but also helps in honing the ability to approach complex problems with confidence. Whether through exercises, real-world applications, or peer discussions, continuing to engage with new challenges will sharpen a student’s ability to tackle problems effectively and efficiently.
Course 3’s Approach to Algebra

The study of algebra in this particular level emphasizes developing a deep understanding of mathematical relationships and the structures that govern them. The approach focuses on building conceptual clarity through problem-solving, where students explore variables, equations, and expressions in a step-by-step manner. By addressing algebraic principles progressively, learners are encouraged to understand the underlying logic rather than just memorizing formulas or procedures.
Key Features of the Algebra Approach
- Focus on Conceptual Understanding: Students are introduced to algebraic ideas in a way that promotes comprehension. Rather than just performing operations, they are guided to understand the “why” behind each step.
- Real-World Applications: Algebraic concepts are applied to everyday situations, making abstract concepts more tangible and relevant. This practical approach helps solidify the importance of algebra in solving real-life problems.
- Building from Fundamentals: The foundation of algebra is solidified by emphasizing core principles, such as the distributive property, simplifying expressions, and solving equations, which are revisited throughout the curriculum to reinforce learning.
Developing Problem-Solving Skills in Algebra
One of the strengths of this method is its emphasis on critical thinking and problem-solving. Students are encouraged to tackle algebraic problems step-by-step, using logical reasoning and strategies such as substitution, factoring, and balancing equations. By regularly engaging in challenging problems, learners gain confidence in applying these skills to more complex algebraic scenarios.
Understanding Word Problems in Course 3
Word problems are an essential part of mathematics, as they help students apply mathematical concepts to real-life situations. In this level, the approach to word problems emphasizes breaking down complex scenarios into manageable steps. By identifying key information, recognizing patterns, and formulating equations, students develop the skills to solve practical problems efficiently. The goal is not only to arrive at the correct solution but to also build confidence in translating everyday situations into mathematical expressions.
Breaking Down the Problem
When tackling word problems, the first step is to understand what is being asked. This often involves reading the problem carefully to extract the important details, such as the numbers, units, and relationships. Once the essential components are identified, students can proceed to translate the words into mathematical operations. This process helps in structuring the problem logically, making it easier to solve.
Strategies for Solving Word Problems
- Identify Key Information: Pinpointing the relevant facts in the problem is crucial for forming the right equation.
- Translate Words into Mathematical Language: Converting word phrases into operations (e.g., “total” means addition, “difference” means subtraction) allows for clearer problem formulation.
- Check for Consistency: Once a solution is found, verifying it by checking the calculations or considering if the result makes sense in context is essential for ensuring accuracy.
Additional Resources for Course 3 Students
In order to enhance understanding and reinforce learning, students have access to a variety of supplementary materials. These resources help bridge gaps in knowledge, offer different perspectives, and provide opportunities for additional practice. From online platforms to textbooks and tutoring services, these tools offer support for tackling more challenging concepts and improving problem-solving abilities.
Online Platforms
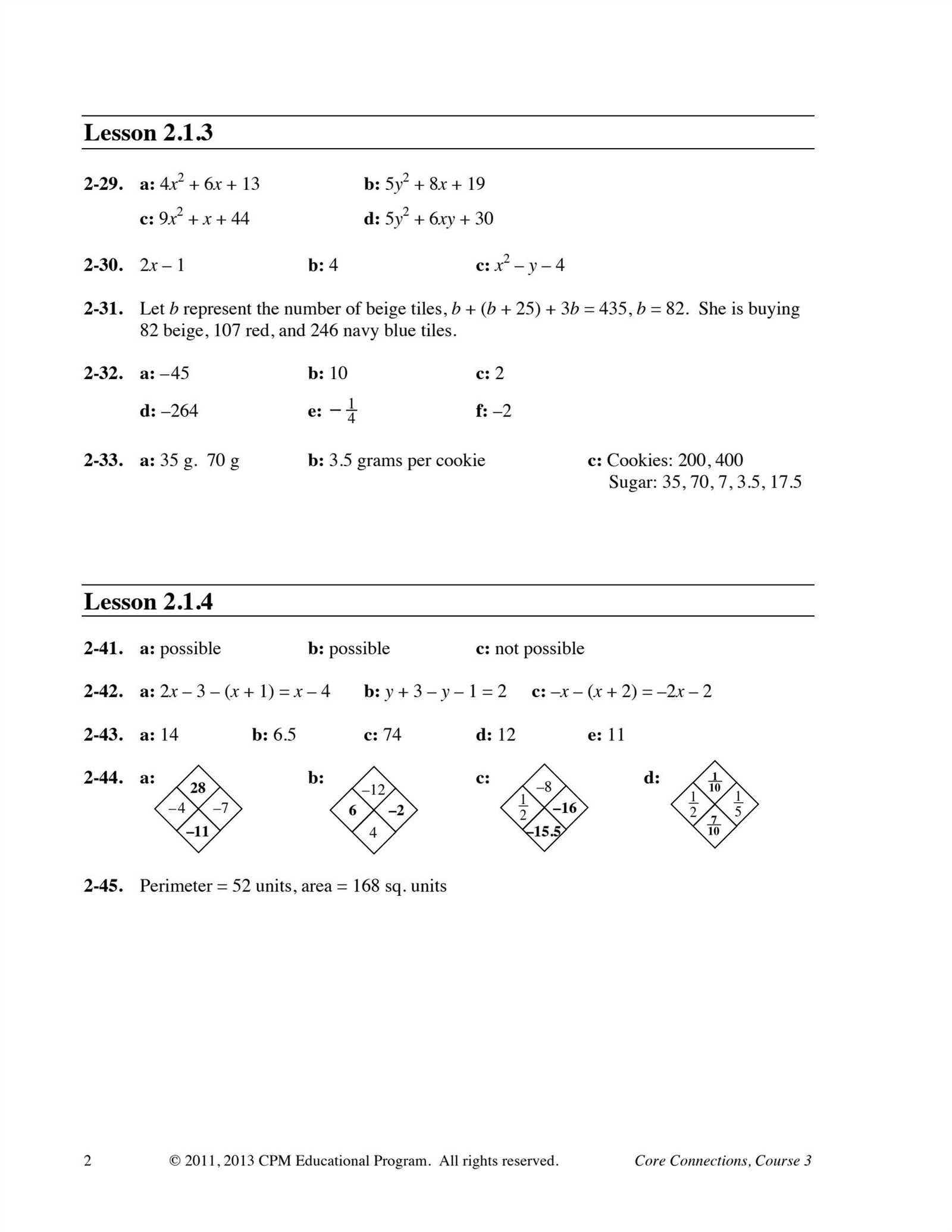
Many students benefit from interactive online platforms that provide instant feedback and step-by-step guidance. These resources can be accessed at any time, allowing students to learn at their own pace and revisit concepts as needed. Some platforms even offer video tutorials, practice problems, and live discussion forums where learners can collaborate.
Printed Resources
In addition to digital platforms, printed materials like workbooks and reference guides are valuable for reinforcing concepts. These resources provide structured exercises, examples, and additional challenges to deepen understanding. Teachers may also recommend specific textbooks that align with the curriculum.
Resource Type Benefits Online Practice Platforms Interactive Instant feedback, video lessons, practice problems Textbooks Printed Clear explanations, examples, in-depth theory Tutoring Services Personalized One-on-one guidance, problem-solving strategies Preparing for Assessments in Course 3
Proper preparation is essential for achieving success in assessments. To ensure thorough understanding and mastery of the material, students should adopt a proactive approach that involves consistent review, practice, and problem-solving. Effective preparation strategies can reduce anxiety and help improve performance when it’s time to test knowledge and skills.
Key Steps for Effective Preparation
- Reviewing Key Concepts: Revisit major topics and theorems covered during lessons. Make sure to understand the foundational principles before moving on to more complex material.
- Practicing Problem Sets: Solve practice problems from past assessments and exercises. This will help reinforce the learned concepts and give students the chance to familiarize themselves with different problem types.
- Time Management: Allocate enough time to each topic during study sessions. Break study time into manageable chunks and avoid cramming at the last minute.
Utilizing Available Resources
- Study Groups: Collaborating with peers in study groups can be a helpful way to discuss complex topics and clarify doubts.
- Instructor Support: Take advantage of office hours or reach out to instructors for clarification on any difficult areas.
- Additional Practice Materials: Explore extra exercises from textbooks or online platforms to gain more exposure to various types of questions.