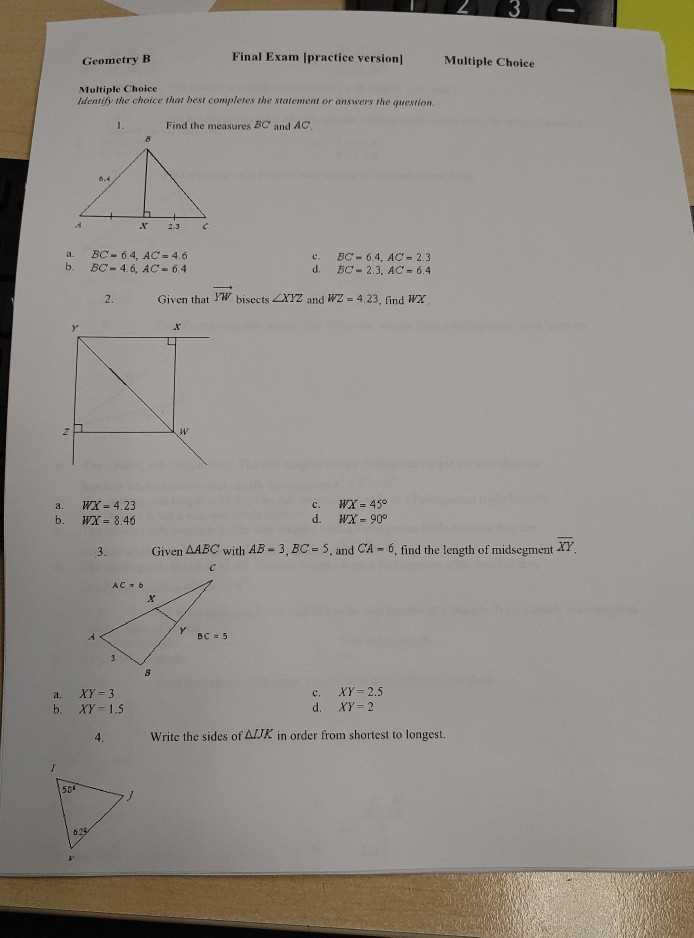
As you prepare for the upcoming assessment in your mathematics course, it’s crucial to focus on understanding the core principles and methods that will be tested. This section aims to guide you through the key topics and problem-solving techniques that are essential for success. With a structured approach, you can build confidence and ensure you’re ready to tackle various challenges that might arise during the assessment.
Mastering critical concepts is vital for achieving the best possible results. By reviewing important theories, formulas, and practical applications, you’ll be able to approach each task with a clear strategy. Whether you’re dealing with shapes, angles, or calculations, understanding the fundamental rules and their interconnections will help you solve problems more effectively.
Throughout this section, we will cover the most important areas, breaking down each one into manageable steps. With practice, repetition, and a focus on clarity, you will be able to handle even the toughest questions with ease. By the end of your preparation, you’ll have the tools and knowledge needed to perform at your best.
Geometry A Final Exam Review Packet Answers
To succeed in your upcoming assessment, it’s important to approach the material with a well-rounded understanding of the key topics. This section provides the essential solutions to the most common problems you may encounter, ensuring you’re prepared for the wide range of questions that may appear. By breaking down each problem into smaller, manageable steps, you can master the process and increase your confidence.
Key Problem-Solving Techniques
When facing complex questions, it’s important to focus on the fundamental techniques that guide you toward the correct solution. Start by analyzing the problem, identifying known values, and applying the appropriate formulas. A methodical approach to solving will make even the most challenging questions seem more approachable. Practice is key to mastering these techniques and reinforcing your understanding of core concepts.
Common Problem Types
While the types of problems may vary, certain categories are frequently tested and require specific strategies. Problems related to shapes, measurements, and algebraic manipulation are some of the most common. By recognizing patterns and familiarizing yourself with typical question formats, you can approach each one with a clear plan. Familiarity with these patterns will help you think critically and solve problems more efficiently.
Understanding the Exam Format
Familiarizing yourself with the structure and layout of the upcoming assessment is essential for successful preparation. Knowing how questions are presented and what types of problems to expect will allow you to plan your approach more effectively. By understanding the format, you can allocate your time wisely and tackle each section with confidence.
Types of Questions
The assessment is typically divided into several sections, each focused on different skills and problem types. Expect a mix of multiple-choice questions, short-answer problems, and more complex tasks that require detailed solutions. Recognizing the format of these questions beforehand can help you prepare strategies for each. Prioritize areas that are commonly tested to ensure you’re not caught off guard.
Time Management Tips
Time management is a crucial part of performing well under test conditions. Knowing how much time to dedicate to each section based on its difficulty will help you avoid spending too much time on any single problem. Allocate extra time for the more complex questions and be sure to leave time for reviewing your work before submitting your responses.
Key Concepts for Geometry Review
Understanding the core principles of the subject is crucial for solving a wide range of problems. Mastering these concepts allows you to tackle questions efficiently, recognizing patterns and applying appropriate methods. In this section, we focus on the essential ideas that form the foundation of the material, helping you to better prepare for the assessment.
Essential Theorems and Formulas
Being familiar with the most important theorems and formulas is critical for solving problems. These fundamental tools allow you to calculate distances, angles, areas, and volumes, which are often the focus of questions. Memorizing key equations and understanding when to apply them will give you a distinct advantage.
Properties of Shapes and Figures
A solid grasp of the properties of different shapes and figures will help you recognize their characteristics when solving problems. Knowing the relationships between sides, angles, and diagonals is essential for answering questions related to polygons, circles, and three-dimensional objects. Understanding these properties deeply will make it easier to visualize and solve complex problems.
Essential Formulas to Memorize
Having a set of key equations at your disposal is crucial for solving a wide variety of problems. These formulas provide the foundation for calculating dimensions, areas, volumes, and angles, and are often the basis of the questions you’ll face. Familiarity with these formulas will help you work through problems more quickly and accurately.
Important Area and Volume Formulas
Certain equations are essential when dealing with two-dimensional and three-dimensional figures. Here is a summary of the most frequently used formulas for calculating area and volume:
| Shape | Formula |
|---|---|
| Rectangle | Area = length × width |
| Circle | Area = π × radius² |
| Triangle | Area = 1/2 × base × height |
| Sphere | Volume = 4/3 × π × radius³ |
| Cylinder | Volume = π × radius² × height |
Key Theorems and Relationships
In addition to basic formulas, understanding theorems related to angles, lines, and triangles is also vital. For example, the Pythagorean theorem helps determine the relationship between the sides of a right triangle. Knowing these relationships ensures that you can solve problems involving angles, distances, and geometric figures with confidence.
Tips for Solving Proofs Efficiently
Solving logical proofs can be one of the more challenging tasks, requiring both a strong understanding of principles and an organized approach. The key to solving them quickly and accurately lies in breaking the problem down into manageable steps. By following a clear process and using the right strategies, you can navigate through proofs with greater ease.
Approach to Structured Reasoning
When solving proofs, start by clearly stating what is given and what you need to prove. Establishing a roadmap early on will guide your thinking and keep you focused. Follow these strategies to ensure you’re on the right path:
- Write down known information before starting, ensuring you don’t miss key details.
- Use diagrams whenever possible to visualize relationships between elements.
- Identify relationships like congruent angles, parallel lines, or right angles, which can help simplify the proof.
- Work backward from the conclusion if you’re unsure where to start, using logical steps to connect the given information to the desired result.
Common Strategies for Proofs
Here are some common techniques that can help you approach proofs more effectively:
- Direct proof: Start from known facts and build toward the conclusion logically.
- Indirect proof: Assume the opposite of what you are trying to prove, then show that it leads to a contradiction.
- Proof by contradiction: Assume the negation of the statement and derive a logical inconsistency.
- Using previously proven theorems: Apply known theorems or postulates to support your reasoning and conclusions.
By using these methods and staying organized, you’ll be able to tackle proofs more efficiently and increase your chances of success.
Geometry Vocabulary You Should Know
Mastering the terminology of this subject is key to understanding the concepts and solving problems efficiently. A solid grasp of the essential terms helps you identify relationships between figures, recognize patterns, and apply the correct methods when working through different types of problems. In this section, we highlight the most important vocabulary that you should be familiar with for success.
By learning these terms and understanding their meanings, you will be able to communicate more effectively and approach problems with clarity. From basic shapes to advanced properties, each term plays a crucial role in solving complex tasks. Here are a few fundamental terms to get you started:
- Angle: The space between two intersecting lines, measured in degrees.
- Parallel lines: Two lines that run in the same direction and never meet.
- Perpendicular lines: Two lines that intersect at a right angle (90°).
- Radius: The distance from the center of a circle to any point on its circumference.
- Congruent: Describes figures or shapes that have the same size and shape.
- Symmetry: When one part of an object or figure mirrors another part.
Understanding these terms will not only help you navigate problems more easily but also build a stronger foundation for more complex topics. As you continue to study, keep expanding your vocabulary to include additional concepts and their applications in different contexts.
Common Mistakes to Avoid in Geometry
While working through problems, it’s easy to make simple errors that can lead to incorrect solutions. Identifying and avoiding these common mistakes is key to improving your understanding and performance. In this section, we will highlight the most frequent pitfalls and provide tips on how to avoid them, helping you to approach each problem more confidently.
Frequent Errors in Problem-Solving
Many mistakes arise from misinterpreting the problem or overlooking important details. Here are some of the most common errors:
- Misapplying formulas: Always double-check that you are using the correct formula for the specific problem. Different shapes and situations require different equations.
- Forgetting units: It’s easy to forget to convert between units, especially when working with different measurements (e.g., from inches to centimeters). Make sure your units are consistent throughout the problem.
- Incorrect assumptions: Don’t assume that all angles or sides are equal unless explicitly stated in the problem. Always base your reasoning on the information given.
- Overlooking key details: Small but crucial details can sometimes be overlooked, such as a missing label or an assumption about parallel lines. Pay close attention to the diagram and the problem’s wording.
Tips for Avoiding Mistakes
To minimize errors and improve accuracy, consider these strategies:
- Read the problem carefully: Before starting, make sure you fully understand the question and what is being asked. Reread the problem if necessary.
- Draw clear diagrams: Visualizing the problem can help you identify important relationships and avoid confusion.
- Check your work: Always review your calculations and reasoning before finalizing your solution. A quick second look can catch many common mistakes.
- Practice regularly: The more you practice, the more familiar you will become with common problem types and the less likely you are to make careless errors.
Step-by-Step Guide to Word Problems
Word problems can often seem overwhelming, but breaking them down into manageable steps makes them much easier to solve. By following a systematic approach, you can transform a complicated situation into a clear, solvable task. In this section, we’ll walk through a structured method for tackling word problems effectively.
To solve word problems, it’s essential to read carefully, extract relevant information, and apply the correct formulas. Taking the time to organize your thoughts and approach each step methodically will improve both accuracy and efficiency.
Step 1: Understand the Problem
Before jumping into calculations, take a moment to read the problem carefully. Identify the key information, including what is given and what you are asked to find. Sometimes, the question will include extra details meant to distract you, so focus on the essential parts of the text.
Step 2: Identify the Variables and Units
Next, translate the words into mathematical expressions. Assign variables to unknown quantities and make sure you understand the units involved. Organizing this information will help you decide which formulas to use.
| Step | Action |
|---|---|
| 1 | Read the problem carefully and highlight key information. |
| 2 | Identify unknown variables and decide on the correct units for each quantity. |
| 3 | Set up an equation based on the given data and the relationships between quantities. |
| 4 | Solve the equation and check if the answer makes sense in the context of the problem. |
Step 3: Set Up the Equation
Once you’ve identified the variables, use the information in the problem to create an equation. Make sure that all relationships are clearly represented and that your equation reflects the logic described in the problem.
Step 4: Solve and Check Your Answer
After setting up your equation, solve for the unknown variable. Once you have an answer, double-check your work to ensure that your solution makes sense in the context of the problem. If necessary, verify your answer by plugging it back into the original problem to see if it fits.
Reviewing Angles and Their Properties
Understanding the different types of angles and their relationships is crucial for solving many types of problems. Angles are a fundamental concept that appear in various contexts, from basic shapes to complex figures. Knowing the properties of each type helps in analyzing and solving problems effectively.
In this section, we will explore the various kinds of angles, how they interact with one another, and the key properties that define them. From complementary to supplementary, each relationship plays an important role in geometric reasoning.
Types of Angles
There are several common types of angles you will encounter:
- Acute Angle: An angle less than 90 degrees.
- Right Angle: An angle exactly 90 degrees.
- Obtuse Angle: An angle greater than 90 degrees but less than 180 degrees.
- Straight Angle: An angle exactly 180 degrees, forming a straight line.
- Reflex Angle: An angle greater than 180 degrees but less than 360 degrees.
Angle Relationships
Angles often interact with each other in specific ways. Some of the most important relationships are:
- Complementary Angles: Two angles that add up to 90 degrees.
- Supplementary Angles: Two angles that add up to 180 degrees.
- Adjacent Angles: Two angles that share a common side and vertex but do not overlap.
- Vertical Angles: Angles that are opposite each other when two lines intersect. These angles are always equal.
By mastering these angle types and relationships, you will have a better understanding of how to approach problems that involve angles and their measurements. This knowledge is essential when working with shapes, lines, and figures in various geometric scenarios.
Triangles and Their Types Explained
Triangles are one of the most basic yet essential shapes in the study of spatial figures. They consist of three sides and three angles, and their properties form the foundation for many geometric concepts. Understanding the different types of triangles based on their sides and angles is crucial for solving a variety of problems and understanding more complex figures.
In this section, we will explore the classification of triangles, including their various types and the distinct properties that define each one. By grasping these concepts, you can easily recognize and work with triangles in a variety of geometric contexts.
Classification by Sides
Triangles can be categorized based on the lengths of their sides. Here are the main types:
- Equilateral Triangle: A triangle where all three sides have equal length, and all three angles measure 60 degrees.
- Isosceles Triangle: A triangle with at least two sides of equal length. The angles opposite these sides are also equal.
- Scalene Triangle: A triangle with all sides of different lengths, and all angles of different measures.
Classification by Angles
Triangles can also be categorized based on their angles. The main types are:
- Acute Triangle: A triangle where all three angles are less than 90 degrees.
- Right Triangle: A triangle where one of the angles is exactly 90 degrees.
- Obtuse Triangle: A triangle where one of the angles is greater than 90 degrees.
By understanding these types, you can easily determine the properties and relationships within a triangle, which is essential for solving a wide range of problems in geometry.
Solving Coordinate Geometry Problems
Working with points on a plane allows us to analyze relationships between geometric shapes and lines. By using a coordinate system, we can easily find distances, midpoints, slopes, and equations that describe various figures. This section will guide you through essential methods for solving problems involving coordinates and their applications.
Understanding the core principles behind coordinate-based problems is important for solving a wide range of challenges. Key concepts like distance between two points, slope of a line, and equations of lines can be applied to more complex situations. Below are some important tools and methods that can help you efficiently solve problems on a coordinate plane.
Distance Between Two Points
To find the distance between two points ((x_1, y_1)) and ((x_2, y_2)) on a coordinate plane, use the distance formula:
Distance Formula:
[
d = sqrt{(x_2 – x_1)^2 + (y_2 – y_1)^2}
]
- Step 1: Identify the coordinates of the two points.
- Step 2: Substitute the values of (x_1), (y_1), (x_2), and (y_2) into the formula.
- Step 3: Simplify the expression to find the distance.
Slope of a Line
The slope of a line is a measure of its steepness. It can be calculated using the slope formula:
Slope Formula:
[
m = frac{y_2 – y_1}{x_2 – x_1}
]
- Step 1: Identify two points on the line, ((x_1, y_1)) and ((x_2, y_2)).
- Step 2: Substitute these coordinates into the slope formula.
- Step 3: Simplify the result to find the slope, (m).
Midpoint Formula
The midpoint of a line segment is the point that is exactly halfway between the two endpoints. To find the midpoint, use the midpoint formula:
Midpoint Formula:
[
M = left(frac{x_1 + x_2}{2}, frac{y_1 + y_2}{2}right)
]
- Step 1: Identify the coordinates of the two endpoints.
- Step 2: Apply the values into the formula for both the x and y coordinates.
- Step 3: Simplify to find the midpoint, (M).
By applying these formulas, you can solve a wide variety of coordinate-based problems efficiently. Mastering these tools is essential for analyzing and solving real-world situations that involve shapes, distances, and slopes.
Understanding Circles and Their Equations
A circle is a fundamental shape in mathematics, defined by all points that are equidistant from a central point. Understanding its structure and the equation that represents it is crucial for solving various problems in spatial reasoning. The equation of a circle provides insight into its size, location, and relationship with other figures on a coordinate plane.
In this section, we will explore how to derive and work with the equation of a circle. You will learn how the center and radius of a circle are represented and how to use this equation for solving related problems.
The Standard Equation of a Circle
The most common form of a circle’s equation on the coordinate plane is the standard equation, which is written as:
Standard Form:
[
(x – h)^2 + (y – k)^2 = r^2
]
In this equation:
- (h, k): The coordinates of the center of the circle.
- r: The radius of the circle, which is the distance from the center to any point on the circle.
To use this equation, you simply need to know the center and radius of the circle. The equation allows you to quickly find any point on the circle or understand the geometric properties of the figure.
Converting to Standard Form
Often, problems will provide the equation of a circle in a different form. To work with these equations effectively, you may need to convert them into the standard form. This can involve completing the square or other algebraic techniques.
- Step 1: Expand and simplify the given equation.
- Step 2: Group the x-terms and y-terms.
- Step 3: Complete the square for both x and y terms to convert the equation to the standard form.
Once in standard form, you can easily identify the center and radius, allowing for a clear understanding of the circle’s properties. This process is essential for solving problems involving distances, tangents, and intersections with other shapes.
Understanding Volume and Surface Area
In the study of three-dimensional objects, two essential properties are volume and surface area. Volume measures the amount of space an object occupies, while surface area calculates the total area that the outer surface of the object covers. Both concepts are vital for a wide range of applications, from practical tasks like measuring liquid capacity to theoretical problems involving spatial relationships.
Understanding how to calculate these properties is key to solving problems that involve solids such as spheres, cylinders, and cubes. By mastering the formulas for volume and surface area, you can easily analyze and work with different 3D shapes.
Volume of Common Solids
Volume is typically measured in cubic units. Each type of solid has its own formula for calculating volume, which depends on the shape and dimensions of the object. Here are some common formulas:
- Cylinder: The volume is found using the formula V = πr²h, where r is the radius of the base and h is the height.
- Cube: The volume is given by V = s³, where s is the length of a side.
- Sphere: The volume is calculated as V = (4/3)πr³, where r is the radius.
- Rectangular Prism: The formula is V = lwh, where l is length, w is width, and h is height.
By using these formulas, you can easily find the volume of various objects as long as you know the necessary measurements.
Surface Area of Common Solids
Surface area refers to the total area of all the faces or outer surfaces of a 3D object. The calculation depends on the shape of the solid. The general approach is to find the area of each face and then sum them together. Below are the formulas for some common shapes:
- Cylinder: Surface area is calculated by A = 2πr² + 2πrh, where r is the radius and h is the height. This includes the areas of the two circular bases and the lateral surface.
- Cube: Surface area is A = 6s², where s is the length of one side.
- Sphere: The surface area formula is A = 4πr², where r is the radius.
- Rectangular Prism: The surface area is A = 2lw + 2lh + 2wh, where l, w, and h are the length, width, and height, respectively.
These formulas allow you to find the surface area of solids by adding up the areas of their individual faces. Whether you are working with containers, packaging, or other 3D objects, understanding how to calculate these properties is essential for many practical applications.
Mastering Transformations in Geometry
Understanding how shapes can be manipulated and moved within a given space is a fundamental skill. Transformations involve changing the position, size, or orientation of objects without altering their fundamental properties. By mastering these processes, you can solve complex problems and analyze spatial relationships more effectively.
There are several types of transformations that you will encounter, each with its own set of rules and techniques. These include translations, rotations, reflections, and dilations. Each transformation affects a figure in a unique way, but all maintain the integrity of the object’s shape and size in some way.
Translation: Shifting Objects
Translation refers to the movement of a shape from one location to another without rotating or resizing it. The object simply slides along a straight path. This transformation is defined by a vector, which specifies both the direction and distance the object will move. In mathematical terms, a translation involves adding or subtracting values to the coordinates of each point in the shape.
- Example: If a point A has coordinates (2, 3) and is translated by the vector (4, -2), the new coordinates of point A will be (6, 1).
Rotation: Turning the Shape

Rotation involves turning an object around a fixed point, known as the center of rotation. The object is moved through a specific angle, and the shape itself does not change. The key here is determining the angle of rotation and the center around which the object rotates. Rotations can occur in either a clockwise or counterclockwise direction, and the degree of rotation is typically specified.
- Example: A point (x, y) rotated 90 degrees counterclockwise around the origin (0, 0) will result in the new coordinates (-y, x).
Reflection: Flipping the Shape
Reflection involves flipping a shape over a line, known as the line of reflection. This creates a mirror image of the object. The position of the shape changes, but its size and orientation remain the same. Common lines of reflection include the x-axis, y-axis, and lines at 45-degree angles.
- Example: A point (x, y) reflected over the y-axis will result in the new coordinates (-x, y).
Dilation: Resizing Objects
Dilation involves resizing an object either by enlarging or reducing it while maintaining its shape. This transformation is defined by a center of dilation and a scale factor. The scale factor determines how much larger or smaller the object will become. A scale factor greater than 1 results in enlargement, while a factor between 0 and 1 results in reduction.
- Example: If a point (x, y) undergoes a dilation with a scale factor of 2, the new coordinates will be (2x, 2y).
By understanding these transformations, you can manipulate shapes in various ways, helping you solve problems that involve symmetry, congruence, and similarity. Mastery of transformations is key to becoming proficient in spatial reasoning and geometry-related tasks.
How to Approach Geometric Proofs
Solving logical arguments and demonstrating the truth of statements in a structured way is a fundamental skill in the study of shapes and their properties. The process involves starting with known facts, building logical steps, and arriving at a conclusion that follows from the premises. Understanding how to break down complex problems into simpler components is key to constructing a successful argument.
When tackling these problems, it’s important to approach them systematically. Start by identifying what is given and what needs to be proved. From there, you can use a variety of logical tools, such as definitions, postulates, and previously proven theorems, to build your proof step by step. Each statement in the proof should logically follow from the one before it, creating a coherent chain of reasoning.
Step-by-Step Process for Writing a Proof
Here’s a structured approach to solving these types of problems:
- Understand the Problem: Carefully read the problem and identify all given information and what you need to prove. Drawing a diagram can be extremely helpful.
- Write Down Known Information: List all the given facts, including any relationships, angles, or sides that are known. These will be the foundation of your proof.
- State What You Need to Prove: Clearly define the conclusion you must reach, making sure it is directly related to the given facts.
- Plan Your Approach: Think about what geometric concepts might apply to the problem, such as congruence, similarity, parallelism, or properties of angles. Determine which definitions, postulates, or theorems could help support your proof.
- Start the Proof: Begin your argument by making a logical first step. Use a valid mathematical principle to move from one statement to the next.
- Work Towards the Conclusion: Continue making logical steps, each one supported by facts, definitions, or previously proven results. Ensure that every statement is justified.
- Conclude the Proof: Once you have reached the statement that was required to be proved, finish with a clear conclusion such as “Therefore, we have proven that [the statement].”
Common Techniques and Strategies
Several strategies can make the process of proving statements more manageable:
- Use of Congruence and Similarity: Look for pairs of triangles that are congruent or similar, as these can provide useful relationships between sides and angles.
- Angle Relationships: Angle pairs such as vertical angles, alternate interior angles, and corresponding angles can often help establish the necessary conditions to prove a statement.
- Consider Special Figures: In problems involving quadrilaterals, circles, or other special shapes, recall the specific properties these shapes have, such as the sum of angles in a triangle or properties of parallel lines.
With practice, you’ll become more proficient at identifying the right approach for each problem and applying the correct logical reasoning to prove the required statements. The key is to stay organized, be patient, and focus on making each step of the proof logically valid.
Reviewing Symmetry and Geometric Shapes
Understanding the properties of different shapes and how they exhibit balance and repetition is essential in many mathematical fields. Symmetry, in particular, plays a critical role in recognizing patterns and understanding the structure of various figures. By exploring how certain shapes maintain uniformity through reflections, rotations, or translations, we gain a deeper insight into their inherent characteristics. This knowledge not only aids in solving complex problems but also enhances our ability to visualize and manipulate shapes effectively.
In this section, we will explore the different types of symmetry that exist in both two-dimensional and three-dimensional figures. Additionally, we will review key geometric shapes and their defining properties, offering a comprehensive understanding of how symmetry influences their characteristics and behavior.
Types of Symmetry
Symmetry is present in many common shapes, and recognizing it can simplify problem-solving. There are several types of symmetry that are essential to understand:
- Reflection Symmetry: A figure has reflection symmetry if it can be divided into two identical halves that are mirror images of each other.
- Rotational Symmetry: A shape has rotational symmetry if it can be rotated about a central point and still appear identical at certain angles.
- Translational Symmetry: A figure exhibits translational symmetry if it can be shifted (translated) along a straight path and still look the same.
- Glide Reflection Symmetry: A combination of reflection and translation, where a figure is reflected over a line and then shifted along that line.
Common Geometric Shapes and Their Properties
Various shapes have unique properties that define their structure and behavior in space. Here are some key figures to review:
- Circles: A circle has infinite lines of reflection symmetry. The center of the circle is equidistant from all points on the perimeter, and the figure exhibits perfect rotational symmetry about its center.
- Squares: A square has four lines of reflection symmetry and exhibits rotational symmetry at 90°, 180°, 270°, and 360°. All sides are of equal length, and all angles are right angles.
- Equilateral Triangles: These triangles have three lines of reflection symmetry and exhibit rotational symmetry at 120° and 360°.
- Rectangles: Rectangles have two lines of reflection symmetry and rotational symmetry at 180° and 360°. Opposite sides are equal in length, and all angles are right angles.
- Regular Pentagons: A regular pentagon has five lines of reflection symmetry and rotational symmetry at 72°, 144°, 216°, and 288°.
By reviewing these shapes and understanding their symmetries, you will develop a strong foundation for solving geometric problems that involve symmetry and spatial reasoning. Each figure’s symmetry can offer helpful clues and shortcuts when proving relationships or finding missing information within a given problem.
Preparing for the Final Exam Efficiently
To achieve success in any comprehensive assessment, effective preparation is key. It’s important to focus on both understanding core concepts and practicing the application of these ideas in various contexts. The more prepared you are, the more confident you will feel during the test, and the better you’ll be at solving problems quickly and accurately. Efficient preparation is not just about studying hard; it’s about studying smart and using your time wisely.
In this section, we will discuss strategies to help you organize your study sessions, identify important topics, and enhance your problem-solving skills. These methods will not only help you understand the material more deeply but also boost your ability to recall information when needed most.
Effective Study Strategies
- Break Down the Material: Divide the content into smaller, manageable sections. Tackle one topic at a time rather than cramming everything in one go. This will make your study sessions more productive and less overwhelming.
- Create a Study Schedule: Plan your study time in advance, making sure to allocate more time to areas where you feel less confident. Consistent study sessions are more effective than last-minute cramming.
- Focus on Key Concepts: Prioritize the most important topics. These often include fundamental principles and formulas that are frequently tested. Knowing these well will give you a solid foundation for tackling other problems.
- Practice Problem-Solving: Simply reading through notes is not enough. Practice solving problems regularly to reinforce your understanding. The more problems you solve, the better you will become at applying your knowledge under pressure.
Maximizing Retention and Focus
- Use Active Recall: Test yourself frequently without looking at your notes. This technique improves long-term retention and helps you identify gaps in your knowledge.
- Take Breaks: Avoid long, uninterrupted study sessions. Take regular breaks to refresh your mind and maintain focus. A 5-10 minute break every hour can greatly enhance your productivity.
- Teach Someone Else: Explaining concepts to a friend or family member helps reinforce your understanding. If you can teach it, you truly understand it.
- Stay Organized: Keep your study materials in order. Use color-coded notes or flashcards to organize concepts, formulas, and strategies. This visual organization helps your brain process and remember the material more effectively.
By implementing these strategies, you will be well on your way to achieving success. Remember that preparation is not about how many hours you study, but how effectively you use the time available. By staying focused, consistent, and organized, you will ensure that you’re ready for any challenge the test may present.