Mastering advanced mathematical principles and problem-solving strategies requires a deep understanding of the foundational techniques that shape complex exercises. This section aims to provide clarity on the essential topics covered in the third stage of the program, guiding learners through critical concepts that are fundamental to success.
Throughout the learning process, it is crucial to recognize how different elements are interrelated, allowing for more efficient approaches to challenges. By focusing on core strategies and sharpening analytical skills, students can significantly improve their ability to tackle even the most difficult problems. With the right mindset and resources, anyone can develop the expertise needed to navigate these advanced topics with confidence.
In this article, we will explore practical methods to enhance your problem-solving abilities, offering insights that will help you break down complex questions into manageable steps. Whether you’re reviewing past material or preparing for future challenges, understanding these techniques will empower you to reach your academic goals effectively.
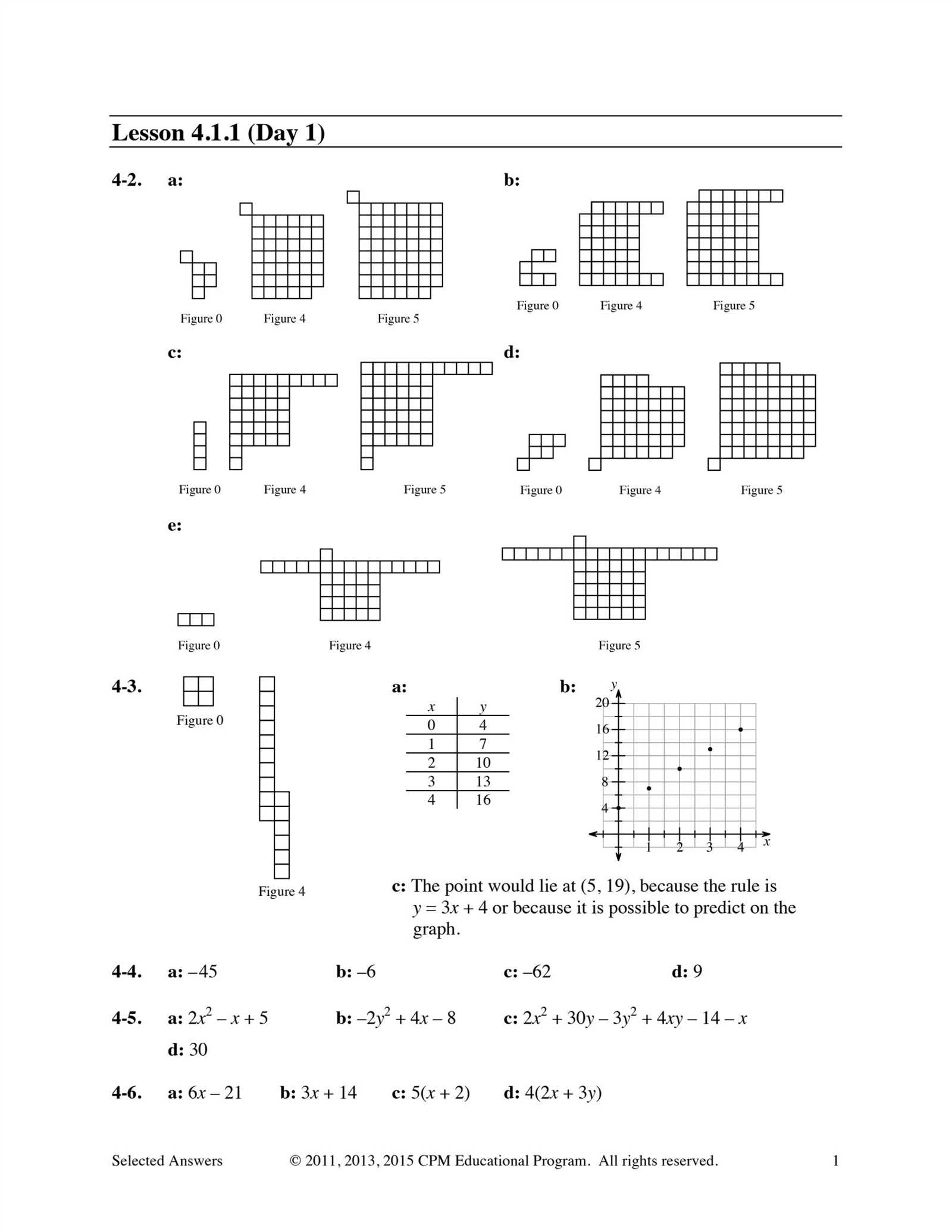
CPM Core Connections Course 3 Answers
In this section, we focus on breaking down the key principles and strategies that are essential to solving the exercises found in the third level of this educational program. By exploring how to effectively approach the problems and apply the right techniques, students can develop a more systematic and confident method for reaching solutions. It’s about more than simply obtaining the right results – it’s about understanding the logic and reasoning behind each step.
Approaching Complex Problems with Confidence
Successfully navigating these types of exercises requires a blend of analytical thinking and the ability to identify patterns. The problems may seem daunting at first glance, but with practice, students will learn to break them down into smaller, more manageable components. By taking the time to understand the problem structure, it becomes easier to apply the appropriate strategies and arrive at a solution.
Strategies for Effective Problem-Solving
Focusing on the process is crucial. Rather than rushing to find the correct answer, students should prioritize understanding the steps involved. This approach helps build a solid foundation that not only improves performance in the current exercises but also prepares learners for more advanced topics in the future. Developing the ability to check your work and verify the solution is an essential skill that can greatly enhance overall proficiency.
Key Concepts in CPM Core Connections

Understanding the fundamental ideas that underpin the material in the third stage of this program is essential for mastering more complex topics. The concepts covered build a strong foundation for learners, enabling them to solve intricate problems with greater ease and confidence. By grasping these key principles, students can improve their problem-solving abilities and tackle even the most challenging exercises.
Essential Mathematical Principles
One of the most important aspects of this stage is recognizing how mathematical principles interact with one another. By understanding the relationships between different concepts, learners can more easily navigate complex exercises. These principles form the backbone of the learning process, guiding students toward the right approach and making it easier to find solutions.
Problem-Solving Techniques
Developing a systematic approach to solving problems is another crucial element. Instead of relying solely on memorization, students are encouraged to understand the process behind each solution. This not only helps them solve problems more efficiently but also enhances their ability to adapt to new challenges. Effective problem-solving is a skill that can be honed through practice and strategic thinking.
| Concept | Explanation | Application |
|---|---|---|
| Patterns | Identifying recurring structures in problems | Helps break down complex problems into simpler parts |
| Relationships | Understanding how variables affect one another | Key for applying formulas and solving equations |
| Logical Reasoning | Drawing conclusions based on given information | Essential for step-by-step problem resolution |
Understanding Course 3 Answer Structure
Grasping the layout and approach behind the solutions provided in the third stage of this program is essential for success. The structure is designed not only to help learners arrive at the correct results but also to deepen their understanding of the underlying principles. By analyzing the way each solution is built, students can develop a more effective problem-solving strategy, ultimately improving their ability to approach future challenges with confidence.
Breaking Down the Solution Process
The key to mastering this section lies in recognizing the step-by-step process that guides each answer. Instead of rushing directly to the final result, learners should focus on the logical sequence of steps that lead to it. This structure ensures that all aspects of the problem are addressed thoroughly, making it easier to identify mistakes and rectify them. By following this structure closely, learners can also increase their overall efficiency in solving similar problems.
Critical Thinking and Application
Critical thinking plays a major role in understanding how each answer is formed. Every solution is not just a final answer but a combination of reasoning, applied techniques, and mathematical principles. The more students practice with this approach, the better they will become at breaking down problems and thinking logically. Applying this mindset across different topics will strengthen their analytical skills and prepare them for more advanced topics ahead.
Essential Skills for Course 3 Success
To excel in this stage of learning, students need to develop a specific set of skills that will enable them to tackle complex problems with ease. These abilities go beyond mere knowledge of concepts–they are about applying logical thinking, maintaining focus, and using strategies that foster understanding. Mastering these skills is crucial for achieving success and preparing for more advanced topics in the future.
Key Abilities for Effective Problem-Solving
Students should focus on cultivating the following skills to succeed:
- Analytical Thinking: The ability to break down complex problems into smaller, more manageable parts.
- Attention to Detail: Identifying key elements in each problem and ensuring accuracy in every step.
- Logical Reasoning: Understanding the sequence of steps needed to solve a problem and predicting outcomes.
- Time Management: Allocating enough time to work through each problem without rushing to conclusions.
Strategies for Strengthening Skills
In addition to these core skills, adopting certain strategies can greatly improve overall performance:
- Practice regularly to reinforce concepts and identify areas of improvement.
- Review past exercises to understand mistakes and learn from them.
- Seek clarification on difficult concepts to ensure a deep understanding.
- Collaborate with peers to exchange problem-solving techniques and strategies.
How to Approach Core Connections Problems
Successfully solving complex problems requires a strategic approach that involves careful analysis and methodical thinking. By breaking down each question into smaller, manageable components, learners can navigate through challenges more efficiently. Developing a clear plan before jumping into calculations or answers helps to stay organized and ensures a more logical progression toward the solution.
Start by thoroughly reading the problem and identifying the key information. Highlight the important data and determine what is being asked. Once you have a clear understanding, outline the steps needed to solve the problem. This will provide a roadmap for your work and reduce the chances of overlooking critical details. It’s essential to stay focused on the main goal while being open to adjusting your approach if necessary.
Tips for Mastering Course 3 Material
Achieving mastery in any subject requires both dedication and effective strategies. To excel in this stage of learning, it’s important to develop a deep understanding of the material while also honing your problem-solving techniques. Here are several practical tips that can help streamline the learning process and set you on the path to success.
Effective Study Habits
Building strong study habits is key to retaining knowledge and improving performance. Focus on the following techniques:
- Consistency: Set aside dedicated study time each day to reinforce concepts and review key material.
- Active Learning: Engage with the material by solving problems, asking questions, and summarizing key ideas.
- Practice with Purpose: Work through exercises strategically, focusing on areas where you need the most improvement.
Maximizing Understanding
To ensure you fully grasp the concepts, try these approaches:
- Break Down Complex Problems: Take each question step by step to avoid feeling overwhelmed.
- Seek Clarification: If a concept is unclear, ask for help or look for additional resources to deepen your understanding.
- Review and Reflect: Regularly go back to past lessons and problems to reinforce learning and identify areas for improvement.
Common Mistakes in Core Connections
When tackling complex exercises, it’s easy to make mistakes that can derail progress. Identifying these common errors early on is crucial for improving both understanding and performance. By being aware of the most frequent missteps, students can learn to avoid them and strengthen their overall problem-solving skills.
Rushing Through the Problem
One of the most common mistakes is rushing to find the solution without fully analyzing the problem. It’s important to take time to read the question carefully and understand what is being asked. Often, students skip important details, leading to incorrect answers. Slowing down and thinking through each step ensures a more accurate approach and helps avoid simple errors.
Neglecting to Check Work
Another frequent mistake is failing to check the work after completing a problem. Relying on first answers can be risky, as mistakes may go unnoticed. Taking the time to verify each step, even if it feels redundant, helps catch mistakes and solidify understanding. A thorough review ensures that all aspects of the problem have been addressed and prevents avoidable errors.
Analyzing Course 3 Questions Effectively
Effectively analyzing questions is crucial to solving complex problems accurately. It requires a focused approach to break down the components of each question and identify the key elements necessary for finding the solution. By thoroughly understanding what is being asked, students can avoid confusion and navigate through challenges with greater clarity.
The first step in analyzing a question is to extract all relevant information. Highlight key terms, numbers, and relationships, and then consider how these elements interact. Understanding the purpose behind each part of the problem helps create a clear path to the solution. By taking time to analyze before jumping into calculations, students can develop more precise and effective strategies.
| Step | Action | Purpose |
|---|---|---|
| Step 1 | Read the question carefully | Identify key elements and understand what is being asked |
| Step 2 | Highlight important information | Focus on terms, numbers, and relationships relevant to the solution |
| Step 3 | Break down the problem into smaller parts | Make complex problems more manageable and easier to solve |
Strategies for Solving Complex Exercises
When facing challenging problems, having a structured approach can make all the difference. Complex exercises often require more than just a basic understanding of concepts; they demand careful thought, logical reasoning, and a step-by-step method for breaking down the problem. Adopting specific strategies not only helps improve problem-solving skills but also increases efficiency and accuracy in finding the right solution.
Approach with a Clear Plan
Before diving into calculations or solving steps, take the time to map out a strategy. Start by analyzing the problem and determining what is being asked. Break the problem down into smaller, more manageable parts. By tackling the issue step by step, you can prevent feeling overwhelmed and ensure each component is addressed properly. Having a clear plan will help guide you through even the most difficult problems.
Use Logical Reasoning to Connect the Dots
One of the key strategies is to use logical thinking to connect different pieces of information. Often, complex exercises require combining various concepts or applying multiple methods in sequence. It’s crucial to recognize patterns and relationships between different elements of the problem. By using logical reasoning to connect the dots, you can discover how to approach the problem from different angles and find the most effective solution.
Time Management During Course 3
Effective time management is essential for success when tackling a demanding set of tasks. Managing your time wisely allows you to balance study, practice, and revision, preventing stress and ensuring consistent progress. By organizing your schedule and prioritizing tasks, you can allocate sufficient time to each area, improving both focus and efficiency.
To make the most of your time, it’s crucial to break down larger tasks into smaller, more manageable segments. Setting specific goals for each study session helps you stay on track and maintain motivation. Here are some practical tips for managing your time effectively:
- Set Clear Deadlines: Establish specific time frames for completing exercises or reviewing material to stay focused and avoid procrastination.
- Prioritize Tasks: Identify the most challenging or important tasks and tackle them first, when your energy and focus are at their peak.
- Use a Timer: Break study sessions into focused intervals, such as 25 minutes of work followed by a 5-minute break (Pomodoro technique), to maintain concentration.
- Eliminate Distractions: Minimize interruptions during study sessions to make the most of your time and improve the quality of your work.
- Review and Adjust: At the end of each week, assess your progress and adjust your schedule as needed to ensure you stay on target.
By employing these strategies, you can manage your time more effectively, ensuring that you stay organized and maintain a steady pace throughout your learning process.
How to Check Your Answers
Checking your work is a critical step in the problem-solving process. Even after completing a task, it’s important to review your solution to ensure that all steps are correct and that you haven’t overlooked any details. A thorough check can reveal errors, help solidify understanding, and improve your ability to apply concepts accurately.
The process of verifying your solution involves more than just looking over the final answer. It requires a careful examination of each step, making sure the logic, calculations, and reasoning align. Below are key strategies for reviewing your work effectively:
Step-by-Step Verification
Start by revisiting each part of the problem. Break the solution down into individual steps and confirm that you’ve followed the correct procedure. If necessary, retrace your logic and calculations to identify where things may have gone wrong.
Double-Check Calculations
Ensure that any mathematical operations are accurate. It’s easy to make simple errors, like miscalculating or forgetting a step. Use a calculator or alternate methods to verify numbers, especially if they seem unusually large or small.
| Step | Action | Purpose |
|---|---|---|
| Step 1 | Revisit the problem | Ensure a clear understanding of what was asked |
| Step 2 | Check the process | Confirm that each step was followed correctly |
| Step 3 | Verify calculations | Ensure accuracy in all numerical steps |
| Step 4 | Compare with known solutions | Cross-check your result with expected outcomes or examples |
By following these steps, you can ensure the accuracy of your work and build a stronger foundation for solving future problems. Taking time to check your answers will also increase your confidence and improve your problem-solving skills over time.
Using Visuals to Understand Core Concepts
Visual aids can significantly enhance the learning process by simplifying complex ideas and making abstract concepts easier to grasp. When working through challenging material, diagrams, charts, and graphs offer a clearer understanding, allowing learners to visualize relationships and patterns that may be difficult to comprehend through text alone. These tools can turn difficult concepts into more tangible ideas, helping reinforce learning and improve retention.
Incorporating visuals into your study routine can help break down complicated problems and highlight key elements. Whether you’re studying mathematical models, scientific processes, or abstract theories, visual representations provide a powerful way to process and apply the information more effectively. Below are some ways in which visuals can support your understanding:
| Visual Tool | Purpose | Benefit |
|---|---|---|
| Diagrams | Illustrate relationships between components | Clarify how elements interact within a system |
| Flowcharts | Visualize steps in a process | Help organize thought processes and identify steps |
| Graphs | Display numerical data trends | Provide a quick overview of patterns and relationships |
| Tables | Compare and contrast information | Make data more accessible and easier to understand |
By using these visual tools, you can gain a deeper insight into complex topics and organize information in a more digestible format. With practice, visual aids can become a vital part of your learning toolkit, helping you better understand key concepts and apply them effectively in problem-solving scenarios.
Reviewing Past Exercises for Better Results
Looking back at previous problems is one of the most effective ways to improve your skills and understanding of complex concepts. By revisiting old exercises, you can identify areas where mistakes were made, refine your approach, and strengthen your ability to tackle similar challenges in the future. This process not only helps reinforce the material but also builds confidence in your problem-solving abilities.
Reviewing past exercises allows you to spot patterns in your mistakes and gain insights into why certain solutions didn’t work. This reflection can lead to better strategies and a deeper comprehension of the subject matter. Below are key reasons why revisiting previous tasks is crucial for improvement:
- Identify Mistakes: Recognizing and understanding past errors helps you avoid repeating them and fine-tune your problem-solving methods.
- Strengthen Problem-Solving Skills: Revisiting challenges gives you the opportunity to apply new strategies and test different approaches, improving your technique over time.
- Reinforce Concepts: Repetition of problems enhances memory retention and deepens understanding of key principles.
- Build Confidence: Successfully solving previously challenging problems boosts your self-assurance and prepares you for future tasks.
By consistently reviewing and reflecting on past exercises, you create a cycle of continuous improvement. This habit ensures that you not only learn from your mistakes but also build upon your successes, leading to better overall results and mastery of the material.
Practical Applications of Core Connections
Understanding theoretical concepts is important, but applying them to real-world scenarios is where true learning begins. Practical applications of foundational knowledge help bridge the gap between abstract ideas and tangible results. By seeing how these concepts work in everyday situations, learners are better equipped to solve complex problems and make informed decisions. Whether in engineering, business, or daily life, applying learned principles can lead to enhanced problem-solving and innovation.
In many fields, the principles learned in structured studies are directly applicable to a wide variety of situations. The ability to connect theoretical concepts with practical challenges allows individuals to analyze, evaluate, and approach problems with a more strategic mindset. Here are a few ways in which foundational knowledge is applied in different fields:
Engineering and Technology
In engineering, the application of mathematical and scientific principles is essential for designing and improving technologies. Whether it’s calculating stress points in structures or optimizing the efficiency of systems, the ability to apply concepts learned in theory ensures precision and reliability in real-world applications.
Business and Economics
In business, concepts such as resource allocation, cost analysis, and strategic planning are regularly used to make informed decisions. By applying learned principles, entrepreneurs and managers can develop more effective business strategies, optimize operational processes, and improve profitability.
By integrating theory with practice, individuals develop a deeper understanding of the material, making it easier to see how abstract principles have practical value. This approach not only enhances learning but also prepares individuals to tackle challenges in diverse industries and real-life situations.
Understanding the Course 3 Framework
Having a clear understanding of the structure and methodology behind any learning program is crucial for success. The framework serves as the foundation for how material is organized, how concepts are introduced, and how learners can effectively progress through the content. A well-designed framework ensures that all essential topics are covered systematically, providing a strong base for further exploration and application.
The approach to structuring the material emphasizes clarity, logical progression, and an emphasis on key concepts. Here’s an overview of how the framework is designed to guide learners through the content:
- Clear Objectives: The framework outlines clear learning goals that guide the student through each step, making sure they understand the desired outcomes before proceeding.
- Step-by-Step Learning: Each concept is introduced in a manageable sequence, gradually building on prior knowledge to prevent overwhelm and reinforce retention.
- Practical Application: Throughout the framework, there is a focus on applying what has been learned to real-life scenarios, ensuring that the knowledge gained is both relevant and useful.
Understanding the framework is not just about knowing the structure; it’s about appreciating the rationale behind how it was designed to benefit learners. It helps students stay focused, build confidence, and apply what they’ve learned in meaningful ways. By following the framework, learners can engage with the material in a thoughtful, productive manner that enhances both understanding and skill development.
Resources for Further Study
Expanding your knowledge beyond the basic material can greatly enhance your understanding of key concepts and improve your ability to apply them. Whether you’re looking for additional practice, in-depth explanations, or a variety of perspectives, there are numerous resources available to support your learning journey. Utilizing these resources can provide you with a deeper insight into complex topics and help solidify your grasp of the material.
Here are some valuable resources to consider:
- Textbooks and Study Guides: Comprehensive textbooks often provide detailed explanations and additional examples. Study guides are also great for summarizing key points and offering practice problems to test your understanding.
- Online Platforms: Websites like Khan Academy, Coursera, and edX offer free and paid courses that cover similar topics. These platforms provide video tutorials, interactive exercises, and discussions that can help reinforce what you’ve learned.
- Peer Groups and Forums: Online communities, such as Reddit or Stack Exchange, allow learners to discuss challenges, share insights, and offer support. Engaging with others can provide new approaches and help clarify difficult concepts.
- Practice Exercises and Mock Tests: Regular practice is key to mastering any subject. Look for exercises that challenge you to apply what you’ve learned. Many websites provide mock tests that simulate the type of questions you may encounter.
By exploring these resources, you can broaden your knowledge and improve your skills, making your study experience more effective and rewarding.