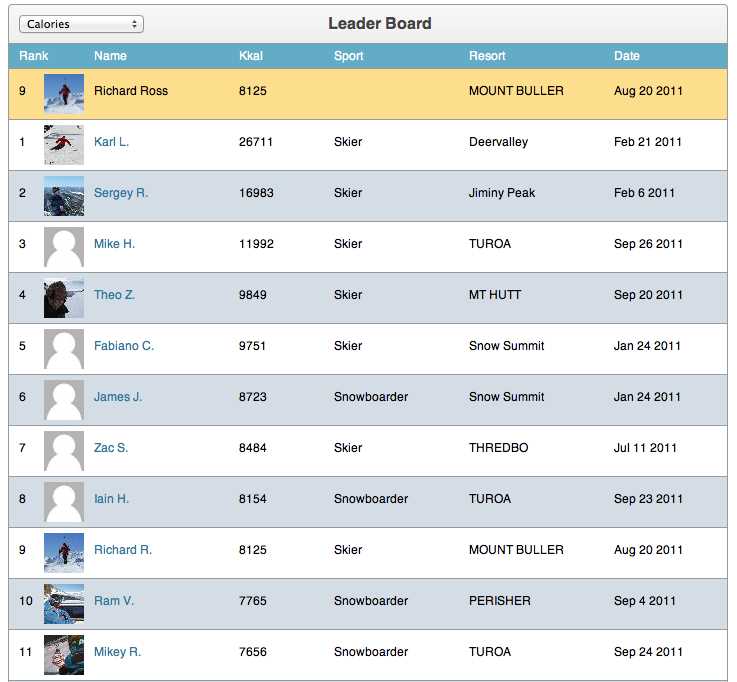
In the world of mathematics, self-assessment and continuous practice are crucial for mastering complex topics. Many platforms offer interactive tools that allow learners to engage with problems, assess their understanding, and improve their skills. This approach helps build confidence and reinforces learning through immediate feedback and targeted exercises.
Consistent practice not only helps solidify foundational knowledge but also challenges students to identify areas where they might need further improvement. By reviewing previous attempts, learners can pinpoint mistakes, understand where they went wrong, and apply the right strategies for future success.
Evaluating progress regularly is essential in tracking growth. These resources provide a structured way to test understanding, making it easier to stay on track and improve over time. Whether you are preparing for an exam or just trying to deepen your knowledge, these tools can be a valuable asset in your educational journey.
Comprehensive Guide to Algebra Nation
This section provides an in-depth overview of a powerful online tool designed to enhance mathematical understanding through interactive exercises. The platform offers students the opportunity to work through problems, gauge their progress, and sharpen their skills in a supportive environment. By engaging with the material actively, learners can grasp essential concepts and prepare for more advanced topics.
Key Features and Benefits
One of the standout aspects of this platform is its ability to offer personalized feedback and tailored practice. Users can access a wide range of problems that adjust in difficulty based on their performance, ensuring that every learner is challenged at the appropriate level. This approach not only makes learning more engaging but also helps students improve at their own pace.
How to Maximize Your Learning Experience
To get the most out of this resource, it is important to approach the exercises methodically. Regular practice, along with thoughtful review of mistakes, ensures steady progress. Utilizing the feedback provided for each problem will also help students refine their understanding and avoid repeating errors in the future. By setting goals and tracking improvement, learners can stay motivated and continue to build their skills effectively.
Understanding Algebra Nation Test Yourself Feature
This feature offers a dynamic way for learners to assess their comprehension and identify areas where they need improvement. By engaging with a variety of practice problems, students can measure their grasp of core concepts and reinforce their understanding through immediate feedback. The platform adapts to the learner’s progress, ensuring a personalized approach to skill development.
Interactive Learning and Feedback
One of the key strengths of this feature is its ability to provide real-time feedback after each attempt. As students work through problems, they receive explanations that clarify their mistakes and highlight the correct methods. This feedback loop not only enhances learning but also helps students recognize and correct misconceptions, making the learning process more effective.
Tracking Progress and Building Confidence
With consistent use, learners can track their progress over time. The feature allows them to revisit challenging problems and measure improvements, building both competence and confidence. Regular interaction with this tool helps ensure that students are well-prepared for future challenges, whether in assessments or practical applications of the material.
How to Access Algebra Nation Tests

Accessing the practice exercises on this platform is a straightforward process that requires only a few simple steps. Once you’re logged in, you can easily navigate through the available sections and select the activities that best suit your learning needs. The platform organizes exercises by topics, allowing you to choose specific areas for practice or work through general problem sets to assess your overall understanding.
Steps to Access Practice Problems
| Step | Description |
|---|---|
| 1 | Log into your account on the platform. |
| 2 | Navigate to the ‘Practice’ or ‘Exercises’ section of the site. |
| 3 | Browse through the available topics or search for specific areas you want to work on. |
| 4 | Select the problem sets that best fit your current skill level and goals. |
| 5 | Start solving problems and track your progress as you go. |
Once you’re in the practice area, you’ll be presented with a variety of exercises that vary in difficulty. Whether you’re focusing on basic concepts or more advanced material, the platform allows you to test your abilities and improve over time. Additionally, regular updates to the exercises ensure that you always have fresh content to challenge yourself with.
Why Algebra Nation Test Yourself Matters
Engaging with interactive practice exercises plays a vital role in solidifying mathematical concepts and improving overall problem-solving skills. This method not only helps students identify their strengths and weaknesses but also provides a deeper understanding of the material. By continually challenging oneself, learners can enhance their retention and application of key principles, preparing them for more advanced topics.
Benefits of Regular Practice
- Helps reinforce foundational knowledge
- Identifies areas that need further focus
- Improves problem-solving speed and accuracy
- Boosts confidence through consistent achievement
- Adapts to the learner’s skill level for personalized growth
Why Consistent Self-Evaluation is Crucial
- Immediate feedback clarifies misunderstandings
- Tracks progress and sets clear goals
- Enables targeted practice on weak areas
- Encourages active participation in learning
- Prepares for assessments and real-life applications
By regularly engaging with this feature, students create an ongoing feedback loop that not only highlights areas for improvement but also provides opportunities for targeted practice. This kind of focused approach leads to measurable improvements in performance and a better grasp of complex concepts, making it an essential tool for anyone looking to succeed in their studies.
Step-by-Step Instructions for Using Test Yourself
Using interactive practice tools is a straightforward process that can significantly improve your mathematical abilities. These exercises are designed to guide you through various problems, providing helpful feedback to enhance learning. By following a clear, step-by-step approach, you can ensure that you are making the most of the platform’s features to boost your understanding.
How to Get Started
- Log in to your account or create a new one if you don’t have one.
- Navigate to the practice section or find the “Exercises” area on the platform.
- Select the topic or category you wish to focus on, based on your current learning needs.
- Choose the difficulty level that matches your skill level or the area you want to improve.
- Start solving problems, ensuring that you carefully read each question and apply the appropriate methods.
Tips for Maximizing Your Learning
- Review feedback after each question to understand any mistakes.
- Revisit areas of difficulty by selecting similar problems for additional practice.
- Set small, achievable goals for each session to track your progress.
- Practice consistently to reinforce concepts and build confidence.
- Use the platform’s tools to adjust difficulty levels and focus on weaker topics.
By following these simple instructions, you can make your practice sessions more effective and efficient. Whether you are preparing for an exam or just trying to improve your skills, this resource provides a structured approach that ensures continuous progress.
Common Algebra Topics Covered in Tests
Practice exercises often span a wide range of fundamental mathematical concepts, focusing on areas that are essential for building a strong understanding. These exercises are designed to reinforce key skills that are vital not only for academic success but also for applying mathematical knowledge in real-world scenarios. The most common topics covered typically include foundational concepts, as well as more advanced applications that help students progress to higher levels of learning.
Some of the most frequently encountered subjects in these assessments include:
- Linear equations and inequalities
- Systems of equations and their solutions
- Polynomials and their operations
- Quadratic equations and factoring
- Exponents and radicals
- Rational expressions and their simplification
- Functions and their properties
- Word problems that involve various mathematical operations
Each of these topics plays a critical role in ensuring a comprehensive understanding of mathematical principles. By practicing these areas regularly, learners can improve their problem-solving abilities and gain the confidence needed to tackle increasingly complex material.
How to Interpret Test Yourself Results
Understanding the feedback from interactive exercises is essential for improving your mathematical skills. The results provide insights into your strengths and weaknesses, helping you identify which areas need more attention. By carefully analyzing your performance, you can make more informed decisions about where to focus your efforts in order to achieve better results in future challenges.
Key Metrics to Focus On
The results typically offer detailed information, highlighting both correct and incorrect responses. Pay special attention to the following aspects:
- Accuracy: The percentage of correct answers indicates how well you understand the material. A higher accuracy rate suggests a strong grasp of the concepts.
- Time Taken: How long it takes to complete each problem provides insight into your efficiency. If you take too much time on simple problems, it might indicate a need for more practice in that area.
- Areas of Difficulty: If you consistently struggle with specific types of problems, these areas should become your focus for further study and review.
Using Results for Improvement
When reviewing your results, it’s important to focus on patterns rather than isolated mistakes. If certain topics are consistently challenging, consider revisiting the foundational concepts related to those topics. Take time to work through similar problems to build a stronger understanding. By analyzing trends in your performance, you can adjust your study plan and track your progress more effectively.
Improving Your Mathematical Skills with Practice

Regular practice is one of the most effective ways to enhance your problem-solving abilities. Engaging with exercises that challenge your skills allows you to become more familiar with various problem types, improving both your speed and accuracy. By consistently practicing, you can gradually strengthen your understanding and overcome obstacles that may have seemed difficult at first.
Key Strategies for Effective Practice

- Start with the Basics: Focus on mastering foundational concepts before moving on to more complex material. A strong foundation will make it easier to tackle advanced problems.
- Vary Your Exercises: Practice a wide range of problem types to ensure you’re well-prepared for different scenarios. This will help you develop flexibility in your problem-solving approach.
- Review Your Mistakes: After completing exercises, take time to analyze any mistakes. Understanding why an answer was incorrect helps prevent similar errors in the future.
- Increase Difficulty Gradually: As you improve, challenge yourself with harder problems. This progressive approach builds confidence and keeps you engaged.
Tracking Your Progress
As you continue practicing, it’s essential to monitor your progress. Set achievable goals for each practice session and celebrate small victories along the way. Tracking improvements over time will motivate you to stay on track and push forward in your learning journey. Remember that persistence is key – the more effort you put in, the more noticeable your improvements will be.
Best Strategies for Successful Test Taking
Preparing for assessments requires more than just understanding the material. It involves adopting strategies that help you manage time effectively, stay calm under pressure, and approach each problem with confidence. By utilizing proven techniques, you can improve your performance and reduce test anxiety, allowing you to perform at your best when it matters most.
Effective Test-Taking Techniques
| Strategy | Description |
|---|---|
| Time Management | Allocate a specific amount of time for each section or question. Avoid spending too much time on any one problem to ensure you complete the entire assessment. |
| Read Instructions Carefully | Always read the instructions before starting. Understanding the requirements of each section helps avoid unnecessary mistakes. |
| Start with Easy Questions | Begin with the questions you find easiest. This builds momentum and boosts your confidence, leaving you more time for difficult problems. |
| Eliminate Obvious Errors | Quickly review answers for obvious mistakes, such as misreading questions or making simple calculation errors. |
| Stay Calm and Focused | If you encounter a challenging question, remain calm. Take a few deep breaths, and break the problem down step by step. |
Review and Reflection
After completing the assessment, take time to reflect on your performance. Review the questions you found challenging and identify areas where you could improve. Consistent reflection on past assessments helps build strategies for better results in the future. With practice and thoughtful preparation, you can become more adept at handling various types of challenges in future evaluations.
Identifying Key Problem Areas in Mathematics

Recognizing areas where you consistently struggle is a crucial step in improving your problem-solving abilities. By pinpointing the topics or types of problems that cause difficulty, you can focus your efforts on strengthening those skills. The key to mastering challenging material lies in understanding the specific obstacles and addressing them through targeted practice and review.
Common problem areas often include concepts that require a deeper level of understanding or involve multiple steps to solve. These areas can range from basic operations to more complex problem types. Identifying these issues allows you to isolate specific weaknesses and take proactive steps toward overcoming them.
Common Struggles in Mathematical Concepts
- Equation Solving: Difficulty in solving equations can arise from misunderstanding how to manipulate variables or apply rules consistently.
- Understanding Functions: The concept of functions, especially when dealing with transformations or compositions, can be a challenge for many learners.
- Word Problems: Translating real-world scenarios into mathematical expressions often causes confusion and errors in problem-solving.
- Graphing and Coordinates: Problems with graphing usually stem from a lack of understanding of the relationship between equations and their graphical representations.
- Fractions and Rational Expressions: Simplifying fractions and dealing with complex rational expressions can be a barrier for those still mastering these operations.
To improve in these areas, it’s important to break down problems into smaller, manageable steps. By consistently reviewing and practicing the problematic concepts, you can gradually build proficiency and confidence. The more focused and strategic your practice is, the quicker you’ll overcome these challenges.
Understanding Question Formats in Assessments
When preparing for an evaluation, it is crucial to familiarize yourself with the different types of questions you might encounter. Understanding these question formats can help you approach each one with the right strategy, increasing your chances of success. Whether the questions are multiple-choice, short answer, or involve problem-solving, knowing how to tackle them can make a significant difference in your performance.
Common Question Formats and Strategies
| Question Type | Description | Strategy |
|---|---|---|
| Multiple-Choice | These questions provide several possible answers, and you must select the correct one. | Eliminate obviously incorrect options first, then choose the most plausible answer from the remaining choices. |
| True or False | These questions require you to decide whether a statement is correct or incorrect. | Look for keywords in the statement that may indicate whether it’s true or false. Pay attention to extreme language like “always” or “never.” |
| Short Answer | These questions require a brief written response, often asking for a direct solution or explanation. | Be concise but thorough, making sure you answer exactly what is asked without going off-topic. |
| Problem-Solving | These questions require you to show your work and find a solution through logical steps. | Break the problem into smaller parts, and solve each part systematically. Check your work to avoid careless mistakes. |
| Fill-in-the-Blank | These questions ask you to complete a sentence or equation by filling in the missing word or value. | Use contextual clues from the sentence or equation to determine the correct term or number to fill in. |
By understanding these formats and practicing with similar questions, you will become more comfortable navigating assessments. This familiarity allows you to focus on solving the problems efficiently, rather than spending time figuring out what is being asked. Over time, you’ll develop the skills to confidently tackle any question that comes your way.
Analyzing Test Feedback for Better Learning
Feedback from assessments is an invaluable tool for understanding your strengths and areas that need improvement. By carefully reviewing the feedback, you can gain insights into specific areas where you may have made mistakes or struggled. This analysis helps identify patterns in your understanding and informs your future study approach, leading to more effective learning over time.
Rather than simply accepting the grades, it’s important to dig deeper into the feedback provided. Whether it’s detailed comments from an instructor or an automated score summary, using this feedback proactively will allow you to target your weaknesses and enhance your overall knowledge base.
Types of Feedback and How to Use Them
- Correct Answers and Explanations: Review the solutions provided for each question you got wrong. Understanding the correct approach will prevent similar mistakes in the future.
- Common Mistakes: Look for recurring errors, such as misinterpreting instructions or applying incorrect formulas. These patterns can guide you in adjusting your study methods.
- General Observations: Some feedback may highlight areas of improvement in your approach or problem-solving strategies. This type of feedback can help refine your techniques for tackling future exercises.
Turning Feedback into Actionable Steps
Once you’ve analyzed the feedback, it’s time to create a plan of action. This might involve revisiting certain topics or spending more time practicing specific problem types. Set clear goals based on the feedback and track your progress as you improve. The key is to use the feedback not only as a reflection of your performance but also as a guide for continuous growth and improvement.
How to Review Your Incorrect Responses
Reviewing incorrect responses is a crucial part of improving your understanding and mastering new concepts. It allows you to identify where things went wrong, correct misunderstandings, and reinforce your learning. Instead of simply acknowledging the errors, taking a systematic approach to review can help you avoid repeating the same mistakes in the future.
When you encounter a mistake, it’s important to understand why the answer was incorrect. Did you misinterpret the question? Did you apply the wrong method? Or was it simply a careless error? By pinpointing the exact cause of the mistake, you can adjust your approach to solve similar problems more accurately next time.
Steps to Effectively Review Mistakes
- Identify the Mistake: Start by figuring out exactly where you went wrong. Was it an error in calculation, logic, or a misunderstanding of the concept?
- Understand the Correct Method: Once you’ve identified the error, look at the correct solution. Study the steps taken to arrive at the right answer and understand the reasoning behind them.
- Practice Similar Problems: After reviewing, practice similar problems to reinforce the correct method. This will help you commit the right approach to memory and build confidence in applying it.
Utilizing Resources to Improve

If you continue to struggle with specific areas, seek additional resources like instructional videos, practice exercises, or guidance from an expert. Sometimes, a different explanation or approach can provide the clarity needed to fully grasp a concept. Continuously revisiting your mistakes and seeking out ways to improve will lead to better mastery of the material.
Tips for Tackling Difficult Math Questions
When faced with challenging problems, it can be easy to become overwhelmed. However, approaching tough questions with a clear strategy can significantly improve your chances of finding the correct solution. Breaking down the problem into smaller, more manageable parts and applying proven techniques will help you stay focused and increase your confidence.
The key to solving difficult questions is to remain patient and methodical. Often, the solution lies in understanding the fundamentals and applying them correctly. By organizing your thoughts and steps, even the most complex problems can become simpler to solve.
Effective Strategies for Problem Solving
- Read the Problem Carefully: Take the time to understand every aspect of the question. Look for keywords and important information that will guide you toward the right approach.
- Break the Problem Into Steps: Divide the problem into smaller tasks. Tackle each part one by one instead of trying to solve everything at once.
- Check for Patterns: Often, difficult problems are variations of simpler problems you’ve already solved. Look for similarities in structure or method.
- Work Backwards: For certain types of problems, starting with the answer and working backwards can provide insights into how to arrive at the correct solution.
- Use Visual Aids: Diagrams, charts, or graphs can often make abstract concepts more concrete and easier to comprehend.
Practice and Persistence
As with any skill, persistence and practice are essential for mastering difficult problems. Consistently working on complex questions, even when they seem challenging, will build your problem-solving abilities over time. Keep a positive attitude and approach each new challenge as an opportunity to grow.
Using Algebra Nation for Exam Preparation
Effective preparation for exams involves more than just reviewing notes and textbooks. Utilizing interactive tools and resources can make a significant difference in how well you grasp the material. One such resource is a comprehensive online platform that offers a range of practice exercises and instructional content designed to help reinforce concepts and improve understanding. These platforms allow students to test their knowledge, identify weak areas, and refine their problem-solving strategies in a focused, organized manner.
By consistently engaging with these online resources, students can build confidence and master challenging concepts. These tools provide structured practice sessions that mirror the types of questions likely to appear on exams, helping to familiarize users with both the content and format of the assessments.
Key Features of Online Platforms for Exam Prep
| Feature | Benefit |
|---|---|
| Interactive Practice Problems | Helps reinforce key concepts through active engagement. |
| Instant Feedback | Allows students to immediately learn from their mistakes and make corrections. |
| Progress Tracking | Provides insight into areas that need further attention and improvement. |
| Comprehensive Tutorials | Offers in-depth explanations for difficult concepts, making learning easier. |
Maximizing Your Results
To get the most out of online resources, it’s important to approach your study sessions with a clear plan. Start by identifying areas where you need the most help, then focus on those topics until you feel confident. Use practice exercises to reinforce learning and track your progress to ensure you’re improving over time. It’s also essential to review incorrect answers and reflect on the reasoning behind each mistake, as this process will help you avoid similar errors during the exam.
How Test Yourself Enhances Algebra Understanding
Interactive tools that allow students to assess their knowledge play a crucial role in reinforcing learning and identifying areas for improvement. By providing opportunities for self-assessment, these resources help to solidify key concepts and encourage deeper comprehension of mathematical principles. Engaging with such platforms can offer valuable feedback, highlight areas where further study is needed, and ultimately improve overall performance in challenging subjects.
When students actively engage with exercises that simulate exam conditions, they not only reinforce what they know but also learn how to approach unfamiliar problems. This process of self-evaluation fosters critical thinking skills and helps students develop a stronger grasp of the material over time.
Key Benefits of Self-Assessment Tools
- Immediate Feedback: Provides instant insight into mistakes, allowing for quick corrections and a deeper understanding of concepts.
- Reinforcement of Concepts: Continuous practice helps to solidify foundational knowledge, ensuring students retain key ideas.
- Identification of Weak Areas: Self-assessment helps pinpoint topics or skills that require additional attention, leading to more focused study sessions.
- Improved Problem-Solving Skills: Regular engagement with problem-solving exercises develops logical thinking and strategy formulation.
Effective Strategies for Maximizing Benefits
To make the most of these self-assessment tools, students should approach them with a strategy in mind. It is essential to review results carefully, noting patterns in mistakes or areas of confusion. Regular practice, combined with reflection on errors, ensures that weaknesses are addressed and that knowledge is strengthened. Additionally, taking the time to revisit difficult concepts outside of practice sessions further supports the learning process.
Tracking Progress and Setting Goals
In any learning journey, the ability to monitor one’s progress and establish clear objectives is crucial for sustained improvement. Tracking progress allows students to see how far they’ve come, while goal-setting provides motivation and direction. By regularly evaluating performance and setting achievable milestones, learners can make informed decisions about their next steps, ensuring that their efforts are aligned with long-term success.
Setting specific, measurable goals is essential for staying focused and on track. It also provides a sense of accomplishment as each goal is met, which can boost confidence and drive further progress. Combining progress tracking with goal-setting helps maintain a steady pace of improvement, encouraging continuous learning and development.
Effective Methods for Tracking Progress
- Regular Self-Evaluation: Periodically reviewing past exercises and identifying strengths and weaknesses can give insight into areas that need more focus.
- Record Keeping: Keeping a log of completed exercises and grades offers a visual representation of progress over time.
- Comparing Performance: Comparing results from different periods can highlight improvement trends and areas where additional practice may be needed.
Setting Clear and Achievable Goals
- Short-Term Goals: Focus on mastering specific concepts or skills within a set timeframe to maintain consistent progress.
- Long-Term Goals: Identify broader objectives, such as improving overall proficiency or preparing for an upcoming challenge, and break them down into smaller, actionable steps.
- Review and Adjust: Regularly assess your goals and adjust them based on your progress. Flexibility allows for adaptation to new challenges and ensures goals remain relevant.