
Preparing for an important assessment in mathematics can feel overwhelming, but with the right approach, you can tackle any challenge with confidence. A solid understanding of the subject matter is essential for success, and the key to achieving that lies in structured preparation and practice.
In this section, we will explore strategies to help you excel in your mathematical tests. From mastering essential concepts to refining problem-solving techniques, we aim to provide guidance that boosts your skills and ensures you are ready to face any questions that come your way.
Key topics will be covered, focusing on improving your ability to solve problems efficiently and effectively. With the right tools and a clear mindset, you’ll be well-equipped to perform at your best and demonstrate your knowledge.
Algebra 2a Final Exam Answers
When preparing for a challenging mathematical test, it’s crucial to understand the core principles that will be assessed. Success relies not only on memorizing formulas but also on developing a deep comprehension of how to apply these concepts in various scenarios. This section focuses on providing insight into the most common question types and strategies to approach them effectively.
Here are some key strategies to help you navigate complex problems:
- Identify the main concepts in each problem to focus your attention on the relevant formula or technique.
- Break down word problems step by step to ensure clarity in your approach.
- Practice solving equations involving multiple variables to gain confidence in manipulating expressions.
- Review different types of functions and their properties to apply them correctly when needed.
Additionally, reviewing common patterns in questions can help you anticipate what will be asked. Many questions in this area focus on:
- Linear equations and their solutions.
- Systems of equations and how to solve them efficiently.
- Quadratic functions and their graphing techniques.
- Rational expressions and simplifying them for easier calculation.
By mastering these areas, you’ll improve your ability to quickly identify the right approach during testing and apply your knowledge accurately to each question. The more you practice, the more intuitive these techniques will become, leading to better performance when it counts most.
Effective Study Tips for Algebra 2a
To succeed in mathematical assessments, a strategic study plan is essential. Consistency and focus are key to mastering complex concepts and solving problems efficiently. By using the right techniques, you can enhance your understanding and improve your ability to tackle challenging tasks during your preparation.
Organize Your Study Sessions
Effective learning begins with proper planning. Break down the material into manageable sections and create a schedule that allows you to cover each topic thoroughly. Instead of cramming, aim for shorter, focused study sessions with regular breaks. This will help your brain absorb and retain information more effectively.
Practice with Purpose
Practice is one of the most powerful tools for mastering mathematical concepts. Working through a variety of problems helps you become familiar with different problem types and strengthens your problem-solving skills. Focus on solving problems that challenge you, and revisit areas where you feel less confident. Regular practice will also help you recognize patterns and strategies, making it easier to approach new questions.
Additionally, reviewing mistakes is crucial. After solving problems, take time to understand where you went wrong and why. This reflection helps you avoid repeating errors and reinforces your learning. Over time, your ability to solve problems quickly and accurately will improve.
Understanding Key Algebra 2a Concepts

Grasping the fundamental principles behind mathematical problems is essential for successful problem-solving. A deep understanding of core concepts allows you to approach complex questions with confidence, as you’ll know how to apply different techniques based on the problem at hand. This section will help you familiarize yourself with some of the most important topics that you’ll encounter in this subject area.
Core Mathematical Principles
Several key areas form the backbone of this discipline. These include solving equations, understanding functions, and working with different types of expressions. Once these core concepts are mastered, it becomes much easier to navigate through more complicated problems. The following table outlines some important topics that you should focus on:
| Concept | Description | Importance |
|---|---|---|
| Equations | Solving linear, quadratic, and other types of equations. | Essential for finding unknown variables in problems. |
| Functions | Understanding how functions relate inputs to outputs. | Helps in graphing and analyzing relationships between variables. |
| Rational Expressions | Simplifying and solving problems involving fractions with variables. | Critical for handling complex expressions and equations. |
| Systems of Equations | Solving multiple equations simultaneously to find common solutions. | Important for dealing with multi-variable problems. |
Applying Key Concepts
Once you have a solid grasp of the key principles, the next step is to apply them to various problems. Practicing these concepts in different contexts will allow you to develop the skills necessary to solve any question confidently. Make sure to approach each problem step by step, ensuring that you understand the process behind each solution.
How to Tackle Difficult Questions
Facing challenging problems can be intimidating, but with the right approach, you can break them down into manageable steps. The key to solving complex tasks lies in staying calm, understanding the underlying concepts, and applying a methodical approach. This section will explore strategies that will help you confidently approach and solve even the toughest problems.
When confronted with difficult questions, it’s important to analyze the problem carefully. Start by identifying the core issue and the information provided. Breaking the problem into smaller parts allows you to focus on one step at a time, preventing confusion and mistakes. The following table outlines an effective approach to solving tough questions:
| Step | Action | Purpose |
|---|---|---|
| 1 | Read the question carefully | Ensure you understand the problem and what is being asked. |
| 2 | Identify known and unknown values | Clarifies what information is available and what needs to be solved. |
| 3 | Break down the problem into smaller parts | Helps simplify complex tasks and focus on individual steps. |
| 4 | Apply relevant formulas or methods | Ensures that the right techniques are used for each part of the problem. |
| 5 | Double-check your work | Reduces the likelihood of errors and confirms your solution is correct. |
By following this structured approach, you will be better equipped to handle difficult problems with confidence and accuracy. Practicing these techniques regularly will improve your ability to manage complex questions and provide correct solutions more efficiently.
Common Mistakes in Algebra 2a Exams
Even the most prepared individuals can make errors during assessments. Understanding the most frequent mistakes and knowing how to avoid them is crucial for improving performance. Many of these errors come from misinterpreting the question, overlooking important details, or rushing through the process without a clear plan. In this section, we will highlight the most common pitfalls and provide tips on how to prevent them.
Here are some common mistakes that often occur:
- Skipping steps in problem-solving, leading to incomplete or incorrect solutions.
- Misunderstanding the wording of word problems, which can cause confusion about what is being asked.
- Incorrectly applying formulas or techniques to problems that require a different approach.
- Forgetting to check answers or verify solutions, leading to missed errors that could easily be caught.
- Overlooking negative signs or misplacing parentheses, which can drastically alter the outcome of calculations.
- Rushing through questions and not managing time effectively, resulting in missed problems or careless mistakes.
By recognizing and addressing these common mistakes, you can significantly improve your accuracy and efficiency. It’s essential to take your time, stay organized, and double-check your work to avoid falling into these traps. Regular practice and awareness of these pitfalls will help you approach tasks more confidently and effectively.
Top Resources for Algebra 2a Help
When preparing for a challenging mathematical assessment, having the right resources can make all the difference. Whether you’re looking for online tutorials, practice exercises, or interactive tools, there are many platforms designed to help you improve your skills and gain a deeper understanding of the material. This section highlights some of the best resources available to assist you in your preparation.
Here are some of the top resources you can use:
- Khan Academy: Offers free, in-depth lessons and practice problems across various mathematical topics. Ideal for reviewing core concepts and tackling tough problems.
- Wolfram Alpha: A powerful computational tool that allows you to input equations and get step-by-step solutions, perfect for checking your work.
- Mathway: An online problem solver that provides instant solutions to a wide range of mathematical problems, helping you understand the process behind the answer.
- PatrickJMT: Provides clear, concise video tutorials on a variety of topics. Great for visual learners who want to see step-by-step solutions in action.
- IXL: An interactive learning platform with tailored exercises and progress tracking, helping you identify areas where you need more practice.
Utilizing these resources effectively can provide you with a solid foundation, help you grasp difficult concepts, and enhance your problem-solving skills. Regularly practicing with these tools will ensure that you are well-prepared for any challenges you may face during your studies.
Strategies for Time Management
Effective time management is essential when preparing for complex assessments. Organizing your time allows you to focus on what matters most, reduce stress, and maximize productivity. By adopting proven strategies, you can efficiently divide your study time, stay on track, and ensure that you approach each task with ample time for review and reflection.
Creating a Structured Study Schedule
A well-planned schedule is the foundation of good time management. By allocating specific times for each topic and adhering to that plan, you can avoid procrastination and ensure balanced preparation. Here’s how you can organize your study time effectively:
- Set clear goals: Define what you need to achieve in each study session, focusing on key topics and areas of difficulty.
- Prioritize tasks: Start with more challenging or unfamiliar topics when your focus is at its peak, and leave easier tasks for later.
- Incorporate breaks: Plan short breaks between study sessions to keep your mind fresh and prevent burnout.
- Stick to a routine: Consistency is key, so try to study at the same time each day to build a productive habit.
Managing Time During Assessments
Time management doesn’t stop once you’re in the middle of an assessment. Being able to manage the time you spend on each question is crucial. Here are some strategies to help you use your time wisely during an assessment:
- Quickly scan the questions: Before starting, glance through the entire assessment to gauge the difficulty of the questions and allocate time accordingly.
- Don’t linger too long on one question: If you’re stuck, move on and return to it later if you have time.
- Set time limits: Give yourself a set amount of time for each section or question, and stick to it to avoid spending too much time on one part.
- Leave time for review: Always ensure you have some time left at the end to review your work and make any necessary corrections.
By implementing these strategies, you can improve both your study routine and your performance during assessments. Time management is not just about working harder–it’s about working smarter and ensuring that you have the time and focus to succeed in every task.
Reviewing Key Formulas for Algebra 2a
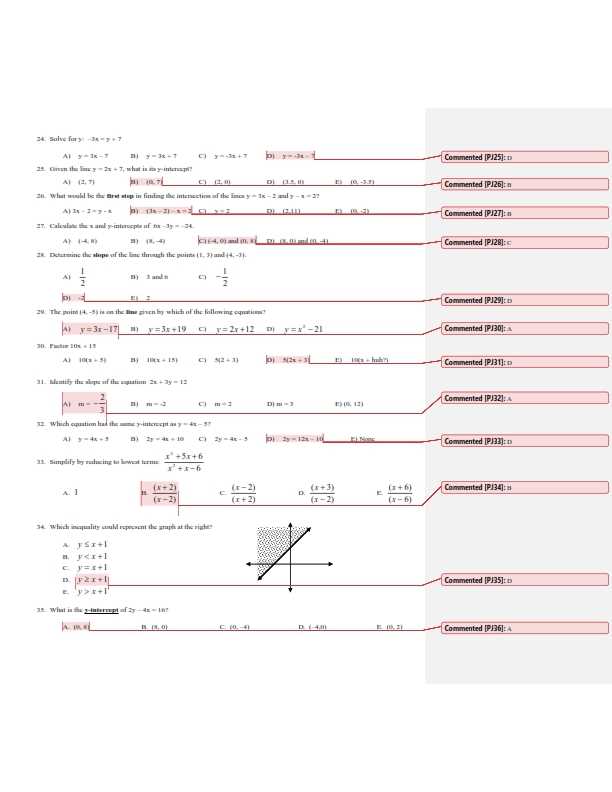
Mastering essential formulas is crucial for solving mathematical problems quickly and accurately. A solid understanding of these key equations allows you to approach various tasks with confidence. In this section, we will review some of the most important formulas that are commonly used and provide a summary of their applications. These formulas serve as the foundation for many problems and can help you navigate complex calculations effectively.
Below is a table that outlines some of the most frequently used formulas, their symbols, and typical applications:
| Formula | Symbol | Application |
|---|---|---|
| Quadratic Formula | x = (-b ± √(b² – 4ac)) / 2a | Used to find the roots of a quadratic equation. |
| Distance Formula | d = √((x₂ – x₁)² + (y₂ – y₁)²) | Calculates the distance between two points in a coordinate plane. |
| Slope Formula | m = (y₂ – y₁) / (x₂ – x₁) | Used to find the slope of a line given two points. |
| Pythagorean Theorem | a² + b² = c² | Used to find the lengths of sides in a right triangle. |
| Exponential Growth Formula | y = a(1 + r)ⁿ | Describes growth over time, such as population or compound interest. |
| Logarithmic Formula | logₐ(b) = c | Used to solve for exponents in equations involving powers. |
These formulas are foundational to solving many types of problems, and regularly reviewing them will help you become more efficient and accurate when applying them. Understanding when and how to use each formula is key to performing well in assessments and solving complex problems with ease.
Importance of Practice Tests
Practice tests play a vital role in preparing for any assessment. They not only allow you to familiarize yourself with the format and types of questions you will encounter but also help you identify areas where you may need further review. By simulating the conditions of the actual test, practice tests can reduce anxiety and improve performance on the real day.
Here are several reasons why practice tests are essential for your preparation:
- Builds confidence: Regularly completing practice tests boosts your self-assurance, as you become more comfortable with the content and time constraints.
- Identifies weak points: These tests highlight areas where you may struggle, allowing you to focus on specific topics that need additional attention.
- Improves time management: Practice tests teach you how to allocate your time effectively, ensuring that you don’t spend too much time on any single question.
- Reinforces learning: Repeated practice strengthens your understanding of key concepts and helps you retain information better over time.
- Simulates real conditions: By practicing under similar conditions, you get a feel for the actual assessment environment, which helps reduce stress on test day.
Incorporating practice tests into your study routine is a proven strategy for improving performance. The more you practice, the more prepared you’ll be, not just for the content, but also for the experience of taking the assessment itself.
How to Solve Word Problems Efficiently
Word problems can often seem overwhelming, but with the right approach, they become much more manageable. The key to solving them efficiently lies in breaking the problem down into smaller, more digestible steps. By understanding the problem’s structure, identifying important information, and applying systematic strategies, you can reach the correct solution with confidence and clarity.
Follow these steps to solve word problems more effectively:
- Read the problem carefully: Take the time to understand what the question is asking. Identify the key information and ignore irrelevant details.
- Define variables: Assign variables to unknown quantities. This makes the problem more structured and easier to solve.
- Set up equations: Translate the words into mathematical equations. Use the relationships between the quantities described in the problem.
- Look for clues: Identify keywords and phrases that indicate mathematical operations, such as “total,” “difference,” or “per.”
- Break the problem into parts: If the problem is complex, split it into smaller steps. Solve each part before combining them to find the final solution.
- Check your work: Once you’ve reached a solution, go back and verify your calculations to ensure accuracy. Make sure the answer makes sense in the context of the problem.
By practicing these steps, you will become more proficient at tackling word problems. The more you practice, the more intuitive these strategies will become, allowing you to solve problems efficiently and with greater ease.
Approaching Systems of Equations
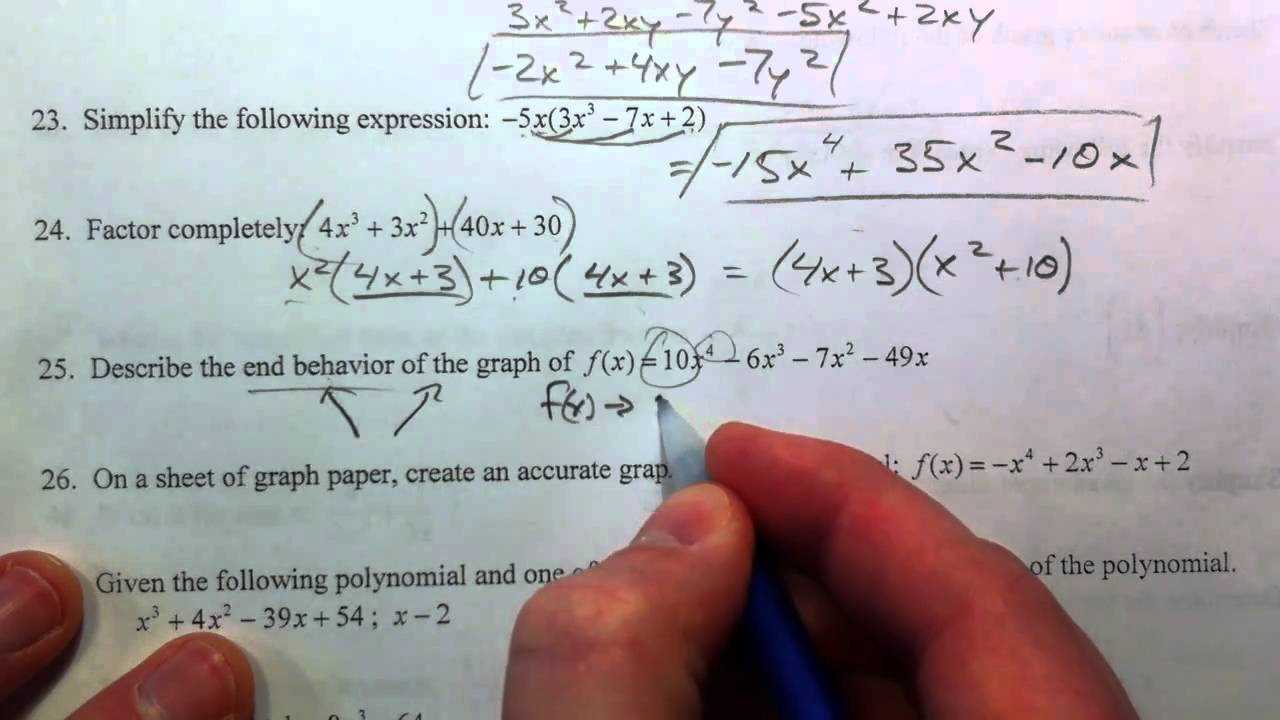
Systems of equations present a set of problems that involve multiple relationships between variables. These types of problems require finding values for the variables that satisfy all the equations simultaneously. The key to solving systems of equations is to choose an appropriate method, such as substitution or elimination, based on the structure of the system.
Methods for Solving Systems
There are three primary methods for solving systems of equations: substitution, elimination, and graphing. Each method has its own advantages depending on the nature of the problem:
- Substitution: This method involves solving one equation for one variable and then substituting that expression into the other equation. It’s particularly useful when one equation is easy to solve for a single variable.
- Elimination: The elimination method involves adding or subtracting the equations in order to eliminate one of the variables, making it easier to solve for the remaining one. This method is efficient when the equations are already aligned with similar terms.
- Graphing: By graphing both equations on a coordinate plane, the solution is where the two lines intersect. This method provides a visual representation of the solution but is less precise without exact graphing tools.
Choosing the Right Method
When deciding which method to use, consider the complexity of the system. If the system involves straightforward coefficients, elimination might be the fastest. If one equation is already solved for a variable, substitution might be the simplest option. Graphing can offer a quick visual check but is often less accurate for exact solutions.
Practice with different types of systems will help you develop an intuition for which method works best in any given situation. By mastering these techniques, you’ll be able to approach systems of equations with greater confidence and efficiency.
Graphing Techniques for Algebra 2a
Graphing is a powerful tool used to visualize mathematical relationships between variables. It helps you understand how equations behave and provides a clear picture of their solutions. Whether dealing with linear, quadratic, or more complex functions, mastering graphing techniques will enhance your ability to interpret and solve problems efficiently.
Key Graphing Techniques
There are several graphing techniques that are essential for solving various types of equations:
- Plotting Points: For simple equations, plotting points can help you visualize the graph. By substituting different values for the variable, you can plot corresponding points and connect them to see the shape of the graph.
- Intercepts: Finding the x- and y-intercepts of an equation provides crucial information about where the graph crosses the axes. This method is particularly useful for linear and quadratic equations.
- Slopes and Curves: For linear functions, understanding the slope helps you draw the line accurately. For nonlinear functions, identifying the curvature is key to understanding the graph’s shape.
- Transformations: Shifting, reflecting, and stretching graphs can help you visualize how changes to an equation affect its graph. These transformations are essential when dealing with more complex functions.
Graphing in Practice
To improve your graphing skills, consider the following steps:
- Start with the basic equation: Write the equation in a simple form that allows you to easily identify key points, slopes, or intercepts.
- Use graphing tools: A graphing calculator or graphing software can help you plot the equation accurately, especially for more complicated functions.
- Label key features: Mark the intercepts, vertices, or asymptotes clearly on the graph to make interpretation easier.
Regular practice with graphing will help you quickly identify trends, understand the behavior of different equations, and solve problems with greater ease.
Graphing Table Example
Here’s an example of how to organize the data for a simple linear equation:
| x | y |
|---|---|
| -2 | 4 |
| 0 | 2 |
| 2 | 0 |
By plotting these points, you can easily graph the equation and understand its linear behavior. With enough practice, these techniques will become second nature, allowing you to tackle more complex problems with confidence.
Using Technology for Algebra 2a Practice
In today’s world, technology offers a wide range of tools to enhance the learning process and make practicing mathematical concepts more engaging and efficient. From interactive software to online platforms, technology can be an invaluable resource for mastering complex topics. These tools provide instant feedback, help visualize problems, and allow students to practice at their own pace, ensuring deeper understanding and improved performance.
Benefits of Using Technology
Technology helps streamline the learning process and offers several benefits for students:
- Interactive Practice: Many platforms allow students to practice problems interactively. This hands-on approach ensures that learners remain engaged and can focus on areas that need improvement.
- Instant Feedback: Online tools and apps often provide immediate feedback, allowing students to quickly identify mistakes and adjust their methods.
- Personalized Learning: Technology allows learners to progress at their own pace, offering tailored exercises based on individual skill levels and needs.
- Visualizing Complex Concepts: Graphing tools and simulation software can help visualize abstract concepts, making them more tangible and easier to understand.
Popular Tools for Practice
Here are some widely-used tools and platforms that can aid in practicing mathematical problems:
- Graphing Calculators: Tools like Desmos or GeoGebra allow students to graph functions, explore their behavior, and understand how equations change with different parameters.
- Online Problem Solvers: Websites such as Khan Academy and Wolfram Alpha offer a vast array of practice problems with step-by-step solutions.
- Math Apps: Mobile apps like Photomath and Microsoft Math Solver provide quick solutions to problems and often include detailed explanations to help students learn from their mistakes.
- Online Courses and Videos: Platforms such as Coursera, Udemy, and YouTube provide tutorials and full courses on a variety of mathematical topics, helping reinforce difficult concepts.
By utilizing these tools, students can deepen their understanding, reinforce their learning, and enhance their problem-solving skills. Incorporating technology into study routines makes learning more dynamic and can help boost confidence in tackling challenging material.
Common Algebra 2a Theorems to Know
In advanced mathematical studies, several fundamental theorems serve as the building blocks for solving complex problems. These theorems provide essential guidelines and principles that help students navigate through various topics. Understanding and applying these key concepts not only boosts problem-solving abilities but also strengthens one’s grasp of broader mathematical relationships and structures.
Among the most important theorems are those related to polynomial behavior, systems of equations, and function properties. Mastery of these principles enables students to tackle a wide array of mathematical challenges with confidence. Below are some of the most important theorems that students should familiarize themselves with:
- The Fundamental Theorem of Algebra: This theorem states that every non-constant polynomial equation has at least one complex root. Understanding this concept is essential for solving higher-degree polynomial equations.
- Quadratic Formula: The quadratic formula provides a method for solving any quadratic equation and is crucial for handling second-degree polynomials.
- Binomial Theorem: This theorem describes the expansion of powers of binomials, which is a core concept for working with polynomials and combinatorics.
- Rational Root Theorem: This theorem helps identify potential rational solutions to polynomial equations by using the factors of the constant term and the leading coefficient.
- Intermediate Value Theorem: It states that if a continuous function has values of opposite signs at the endpoints of an interval, then it must have a root within that interval. This is useful for solving equations and understanding continuous functions.
These theorems form the backbone of many advanced topics. Having a solid understanding of their principles allows students to approach more complex problems with ease and ensures greater success in mastering mathematical concepts.
How to Stay Calm During the Exam
When faced with an important assessment, it’s natural to feel pressure. However, maintaining composure is key to performing at your best. Staying calm allows you to think clearly, make better decisions, and manage time effectively. There are various techniques you can use to maintain your focus and calmness throughout the process.
Here are some strategies to help you stay calm and focused:
- Deep Breathing: Take slow, deep breaths to relax your mind and body. This simple technique helps reduce anxiety and improves concentration.
- Positive Visualization: Visualize yourself successfully completing the assessment. Imagine solving problems with ease and feeling confident throughout the process.
- Break the Test Into Sections: Instead of focusing on the entire task, break it into smaller sections. Tackle each part individually, which can make the overall assessment seem more manageable.
- Stay Organized: Keep track of time and work through the questions in an organized manner. Mark questions you find challenging and return to them later, ensuring that you don’t waste time on one question.
- Stay Hydrated: Bring water and stay hydrated during the assessment. Dehydration can affect cognitive function and increase stress levels.
Implementing these methods will help you maintain clarity and control, making the entire process smoother and more manageable. By staying calm, you give yourself the best chance to perform at your highest level and finish with confidence.
Boosting Confidence for Final Exams
Approaching a major assessment with confidence can significantly improve performance. Building self-assurance before tackling a challenging task is essential for success. Whether it’s through preparation, positive thinking, or managing stress, there are several ways to boost your confidence and approach the test with a clear and positive mindset.
Here are some effective methods to help you increase your confidence before facing any major test:
- Consistent Practice: The more you practice, the more confident you become. Work through problems regularly and review key concepts. This consistent effort reinforces your understanding and minimizes uncertainty.
- Positive Self-Talk: Replace negative thoughts with empowering affirmations. Remind yourself of your strengths and the work you’ve put in. Positive thinking can reduce anxiety and build self-belief.
- Simulate Real Test Conditions: Practice under timed conditions to mimic the actual test. This helps you get used to the pressure and manage your time more effectively.
- Get Enough Rest: A well-rested mind is more focused and alert. Make sure to get a good night’s sleep before the day of the assessment to enhance your cognitive function and performance.
- Review Mistakes: Go over any mistakes you’ve made in practice tests. Understanding why you got something wrong and correcting it will improve your skills and increase your confidence for the real test.
By incorporating these strategies into your preparation, you can walk into the assessment feeling more confident, focused, and ready to perform your best. Confidence comes from preparation and a positive mindset, so approach each test as an opportunity to showcase what you’ve learned.
Why Algebra 2a is Important for Future Studies
The skills and knowledge gained from advanced mathematical courses provide a strong foundation for future academic pursuits and practical problem-solving. Understanding key concepts such as equations, functions, and graphing techniques is crucial for success in various fields, including engineering, economics, science, and technology. The ability to think analytically and solve complex problems is not only valuable in the classroom but also in real-world applications.
Developing Critical Thinking and Analytical Skills
One of the primary reasons this subject is essential for future studies is that it enhances critical thinking and analytical reasoning. By working through mathematical problems, students learn how to approach challenges logically, break down complex tasks into manageable steps, and evaluate multiple solutions. These skills are transferable to many fields of study, from physics to data science, making them indispensable for students pursuing careers in technology, healthcare, and finance.
Building a Strong Foundation for Higher Education
In many higher education programs, particularly in the STEM (Science, Technology, Engineering, and Mathematics) fields, the principles learned in advanced mathematical coursework are crucial. Concepts such as systems of equations, functions, and inequalities are building blocks for more advanced studies in subjects like calculus, physics, and computer science. Without a solid understanding of these concepts, students may struggle with more complex material in their college courses.
By mastering these foundational skills now, students are better prepared for future academic challenges and can pursue a wide range of career opportunities that require strong mathematical knowledge and problem-solving abilities.
Key Topics to Review Before the Exam
To ensure a successful performance in any academic assessment, it is important to review and consolidate key concepts learned throughout the course. This process helps reinforce understanding, identify areas that need further practice, and build confidence. By focusing on the essential topics, students can approach the challenge with a clearer mindset and improved problem-solving skills.
When preparing for assessments in advanced mathematics, certain areas require more attention due to their complexity and their role as foundational elements for solving various types of problems. These topics form the core of the curriculum and are frequently tested. Below are some of the critical concepts to review:
- Equations and Inequalities: Understanding how to solve linear, quadratic, and higher-order equations, as well as inequalities, is crucial for tackling a wide range of problems.
- Functions: Review different types of functions, including linear, exponential, and quadratic, and understand how to analyze their graphs and equations.
- Systems of Equations: Be prepared to solve systems of equations using various methods such as substitution, elimination, and graphical solutions.
- Polynomials: Understand operations with polynomials, including addition, subtraction, multiplication, and division, as well as factoring and solving polynomial equations.
- Rational Expressions: Practice simplifying, multiplying, dividing, and solving problems involving rational expressions and equations.
- Radicals and Exponents: Ensure mastery of simplifying radical expressions and working with exponents, including laws of exponents and rational exponents.
By revisiting these topics, students can ensure they are fully prepared for the assessment and are able to tackle a wide range of problems with confidence and clarity.