Cracking the AP Calculus AB and BC Exams Answers

Success in rigorous academic challenges requires more than just basic knowledge; it demands an in-depth understanding of fundamental principles and the ability to apply them under pressure. Whether tackling complex problems or analyzing intricate concepts, being well-prepared for critical evaluations is essential for achieving top results.

By focusing on key strategies, familiarizing oneself with common problem types, and practicing consistently, students can sharpen their skills and boost their confidence. It’s about finding patterns, recognizing core techniques, and developing an approach that can solve a wide range of mathematical questions effectively and efficiently.

Effective preparation involves understanding what to expect, honing problem-solving skills, and mastering specific mathematical techniques that frequently appear in academic challenges. Developing these skills not only leads to better performance but also fosters a deeper appreciation for the subject matter itself.

Cracking the AP Calculus AB and BC Exams Answers

Achieving high scores on advanced assessments requires more than just memorizing formulas. It involves a strategic approach that focuses on understanding underlying principles and effectively applying them in various scenarios. By mastering key concepts, recognizing common problem structures, and practicing with purpose, students can tackle even the most challenging questions with confidence.

Building Strong Foundations in Key Concepts

Strong performance stems from a solid grasp of core topics. Focus on mastering essential areas such as derivatives, integrals, and series. Understanding how these concepts interconnect will allow you to approach questions from multiple angles, leading to more efficient problem-solving. A deep understanding of these areas ensures that you can confidently address a wide variety of problems, from straightforward calculations to more complex analytical challenges.

Effective Test-Taking Strategies

Knowing how to approach each question is just as important as understanding the material. Practice time management techniques to ensure that you allocate appropriate time to each section. Use process-of-elimination methods for multiple-choice questions and always check your work, especially in multi-step problems. Remember, being strategic about pacing and focus is often the key to performing well under pressure.

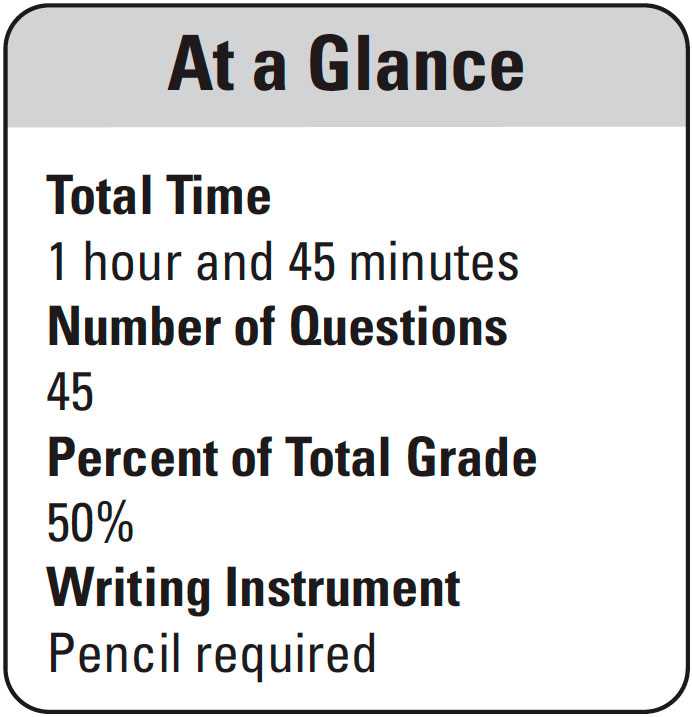
Understanding the AP Calculus Exam Structure

To achieve success in any challenging assessment, it is essential to understand its structure and format. Knowing what to expect can greatly reduce anxiety and improve performance. Familiarity with the layout and types of questions will allow students to devise an effective strategy for managing time and maximizing their potential.

Overview of Assessment Format

The evaluation is divided into multiple sections, each testing different aspects of mathematical proficiency. One part typically includes multiple-choice questions, while another focuses on free-response problems. Understanding how each section is weighted can help prioritize study efforts and allocate time appropriately during the test. A balanced approach to both parts ensures that students are well-prepared for every challenge.

Time Management and Pacing

Effective time management is crucial for navigating a rigorous assessment. Each section comes with specific time limits, making it vital to practice pacing. Students should familiarize themselves with the number of questions in each part and estimate the time required per question. This strategy can lead to better time distribution and reduce the chance of running out of time before completing all questions.

Key Concepts in AP Calculus AB

Mastering fundamental ideas is crucial for success in any advanced mathematical assessment. Understanding core topics such as rates of change, area under curves, and motion can provide a strong foundation for solving a variety of complex problems. These principles are not only essential for academic achievement but also play a significant role in real-world applications.

Focus on building a deep understanding of how derivatives and integrals work, as these are central to many problem types. Derivatives help describe how quantities change, while integrals allow for the calculation of accumulated values. A solid grasp of these concepts enables students to approach problems methodically and with confidence.

Critical Topics for AP Calculus BC

For those tackling more advanced content in this mathematical assessment, focusing on key areas of study is essential. Understanding these critical topics provides the framework needed to approach the most complex questions with clarity and precision. Topics that extend beyond basic principles often require a deeper level of analysis and application, making them vital to success in higher-level problem-solving.

Advanced Concepts to Focus On

Several topics play a significant role in tackling more advanced challenges. Mastering these areas will ensure that you are well-prepared for the specific demands of the assessment:

• Series and Sequences – Understanding convergence, divergence, and how to work with infinite sums is crucial.
• Parametric Equations – Recognizing how to manipulate and analyze curves defined parametrically expands your problem-solving toolkit.
• Polar Coordinates – Mastering how to work with polar graphs and converting between rectangular and polar forms is key.
• Advanced Integration Techniques – Techniques like integration by parts, partial fractions, and improper integrals are essential for solving complex problems.

Strategies for Tackling Complex Problems

In addition to mastering core concepts, developing effective strategies for tackling intricate problems is equally important. Consider these techniques to enhance your performance:

1. Identify the core problem type before beginning the solution process.
2. Break down complex problems into smaller, manageable steps.
3. Use visualization tools, such as graphs, to help understand spatial relationships.
4. Practice working with a variety of problem sets to build familiarity with different question types.

How to Approach Free-Response Questions

When faced with open-ended problems, it’s essential to stay organized and methodical. These questions often require a deeper understanding of concepts and a clear, logical approach to finding solutions. Success lies not just in solving the problem, but in presenting your work in a structured, easy-to-follow manner.

Step-by-Step Problem Solving

Breaking down each problem into smaller, manageable parts can make a complex question seem much more approachable. Here are some tips for tackling free-response tasks:

• Read the question carefully and identify key components that need to be addressed.
• Highlight or underline important information provided in the prompt.
• Plan your approach before diving into calculations – decide on the method or formula that best fits the problem.
• Write out every step clearly, showing how you arrived at each part of your solution.
• Double-check your work to ensure accuracy and clarity.

Time Management for Open-Ended Problems

In open-ended questions, balancing thoroughness with time management is key. Follow these strategies to stay on track:

1. Quickly assess the complexity of the problem and allocate your time accordingly.
2. Don’t get bogged down in one section – move on if you’re stuck and come back later if necessary.
3. Always leave a few minutes at the end to review your solutions and ensure nothing has been overlooked.

Mastering Multiple-Choice Strategies

Approaching multiple-choice questions requires a different set of strategies compared to free-response tasks. These questions test not only knowledge but also the ability to quickly analyze and eliminate incorrect options. Efficiently narrowing down choices can make a significant difference in your score, especially when faced with time constraints.

Effective Elimination Techniques

When confronted with a set of options, the first step is to eliminate obviously incorrect answers. This process allows you to focus on the remaining choices, improving your chances of selecting the correct one. Follow these techniques for more effective elimination:

• Look for extreme or illogical answers that are unlikely to be correct.
• Identify patterns in the answers–often, multiple-choice questions will have one answer that stands out in terms of format or structure.
• Check for units and consistency with the problem’s context to rule out irrelevant answers.

Time-Saving Strategies

In multiple-choice sections, time management is crucial. Here are some strategies to make sure you maximize your performance while staying on schedule:

1. Quickly scan all questions at the start to identify the easier ones and tackle them first.
2. If you’re unsure of an answer, make an educated guess and move on. Don’t spend too long on any one question.
3. Return to harder questions at the end, if time allows, to revisit and double-check your choices.

Utilizing Practice Exams for Success

Taking practice tests is one of the most effective ways to prepare for any challenging assessment. By simulating real test conditions, practice tests help build familiarity with question formats, improve time management, and highlight areas that need further review. Incorporating regular practice into your study routine can significantly boost confidence and performance on test day.

Benefits of Practice Tests

Regularly working through practice questions offers several advantages beyond just reviewing content:

• Improves test-taking stamina and reduces anxiety by mimicking real-time conditions.
• Identifies knowledge gaps, allowing focused review of weak areas.
• Enhances problem-solving speed, which is crucial for managing time effectively.

How to Maximize the Value of Practice Tests

It’s not just about taking as many practice tests as possible. The key lies in how you approach and analyze your performance. Use these strategies to maximize the benefits:

Step	Action
1	Take the practice test under timed conditions to simulate the real exam environment.
2	Review the results in detail, focusing on both correct and incorrect answers.
3	Identify patterns in mistakes and focus on improving those areas in your next study session.
4	Repeat practice tests regularly to track progress and fine-tune your test-taking skills.

Time Management Tips During the Exam

Effective time management is critical to performing well on any rigorous assessment. With a limited amount of time to answer a variety of questions, balancing speed and accuracy is key. The ability to efficiently allocate time across different sections can make the difference between completing the test and rushing through the final questions.

Prioritizing Tasks and Questions

Start by quickly scanning through the entire assessment to get a sense of its layout and difficulty. Prioritize questions based on familiarity and complexity. For example, tackle the easier questions first to gain confidence and ensure quick points. This allows more time to focus on more challenging problems later. Consider these strategies:

• Complete multiple-choice questions before moving to free-response tasks to save time for more complex solutions.
• If a question is taking too long, skip it and return to it later after completing others.
• Use all available time to review your answers, especially in areas where you had doubts.

Allocating Time Wisely

Set specific time limits for each section or type of question to avoid spending too much time on any one part. Break down the total available time into smaller intervals, and stick to those limits. Follow these tips to stay on track:

• Divide your time based on the number of questions and their complexity. For example, allocate more time for free-response sections than for multiple-choice.
• Use a watch or timer to track time as you progress through the test, ensuring you don’t get caught up on any one section.
• If time is running out, focus on answering questions you know well and leave difficult ones for later.

How to Tackle Word Problems

Word problems often present a unique challenge, as they require translating real-world scenarios into mathematical equations. The key to solving them effectively is to break down the information systematically, identify relevant concepts, and apply appropriate methods. By approaching these questions step-by-step, you can avoid feeling overwhelmed and ensure you’re solving the problem logically.

Understanding and Organizing the Problem

Before jumping into calculations, take time to understand the problem and organize the given information. Follow these steps:

• Read the problem carefully and underline or highlight key details such as quantities, rates, and relationships.
• Identify what the question is asking for, whether it’s a rate of change, an area, or some other quantity.
• Sketch a diagram if applicable, as visualizing the problem can often make it clearer.

Setting Up Equations and Solving

Once you have a solid understanding of the problem, proceed to set up the necessary equations. Use the relevant formulas or principles to express the relationship between different elements in the problem. Follow these guidelines:

• Write down the equation or formula you’ll be using, making sure all variables are defined.
• Substitute known values into the equation and solve step by step.
• Double-check your work to ensure the solution makes sense in the context of the problem.

Common Mistakes to Avoid in AP Calculus

While preparing for complex assessments, students often make simple mistakes that can significantly impact their performance. These errors are usually not due to a lack of knowledge but result from missteps in problem-solving or misinterpretation of the question. Being aware of common pitfalls can help you avoid them and improve your overall score.

Misinterpreting Questions

One of the most frequent mistakes is misinterpreting the problem. Rushing through a question without fully understanding its requirements can lead to incorrect solutions. Here are a few ways to avoid this:

• Read the question carefully: Pay attention to specific details, such as units, given values, and what the problem is asking for.
• Identify key terms: Terms like “rate of change,” “maximum,” and “limit” signal which concepts to apply. Misunderstanding these terms can lead to using the wrong methods.

Skipping Step-by-Step Solutions

Another common mistake is skipping steps in calculations, especially when dealing with complex problems. Even if you can solve the problem in your head, it’s important to write down each step for clarity and accuracy. Consider these tips:

• Show all work: This ensures you don’t miss any details and helps in identifying where mistakes occur if the final answer is wrong.
• Check calculations: Small errors in arithmetic or algebra can lead to incorrect results. Double-check your work to catch these issues.

Overlooking Units and Conditions

In problems involving rates, areas, or volumes, overlooking units or boundary conditions can lead to significant errors. Always remember to:

• Pay attention to units: Ensure that units are consistent throughout the problem, and convert them if necessary.
• Check initial and boundary conditions: Many problems are incomplete without the right assumptions or initial values, which can drastically affect the solution.

Effective Study Plans for AP Calculus

Creating a focused and structured study plan is essential for mastering complex mathematical concepts and performing well in assessments. A well-organized schedule helps in balancing review sessions, practice problems, and concept reinforcement, ensuring that all key areas are covered thoroughly. By setting clear goals and managing time effectively, students can make significant progress in their preparation.

Building a Study Schedule

Start by breaking down the material into manageable sections, assigning specific topics to different days or weeks. It’s important to prioritize the areas that need more attention while still reviewing previously covered material to keep it fresh. Here are a few steps to build an effective study schedule:

• Identify key topics: Focus on areas of difficulty, but make sure to include regular reviews of well-known concepts to maintain mastery.
• Set realistic goals: Aim to cover one or two topics per study session, ensuring deep understanding before moving on to new material.
• Be consistent: Regular, shorter study sessions are often more effective than long, irregular study marathons.

Incorporating Practice and Review

While understanding theory is vital, practice is just as crucial. Solving problems helps solidify knowledge and identify areas of weakness. Incorporate both timed practice tests and problem-solving exercises into your plan. Consider the following strategies:

• Use practice problems: Work through problems from previous assessments and additional worksheets to improve problem-solving speed and accuracy.
• Review mistakes: After each practice session, go over any errors and understand why the solution didn’t work. This will help you avoid repeating the same mistakes.
• Simulate test conditions: Take practice tests under timed conditions to improve your ability to manage time during the actual assessment.

The Role of Graphing Calculators

In advanced mathematics, tools like graphing calculators play a crucial role in helping students visualize complex problems and solve them more efficiently. These devices not only assist in graphing functions but also provide support for performing calculations, exploring limits, derivatives, and integrals, which are essential for success in assessments. By understanding the full capabilities of a graphing calculator, students can use it as an invaluable resource during both study sessions and testing scenarios.

Visualizing Mathematical Functions

One of the most powerful features of graphing calculators is their ability to visually represent mathematical functions. This helps students better understand the behavior of equations, such as how they change over intervals or approach specific values. Visualizing these concepts can simplify problem-solving, especially for:

• Identifying critical points: A graphing calculator can quickly show where a function increases, decreases, or changes direction, helping you identify maxima, minima, and inflection points.
• Graphing complex functions: For functions with multiple variables or intricate behavior, calculators allow students to plot graphs with ease, offering insights into relationships between variables.

Efficient Calculations and Error Checking

Beyond graphing, graphing calculators are essential for performing quick and accurate calculations, especially during a time-sensitive test. They can assist in various operations, including:

• Solving equations: Graphing calculators can find solutions to both algebraic and transcendental equations, saving time and reducing the potential for manual errors.
• Checking work: After completing a problem manually, using a graphing calculator to verify results ensures accuracy and provides confidence in your calculations.

Mastering Limits and Continuity

Understanding the behavior of functions near specific points is fundamental in higher mathematics. Mastering these concepts helps students approach problems involving rates of change, area under curves, and more. Grasping the ideas of approaching values and determining the smoothness of functions lays a strong foundation for tackling advanced topics. These concepts are essential in a variety of problem-solving scenarios and play a critical role in many areas of mathematical analysis.

Understanding Limits

Limits are central to understanding how functions behave as inputs approach certain values. The ability to compute limits and interpret their meaning helps clarify a function’s behavior at points of interest. Key steps to mastering limits include:

• Identifying indeterminate forms: Recognizing forms such as 0/0 or infinity/infinity is essential when evaluating limits. In these cases, students should use algebraic manipulation, factoring, or other techniques to resolve the limit.
• Using limit laws: Familiarity with limit properties such as the sum, product, and quotient rules allows students to break down complex limits into simpler parts.
• Applying special limits: Limits involving infinity, piecewise functions, or trigonometric functions require specific strategies for solving, such as L’Hopital’s Rule or direct substitution.

Grasping Continuity

Continuity is a property of functions that ensures they behave smoothly without jumps or breaks. Understanding whether a function is continuous at a given point is essential when solving problems related to optimization or integration. Key points about continuity include:

• Defining continuity: A function is continuous at a point if its value matches the limit as the input approaches that point. Understanding this definition is essential for identifying whether a function is continuous over a given interval.
• Recognizing discontinuities: Discontinuities can occur in functions due to jumps, vertical asymptotes, or removable discontinuities. Identifying these is crucial for solving problems involving limits and continuity.
• Applying continuity tests: A function’s continuity can be tested using various criteria, including evaluating left and right-hand limits and checking for jumps or breaks in the graph.

Integration Techniques You Must Know

Mastering various integration techniques is crucial for solving a wide range of mathematical problems. Understanding how to approach different integrals efficiently enables students to tackle both straightforward and complex questions with ease. Several methods are available, each suited to specific types of integrands, and knowing when to apply them can make a significant difference in performance. Below are the essential strategies for integrating functions that every student should be familiar with.

Basic Integration Methods

The following methods are fundamental and should be practiced regularly:

• Substitution: This technique simplifies complex integrals by making a substitution that turns the integral into a simpler form. It’s useful for functions where a part of the integrand is the derivative of another part.
• Integration by Parts: This method is based on the product rule for differentiation. It’s helpful when the integrand is a product of two functions, where one can be easily integrated and the other differentiated.
• Partial Fractions: Used for rational functions, this technique decomposes the integrand into simpler fractions that are easier to integrate.

Advanced Integration Strategies

For more complicated integrals, these advanced methods are invaluable:

• Trigonometric Substitution: This approach is used for integrals involving square roots of expressions like a? – x? or x? + a?. It involves substituting a trigonometric function to simplify the integral.
• Improper Integrals: These integrals deal with infinite limits or integrands that approach infinity within the interval of integration. Techniques for handling these require careful analysis of convergence.
• Reduction Formulas: Often used to reduce higher powers or complicated trigonometric integrals to simpler forms for easier integration.

Integration Table

The following table provides some common integrals and their corresponding formulas, which are essential for solving typical problems:

Integral	Formula
?x^n dx	(x^(n+1))/(n+1) + C, for n ? -1
?e^x dx	e^x + C
?sin(x) dx	-cos(x) + C
?cos(x) dx	sin(x) + C
?1/x dx	ln|x| + C

By mastering these techniques and knowing when to apply each one, you will be able to solve a wide range of integration problems efficiently and accurately.

Understanding Series and Sequences

Sequences and series are foundational concepts in advanced mathematics, essential for analyzing patterns and behavior over time. A sequence represents a list of numbers arranged in a specific order, while a series involves the summation of terms in a sequence. Understanding how to manipulate these structures is key to solving a variety of problems, from finding limits to evaluating sums and applying convergence tests. This section explores the key ideas behind sequences and series, providing tools for mastering their complexities.

Sequences: Building Blocks of Progressions

A sequence consists of a list of numbers that follow a specific rule. These numbers can be finite or infinite, and they represent progression through time or steps. Sequences are often classified based on their patterns.

• Arithmetic Sequence: A sequence where the difference between consecutive terms remains constant. The general form is a, a+d, a+2d, a+3d, …, where d is the common difference.
• Geometric Sequence: A sequence where each term is obtained by multiplying the previous term by a constant. The general form is a, ar, ar?, ar?, …, where r is the common ratio.
• Fibonacci Sequence: A famous sequence where each term is the sum of the two preceding terms. The sequence starts with 0, 1, 1, 2, 3, 5, …

Series: Summing Up Terms

A series is the sum of terms from a sequence. Like sequences, series can be finite or infinite, with each term being added together according to a specific rule.

• Arithmetic Series: The sum of terms in an arithmetic sequence. The sum of the first n terms is given by S? = (n/2) * (2a + (n – 1) * d), where a is the first term, d is the common difference, and n is the number of terms.
• Geometric Series: The sum of terms in a geometric sequence. For an infinite geometric series, the sum is S = a / (1 – r), where a is the first term and r is the common ratio, provided |r| .
• Power Series: A series of the form ?a?x?, which represents functions in terms of an infinite sum of powers of x.

Convergence and Divergence

One of the key aspects when dealing with series is determining whether they converge or diverge. A convergent series approaches a specific value as the number of terms increases, while a divergent series continues to grow without bound.

• Convergence Tests: Various tests, such as the Ratio Test, Root Test, and Integral Test, can be used to determine if a series converges to a finite value.
• Divergence: If a series does not approach a limit or if the terms do not approach zero, it is said to diverge.

Grasping these concepts is vital for solving problems involving sums and limits. By recognizing the types of sequences and understanding the behavior of series, students can efficiently tackle complex problems and deepen their understanding of advanced mathematical topics.

Strategies for Tackling Derivatives

Mastering the concept of derivatives is crucial for solving problems related to rates of change, slopes of curves, and optimization. To succeed in these types of questions, it’s important to have a clear approach and use systematic strategies. Whether the task involves basic differentiation or more complex applications, applying the correct method and checking each step carefully will lead to better outcomes. Below are some useful techniques and tips for approaching differentiation problems.

Step-by-Step Approach to Differentiation

When solving derivative problems, break down the process into manageable steps to avoid mistakes and ensure clarity.

• Identify the function type: Determine if the function is a polynomial, trigonometric, logarithmic, or a product or quotient of functions. This helps you select the appropriate rule for differentiation.
• Apply the correct differentiation rule: Use rules such as the power rule, product rule, quotient rule, and chain rule based on the structure of the function.
• Simplify after differentiating: Once you’ve differentiated, simplify your expression to ensure it’s in its most usable form.
• Check for special cases: Look out for functions with constants, exponents, or non-differentiable points that could affect the derivative calculation.

Common Rules for Differentiation

There are several basic rules that can be applied to most differentiation problems. Familiarize yourself with these rules to save time and improve accuracy.

Rule	Description	Example
Power Rule	Used for differentiating terms of the form f(x) = ax^n, where a and n are constants.	f'(x) = nax^(n-1)
Product Rule	Used for differentiating products of two functions: f(x) = u(x) * v(x).	f'(x) = u'(x)v(x) + u(x)v'(x)
Quotient Rule	Used for differentiating quotients of two functions: f(x) = u(x) / v(x).	f'(x) = [v(x)u'(x) – u(x)v'(x)] / [v(x)]?
Chain Rule	Used when differentiating composite functions: f(g(x)).	f'(x) = f'(g(x)) * g'(x)

Common Mistakes to Avoid

While differentiating, it’s easy to make small errors that can lead to incorrect solutions. Here are some common mistakes to watch out for:

• Forgetting to apply rules correctly: Ensure you use the appropriate rule for each type of function (e.g., using the chain rule when dealing with composite functions).
• Incorrect simplification: After differentiating, be careful to simplify your expression properly, especially with terms that involve powers or trigonometric functions.
• Missing negative signs: Watch out for terms where the sign might change, such as when differentiating an even power of a function.
• Overlooking special cases: Remember to consider whether there are points where the function may not be differentiable (e.g., at sharp corners or discontinuities).

By mastering these strategies and avoiding common pitfalls, you’ll be well-equipped to tackle derivative-related questions with confidence and precision. With regular practice, these techniques will become second nature, helping you efficiently approach complex problems during assessments.

How to Handle AP Exam Stress

Stress is a natural response to challenging situations, especially when facing important assessments. Managing this stress effectively can help improve performance and maintain mental well-being. By incorporating healthy habits and focusing on the task at hand, you can minimize anxiety and approach your preparation and test-taking with a clearer mind. Below are some practical strategies for handling pressure and staying composed during high-stakes evaluations.

Preparation and Organization

A solid plan and consistent preparation are essential for reducing stress. When you know you’re well-prepared, it boosts confidence and minimizes last-minute panic.

• Start early: Avoid cramming by spreading out your study sessions over weeks or months. This will help retain information and ease the pressure.
• Set realistic goals: Break down study material into manageable chunks. Focus on mastering smaller sections before moving on to the next.
• Prioritize your health: Exercise, healthy meals, and adequate sleep are crucial for optimal brain function. Don’t neglect your well-being in favor of last-minute studying.
• Practice with mock tests: Simulate real test conditions with timed practice sessions to help reduce nervousness when faced with similar situations during the actual assessment.

Mindset and Relaxation Techniques

Developing a calm and positive mindset can be a game-changer when dealing with stress. It’s important to focus on progress rather than perfection and approach the situation with confidence.

• Deep breathing: Practice deep breathing exercises to relax your nervous system. Inhale slowly for a count of four, hold for four, and exhale for a count of four.
• Visualization: Visualize yourself successfully completing the tasks at hand. Imagining positive outcomes can help reduce feelings of fear and self-doubt.
• Take breaks: Study for 30–45 minutes at a time, then take short breaks to refresh your mind. This helps maintain focus and prevents burnout.
• Positive affirmations: Reassure yourself by repeating positive statements like “I am prepared” or “I am capable.” This helps combat negative thoughts and keeps you focused on your abilities.

During the Assessment

When the test day arrives, use strategies to remain calm and focused in the moment. Managing your time and mindset during the test can greatly impact your performance.

• Stay calm: If you feel overwhelmed, pause for a moment, take a deep breath, and refocus. A clear mind will help you tackle each question more effectively.
• Time management: Pace yourself throughout the assessment. Allocate time for each section and leave space at the end to review your answers.
• Focus on one question at a time: Avoid getting stuck on difficult questions. If a problem is too challenging, move on and return to it later with fresh eyes.
• Trust your preparation: Remind yourself that you’ve studied and practiced. Trust in your abilities, and don’t second-guess yourself unnecessarily.

By maintaining a healthy balance between preparation, relaxation, and focus, you can handle stress more effectively and approach assessments with confidence. Stress is a natural part of any challenging situation, but it doesn’t have to control your outcome. With the right strategies, you can thrive under pressure.

Reviewing Past AP Exam Questions

Going through previous assessment questions is one of the most effective ways to strengthen your understanding of key concepts and improve your test-taking abilities. By analyzing past problems, you can familiarize yourself with the format, identify recurring themes, and learn how to approach different types of challenges. This practice not only reinforces your knowledge but also builds confidence in tackling similar questions during the actual evaluation.

Benefits of Reviewing Past Questions

Reviewing past questions offers several advantages that can help sharpen both your knowledge and test-taking skills:

• Familiarity with Question Formats: Understanding the typical structure of questions will help you navigate through them more efficiently during the actual assessment.
• Identify Key Topics: You’ll be able to pinpoint frequently tested areas and allocate more time to mastering these concepts.
• Improve Problem-Solving Skills: By practicing, you develop strategies for solving problems more quickly and accurately.
• Boost Confidence: Repeated exposure to past questions can reduce anxiety and increase self-assurance when facing unfamiliar challenges.

Effective Strategies for Reviewing Past Questions

Simply looking at previous questions is not enough. To make the most of this review process, consider the following strategies:

• Analyze Solutions: Don’t just solve the problems–study the solutions carefully. Understand the reasoning behind each step to grasp the underlying principles.
• Simulate Test Conditions: Time yourself when working through practice questions to replicate the pressure of real test scenarios. This will help you manage your time better and reduce stress on the actual day.
• Work on Mistakes: After attempting past questions, focus on your errors. Identify patterns in the mistakes you make and work on improving those specific areas.
• Mix and Match Topics: Don’t focus only on one topic. Instead, mix questions from different sections to simulate the variety of challenges you’ll encounter.

Resources for Accessing Past Questions

There are several ways to access previous questions to aid your preparation:

• Official College Board Materials: The College Board offers sample questions and previous papers that give an accurate representation of the assessment’s format and difficulty level.
• Study Guides: Many test prep books include a selection of past questions along with detailed explanations to help reinforce your learning.
• Online Resources: Various educational websites offer free or paid access to past assessment questions and additional practice tests.

By reviewing past questions and adopting a structured approach to practice, you can enhance your understanding, improve your performance, and reduce stress during your next evaluation. This preparation method serves as a reliable tool for mastering essential content and becoming more adept at handling different types of questions.