
Success in any advanced mathematics assessment largely depends on how well a student can tackle complex problems under time constraints. Mastering essential concepts is crucial to building confidence and improving performance. A structured approach to practice can significantly enhance the likelihood of achieving a high score.
Reviewing a variety of practice problems, along with their detailed solutions, offers an excellent opportunity for self-assessment. By analyzing explanations for each solution, students can identify common mistakes and refine their understanding. This process fosters critical thinking and the ability to apply learned concepts in different contexts.
In this section, we’ll explore how working through problems with accompanying explanations can streamline your preparation. Developing a deeper understanding of the subject matter is the key to tackling even the most challenging sections of the test with ease. Whether it’s mastering algebraic techniques or understanding geometric principles, the goal is to improve problem-solving abilities and build a solid foundation for success.
Sample AP Precalculus Exam Questions Answer Key
Reviewing practice exercises along with their detailed solutions is an essential part of mastering any advanced mathematics subject. By studying step-by-step explanations, students can strengthen their problem-solving skills and identify areas that need improvement. This method of learning helps not only in understanding the correct approach but also in preventing common errors that may arise during the actual test.
Understanding the Solution Process
Each problem-solving process is designed to highlight key concepts and techniques needed to solve similar problems on the actual assessment. By breaking down each step, learners can gain insight into how to approach different types of challenges effectively. This approach encourages a deeper understanding of the material, ensuring that students are well-prepared for any variations they may encounter.
Maximizing Benefits of Review
Going over solutions not only helps clarify tricky concepts but also builds confidence. By regularly practicing and reviewing, students can develop a mental roadmap for tackling problems swiftly and accurately. This ongoing review process ensures a well-rounded mastery of the subject, giving learners the tools they need to succeed on test day.
Overview of Precalculus Exam Format
Understanding the structure of a challenging mathematics assessment is crucial for effective preparation. The test typically evaluates a broad range of topics that require strong analytical and problem-solving abilities. It consists of multiple sections, each designed to test different aspects of mathematical knowledge, from algebraic manipulations to graphing functions and solving complex equations.
Section Breakdown
The assessment is generally divided into several sections, each focusing on a specific area of mathematics. These sections often include multiple-choice questions and free-response problems, where students must demonstrate their reasoning and explain their steps clearly. The balance between different types of problems ensures that the test evaluates both conceptual understanding and technical skills.
Time Management and Strategy

Efficient time management is essential during the test. Students must allocate enough time to each section while maintaining a steady pace to avoid rushing through problems. Developing strategies to tackle different problem types and focusing on accuracy can make a significant difference in overall performance.
Understanding Key Topics in Precalculus
Mastering the core concepts of advanced mathematics is essential for success in any related assessment. These key topics form the foundation for solving complex problems and require a deep understanding of both theory and application. From algebraic techniques to trigonometric functions, each concept builds upon the last, creating a comprehensive framework for tackling higher-level challenges.
One of the most important areas to focus on is functions. Understanding how they behave, how to graph them, and how to manipulate their equations is critical. Similarly, a solid grasp of geometric principles, including transformations and coordinate systems, is vital for solving problems related to angles and shapes. By breaking down these fundamental topics, students can approach even the most intricate problems with greater confidence and clarity.
How to Approach Sample Exam Questions
When preparing for an advanced mathematics test, it is crucial to approach practice exercises strategically. Each problem offers an opportunity to strengthen your understanding of mathematical concepts and improve your problem-solving techniques. By following a methodical process, you can ensure that you are maximizing your preparation and building the skills needed to succeed under exam conditions.
The first step is to carefully read each problem, taking note of any key information and identifying the underlying concept being tested. Next, consider the most efficient methods for solving the problem–whether it’s applying algebraic manipulations, graphing functions, or utilizing geometric principles. Finally, make sure to review your work to check for errors and refine your approach for similar challenges in the future.
| Step | Description |
|---|---|
| Read Carefully | Identify important details and understand the problem’s requirements. |
| Plan Your Approach | Decide on the most efficient method to solve the problem based on its type. |
| Execute and Check | Solve the problem and verify your solution to avoid mistakes. |
Common Mistakes to Avoid During the Test
During any challenging mathematics assessment, it is easy to make avoidable errors that can cost valuable points. Recognizing these common pitfalls and understanding how to prevent them is essential for achieving a high score. Often, these mistakes are the result of rushing, misinterpreting instructions, or overlooking critical steps in the solution process.
Misreading Problems
One of the most common mistakes students make is misinterpreting the problem’s requirements. It is important to read each statement carefully, paying attention to every detail. Skimming through the instructions can lead to overlooking essential information that changes how a problem should be approached.
Skipping Steps in the Solution Process
Another frequent error is skipping intermediary steps in the process of solving a problem. While it may seem tempting to rush toward the final answer, each step plays a vital role in ensuring the solution is correct. Taking the time to show all work helps avoid miscalculations and allows for easier correction if an error occurs.
Effective Strategies for Time Management
Managing time effectively during a challenging assessment is key to ensuring success. Without proper time allocation, it is easy to either rush through tasks or run out of time before completing the entire test. Developing strong time management skills allows you to stay focused and organized, ensuring that each section receives the attention it deserves.
Setting Time Limits for Each Section
One effective strategy is to set clear time limits for each section of the test. This helps prevent spending too much time on any one problem while allowing enough time to address all areas. Here are a few tips to follow:
- Divide the total time by the number of sections or problems.
- Assign a reasonable amount of time to each question based on its difficulty.
- Use a timer or watch to track your progress and stay on schedule.
Prioritizing Problems Based on Difficulty
Another useful technique is to prioritize problems by their level of difficulty. Start with questions you feel most confident in and leave the more challenging ones for later. This approach helps you build momentum and ensures you tackle easier problems first, making the most of your time. Consider these strategies:
- Quickly scan the test to identify familiar and easy problems.
- Complete the easier questions first to secure quick points.
- Return to the more challenging questions after finishing the simpler ones.
Key Concepts in Functions and Graphs
Understanding the behavior of mathematical relationships is essential for solving complex problems. Functions and their graphical representations play a crucial role in this process. Mastery of these concepts allows students to analyze relationships between variables, interpret data, and solve equations more effectively. By focusing on the properties of functions and their graphs, learners can develop a deeper insight into how different types of functions behave under various conditions.
One important aspect to grasp is how different transformations, such as shifts, reflections, and stretches, affect the graph of a function. Recognizing these changes can help predict the behavior of a function without fully calculating every point. Additionally, understanding domain and range is vital, as it defines the set of possible input and output values, guiding how to approach problem-solving.
Key Topics to Focus On:
- Types of functions: linear, quadratic, exponential, etc.
- Graphical transformations: translations, reflections, and dilations.
- Identifying asymptotes and intercepts for more accurate graphing.
- Domain and range interpretation based on the function’s behavior.
Trigonometry Essentials for AP Precalculus
Trigonometry is a fundamental area of mathematics that plays a vital role in understanding relationships between angles and sides in geometric figures. A solid grasp of trigonometric principles is essential for solving problems involving periodic functions, waves, and circular motion. In advanced mathematics, trigonometry forms the foundation for more complex topics, and mastering its key concepts helps students approach problems with greater confidence.
Key concepts to focus on include the basic trigonometric functions–sine, cosine, and tangent–and their relationships to right triangles. It’s also important to understand the unit circle and how to use it to find values of these functions for different angles. Additionally, students should familiarize themselves with the identities that simplify trigonometric expressions, such as Pythagorean and reciprocal identities, as well as learn how to apply them in various problem-solving scenarios.
Algebraic Skills Needed for Success
Algebra forms the backbone of many mathematical concepts, and a strong understanding of its principles is essential for success in higher-level problem-solving. Mastering algebraic techniques allows students to manipulate expressions, solve equations, and analyze relationships between variables. With these skills, students can confidently approach both simple and complex mathematical tasks with precision and efficiency.
Core algebraic skills include simplifying expressions, solving linear and quadratic equations, and working with inequalities. Additionally, proficiency in factoring, working with exponents, and understanding the rules of polynomials is crucial. Developing a methodical approach to these tasks will help build the foundation for tackling more advanced topics in mathematics.
| Skill | Description |
|---|---|
| Simplifying Expressions | Reducing expressions to their simplest form for easier manipulation. |
| Solving Equations | Finding the value of a variable that satisfies an equation. |
| Factoring | Breaking down polynomials into simpler expressions to solve problems more efficiently. |
| Working with Exponents | Applying the laws of exponents to simplify expressions and solve problems. |
Calculus Concepts in Precalculus Questions
Although calculus is often considered a more advanced field of study, its foundational concepts are deeply intertwined with earlier mathematical topics. Many of the ideas and techniques used in calculus, such as limits, derivatives, and rates of change, can be introduced and explored within the context of earlier coursework. Understanding these fundamental concepts at an early stage prepares students for more advanced mathematical analysis and enhances their ability to solve complex problems.
Limits and Continuity
One of the most important concepts from calculus that often appears in introductory coursework is the idea of limits. Limits describe how a function behaves as it approaches a particular point, which is critical for understanding the behavior of more complex functions. Even without delving deeply into derivatives or integrals, the basic understanding of limits helps students analyze function behavior, especially in real-world applications.
Rates of Change and Slopes
Another key calculus concept found in earlier mathematical study is the concept of rates of change. This is often explored through problems involving slopes of lines, which represent the rate at which one quantity changes relative to another. The idea of instantaneous rates of change can also be introduced using simple functions, laying the groundwork for the more complex calculations encountered in calculus.
Practice Questions to Strengthen Knowledge
Engaging with practical exercises is essential for reinforcing concepts and improving problem-solving skills. By regularly practicing a variety of problems, students can deepen their understanding, identify areas of weakness, and gain the confidence needed to tackle more complex challenges. Applying learned techniques in different scenarios allows for better retention and mastery of key topics.
Types of Problems to Practice
Focusing on a wide range of problem types ensures that you are well-prepared for any scenario. Here are a few categories of exercises that will help solidify your mathematical foundation:
- Function Analysis: Practice identifying key features of different functions, including domain, range, and behavior at extreme values.
- Graph Interpretation: Solve problems that require you to analyze and interpret the graphical representation of functions.
- Algebraic Manipulations: Work through problems involving simplifications, factoring, and solving equations to strengthen algebraic skills.
- Rate of Change: Solve problems that involve calculating the rate at which one quantity changes in relation to another.
Benefits of Regular Practice
By committing to regular practice, students not only improve their problem-solving speed but also build critical thinking skills that are essential for success. The more diverse the problems, the more prepared you’ll be to apply your knowledge under varying circumstances. Consistency is key–small, focused practice sessions can lead to significant improvements over time.
Importance of Answer Key for Self-Assessment
Utilizing a solution guide is a crucial step in the learning process, as it provides an opportunity for self-reflection and assessment. By comparing one’s own solutions with the correct ones, students can identify strengths and weaknesses in their understanding. This process not only helps in correcting mistakes but also promotes independent learning, allowing individuals to track their progress and adjust their study strategies accordingly.
Benefits of Self-Assessment
Self-assessment offers numerous advantages that contribute to academic success:
- Identifying Mistakes: Reviewing solutions helps pinpoint errors in calculations, reasoning, or application of concepts, making it easier to correct them.
- Boosting Confidence: Successfully solving problems independently builds confidence, while recognizing areas for improvement provides clarity on where to focus further effort.
- Enhancing Retention: Actively engaging with the material through self-assessment encourages better retention, as it reinforces key concepts and methods.
- Developing Problem-Solving Skills: Regular review of one’s work fosters critical thinking and problem-solving skills, which are essential for more advanced mathematical challenges.
How to Use Solution Guides Effectively
To maximize the benefits of a solution guide, students should approach the material systematically. After attempting a problem, compare the steps taken with the provided solution. Take note of any discrepancies and revisit those areas to deepen understanding. It’s also helpful to attempt the problem again after reviewing the solution to ensure full comprehension.
Breaking Down Answer Key Explanations
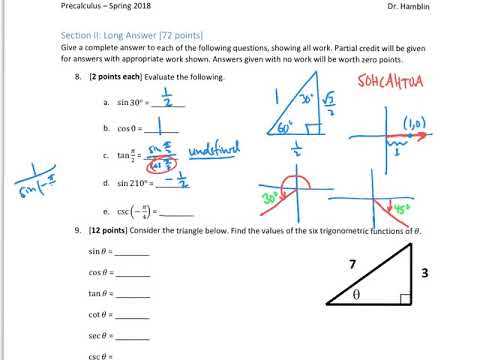
Understanding the step-by-step breakdown of a solution is essential for mastering problem-solving techniques. The clarity provided by detailed explanations helps learners grasp not only the correct final answer but also the methods and reasoning that lead to it. By examining each step closely, students can learn how to approach similar challenges in the future, ensuring they build a deeper understanding of the underlying principles.
Key Elements in Solution Explanations
When reviewing a detailed solution, it’s important to focus on several critical components that contribute to the clarity and effectiveness of the explanation:
- Step-by-Step Process: Clear, sequential steps allow the reader to follow along easily and understand the logical flow from one action to the next.
- Highlighting Key Concepts: Important mathematical principles or methods used in each step should be emphasized to demonstrate how they are applied in context.
- Visual Aids: Graphs, diagrams, or tables can offer additional insight, making abstract concepts more tangible and accessible.
- Justifications: Each step should be accompanied by a rationale, explaining why a particular method or formula was chosen, which fosters a deeper understanding of the reasoning behind the solution.
Benefits of Analyzing Solution Explanations
Taking the time to break down and analyze solution explanations provides several key benefits for learners:
- Developing Problem-Solving Skills: By understanding how different problems are approached, students learn how to adapt strategies and techniques to new challenges.
- Reinforcing Concepts: Revisiting the reasoning behind each step reinforces core concepts, making them easier to recall and apply in future situations.
- Identifying Mistakes: Reviewing solutions helps identify where things went wrong in one’s own work, offering an opportunity to correct misunderstandings and prevent future errors.
Using Answer Keys for Effective Review

Utilizing solution guides is a powerful strategy for improving comprehension and reinforcing learned concepts. When reviewing complex material, simply knowing whether an answer is correct is not enough. Understanding the reasoning behind each solution is crucial for long-term retention and mastering the subject. By dissecting the provided solutions, learners can identify areas for improvement and solidify their grasp of key topics.
To make the most of a solution guide, follow a structured approach to review that focuses on both the correct answers and the methods used to reach them. This ensures that learning is more than just memorization and encourages a deeper understanding of the material.
Steps for Effective Review Using Solution Guides
Here are some tips on how to use solution guides for an efficient and comprehensive review:
- Understand Each Step: Instead of just checking if the final result is correct, review every step of the solution. Pay attention to how each decision is made and what rules or principles are being applied.
- Compare Your Approach: If your solution differs from the guide’s, analyze where your methods diverged. Understanding the mistakes or differences will help you improve your own approach next time.
- Focus on Concepts: Identify the core concepts or techniques being used in each problem. Are there specific strategies you should revisit or strengthen? Focus on these concepts during your review.
- Practice Similar Problems: After reviewing the solution, try solving similar problems on your own. This will help reinforce the method and boost your confidence in applying it to new challenges.
Maximizing Retention through Active Review
Merely reading through solutions may not be enough to ensure mastery. To maximize retention, engage in active review techniques:
- Teach the Solution: Explaining the solution to someone else, or even to yourself, is a powerful method for solidifying your understanding and pinpointing gaps in your knowledge.
- Summarize Key Takeaways: After reviewing the solution, write down the key points or strategies that you found most helpful. This will reinforce your learning and provide a reference for future practice.
- Set Up Timed Practice: Challenge yourself by solving similar problems within a time limit, simulating exam conditions. This practice can help you become more efficient and confident under pressure.
Building Confidence Before the AP Exam
Confidence plays a crucial role in how effectively you perform during an assessment. The period leading up to the test is the perfect opportunity to reinforce your skills and foster a positive mindset. Building confidence is not just about knowing the material, but also about developing a sense of self-assurance that helps you approach the test with a calm, focused attitude.
There are several strategies you can use to enhance your preparedness and confidence before the big day. Consistent practice, mastering key concepts, and addressing any weaknesses will provide a solid foundation. Equally important is maintaining a healthy mindset–understanding that every challenge faced is an opportunity to grow and improve.
Master Key Topics Through Regular Practice
One of the best ways to gain confidence is through consistent practice. By regularly solving problems and reviewing concepts, you ensure that your knowledge is fresh and your skills are sharp. Focus on areas where you feel less secure, as these are often the ones that can make the difference between success and hesitation.
- Daily Practice: Dedicate a set amount of time each day to solving practice problems. This will help solidify your understanding of essential concepts and enhance your problem-solving abilities.
- Focus on Weak Areas: Identify your weakest areas and spend extra time reinforcing them. This will help you feel more confident in tackling questions related to these topics.
- Simulate Real Conditions: Practice under timed conditions to simulate the actual test environment. This will help you get comfortable with the time constraints and reduce any test-day anxiety.
Adopt a Positive Mindset
In addition to practicing, developing a positive mindset is key to building confidence. Negative thoughts can easily undermine your preparation, but with the right mindset, you can overcome challenges more effectively. A calm and focused approach will help you stay clear-headed when it matters most.
- Visualize Success: Take a few minutes each day to imagine yourself calmly navigating the test and answering questions confidently. Visualization can help reduce anxiety and improve your overall performance.
- Focus on Progress: Rather than stressing over what you don’t know, focus on what you’ve accomplished so far. Celebrate small victories along the way to stay motivated and confident.
- Stay Relaxed: Incorporate relaxation techniques such as deep breathing or meditation into your study routine. Reducing stress levels will help you feel more at ease and improve your focus during the test.
Additional Resources for Exam Preparation
In addition to practice materials and self-study, there are a wide variety of supplementary tools that can help enhance your understanding and improve your readiness for the assessment. These resources are designed to reinforce key concepts, provide additional practice opportunities, and offer expert insights that can make a significant difference in your preparation.
By utilizing different forms of learning, such as interactive platforms, instructional videos, and collaborative study groups, you can expand your knowledge and sharpen your problem-solving skills. Whether you’re looking for online tutorials or supplementary books, there are plenty of options available to support your study journey.
Online Learning Platforms and Tutorials
Digital platforms offer a flexible way to access high-quality lessons and practice exercises. Many websites provide free or paid resources that cater to a wide range of learning styles. These platforms often include detailed explanations, interactive quizzes, and video tutorials that break down complex topics into manageable sections.
- Khan Academy: Offers free courses with video lessons and practice exercises on a variety of mathematical topics. Ideal for reviewing fundamental concepts and practicing problems at your own pace.
- Coursera: Provides access to online courses from top universities. These courses often include video lectures, assignments, and forums for discussing challenging topics with peers.
- EdX: Offers online courses and certifications, including mathematics courses from reputable universities, giving learners the chance to deepen their understanding of core concepts.
Study Groups and Peer Collaboration
Sometimes, working with others can enhance your learning process and provide new perspectives on difficult concepts. Study groups offer an opportunity to collaborate with peers, ask questions, and engage in discussions that can help solidify your understanding.
- Study Sessions: Organize group study sessions with classmates or friends who are also preparing for the same test. Discussing topics and solving problems together can help clarify confusing areas.
- Online Forums: Participate in online study groups and forums where you can ask questions, share tips, and learn from others. Platforms like Reddit and Stack Exchange often have communities dedicated to helping students prepare for tests.
- Tutoring Services: If you need personalized support, consider hiring a tutor. Many tutoring services offer specialized help in mathematics and other subjects, tailoring lessons to your specific needs.