
For those preparing for rigorous evaluations in the field of modern science, mastering core principles is essential. Understanding complex phenomena requires not only theoretical knowledge but also the ability to apply this understanding in practical scenarios. This section provides valuable insights into tackling the most challenging topics, helping students strengthen their grasp on key ideas.
Focused practice on problem-solving and critical thinking is crucial for success. By engaging with well-structured examples, learners can enhance their skills and deepen their comprehension. This approach enables them to identify common patterns and better navigate the complexities encountered in assessments.
In this guide, we explore a range of essential concepts, providing clarity and practical strategies for addressing the most frequently encountered topics. Whether preparing for a test or refining one’s expertise, these resources offer an effective pathway to mastery.
Advanced Scientific Concepts: Key Problems and Solutions
In the study of complex scientific principles, it is essential to not only understand the underlying theories but also to be able to tackle practical problems that reflect real-world applications. This section focuses on common challenges encountered by learners in mastering the key topics, offering structured problems designed to improve both comprehension and problem-solving skills. Here, we break down essential topics into easily digestible problems with their corresponding solutions.
Through careful analysis of each issue, students can gain a deeper understanding of how to approach difficult tasks, sharpen their critical thinking, and strengthen their knowledge base. The examples provided will guide you step by step, ensuring clarity and offering effective solutions that help reinforce the concepts discussed.
| Problem Type | Example | Solution Approach |
|---|---|---|
| Wave Function Analysis | Determine the behavior of a particle in a confined space. | Use Schrödinger’s equation to model the system and find the particle’s wave function. |
| Energy Calculations | Calculate the energy levels of an electron in an atom. | Apply the Bohr model to determine quantized energy states and use the relevant formulas. |
| Measurement Problem | Find the probability distribution of a particle’s position. | Use the square of the wave function and integrate over the relevant spatial domain. |
| Entanglement Scenarios | Analyze a system of two entangled particles. | Apply principles of non-locality and examine how measurement on one affects the other. |
By practicing these types of problems, learners can develop the necessary skills to excel in assessments and better understand the intricacies of advanced scientific theories. Each solution provides a clear method for breaking down complex issues into manageable steps, ensuring a comprehensive understanding of the subject matter.
Key Concepts in Quantum Mechanics
Understanding the foundational principles of the field requires a deep dive into several core ideas that govern the behavior of subatomic particles. These concepts form the building blocks of a complex theory, offering insights into the strange and counterintuitive nature of the microscopic world. Grasping these key ideas is essential for anyone seeking to explore more advanced topics and applications.
Wave-Particle Duality
One of the most fundamental concepts is the dual nature of particles. At the microscopic scale, entities like light and electrons exhibit characteristics of both waves and particles. This duality challenges our classical understanding, where waves and particles were considered separate categories. By examining phenomena such as the double-slit experiment, we gain a better understanding of how particles behave differently depending on observation and the experimental setup.
Superposition Principle

The principle of superposition states that a system can exist in multiple states simultaneously until it is measured. This concept is central to understanding the behavior of particles in systems like atoms and molecules. The superposition principle leads to intriguing phenomena, such as interference patterns, and is crucial for explaining various effects in advanced scientific fields like computing and cryptography.
Understanding Wave-Particle Duality
At the heart of many intriguing phenomena in modern science lies the concept that subatomic particles can exhibit both wave-like and particle-like behaviors. This duality challenges our classical understanding of the universe, where entities were traditionally classified as either waves or particles. Instead, at the microscopic level, particles like light and electrons appear to blur the line between these two categories, displaying characteristics of both, depending on the circumstances and the way they are observed.
The most famous experiment illustrating this behavior is the double-slit experiment, where particles such as electrons create an interference pattern typical of waves when not directly observed. However, when an attempt is made to measure which slit the particles pass through, they behave as individual particles, not waves. This paradox forces us to rethink the very nature of reality at the smallest scales and raises questions about the role of observation in shaping outcomes.
Understanding this concept is essential for grasping more advanced ideas in the field, as it forms the foundation for much of the theory behind atomic models, light behavior, and even modern technologies like electron microscopes and particle accelerators.
Famous Quantum Theories Explained
Several groundbreaking theories have reshaped our understanding of the microscopic world. These theories, developed by brilliant minds over the past century, form the foundation for much of the modern scientific framework. Each theory addresses different aspects of reality at the smallest scales, providing insights that challenge classical views and unlock new ways of looking at nature. In this section, we will explore some of the most famous theories and their key concepts.
Heisenberg’s Uncertainty Principle
One of the most influential ideas in modern science, Heisenberg’s principle asserts that it is impossible to simultaneously know both the position and momentum of a particle with perfect accuracy. This limitation is not due to measurement errors, but rather an intrinsic property of nature itself. The principle challenges the deterministic worldview of classical mechanics and introduces an element of unpredictability at the atomic level.
- Position and momentum are inversely related in terms of precision.
- The more accurately we measure one, the less accurately we can measure the other.
- This principle has profound implications for the nature of reality and the limits of observation.
Schrödinger’s Wave Equation
Schrödinger’s equation is a fundamental result in the study of wave behavior in microscopic systems. It describes how the wave function, which represents the probabilities of finding a particle in a particular state, evolves over time. The equation provides a mathematical framework for understanding phenomena like atomic energy levels and the behavior of particles in various potential fields.
- The wave function provides a complete description of a system’s state.
- The equation helps predict how systems change over time.
- It is essential for understanding electron configurations in atoms and molecules.
Superposition Principle and Measurement
One of the most fascinating aspects of the microscopic world is the ability of particles to exist in multiple states simultaneously. This phenomenon, often referred to as superposition, defies our classical understanding of how objects should behave. It suggests that, until observed or measured, a system can be in a combination of all possible states, with the final outcome only determined when measurement occurs. This concept has profound implications for how we understand the nature of reality at the smallest scales.
Understanding Superposition
The principle of superposition is central to describing the behavior of particles in systems such as atoms. A particle, like an electron, can exist in several possible positions or energy levels at the same time. It is only when an observation or measurement is made that the particle “collapses” into a single, definite state. This unpredictability highlights the fundamental difference between the macroscopic world and the behavior of particles in the subatomic realm.
The Role of Measurement
The act of measurement plays a critical role in the superposition principle. Unlike classical systems, where observation does not alter the state, measuring a quantum system affects its state directly. The famous thought experiment known as Schrödinger’s cat illustrates this point: a cat inside a box can be simultaneously alive and dead until the box is opened and the state is measured. In reality, this means that the very act of measuring determines the outcome, forcing a particle to choose a specific state from the possibilities.
Key Implications:
- Measurement affects the system: The state of a system changes upon observation.
- Probability over certainty: Before measurement, outcomes are based on probabilities.
- Wave function collapse: The system transitions from a superposition to a single state upon measurement.
Solving Problems in Quantum States
In the study of microscopic systems, solving problems related to the behavior of particles requires a solid understanding of the fundamental principles that govern their states. Particles in these systems do not follow the simple rules of classical mechanics, and their behavior is often governed by complex mathematical models. To solve problems in this realm, it is essential to apply the right tools and techniques to correctly interpret the outcomes and predictions of these systems.
Using Wave Functions to Describe Systems
One of the most powerful tools for understanding particle behavior is the wave function. It describes the probabilities of finding a particle in different states and positions. Solving problems in this context typically involves determining the wave function that describes the system and then using it to calculate observable quantities, such as energy levels or momentum. The wave function evolves over time according to the Schrödinger equation, providing a dynamic picture of the system’s behavior.
Calculating Energy Levels and Observables
Once the wave function is known, it is possible to extract important physical quantities. For example, energy levels can be calculated using the time-independent Schrödinger equation for systems like atoms and molecules. The solution to this equation provides discrete energy values, which are essential for understanding atomic structure. Additionally, measuring other observables, such as position or momentum, requires using operators that act on the wave function to extract the corresponding values.
Key Strategies for Solving Problems:
- Identify the system: Understand the specific system being studied (e.g., particle in a box, harmonic oscillator).
- Apply the Schrödinger equation: Use this equation to model the behavior of the system over time.
- Interpret the results: Use the wave function to calculate measurable quantities and understand the system’s behavior.
Quantum Entanglement and Its Implications
One of the most intriguing phenomena in the microscopic world is the concept of particle interconnection, where the state of one particle becomes directly linked to another, regardless of the distance between them. This phenomenon, often referred to as entanglement, challenges our classical notions of separateness and locality. When two particles become entangled, their properties are no longer independent; instead, measuring one will instantly determine the state of the other, even if they are far apart. This extraordinary feature has profound implications for our understanding of reality and opens new possibilities for technological advancements.
Entanglement has been experimentally verified in various settings, showing that particles that interact and become entangled remain inextricably linked, regardless of the space separating them. This connection happens instantaneously, far surpassing the speed of light, which seems to violate traditional laws of causality and communication. As such, entanglement raises fundamental questions about the nature of information, communication, and the very structure of the universe.
Implications for Communication and Computing
The most notable applications of entanglement lie in fields like secure communication and computing. In particular, entanglement-based systems, such as quantum cryptography, exploit the peculiar nature of particle states to create unbreakable encryption methods. Since any attempt to measure or observe the entangled particles would disturb their state, an eavesdropper is immediately detected, ensuring the security of the transmitted information.
Challenges to Classical Views of Reality

The strange behavior of entangled particles challenges the very foundations of classical physics, particularly concepts like locality and determinism. It suggests that particles can be correlated in ways that defy our intuitive understanding of cause and effect. This non-locality, where particles affect each other instantly across vast distances, has prompted a re-evaluation of established scientific principles and sparked debates over the fundamental nature of reality itself.
Key Concepts:
| Concept | Description |
|---|---|
| Non-locality | Particles can instantaneously influence one another, regardless of distance. |
| Quantum Teleportation | The process by which quantum information is transferred from one location to another using entanglement. |
| Quantum Cryptography | Secure communication systems that leverage entanglement to detect eavesdropping and ensure privacy. |
The Role of Heisenberg’s Uncertainty Principle
In the microscopic realm, the limitations of measurement play a crucial role in shaping our understanding of particle behavior. Heisenberg’s principle reveals that there are inherent restrictions to how precisely certain pairs of properties, such as position and momentum, can be known simultaneously. This fundamental aspect of nature underscores a departure from classical mechanics, where such measurements could, in theory, be made with absolute precision. Instead, the uncertainty principle introduces a level of unpredictability that challenges our intuitive notions of reality.
According to this principle, the more accurately one property is measured, the less precisely the other can be known. This relationship between position and momentum is not a result of technological limitations, but a reflection of the intrinsic characteristics of particles at the smallest scales. As a result, it is impossible to obtain a complete, deterministic description of a system, which has profound implications for how we understand the behavior of matter in the microscopic world.
Implications for Measurement and Observation
The uncertainty principle highlights the delicate nature of measurement in microscopic systems. In classical mechanics, one could observe the position or speed of an object without disturbing its state. However, at the atomic and subatomic level, the act of measuring one property influences the other. This fundamental limitation forces a rethinking of the observer’s role in scientific inquiry, as observation itself becomes a factor that alters the system being studied.
Impact on Scientific Theories
The principle challenges long-held assumptions about the predictability and determinism of physical systems. In classical theories, it was assumed that if one knew the initial conditions of a system, its future behavior could be predicted with certainty. However, the uncertainty principle introduces a level of randomness and unpredictability, meaning that even with perfect knowledge of a system’s state, only probabilities, not certainties, can be determined.
Key Takeaways:
- Inherent uncertainty: The uncertainty is not due to measurement errors but a fundamental feature of nature.
- Indeterminacy of particles: Certain pairs of properties, such as position and momentum, cannot be precisely determined at the same time.
- Implications for predictability: The principle limits the predictability of particle behavior, emphasizing probabilities over certainties.
Schrödinger Equation in Quantum Physics
The Schrödinger equation is a foundational mathematical tool used to describe the evolution of systems at the microscopic scale. It provides a way to understand how the state of a system changes over time, based on its energy and interactions with the environment. Rather than focusing on definite outcomes, the equation predicts the probabilities of various outcomes, capturing the inherent uncertainty of particles at small scales. The equation’s solutions are key to determining how particles behave under different conditions, forming the backbone of modern theory in microscopic systems.
At its core, the Schrödinger equation outlines how a system’s wave function evolves, offering predictions about observable quantities such as position, momentum, and energy. These predictions are probabilistic, meaning that instead of giving a precise outcome, they describe a range of possible states and the likelihood of each. This concept fundamentally shifts how we view the nature of reality in microscopic systems.
Time-Dependent vs Time-Independent Schrödinger Equation
The Schrödinger equation comes in two main forms: time-dependent and time-independent. Each version is used to describe different aspects of systems, depending on the situation at hand.
- Time-Dependent Schrödinger Equation: Used to describe the behavior of a system over time. It accounts for how the wave function evolves in response to external forces or changes.
- Time-Independent Schrödinger Equation: Often used for systems where the external conditions do not change with time. This form helps calculate the energy levels of a system, such as an electron in an atom.
Applications of Schrödinger’s Equation

One of the most important applications of the Schrödinger equation is in determining the energy levels of atoms and molecules. By solving the equation for different systems, scientists can predict the allowed energy states of particles, such as electrons orbiting an atom’s nucleus. These energy levels explain many physical phenomena, from the structure of atoms to the behavior of electrons in semiconductors.
- Atomic structure: The equation helps explain how electrons are arranged in atoms and how they transition between different energy levels.
- Chemical bonding: By solving the equation for molecules, it is possible to understand the forces that hold atoms together in chemical bonds.
- Material properties: In condensed matter physics, the equation is used to describe the behavior of particles in solids and liquids, aiding in the design of new materials.
Quantum Tunneling and Real-World Applications

One of the most fascinating phenomena in the microscopic world is the ability of particles to pass through barriers that would typically be insurmountable in classical mechanics. This effect, known as tunneling, occurs when a particle has enough probability to cross an energy barrier, even if it does not have enough energy to overcome it in a classical sense. While this idea seems counterintuitive, it has been observed and applied in many areas of technology and science. The understanding of this process has led to a variety of practical applications that have transformed industries and technology.
Despite its seemingly strange nature, tunneling is essential in explaining certain behaviors of particles, especially when they interact with barriers, such as energy walls or potential fields. The phenomenon plays a central role in numerous real-world applications, from electronics to nuclear reactions, and is a cornerstone of many modern technological advancements.
Applications in Electronics
Tunneling is widely used in modern electronics, particularly in devices that rely on very small scales. Some of the most important applications include:
- Tunneling Diodes: These semiconductor devices use the principle of tunneling to allow current to flow in a manner that differs from typical diodes, leading to faster switching times and improved performance in specific applications.
- Flash Memory: In flash storage devices, tunneling is used to move electrons across an insulating barrier, which is essential for storing data. This enables the rapid writing and erasing of data on memory chips.
- Transistor Technology: As transistors continue to shrink, tunneling plays a crucial role in the behavior of modern microelectronics, influencing the development of faster and more efficient processors.
Applications in Nuclear and Astrophysical Processes
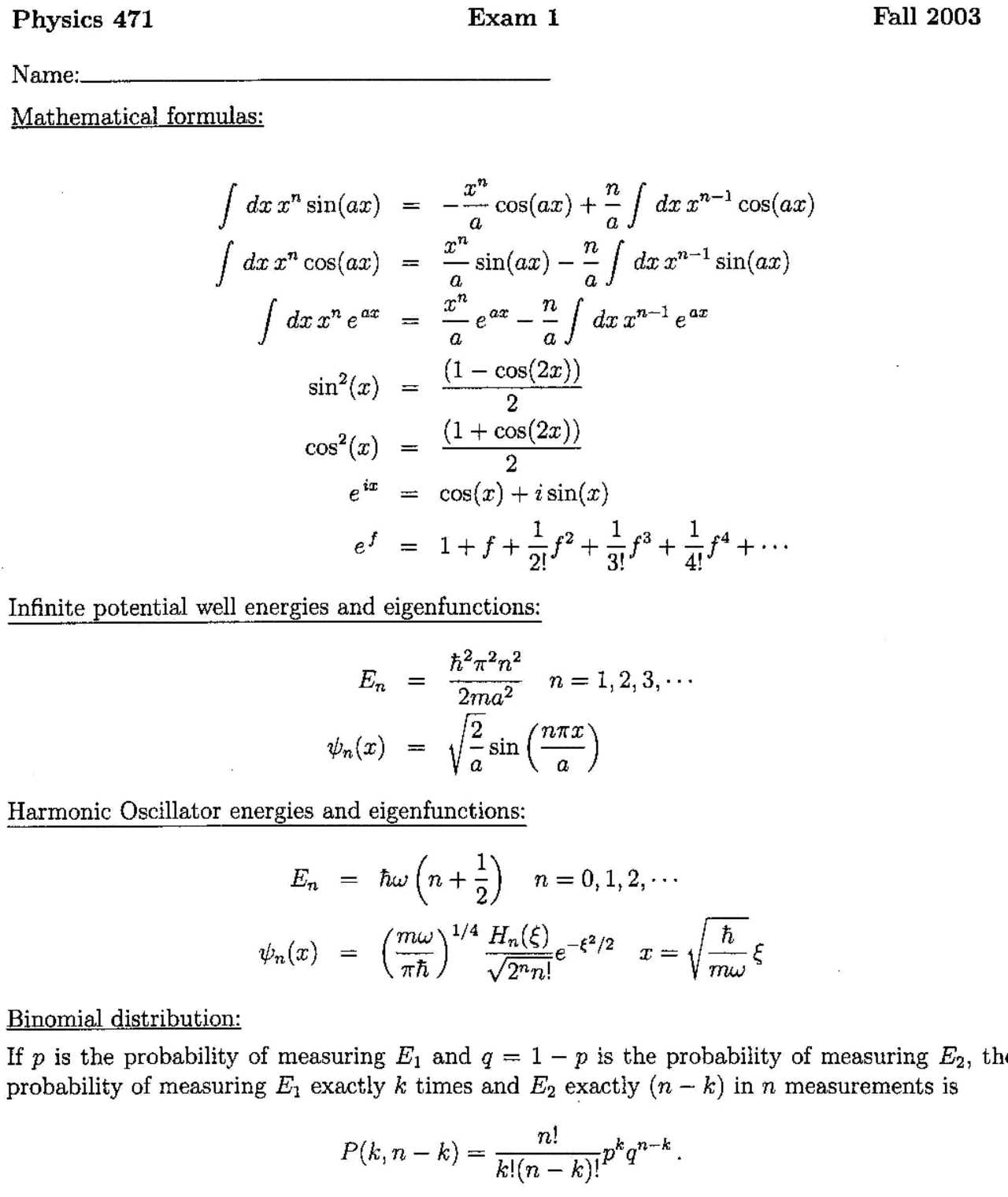
Beyond electronics, tunneling is also significant in understanding nuclear processes, including:
- Fusion Reactions: In stars, including our Sun, nuclear fusion occurs due to tunneling. Protons, which would not typically have enough energy to overcome electrostatic repulsion, can tunnel through the barrier, leading to fusion and the release of vast amounts of energy.
- Radioactive Decay: The decay of certain unstable nuclei is governed by tunneling. The particles involved, such as alpha particles, tunnel through potential barriers, allowing them to escape the nucleus and result in radiation.
Important Quantum Physics Formulas
The study of subatomic particles and their interactions is governed by several key mathematical expressions that allow scientists to predict and understand complex behaviors. These equations are essential for analyzing the properties of particles, their energy states, and how they behave under various conditions. By solving these formulas, researchers can gain insights into phenomena that are otherwise invisible at the macroscopic scale. Below are some of the most important formulas that form the foundation of this field.
Schrödinger’s Equation
This equation is one of the most fundamental in the study of microscopic systems. It describes how the wave function of a system evolves over time, allowing for predictions about a system’s behavior.
The time-dependent Schrödinger equation is given by:
iħ ∂ψ/∂t = Hψ
Where i is the imaginary unit, ħ is the reduced Planck constant, ψ is the wave function, and H is the Hamiltonian operator representing the total energy of the system.
Heisenberg’s Uncertainty Principle
Heisenberg’s principle is central to the understanding of measurement limitations in subatomic systems. It states that the more precisely the position of a particle is known, the less precisely its momentum can be known, and vice versa.
The formula for this principle is:
Δx Δp ≥ ħ/2
Where Δx is the uncertainty in position, Δp is the uncertainty in momentum, and ħ is the reduced Planck constant.
Planck’s Relation
Planck’s relation relates the energy of a photon to its frequency. This relationship is crucial in understanding electromagnetic radiation at the quantum level.
The formula is:
E = hν
Where E is the energy of the photon, h is Planck’s constant, and ν is the frequency of the electromagnetic wave.
de Broglie Wavelength

The de Broglie hypothesis suggests that all particles exhibit wave-like properties, with the wavelength being inversely proportional to the particle’s momentum.
The de Broglie wavelength is given by:
λ = h/p
Where λ is the wavelength, h is Planck’s constant, and p is the momentum of the particle.
Applications of Quantum Mechanics in Technology

The principles governing the behavior of particles at the microscopic level have led to groundbreaking innovations in modern technology. By understanding how these particles interact, behave, and transmit energy, scientists and engineers have been able to develop a wide range of devices and systems that have revolutionized various industries. From communications to computing, the impact of these discoveries can be seen in everyday technologies that push the boundaries of performance and efficiency.
Several technological advancements are based on the manipulation of subatomic particles and their wave-like properties, which allow for enhanced control, miniaturization, and speed. These applications span a broad spectrum, from medical imaging to secure communication networks, illustrating how these concepts have shaped modern technological landscapes.
Advances in Computing
One of the most promising areas where these concepts are making a significant impact is in the realm of computing. Devices that operate at the atomic scale allow for faster, more efficient processing power. Notable applications include:
- Quantum Computers: These machines leverage the principles of superposition and entanglement to perform complex calculations far faster than classical computers. They hold the potential to solve problems in fields such as cryptography, material science, and optimization.
- Transistor Development: The shrinking of transistors has been made possible by the understanding of subatomic interactions, enabling the continued miniaturization of microprocessors in electronic devices.
- Advanced Algorithms: Quantum principles inspire algorithms that can perform tasks like sorting and searching exponentially faster, opening new possibilities in data analysis and artificial intelligence.
Applications in Communication and Security
In addition to computing, these principles have been applied to enhance security and communication systems. By harnessing the unique properties of subatomic particles, new technologies are being developed to ensure the integrity and confidentiality of data transmission:
- Quantum Cryptography: Using entangled particles, quantum cryptography enables virtually unbreakable encryption methods, ensuring that sensitive data remains secure during transmission.
- Quantum Key Distribution (QKD): QKD allows for the exchange of encryption keys with absolute security, making eavesdropping on communication nearly impossible.
- Quantum Networks: These emerging technologies promise to revolutionize communication systems by creating ultra-secure, fast networks that leverage the quantum properties of particles.
Challenges in Quantum Computing Exams

The complexity of evaluating knowledge in the field of advanced computational systems lies in the fundamental differences between classical and cutting-edge approaches. These challenges arise from the abstract nature of certain concepts, the intricate calculations required, and the sophisticated tools necessary to fully grasp the theoretical and practical applications of these systems. As this field is still rapidly developing, understanding and assessing proficiency can be particularly difficult.
Students and professionals alike face difficulties in mastering the deep and often non-intuitive principles that govern these technologies. This section explores the primary hurdles encountered during assessments related to this area, focusing on both theoretical understanding and problem-solving skills.
Conceptual Understanding and Mathematical Complexity
One of the biggest obstacles is the sheer complexity of the mathematical models and theoretical concepts involved. The advanced mathematical techniques required to model these systems can be challenging to grasp. Some of the key issues include:
- Abstract Mathematical Foundations: The need for understanding linear algebra, complex numbers, and tensor calculus, which are essential to solving problems in this domain, can be daunting for students without a strong mathematical background.
- Non-Intuitive Concepts: Concepts like superposition and entanglement can be difficult to visualize and apply, making it hard for students to fully comprehend their implications in computational tasks.
- Advanced Algorithms: The algorithms that underlie modern computational systems often require a deep understanding of both theory and application, making it difficult for students to apply them correctly in problem-solving scenarios.
Technological Limitations and Practical Challenges
Another layer of difficulty arises from the practical aspects of working with advanced computing systems. The technology is still evolving, and many of the tools required to simulate or experiment with these systems are not widely accessible. The main challenges here include:
- Limited Access to Real Systems: Due to the experimental nature of this field, many assessments rely on simulations or approximations, making it hard for students to work with actual devices.
- Resource-Intensive Computations: The computational power needed to test and validate theories or algorithms can be significant, and the lack of appropriate resources can impede students’ ability to engage with the subject effectively.
- Fast-Paced Advancements: As the field continues to evolve rapidly, students may find themselves dealing with concepts that are quickly outdated or evolving, leading to challenges in staying up-to-date with the most relevant material for exams.
Common Mistakes in Quantum Physics Exams
When tackling assessments in advanced scientific fields, students often make errors that can significantly impact their performance. These mistakes typically stem from misunderstandings of fundamental principles, misapplication of mathematical methods, or overlooking the subtleties of complex theoretical concepts. Recognizing these common pitfalls can help avoid them and improve one’s ability to succeed in this challenging subject.
In this section, we will outline some of the most frequent mistakes that students make, highlighting the key areas where carelessness or lack of clarity can lead to incorrect results. Understanding these mistakes will provide valuable insight into how to approach tasks more effectively and ensure a stronger grasp of the subject matter.
Mathematical Missteps
One of the main sources of error is the mathematical techniques required to solve complex problems. Some of the most common issues in this area include:
- Misapplication of Operators: Confusing the use of operators such as momentum or position, especially when they are non-commutative, can lead to incorrect outcomes.
- Incorrect Use of Formulas: Relying on memorized equations without understanding the context or the underlying principles can result in errors in calculations, particularly when these formulas are applied to new or slightly altered problems.
- Sign Errors: It’s easy to overlook negative signs when performing calculations, especially in problems involving complex numbers, leading to an entirely wrong result.
Conceptual Confusion
Understanding the deeper concepts behind the theories is just as important as applying mathematical tools. Some frequent misunderstandings include:
- Confusing Wave-Particle Duality: Failing to properly interpret the dual nature of particles–both as waves and as discrete entities–can result in misinterpretation of experimental data.
- Overlooking Superposition: A common mistake is not fully appreciating how particles can exist in multiple states simultaneously, which can lead to incorrect conclusions about measurement and state collapse.
- Misunderstanding Uncertainty Principle: The uncertainty principle is often misunderstood as simply a limit on measurement precision, rather than a fundamental property of nature itself, leading to inaccurate conceptualization in problem-solving.
Neglecting Boundary Conditions and Assumptions
Another common mistake is not carefully considering the assumptions or boundary conditions that apply to a given problem. This can lead to oversimplification or misapplication of principles:
- Ignoring Initial Conditions: When solving for systems, especially in wavefunctions, students often forget to properly set the initial conditions, which leads to incorrect solutions.
- Overlooking Symmetry Considerations: Symmetries in a system are crucial for simplifying calculations, and neglecting them can complicate the solution or lead to unnecessary errors.
- Underestimating Boundary Effects: In many problems, boundary conditions play a critical role in determining the behavior of systems. Ignoring them can result in solutions that do not match real-world behavior.
Strategies for Solving Quantum Problems
Solving problems in advanced scientific fields requires a structured approach, as the concepts involved can be complex and abstract. To successfully navigate these challenges, it is essential to develop effective strategies that simplify the process and ensure accurate solutions. These strategies typically involve breaking down problems into smaller, manageable parts, applying appropriate mathematical tools, and maintaining a clear understanding of the underlying principles.
In this section, we will explore key approaches that can help in tackling difficult problems. By implementing these strategies, students can improve their problem-solving skills and gain a deeper understanding of the subject matter, leading to more successful outcomes.
Understanding the Fundamentals
Before diving into the specifics of a problem, it is crucial to have a solid grasp of the basic principles. Ensuring a clear understanding of core concepts will provide the foundation for tackling more complex problems. Some key strategies include:
- Review Core Theories: Always start by reviewing the fundamental principles relevant to the problem. This includes understanding the basic laws that govern the system you are working with.
- Identify Key Variables: Pinpoint the key variables involved and how they relate to each other. This will help in determining the approach to solving the problem.
- Visualize the Problem: If possible, create a diagram or visualize the system. This can often make abstract concepts more tangible and easier to manipulate mathematically.
Applying Mathematical Techniques
Once you have a clear understanding of the principles, the next step is to apply the appropriate mathematical methods. This can often involve complex calculations, so a strategic approach is necessary:
- Start with Simplifications: Begin by simplifying the problem, using approximations or symmetries that can reduce complexity. This can make the calculations more manageable.
- Break the Problem into Steps: Solve the problem in steps. Start with a general solution and refine it, step by step, focusing on smaller, solvable components.
- Check Units and Dimensions: Ensure that all units are consistent and that the dimensions of your quantities make sense. This can help catch errors early on.
- Use Known Results: Apply known solutions or formulas wherever applicable. This can save time and improve the accuracy of your work.
Exam Tips for Quantum Physics Students
Preparing for assessments in complex scientific fields requires a well-organized approach. To perform well, it is essential to not only grasp the theoretical concepts but also apply them effectively under time pressure. Students can benefit from a range of strategies that enhance understanding, improve retention, and optimize performance during evaluations.
This section outlines practical tips that can help students navigate the challenges of studying advanced topics. By following these recommendations, learners can refine their study habits, boost confidence, and maximize their chances of success.
Effective Study Strategies
To perform well, students should adopt efficient study habits that focus on both understanding the material and practicing application. Here are some helpful techniques:
- Active Recall: Instead of passively reading through notes, actively test your knowledge by recalling concepts from memory. This improves retention and helps identify areas that need more attention.
- Practice Regularly: Working through problems regularly reinforces understanding and helps develop the ability to solve similar questions efficiently during the assessment.
- Focus on Core Principles: Ensure that the key concepts are well understood, as they form the foundation for more advanced topics. Mastery of basic principles will make tackling more complex problems easier.
- Use Multiple Resources: Don’t rely on just one textbook. Consult different learning materials, such as online resources, tutorials, or peer discussions, to gain different perspectives on challenging topics.
Time Management Tips
Time management is a crucial skill when preparing for complex tests. Effectively managing your study time will help reduce stress and improve performance:
- Create a Study Schedule: Plan your study time well in advance, ensuring that you allocate sufficient time for each topic. Focus on more challenging areas while maintaining a balance with simpler topics.
- Prioritize Important Topics: Identify key topics that are likely to appear in the evaluation and spend extra time on these areas. A focused approach can yield better results.
- Simulate Test Conditions: Practice solving problems within a set time frame. This will not only improve your speed but also help reduce anxiety during the real assessment.
Reviewing Key Quantum Physics Experiments
Exploring landmark experiments is essential for gaining a deeper understanding of the fundamental principles that govern microscopic phenomena. These critical studies not only provide empirical support for various theoretical concepts but also challenge our intuitions about the nature of reality. Reviewing these experiments helps solidify knowledge and serves as a valuable resource for addressing complex problems.
This section highlights some of the most influential experiments that have shaped modern scientific thought. By examining their results, methods, and implications, students can better appreciate the evolving nature of the field and the experimental underpinnings that validate theoretical frameworks.
Key Experiments in Understanding Microscopic Behavior
The following table presents a selection of significant experiments, offering insight into their methods, outcomes, and scientific impact:
| Experiment | Objective | Key Findings |
|---|---|---|
| Double-Slit Experiment | To demonstrate the wave-particle duality of light | Light behaves as both a wave and a particle depending on the observation method, suggesting fundamental unpredictability in measurement. |
| Photoelectric Effect | To study the interaction between light and matter | Light of a certain frequency can eject electrons from metal, providing evidence of the particle nature of light. |
| Heisenberg’s Uncertainty Principle | To investigate the limits of precision in measuring microscopic systems | It is impossible to simultaneously measure both the position and momentum of a particle with arbitrary accuracy. |
| Bell’s Theorem | To test the validity of local hidden variable theories | Demonstrated that quantum mechanics predicts correlations that cannot be explained by any local hidden variables, supporting the non-locality of quantum theory. |
Impact on Modern Science
Each of these experiments has had a profound influence on the way we understand the world at the smallest scales. Their results continue to inspire ongoing research and shape innovations in technology, from quantum computing to advanced materials. By delving into the details of these groundbreaking studies, students can build a solid foundation for future exploration and problem-solving in this fascinating domain.