
Math can often feel overwhelming, especially when encountering challenging problems that seem difficult to solve. However, breaking down each exercise step by step can lead to greater understanding and mastery. In this section, we will focus on providing clear and effective solutions to enhance your skills.
By exploring different techniques and approaches, students can improve their problem-solving abilities. It’s important to practice regularly and approach each task with a logical mindset, ensuring that each solution is both accurate and easy to understand. Clear explanations and strategic methods are key to making even the most complex questions manageable.
Math Exercise Solution Insights
Understanding the solutions to complex mathematical problems can significantly enhance a student’s problem-solving skills. By carefully analyzing each step, learners can uncover patterns and techniques that simplify seemingly complicated exercises. This section provides an in-depth look at the methods used to solve specific tasks, ensuring clarity and boosting confidence in tackling similar questions.
Approach to Solving Exercises
Breaking down each question into smaller, manageable parts helps to identify the most effective strategy for finding the solution. It’s important to focus on key concepts, such as operations and relationships between numbers, and to approach each part methodically. Consistency is crucial when solving problems, as applying the same principles repeatedly leads to greater proficiency.
Enhancing Understanding through Detailed Explanations
Clear, step-by-step breakdowns offer more than just the final result–they help to explain the reasoning behind each decision made. Thorough explanations ensure that learners not only know how to arrive at the correct answer but also understand why each step is necessary. This deeper comprehension builds a stronger foundation for tackling more advanced topics.
How to Solve Mathematical Problems Efficiently
Mastering the process of solving mathematical challenges requires a structured approach. By using effective strategies and techniques, complex problems can be simplified into manageable steps. This section will explore methods that increase efficiency, helping learners solve problems quickly and accurately while ensuring a solid understanding of the concepts involved.
Breaking Down the Problem
The first step in solving any problem is to thoroughly understand what is being asked. Begin by identifying the key elements and relationships between the variables. Once you have a clear picture, break the problem into smaller, simpler parts. Tackling each part individually will make the overall solution easier to reach.
Applying Efficient Strategies
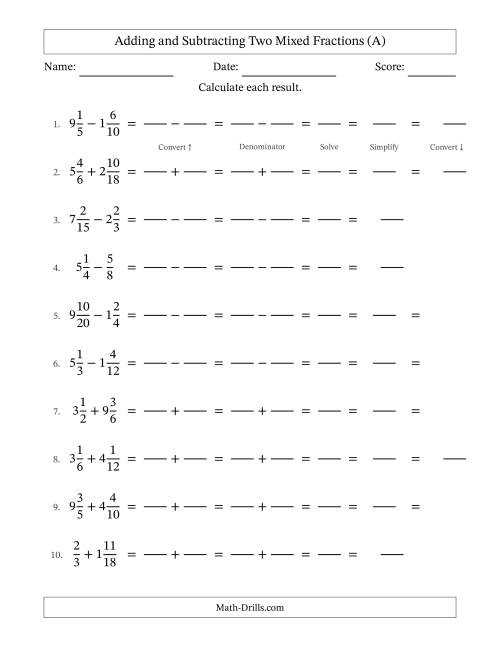
Efficiency comes from knowing which methods to apply to different types of problems. Whether it’s using substitution, factoring, or another technique, knowing when and how to use these strategies saves time and reduces the likelihood of errors. Regular practice and familiarity with various approaches will improve speed without sacrificing accuracy.
Understanding Key Concepts in Mathematical Problem Solving
Grasping fundamental ideas is essential to mastering any mathematical topic. By focusing on core principles, students can build a strong foundation that makes tackling more complex problems easier. This section explores the vital concepts that serve as the building blocks for solving mathematical exercises and how they connect to one another.
One of the most important aspects of problem-solving is recognizing patterns and relationships. Whether it’s understanding the properties of numbers or the behavior of operations, these foundational concepts help simplify the process. A solid understanding of these basics not only improves accuracy but also speeds up the time it takes to find solutions.
Step-by-Step Guide to Solving Exercises
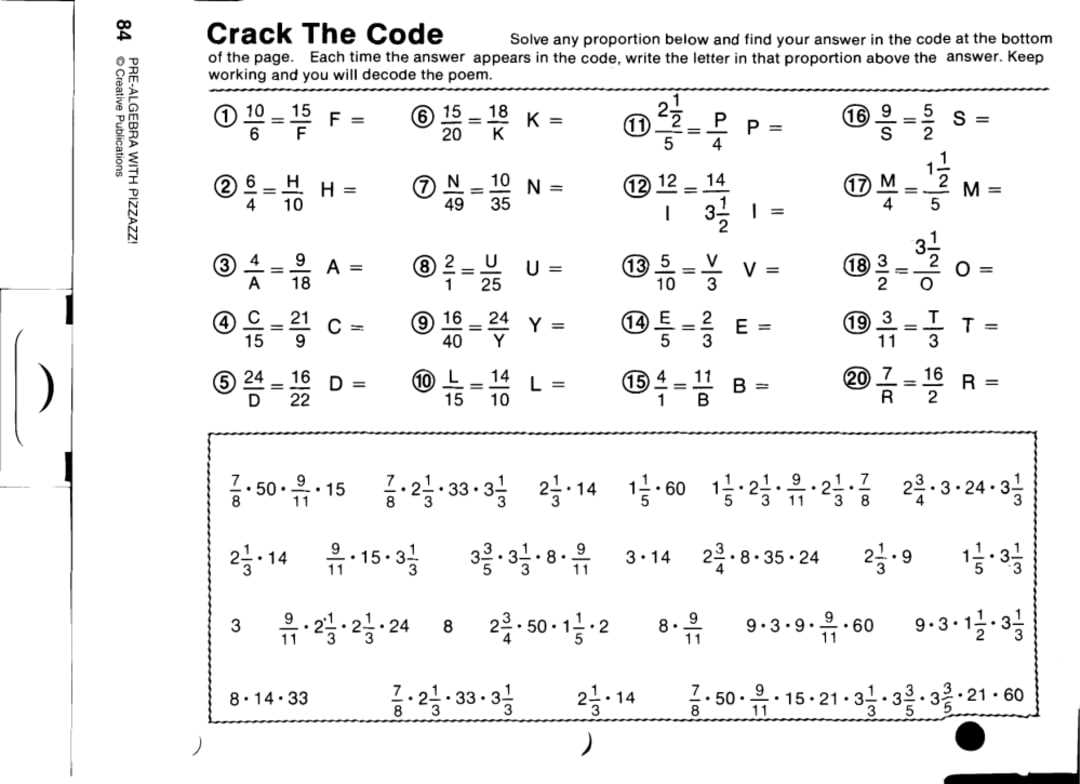
Breaking down problems into smaller, manageable parts is a key strategy for achieving success in mathematics. This section provides a structured approach to solving the exercises, ensuring that each step is clearly explained and easy to follow. By following this guide, learners will be able to confidently work through challenges and understand the reasoning behind each solution.
Start by identifying the core elements of each task. Focus on understanding the relationships between the numbers or variables, and think about the operations needed to simplify or solve the problem. As you move through each step, check your work to ensure accuracy, and take note of any patterns that might emerge to help with future problems.
Common Mistakes in Mathematical Problem Solving and How to Avoid Them
Even experienced learners can make errors when solving mathematical exercises. These mistakes often occur due to misunderstandings of basic concepts or rushing through steps. Identifying these common pitfalls is the first step in improving accuracy and confidence. In this section, we’ll explore frequent errors and provide tips for avoiding them.
A common mistake is neglecting to properly follow the order of operations. Skipping steps or applying operations in the wrong order can lead to incorrect results. Always double-check the sequence in which calculations should be performed. Additionally, overlooking negative signs or misinterpreting word problems can cause confusion. Paying close attention to details and taking the time to understand each part of the task helps prevent such errors.
Why This Approach Works for Students
Effective learning requires engagement and clarity, and certain methods have proven to be particularly successful in helping students understand complex concepts. This approach not only breaks down challenging tasks but also makes the process enjoyable. By incorporating creativity and structure, students are able to grasp difficult topics more easily and retain the knowledge longer.
Key Benefits of This Approach
- Interactive Exercises: Engaging students through various activities encourages them to think critically and apply what they’ve learned.
- Structured Learning: A well-organized framework helps students process and retain material more effectively, reducing frustration.
- Immediate Feedback: The ability to check solutions quickly ensures that students can identify mistakes early and learn from them.
How Students Benefit
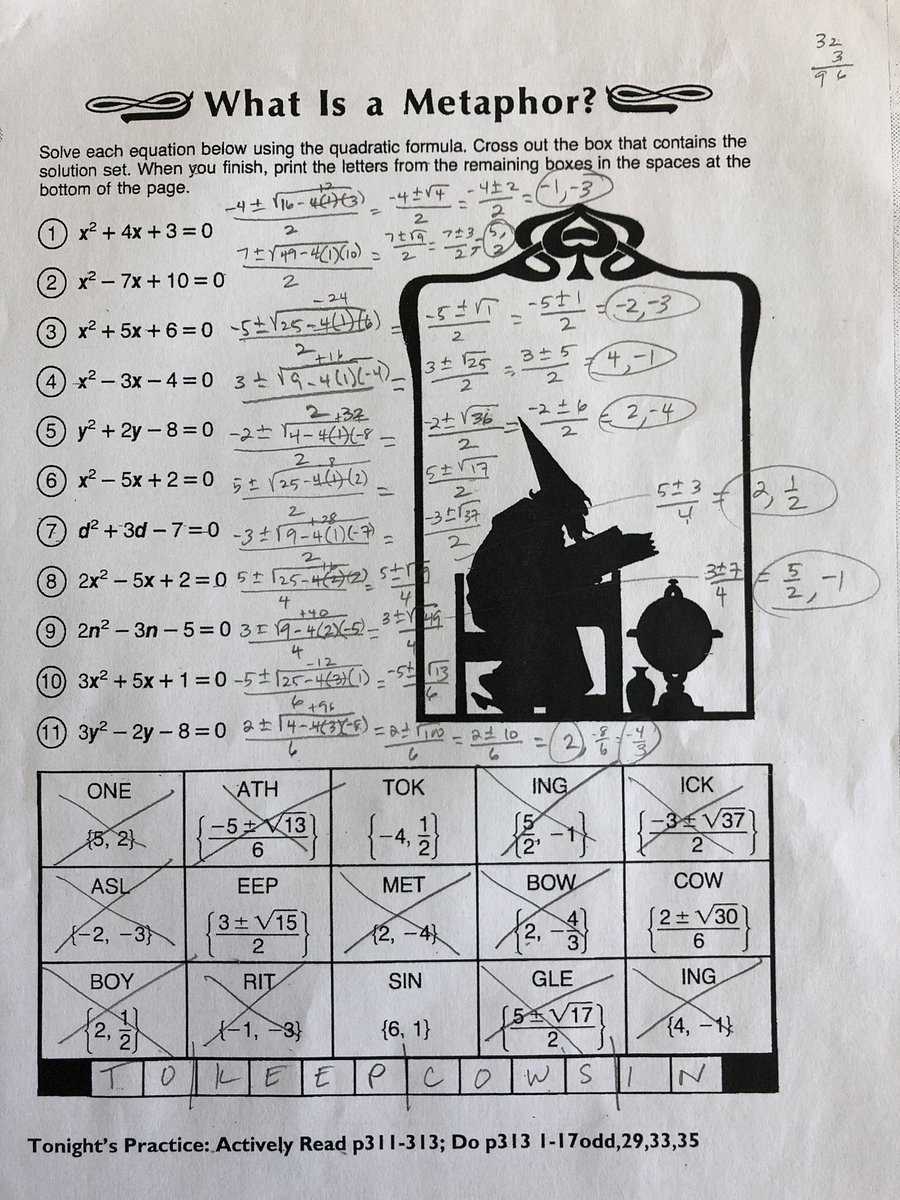
Students who use this method report a greater sense of accomplishment and confidence in their abilities. The clear explanations and varied exercises provide a solid foundation that makes even the most complex tasks manageable. Over time, these tools help improve overall performance and foster a positive attitude towards learning.
Tips for Improving Your Mathematical Skills
Improving your problem-solving abilities requires consistent practice and a focus on mastering the fundamentals. By strengthening your understanding of core concepts and developing effective strategies, you can approach even the most difficult challenges with confidence. This section offers practical advice for enhancing your mathematical skills and building a solid foundation for more advanced topics.
One of the most important tips is to practice regularly. The more problems you solve, the more familiar you become with different types of tasks and techniques. Don’t shy away from mistakes–each error is an opportunity to learn and refine your approach. Additionally, always review your work to ensure accuracy, and try different methods to find the most efficient solution for each problem.
How Math Helps in Real-World Scenarios
Mathematical concepts are not just abstract ideas–they have practical applications that influence many aspects of everyday life. From budgeting and shopping to engineering and technology, the ability to solve mathematical problems enables us to make informed decisions and navigate the world more effectively. This section explores how the skills gained from solving mathematical exercises are used in real-world situations.
One key area where these skills are essential is financial management. Whether you’re calculating the total cost of a purchase, figuring out interest rates, or planning for long-term savings, mathematical reasoning plays a crucial role. Below is an example of how math can be used to calculate the total cost of an item after tax:
| Item Price | Tax Rate | Tax Amount | Total Cost |
|---|---|---|---|
| $50 | 8% | $4 | $54 |
By applying mathematical skills, you can easily calculate the total cost by determining the tax amount and adding it to the original price. This basic concept of working with percentages and simple operations has wide-reaching implications across various sectors and daily tasks.
Breaking Down Complex Mathematical Expressions
When faced with intricate mathematical problems, it can be difficult to know where to begin. The key to solving these challenges lies in breaking them down into smaller, more manageable parts. By understanding the underlying components and applying systematic approaches, complex expressions can be simplified step by step, making even the most complicated tasks accessible.
The first step is to identify the different elements within the expression, such as constants, variables, and operators. From there, it’s important to apply the appropriate order of operations to reduce the complexity. Techniques like factoring, expanding, or grouping terms can help reveal a more straightforward path to the solution. Always remember to check your work as you go along to ensure accuracy and clarity in each step.
Using the Solution Guide Effectively
While it’s important to work through problems independently, having access to a solution guide can greatly enhance your learning process. However, it’s essential to use this resource wisely to gain the most benefit. Simply looking at the answers without understanding the steps can limit your progress. Instead, follow a strategic approach to maximize your understanding and retention of the material.
Best Practices for Using the Solution Guide
- Attempt Problems First: Before consulting the guide, try solving the problem on your own. This helps you identify areas where you need improvement.
- Understand the Steps: Review the solutions carefully and focus on understanding how each step was reached. Ask yourself why each action was taken.
- Check Your Work: Compare your approach with the solution guide to identify any mistakes. Understanding where you went wrong can be more valuable than simply seeing the correct result.
- Practice Regularly: Revisit similar problems after studying the guide to reinforce your learning. Repetition is key to mastering the concepts.
By using the solution guide as a learning tool rather than just a reference, you can build a deeper understanding of the material and improve your problem-solving skills over time.
What Makes This Method Unique
What sets this approach apart is its ability to combine creativity and structure in a way that makes learning both effective and engaging. It emphasizes not only understanding core concepts but also enjoying the process of learning. Through unique exercises and a fun, interactive format, students are encouraged to explore mathematical problems in a fresh and motivating way.
Engaging Exercises
One of the standout features is the variety of problems that cater to different learning styles. These tasks are designed to challenge students while keeping them interested, offering puzzles and problems that encourage critical thinking. This combination of enjoyment and challenge helps students build confidence and competence in their skills.
Clear and Structured Learning
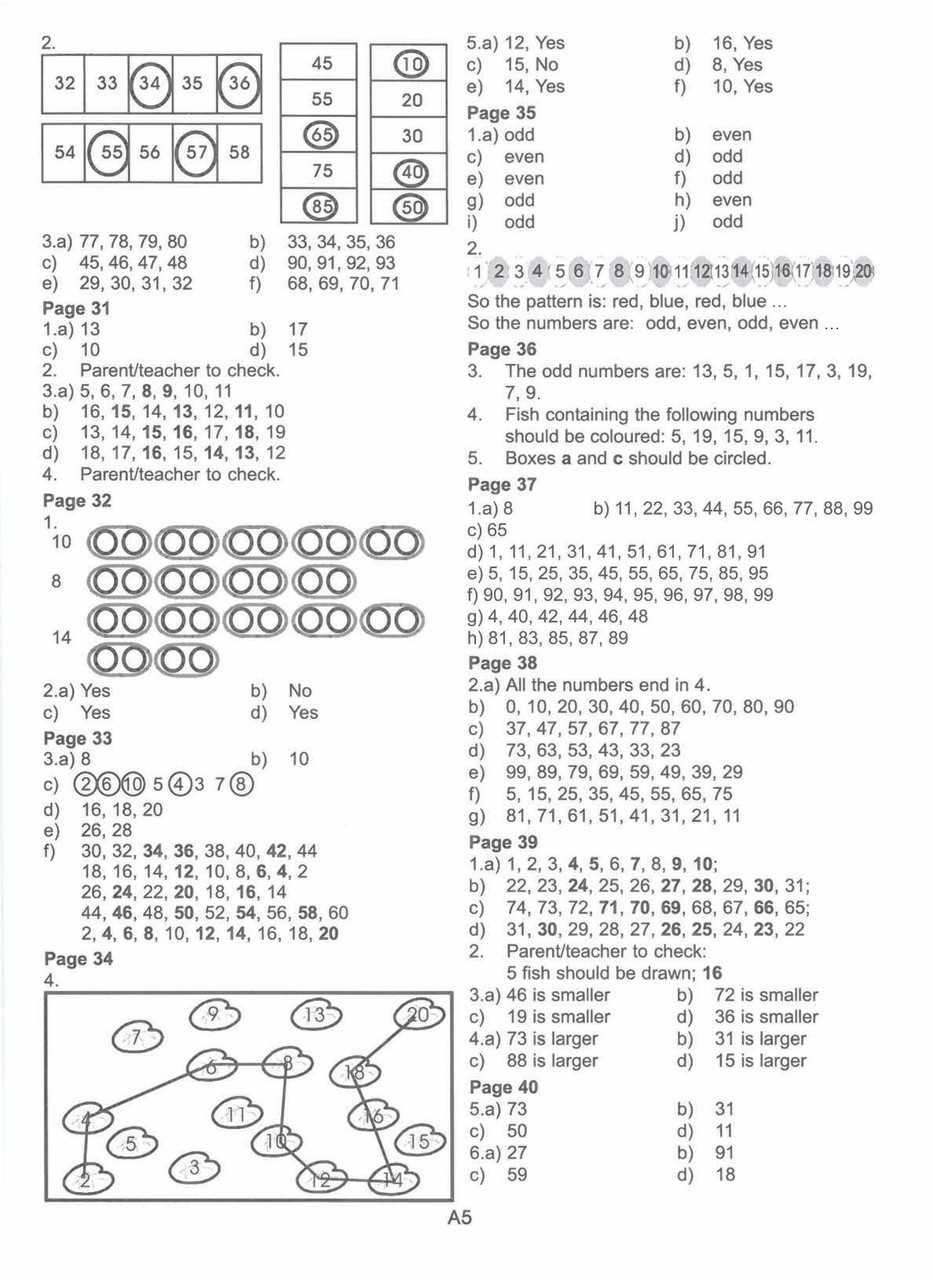
In addition to being engaging, the method provides a clear path to mastery. Each topic is broken down into manageable sections, allowing students to progress step-by-step. This structured approach ensures that students build a solid foundation before tackling more complex topics, making the learning experience both satisfying and rewarding.
Building Confidence in Learning
Developing confidence in solving mathematical problems is a crucial part of mastering the subject. Many students face challenges when approaching complex problems, but by building a strong foundation and practicing regularly, they can gain the confidence needed to tackle more difficult tasks. This section explores strategies that can help students feel more assured in their learning journey.
Effective Strategies for Building Confidence
- Start with the Basics: Begin by mastering fundamental concepts before moving to more advanced material. A solid understanding of core ideas builds confidence for more complex problems.
- Practice Regularly: Consistent practice is key. The more problems you solve, the more familiar and confident you’ll become with the techniques required.
- Break Problems into Steps: Complex problems can seem overwhelming. By breaking them down into smaller, manageable steps, students can avoid frustration and stay motivated.
- Celebrate Small Wins: Every solved problem, no matter how small, is a step toward greater understanding. Acknowledge these victories to build a positive mindset.
Overcoming Common Challenges
It’s normal to feel uncertain or frustrated when faced with new or challenging material. However, learning from mistakes is an essential part of growth. By viewing setbacks as learning opportunities and approaching each problem with a patient, problem-solving mindset, students can gradually build the resilience and confidence needed for success.
How to Approach Word Problems in Mathematics
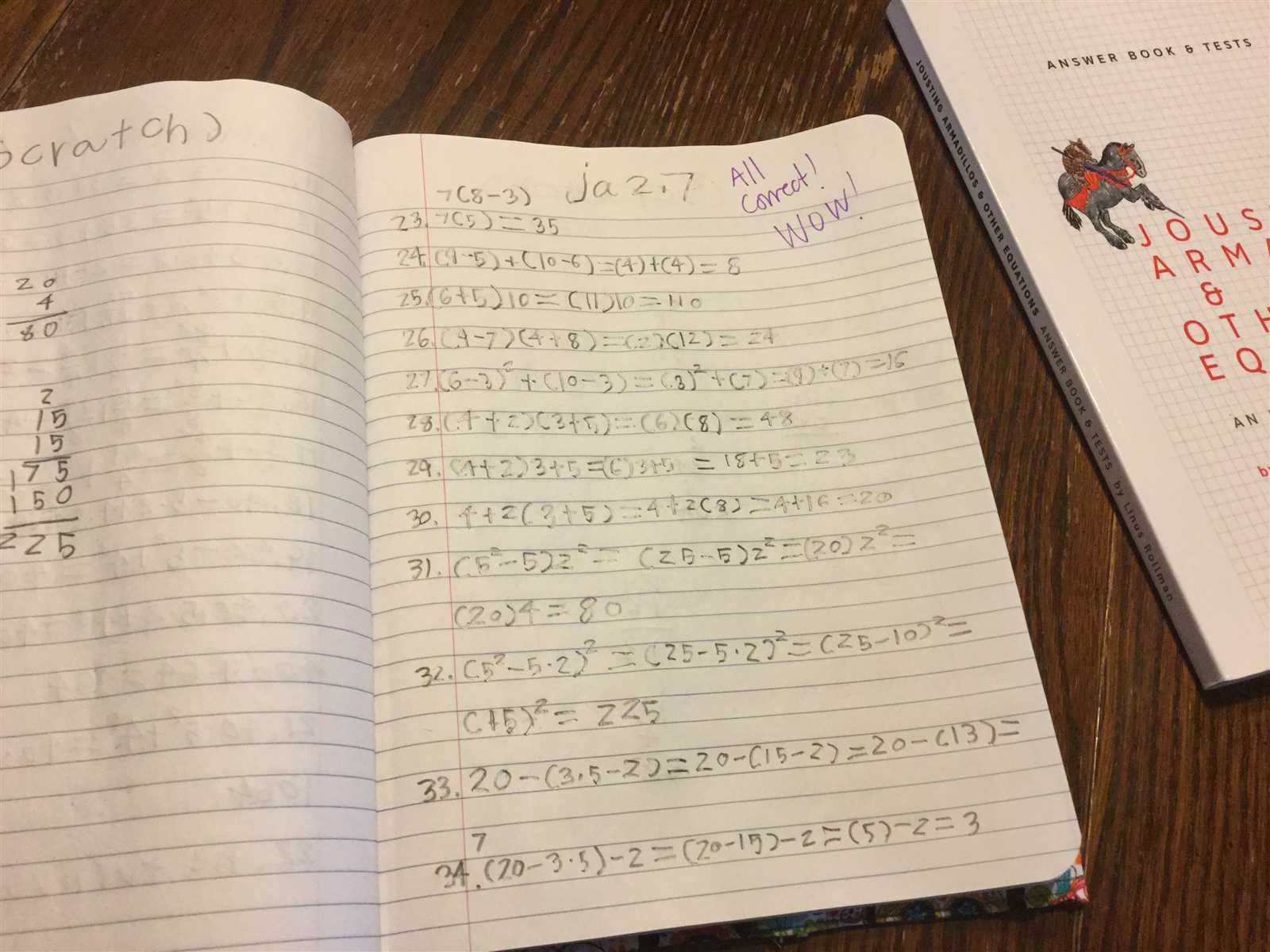
Word problems can often seem intimidating because they require not just mathematical skills, but also the ability to translate a real-world situation into a solvable equation. However, with the right approach, these problems can become much more manageable. The key is to break down the information and follow a structured method to extract the relevant details and apply the appropriate strategies for solving them.
Steps for Solving Word Problems
- Read the Problem Carefully: Take your time to understand the situation. Identify key facts, numbers, and relationships within the text.
- Define Variables: Assign symbols or letters to unknowns, making it easier to form equations.
- Translate into Mathematical Language: Convert the words into mathematical expressions or equations based on the relationships between the variables.
- Solve the Equation: Use appropriate methods or formulas to find the unknown values, keeping the structure of the problem in mind.
- Check Your Work: After solving, go back through the problem to ensure that your solution makes sense in the context of the original scenario.
Tips for Success
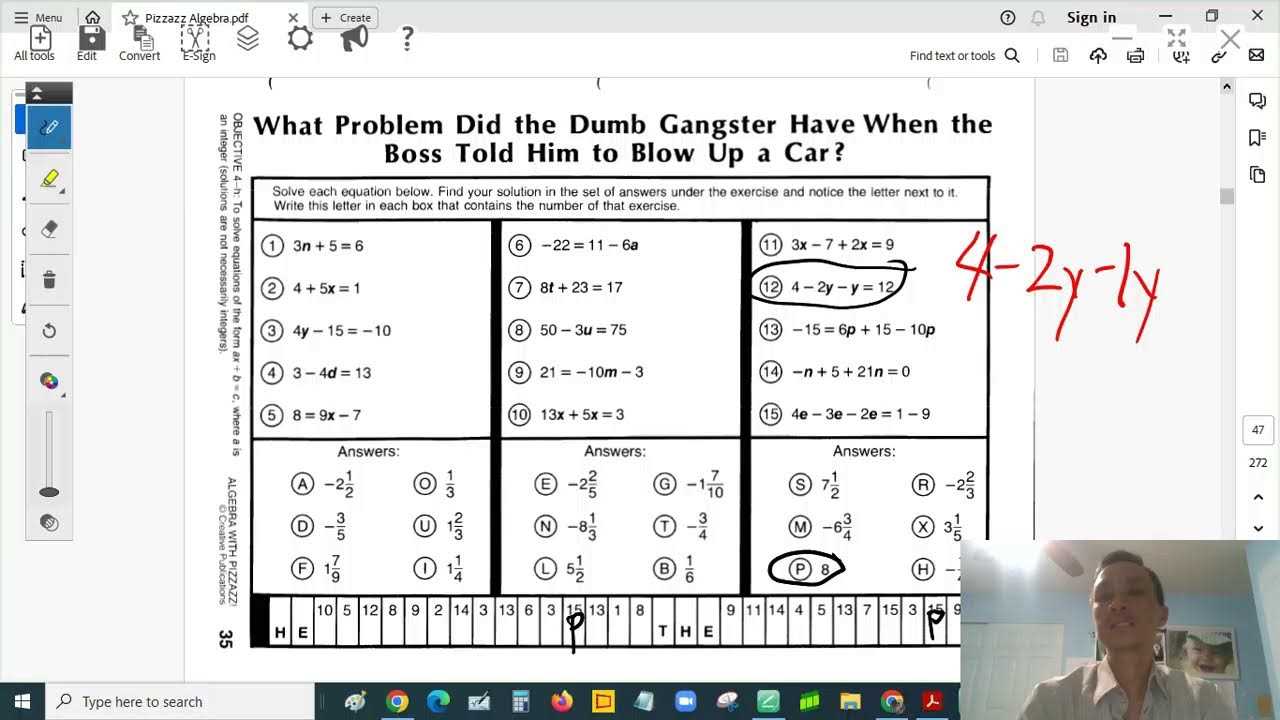
- Identify Keywords: Words like “total,” “difference,” “product,” or “per” often signal specific mathematical operations.
- Draw Diagrams: Visualizing the problem can help you understand it more clearly and identify relationships between the elements.
- Practice Regularly: The more word problems you solve, the more familiar you’ll become with different problem types and techniques.
By following these steps and practicing consistently, word problems will become less daunting, and you’ll be able to approach them with greater confidence and efficiency.
Mastering Basic Mathematical Operations
Understanding the fundamental operations is crucial for solving mathematical expressions and equations efficiently. These basic skills form the foundation of more complex problem-solving techniques. By mastering addition, subtraction, multiplication, and division, students can confidently tackle a wide range of problems and develop stronger mathematical reasoning abilities.
Key Operations to Focus On
- Addition: The process of combining numbers to find their sum. Practice with both positive and negative numbers to build fluency.
- Subtraction: This operation involves finding the difference between two numbers. Mastering subtraction of positive and negative values is essential.
- Multiplication: Repeated addition of a number. Understanding times tables and the distributive property will help improve speed and accuracy.
- Division: Splitting a number into equal parts. Division is the inverse operation of multiplication and requires understanding both long division and fractional division.
Strategies for Mastery
- Practice Regularly: Repetition is key to reinforcing these skills. The more you practice, the more intuitive these operations become.
- Understand the Rules: Recognizing patterns and knowing how operations work with positive and negative numbers will help you handle complex problems.
- Use Visual Aids: Drawing number lines or using manipulatives can help visualize the relationship between numbers in operations.
- Apply to Real-Life Situations: Apply these basic operations to everyday scenarios, such as budgeting, shopping discounts, or measuring ingredients in recipes.
By mastering these basic operations, you will build a solid foundation that will allow you to tackle more advanced mathematical challenges with confidence and ease.
Visualizing Mathematical Problems for Better Understanding
Visualizing mathematical problems can greatly enhance comprehension and problem-solving abilities. By translating abstract concepts into visual forms, students can make sense of complex relationships between numbers and variables. This approach helps to break down intimidating equations into more manageable parts, making it easier to identify patterns and solve problems efficiently.
Effective Visualization Techniques
- Graphs and Charts: Plotting equations on graphs allows for a clearer understanding of how variables interact. This is especially useful for understanding linear relationships or identifying trends in data.
- Diagrams and Models: Using visual models such as number lines or geometric shapes can help represent operations and equations visually. This can make abstract concepts, like adding or subtracting variables, more tangible.
- Tables: Organizing data or steps in a table format can simplify the process of solving equations, helping to track progress and see patterns more clearly.
Practical Example: Solving an Equation
Consider the equation 2x + 3 = 7. Visualizing this can help clarify the steps needed to solve it. First, plot the equation on a number line, marking the constants and the variable. Then, manipulate the equation step by step, adjusting the number line to represent each operation. This method provides a clear view of the problem and solution process.
| Step | Equation | Visualization |
|---|---|---|
| 1 | 2x + 3 = 7 | Start with the equation on a number line. |
| 2 | 2x = 4 | Subtract 3 from both sides to isolate the variable. |
| 3 | x = 2 | Divide both sides by 2 to solve for x. |
By visualizing the problem and solution, the steps become more intuitive, and the relationship between the numbers is easier to grasp. Using these methods can improve both understanding and speed when tackling similar problems.
Preparing for Math Tests with Solution Guides
Effective test preparation requires both practice and understanding of how to approach problem-solving systematically. Using solution guides not only helps to reinforce concepts but also provides a way to evaluate your progress and refine your methods. These tools can be extremely beneficial when revisiting complex problems, ensuring you are ready for the test and confident in your abilities.
Steps for Efficient Test Preparation
- Review Key Concepts: Before diving into problems, ensure that you have a solid understanding of the fundamental concepts. Reviewing notes or textbook examples can help clarify any doubts.
- Practice Regularly: The more problems you solve, the more familiar you become with various techniques. Consistent practice ensures that you are prepared for any problem type that might appear on the test.
- Check Your Work: After solving problems, use a solution guide to verify your answers. Understanding where mistakes occurred helps you avoid repeating them during the test.
- Understand Step-by-Step Solutions: Simply looking at answers is not enough. Go through each step carefully to understand the reasoning behind every move. This helps build problem-solving skills that can be applied to new, unfamiliar problems.
Practical Example: Solving an Equation
Let’s consider the equation 3x – 5 = 10. Here’s how to break it down step by step using a solution guide:
| Step | Equation | Explanation |
|---|---|---|
| 1 | 3x – 5 = 10 | Start with the given equation. |
| 2 | 3x = 15 | Add 5 to both sides to isolate the term with the variable. |
| 3 | x = 5 | Divide both sides by 3 to solve for x. |
By following each step, you can see exactly where and why each action is taken. This methodical approach, combined with reviewing the solution guide, will ensure that you are well-prepared for any problem that comes your way during a test.