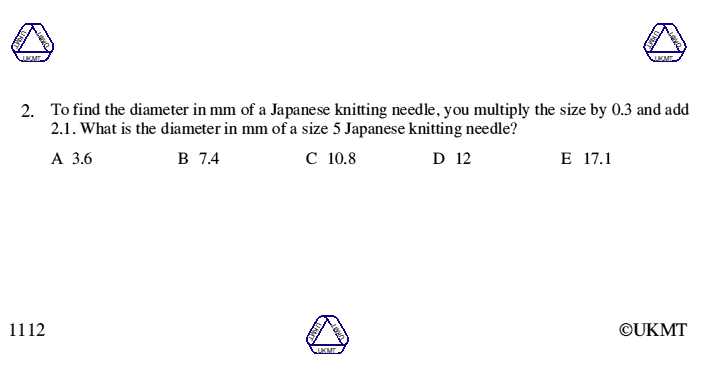
Competitions that challenge students’ problem-solving skills offer a unique opportunity to test mathematical understanding. These contests are designed to push participants to think critically, analyze complex scenarios, and apply various strategies to find the best solutions. With a variety of question types and difficulty levels, these events help foster a deep appreciation for logic and reasoning.
In this section, we will provide a comprehensive breakdown of the questions from a particular competition, focusing on detailed solutions and strategies. By reviewing each problem in depth, we aim to help participants and enthusiasts understand the reasoning behind each solution. Whether you’re preparing for a future contest or simply looking to improve your problem-solving abilities, these explanations will offer valuable insights.
Our goal is not only to present the correct solutions but also to highlight effective approaches for tackling challenges of this nature. By studying the steps taken to arrive at the answers, participants can improve their problem-solving techniques and gain a clearer understanding of the mathematical concepts involved. Thorough analysis and careful explanation are key to mastering these types of problems.
Math Kangaroo 2011 Answers Overview
This section provides an in-depth look at the solutions to the questions posed in a renowned global competition. By examining each problem carefully, we aim to offer valuable insights into the logical approaches and strategies needed to solve them effectively. Our goal is to give readers not only the correct solutions but also an understanding of how to approach similar challenges in the future.
Key Insights into the Problem-Solving Process
The competition featured a variety of problems that tested both basic concepts and advanced techniques. Each question was designed to assess different aspects of mathematical thinking, from pattern recognition to critical analysis. Understanding the reasoning behind each solution is crucial for enhancing problem-solving skills and improving performance in similar events.
Approaches to Improve Performance
To excel in these types of competitions, it’s essential to develop a range of problem-solving methods. Studying solutions carefully and recognizing the patterns used in each step can greatly improve your approach to future challenges. By focusing on strategic thinking, participants can hone their skills and gain confidence in their ability to tackle even the most difficult problems.
Understanding the Competition Format
Each year, the global event follows a structured format designed to test participants’ abilities across a range of mathematical concepts. The format is carefully crafted to challenge both basic and advanced reasoning skills, ensuring that every level of student can engage with the problems presented. Understanding this format is key to navigating the contest successfully.
General Structure of the Event
The competition consists of multiple-choice questions with varying levels of difficulty. Each question is designed to assess a specific mathematical skill or concept. Participants are given a limited amount of time to complete the entire set of problems, making time management a critical aspect of the event.
- Multiple Choice Questions: Each question has a set of potential answers, one of which is correct.
- Varied Difficulty Levels: Problems range from simple to complex, allowing participants to demonstrate their skills across a spectrum of challenges.
- Time Constraints: Participants must work within a fixed timeframe, emphasizing efficiency in problem-solving.
Preparing for the Format
To perform well, it’s essential to understand the time constraints and the types of questions that may appear. Focusing on logical reasoning, pattern recognition, and problem-solving strategies will provide a strong foundation for tackling the diverse questions presented during the contest.
- Familiarize yourself with the structure of multiple-choice questions.
- Practice solving problems under time pressure to build efficiency.
- Review a wide range of mathematical concepts to be prepared for all question types.
Detailed Solutions for All Questions
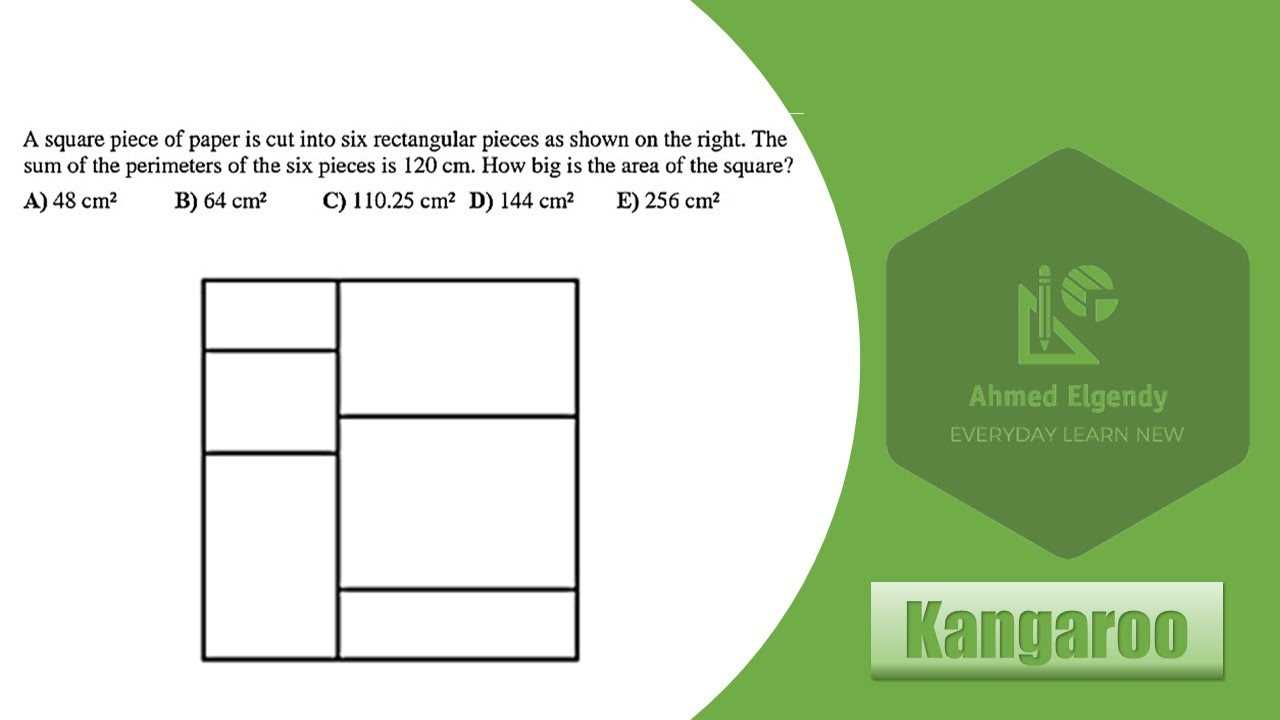
This section provides comprehensive explanations for each problem presented in the competition, offering step-by-step solutions that demonstrate the best approaches to solving them. The goal is to break down the reasoning behind each answer, ensuring that participants understand not only the correct solution but also the methodology that leads to it.
Step-by-Step Approach
Each problem is carefully analyzed, and the solution is presented in a clear, logical sequence. The process begins with identifying the core concept being tested and follows through with the necessary calculations or logical deductions. By outlining each step, we aim to make the solution process transparent and easy to follow.
- Problem Breakdown: We start by identifying the key components of each question to determine the appropriate approach.
- Solution Process: A detailed explanation of how to solve the problem is provided, including intermediate steps and reasoning.
- Final Answer: We conclude with the correct answer, along with an explanation of how it was derived.
Conceptual Insights
Understanding the underlying concepts behind each problem is crucial for tackling similar challenges in the future. We highlight important mathematical principles and techniques that can be applied not only to this competition but to a wide range of problem-solving scenarios.
Tips for Solving Competition Problems
To succeed in problem-solving competitions, it’s essential to approach each question with a clear strategy and mindset. With the right techniques and preparation, even the most challenging problems can be tackled effectively. This section provides useful tips to enhance your performance and improve your problem-solving skills.
Effective Strategies for Tackling Problems
Successful competitors rely on several key strategies to approach problems efficiently. The following tips will help you identify the most effective methods to apply in any situation.
- Understand the Problem: Carefully read each question to identify what is being asked. Break down complex problems into smaller, manageable parts.
- Eliminate Incorrect Options: For multiple-choice questions, narrow down the options by eliminating those that are obviously wrong.
- Manage Your Time: Allocate time for each question based on its difficulty. Avoid spending too much time on any single problem.
- Check Your Work: If time allows, review your answers to ensure they are accurate and complete.
Preparation Tips
Preparation is key to performing well in competitive environments. The following steps will help you develop the skills needed to succeed.
- Practice regularly with a variety of problems to build familiarity with different types of questions.
- Work on improving your speed without sacrificing accuracy.
- Review past competitions to understand the format and question patterns.
Competition Answer Key
This section provides the complete key to the problems from the contest, outlining the correct solutions for each question. The goal is to give participants a clear reference to check their results and understand where they may have made errors. The key is organized in a format that matches the original structure of the competition, allowing for easy comparison of answers.
| Question Number | Correct Answer |
|---|---|
| 1 | A |
| 2 | C |
| 3 | B |
| 4 | D |
| 5 | A |
| 6 | C |
| 7 | B |
| 8 | D |
| 9 | A |
| 10 | C |
Breaking Down Each Competition Section
To better understand the structure of the event and the types of questions it presents, we will examine each section individually. The competition is divided into several parts, each with a distinct focus, testing a variety of mathematical skills. By analyzing each section, participants can identify areas of strength and opportunities for improvement.
Section 1: Basic Concepts and Logic
The first section generally focuses on foundational mathematical concepts, such as arithmetic, number theory, and basic geometry. Questions in this part are designed to assess logical thinking and the ability to apply simple rules to solve problems quickly. Participants can expect a series of straightforward questions that require solid understanding of basic principles.
Section 2: Intermediate Problem-Solving
The second section often increases in complexity, introducing problems that require more advanced reasoning and the application of multiple concepts. Participants may need to combine skills from different areas, such as algebra, probability, and pattern recognition, to find the correct solutions. While the problems remain solvable, they require a higher level of analysis and creative thinking.
Strategies for Tackling Competition Questions
Approaching competitive problem-solving requires a blend of preparation, logical thinking, and efficient strategies. To succeed in these types of events, it’s essential to have a plan for how to approach each question. By using the right techniques, you can improve your ability to solve problems quickly and accurately, even under time pressure.
One of the most effective strategies is to first read the question carefully and identify the key elements. Focus on understanding what is being asked before jumping into calculations. Often, a thoughtful approach can reveal shortcuts or patterns that simplify the problem-solving process.
Another useful technique is to start with the easier questions to build confidence and secure quick points. Once these are completed, you can move on to more challenging problems, which will require deeper thinking and perhaps multiple steps to solve. Time management is key in ensuring that you don’t get stuck on one problem for too long.
Additionally, practicing with past questions and similar problems will help you become familiar with the types of questions that typically appear. This will give you a better sense of how to approach unfamiliar problems and recognize common patterns.
Common Mistakes in the Competition
During problem-solving contests, participants often make a few recurring mistakes that can hinder their performance. These mistakes can be avoided with proper preparation and a clear strategy. In this section, we highlight some of the most frequent errors made by participants and offer advice on how to prevent them.
Common Mistakes to Avoid
One of the most common issues is misunderstanding the question. Many participants rush through the instructions or miss important details that affect the solution process. It’s crucial to read each question carefully and ensure you fully understand what is being asked before attempting a solution.
- Misinterpreting the Question: Often, problems contain extra information or tricky wording that can lead to confusion. Take time to clarify each part of the question before starting.
- Skipping Steps: In the rush to solve a problem quickly, it’s easy to skip intermediate steps, leading to mistakes in calculations or logic. Ensure you follow each step systematically.
- Overcomplicating Simple Problems: Some problems may seem more complex than they are. Avoid overthinking by looking for simpler, more straightforward solutions.
Time Management Errors
Another common mistake is poor time management. Participants often spend too much time on difficult questions and neglect the easier ones. It’s important to allocate your time wisely across the entire set of problems.
- Start with the easiest questions to secure quick points.
- If a question seems too difficult, skip it and return to it later.
- Keep an eye on the clock to avoid running out of time for the remaining questions.
Problem Types Explained
In any problem-solving competition, understanding the different types of problems you may encounter is essential for efficient preparation. Each type of question is designed to test specific skills, ranging from basic arithmetic to complex reasoning. By familiarizing yourself with the common categories of questions, you can approach them with the right strategies and mindset.
Categories of Problems
The problems in this competition can be divided into several distinct types. Each one requires different techniques and approaches to solve effectively. Below are the most common problem types you will encounter.
- Arithmetic Problems: These questions test basic number operations such as addition, subtraction, multiplication, and division. They often require quick mental calculations and a strong grasp of basic concepts.
- Algebraic Problems: Algebraic questions involve equations, expressions, and patterns. You may need to simplify expressions or solve for unknowns, requiring a solid understanding of algebraic rules.
- Geometry Problems: Geometry questions involve shapes, angles, areas, and volumes. These problems test spatial reasoning and the ability to apply geometric principles to solve real-world scenarios.
- Logical Reasoning: These problems assess your ability to think logically and solve puzzles. They often involve patterns, sequences, and deduction, challenging your problem-solving skills.
- Word Problems: Word problems provide real-life scenarios where mathematical concepts must be applied. They require reading comprehension, translating words into equations, and solving them step-by-step.
Approaching Different Problem Types
Each type of problem requires a slightly different strategy. Here are a few tips to help you solve them more effectively:
- For arithmetic questions, focus on speed and accuracy. Practice mental math to improve your reaction time.
- For algebra, break down equations into smaller parts and use substitution or elimination methods when necessary.
- For geometry, draw diagrams whenever possible to better visualize the problem.
- For logical reasoning, look for patterns and use process of elimination to narrow down possibilities.
- For word problems, carefully identify key information and translate it into mathematical operations before solving.
Step-by-Step Approach to Solutions
A systematic approach to solving problems is crucial for achieving success in any competitive event. By breaking down each question into smaller, manageable steps, you can ensure clarity and accuracy in your solutions. This step-by-step method not only helps to organize your thoughts but also reduces the chances of making careless errors.
The first step is to carefully read and understand the problem. Before you begin solving, make sure you know exactly what is being asked. Look for key details, such as known values, what needs to be determined, and any restrictions or conditions provided in the question.
Next, identify the relevant concepts or formulas that can help you solve the problem. This could involve recalling basic principles, such as arithmetic rules, algebraic identities, or geometric properties. Having a clear mental reference of the necessary tools can streamline the process.
Once you have a plan, start solving the problem step by step. Break down each task into smaller parts and tackle them one at a time. If the problem involves multiple steps, focus on completing one section before moving to the next. This approach minimizes confusion and helps keep track of your progress.
Finally, after completing the solution, take a moment to double-check your work. Verify that all calculations are correct and that the final answer makes sense in the context of the question. A quick review can often catch small mistakes that might have been overlooked in the initial solution process.
Key Insights from the Competition
Reflecting on the experiences of previous years, there are several key lessons and patterns that emerge, which can greatly enhance preparation for future events. By analyzing past contests, we can identify areas of focus, key problem-solving strategies, and approaches that can improve performance in future challenges. These insights provide valuable guidance for participants aiming to excel in similar events.
Common Themes and Concepts
One of the key takeaways is that many questions are designed to test a participant’s ability to apply basic concepts in creative ways. The problems often involve simple principles but are framed in a way that challenges your ability to think critically and logically. Recognizing the recurring themes across different question types can help participants prepare more effectively.
Another significant insight is the importance of time management. Many students tend to spend too much time on individual questions, which can lead to rushing through easier problems. Learning to balance time across all questions ensures that more points can be secured in the time available.
Strategy for Success
Effective preparation involves practicing under timed conditions to simulate the pressure of the actual event. Additionally, focusing on problem-solving techniques such as breaking down complex problems into smaller steps, identifying patterns, and eliminating incorrect answers can lead to improved results.
| Focus Area | Actionable Insight |
|---|---|
| Critical Thinking | Apply basic concepts in novel ways to solve challenging problems. |
| Time Management | Distribute time wisely across questions to avoid spending too long on any single problem. |
| Preparation | Practice under timed conditions and simulate competition scenarios. |
| Problem Solving | Break down complex problems into simpler steps and identify patterns. |
How to Prepare for the Competition
Effective preparation for any competitive event involves a combination of focused practice, strategy, and mental readiness. By understanding the structure of the challenge and identifying key areas to focus on, participants can improve their problem-solving skills and increase their chances of success. Preparation is not just about practicing problems, but also about developing a structured approach to tackle challenges efficiently.
Key Areas to Focus On
Start by familiarizing yourself with the types of problems commonly presented in the competition. These can range from basic arithmetic and algebra to logic-based puzzles and geometry. Recognizing the patterns in problem types allows you to concentrate on areas where you may need improvement. Practice is essential, but targeted practice is even more effective.
In addition to practicing specific problem types, it’s important to work on speed and accuracy. Many participants struggle to balance both, but with regular timed practice, you can develop a sense of how to efficiently allocate your time across questions. This is especially important for competitive settings where every second counts.
Effective Study Strategies
One of the most effective ways to prepare is by taking practice tests under conditions similar to the actual event. This helps build endurance and familiarize you with the format. Reviewing your mistakes and understanding why an answer is incorrect is crucial to preventing those same errors in the future.
It is also helpful to work with peers or mentors who can provide additional insights and explain difficult concepts. Collaborative learning can expose you to different problem-solving strategies and provide new perspectives on challenging questions.
Answer Clarifications for the Competition
When preparing for or reviewing past challenges, it is crucial to understand the reasoning behind each solution to ensure comprehensive learning. Clarifications of specific answers help participants grasp the underlying principles and refine their approach to similar problems. By breaking down each solution step by step, you can improve both your problem-solving skills and your understanding of the logic involved.
Understanding the Process is essential in this context. Many problems in competitions are designed to test not just basic knowledge but also the ability to apply concepts in unfamiliar settings. Clarifying why certain answers are correct and why others are not can shed light on common pitfalls and help avoid future mistakes. Each explanation contributes to a deeper understanding of the method used to arrive at the correct solution.
Reviewing Mistakes is a valuable part of the learning process. When you revisit questions and identify why an answer was incorrect, you can recognize patterns of errors, whether they stem from misreading the problem or rushing through calculations. This self-reflection allows you to adjust your strategy for future problems, ensuring a more effective approach when facing similar challenges.
In addition to reviewing solutions, it is beneficial to work through alternative strategies for the same problem. This helps build flexibility in problem-solving and improves your ability to adapt to different question types. Exploring multiple methods encourages a deeper understanding and better prepares you for unexpected questions in future events.
Mathematical Concepts from the Competition
The competition presents a variety of mathematical principles designed to test a participant’s ability to think critically and apply knowledge in different contexts. The problems often involve concepts that span basic arithmetic to more complex topics, such as logic, algebra, and geometry. By understanding these fundamental ideas, participants can improve their problem-solving skills and develop a stronger mathematical foundation.
Key Concepts Explored
Among the key concepts frequently covered are:
- Number Theory – Examining properties of integers, divisibility rules, and prime numbers.
- Geometry – Understanding shapes, angles, areas, and volume calculations.
- Combinatorics – Focusing on counting, permutations, and combinations.
- Algebra – Solving equations, understanding variables, and manipulating expressions.
Problem Structure
The problems typically present a series of challenges that require not only the application of specific formulas or techniques but also the ability to think creatively. For example, a participant might be asked to apply geometric reasoning to solve a problem involving both shape properties and algebraic manipulation. This cross-disciplinary approach ensures that contestants develop a well-rounded understanding of various mathematical fields.
In addition, the competition often includes questions that encourage participants to reason through problems logically and systematically. These types of questions push participants to explore different methods of problem-solving, fostering a deeper grasp of each concept.
| Concept | Description |
|---|---|
| Number Theory | Study of integers and their properties, such as prime numbers and divisibility rules. |
| Geometry | Focus on spatial reasoning, including calculating areas, perimeters, and volumes. |
| Combinatorics | Exploration of counting principles, permutations, and combinations. |
| Algebra | Solving equations and working with algebraic expressions and variables. |
What to Expect from Future Competitions
As the competition continues to evolve, participants can anticipate a variety of exciting and challenging problems designed to test their reasoning abilities and mathematical knowledge. Future editions will likely feature a balance between traditional problem types and new, innovative questions that push the boundaries of standard problem-solving. Contestants can expect to face increasingly diverse topics and formats, aimed at enhancing their critical thinking and analytical skills.
Looking ahead, it’s expected that the focus will continue to be on fostering logical thinking, creativity, and problem-solving techniques. Participants will encounter a range of question types, from straightforward calculations to more complex scenarios requiring deep conceptual understanding. With each iteration, the challenge will grow, encouraging students to prepare not only by mastering core concepts but also by developing strategies for tackling unfamiliar problems.
Potential Changes in Format
While the core structure of the event will remain centered around individual problem-solving, future competitions may experiment with different formats. This could include:
- Collaborative Challenges – Opportunities for teams to solve problems together, fostering teamwork and communication.
- Interactive Problem-Solving – Incorporating technology to allow participants to solve problems using digital tools or simulations.
- Time-Limited Rounds – Introducing faster-paced rounds to test quick thinking under pressure.
With these possible changes, participants will be required to adapt their approach and sharpen their problem-solving techniques to succeed. The future promises to be an exciting and unpredictable journey for all involved.
Learning from Past Competition Results
Analyzing the outcomes of previous competitions provides valuable insights into the types of challenges that contestants face and the strategies that lead to success. By reflecting on past performances, participants can identify common themes, problem-solving approaches, and areas for improvement. This analysis not only helps in preparing for future events but also fosters a deeper understanding of the underlying mathematical principles.
Through the study of past results, it becomes clear that certain problem types are recurrent, offering clues about the most important areas to focus on during preparation. In addition, reviewing how successful participants approached complex problems can inspire new techniques and creative solutions. Learning from the mistakes and successes of others is an essential step in enhancing one’s skills and boosting confidence.
Key Takeaways from Previous Results
Some of the key takeaways from past competitions include:
- Consistency in Problem Types – Certain categories, such as logic puzzles and number theory, tend to appear frequently, making them critical areas to review.
- Time Management – Successful participants often excel at managing their time effectively, balancing quick solutions with more involved problem-solving approaches.
- Adaptability – The ability to stay flexible and adapt to unexpected question formats is crucial for success.
By learning from the experiences of past participants, aspiring competitors can sharpen their skills, avoid common pitfalls, and approach future challenges with greater confidence and strategy.