In the initial part of any mathematical curriculum, the focus is often on foundational principles that help students build a strong base for more complex topics. This section is designed to guide learners through essential problem-solving methods and core ideas that are crucial for mastering advanced mathematics. By working through the various exercises, you will begin to understand how to apply these concepts in different contexts and scenarios.
Structured exercises allow learners to approach problems step by step, ensuring that each solution is fully understood. The practice offered here helps clarify key operations and lays the groundwork for tackling more challenging problems. As you progress, you will notice how the concepts naturally connect, helping you to solve even more intricate equations and mathematical expressions.
Focused learning in this section offers a comprehensive approach, turning abstract ideas into clear, manageable tasks. By following through with the problems provided, you can reinforce your understanding and gradually gain the confidence needed to tackle more advanced topics in your studies.
Core Connections Algebra Chapter 1 Answers
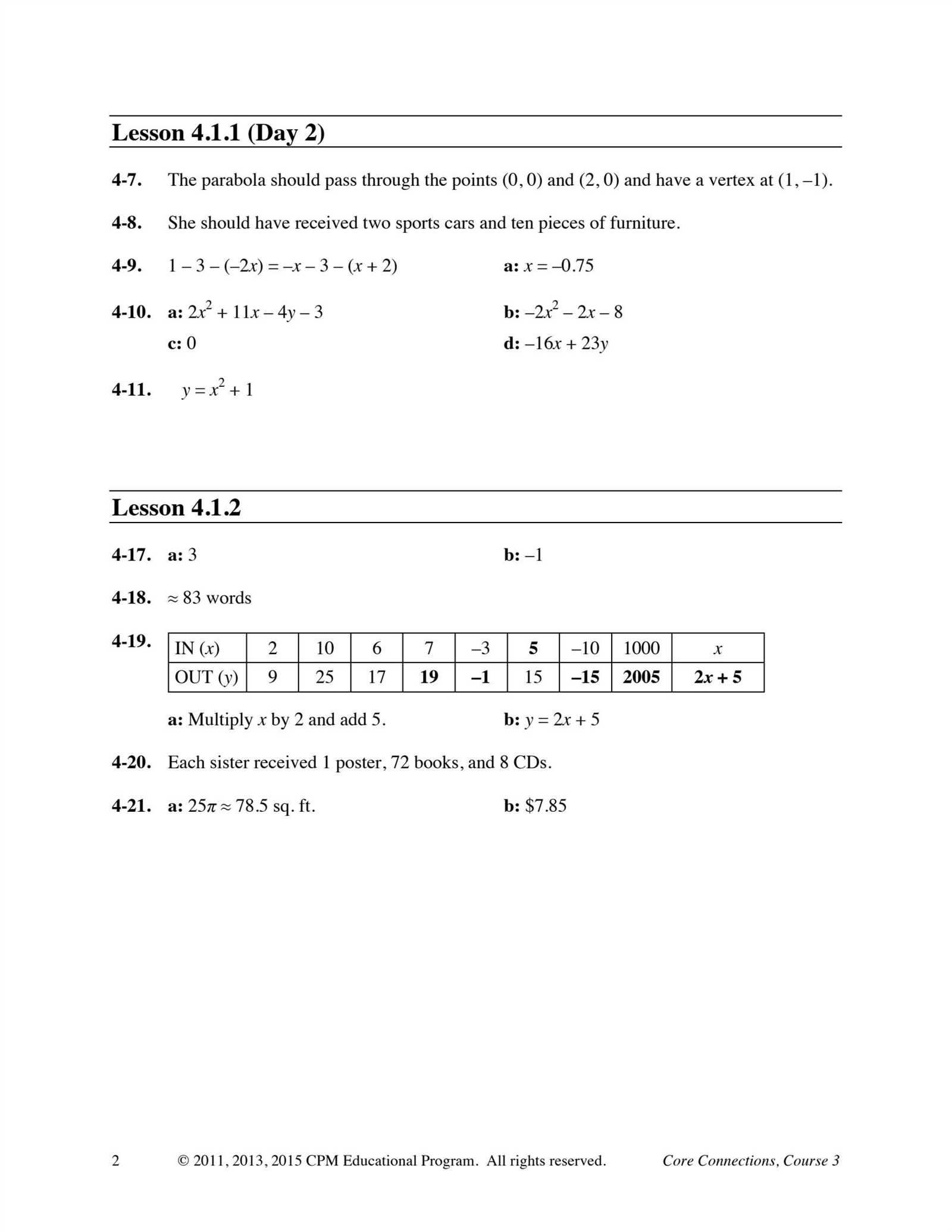
This section provides clear solutions and detailed explanations for key exercises designed to enhance understanding of foundational mathematical concepts. By working through these problems, students can develop a deeper grasp of essential techniques, improving their ability to solve various types of equations and expressions.
Each solution is carefully explained to highlight the thought process behind the steps, ensuring that learners not only arrive at the correct result but also understand why each step is necessary. This methodical approach encourages critical thinking and strengthens problem-solving skills, which are crucial for mastering more advanced material in mathematics.
Through consistent practice and review, students will build confidence in their ability to approach different problems. Whether revisiting familiar methods or learning new strategies, this section serves as a valuable resource for reinforcing core mathematical knowledge.
Understanding the Basics of Chapter 1
The beginning of any mathematical curriculum typically focuses on introducing essential concepts and skills that serve as the foundation for more complex topics. Mastery of these initial lessons is crucial, as they help students build a strong framework for tackling increasingly difficult problems.
In this section, we explore fundamental techniques that are critical to progressing in mathematics. These concepts are not only practical for solving basic problems but also act as building blocks for higher-level reasoning and analysis. By focusing on these basics, students develop the ability to approach a wide range of mathematical challenges with confidence.
- Learning to identify and apply mathematical principles effectively.
- Understanding how operations interact in various types of equations.
- Developing strategies for logical problem-solving and pattern recognition.
As learners progress through these exercises, they gain a deeper understanding of how to break down complex tasks into manageable steps. This gradual learning process fosters clarity and mastery of key skills needed for success in future studies.
Key Concepts Covered in Chapter 1
This section focuses on the essential principles that serve as the foundation for mathematical problem-solving. By understanding these core ideas, students can approach more complex topics with greater ease and confidence. The following concepts are introduced and explored in detail to provide a solid understanding of basic mathematical techniques.
Fundamental Operations and Their Interactions
One of the first concepts covered is how different operations–such as addition, subtraction, multiplication, and division–interact with each other. Understanding the relationship between these operations is crucial for solving equations and simplifying expressions efficiently.
Equation Building and Solving Techniques
Students are introduced to the process of constructing and solving equations. This concept emphasizes how to manipulate variables and constants in an equation to isolate and find unknown values. Mastering this skill is essential for progressing to more complex mathematical problems.
Key concepts explored:
- Understanding the order of operations (PEMDAS).
- Solving for unknowns in linear equations.
- Applying basic principles to simplify expressions.
These fundamental ideas lay the groundwork for mastering more advanced topics. As students continue to practice, they will become more proficient in using these concepts in a variety of mathematical contexts.
Step-by-Step Problem Solving Techniques
Effective problem solving requires a systematic approach that allows students to break down complex tasks into manageable steps. By following a structured method, learners can confidently tackle various mathematical challenges and reach solutions with clarity. The key to mastering this skill lies in developing a logical process that can be applied across different types of problems.
Step 1: Identify the Problem
The first step is to clearly understand the problem at hand. Carefully read through the given information, highlighting important values, variables, and relationships. This helps ensure you know exactly what is being asked and what information is provided.
Step 2: Plan the Approach
Next, choose the most suitable method or technique for solving the problem. This could involve identifying the type of equation or operation needed and deciding whether simplification, substitution, or another approach is required.
Step 3: Execute the Solution
With a plan in place, begin solving the problem step by step. Apply the chosen method carefully, ensuring each operation is performed correctly. Keep track of intermediate results to avoid errors.
Step 4: Review and Verify
After obtaining a solution, it’s essential to review each step to ensure no mistakes were made. Double-check calculations, and verify that the final answer satisfies the original problem.
By following these steps, students can develop a clear, methodical approach to solving problems, improving both accuracy and efficiency.
Common Mistakes to Avoid in Algebra
When tackling mathematical problems, especially those involving variables and equations, it’s easy to make mistakes that can lead to incorrect results. Being aware of common errors and understanding how to avoid them is crucial for developing strong problem-solving skills. This section highlights frequent pitfalls and offers tips on how to prevent them, ensuring a more accurate and efficient approach to solving problems.
Misinterpreting the Problem
One of the most common mistakes is misunderstanding what the problem is asking. It’s important to carefully read the question and identify key pieces of information before jumping to conclusions. Skipping this step can lead to incorrect assumptions and faulty solutions.
Neglecting the Order of Operations
Another frequent error is ignoring the proper sequence of operations. Failing to follow the correct order–such as applying parentheses, exponents, multiplication, division, addition, and subtraction (PEMDAS)–can result in wrong answers. Always double-check that operations are performed in the correct order to ensure accuracy.
Overlooking Negative Signs
Negative signs can be easily overlooked, especially when working with equations that involve multiple steps. It’s important to be cautious when adding or subtracting negative numbers, as a small mistake in handling negative values can lead to significant errors in the final solution.
Incorrectly Solving for Variables
When isolating variables in equations, students often make mistakes by not applying inverse operations correctly. Make sure to reverse the operations properly, whether it’s dividing by a coefficient or subtracting a constant, to solve for the unknown variable accurately.
Being mindful of these common errors will help you approach problems with greater confidence and precision, ultimately leading to better results in your studies.
How to Approach Algebraic Equations
When faced with equations involving variables, it’s important to follow a clear and logical approach to simplify and solve them. The goal is to isolate the unknown value by systematically applying operations and ensuring each step is carefully executed. By following a methodical process, students can approach equations confidently, reducing the risk of errors and improving their problem-solving skills.
The first step is to identify what is given and what needs to be solved for. Once this is clear, you can begin isolating the variable by using inverse operations. Whether it’s adding, subtracting, multiplying, or dividing, each step should move you closer to solving for the unknown. Always remember to perform the same operation on both sides of the equation to maintain balance.
| Step | Action | Example |
|---|---|---|
| Step 1 | Identify the equation and variable | 3x + 4 = 10 |
| Step 2 | Isolate the variable by using inverse operations | Subtract 4 from both sides: 3x = 6 |
| Step 3 | Simplify further | Divide both sides by 3: x = 2 |
By following this step-by-step process, you’ll be able to approach and solve equations more effectively, ensuring accuracy and building a solid foundation for more complex mathematical challenges.
Breaking Down Methodical Problem Solving
When approaching mathematical problems, having a structured method is key to successfully breaking down and solving complex equations. The method emphasizes a step-by-step process that guides learners from understanding the problem to applying the correct operations for finding solutions. This approach not only helps in solving equations but also in building a deeper understanding of underlying concepts.
Understanding the Problem
The first step in any problem-solving process is thoroughly understanding the problem’s requirements. Carefully read through the given information and identify the key elements, such as known values, variables, and what is being asked. By clearly defining the problem, students can avoid confusion and focus on the most relevant details.
Strategic Application of Operations
After analyzing the problem, the next step is to apply the correct operations to isolate the variable or unknown. This involves using inverse operations such as addition, subtraction, multiplication, or division in a logical sequence. Each operation should be applied consistently on both sides of the equation to maintain equality.
By breaking down problems in this structured manner, students can systematically approach and solve even the most complicated equations. This method encourages critical thinking and precision, leading to a more thorough understanding of mathematical problem-solving strategies.
Importance of Practice in Mathematics
Consistent practice is essential for mastering any skill, and mathematics is no exception. Regularly working through problems not only reinforces concepts but also helps students internalize techniques and develop confidence in their ability to solve increasingly complex tasks. By dedicating time to practice, learners improve their problem-solving speed and accuracy, making mathematical challenges easier to tackle.
Building Strong Foundations
One of the main benefits of regular practice is that it helps solidify foundational concepts. As students repeatedly work through various types of problems, they become more familiar with common patterns and techniques. This deep familiarity with basic principles lays the groundwork for more advanced topics, ensuring that students are well-equipped to handle more challenging material as they progress.
Improving Speed and Confidence
Through continuous practice, students not only gain a better understanding of the material but also improve their efficiency in solving problems. The more familiar a student becomes with different types of equations and problem-solving strategies, the quicker they can identify the best approach. This increased speed, combined with enhanced accuracy, helps build self-confidence, empowering students to tackle more complex problems without hesitation.
Ultimately, practicing regularly is the key to mastering mathematical concepts. The more time and effort put into solving problems, the more proficient and confident students become in their mathematical abilities.
Tips for Mastering Chapter 1 Topics
Mastering the foundational concepts in any subject requires both strategic preparation and consistent practice. The key to success in the first section of any mathematical curriculum is breaking down the material into smaller, manageable pieces and understanding each concept before moving forward. By following some key strategies, learners can strengthen their understanding and ensure they are well-prepared for the more advanced topics that follow.
Understand the Basics First
Before diving into complex problems, ensure that you have a solid grasp of the foundational concepts. Understanding the fundamental operations and their relationships is crucial, as more advanced topics often build on these basic skills. Take time to review each core idea, and don’t hesitate to revisit previous lessons if something is unclear. A strong foundation will make learning more advanced topics much easier.
Practice Regularly and Persistently
Consistency is key when it comes to mastering any mathematical topic. Regular practice helps reinforce what you’ve learned and allows you to identify areas where you may need further clarification. Set aside dedicated time each day or week to work through problems, and seek out additional exercises to test your understanding. The more you practice, the more confident and proficient you’ll become in applying the concepts effectively.
By following these tips, students can build a deeper understanding of the material and develop the problem-solving skills necessary to succeed. Focusing on the basics and committing to regular practice will help you master even the most challenging topics with confidence.
Solutions for Example Problems Explained
Understanding how to solve problems step-by-step is essential for mastering any mathematical concept. By breaking down example problems, learners can see the logical progression of operations and how each step leads to the final solution. This method helps build confidence and clarity in applying various techniques to similar problems in the future.
Step-by-Step Problem Breakdown
Let’s look at a sample problem and how to approach it methodically. Breaking the process into clear, manageable steps allows for easier understanding and application. Here’s how to solve a typical equation:
- Step 1: Identify the given equation and understand what is being asked. For example, if you have 2x + 5 = 11, your goal is to solve for x.
- Step 2: Isolate the variable. Subtract 5 from both sides of the equation: 2x = 6.
- Step 3: Solve for x by dividing both sides by 2: x = 3.
Common Mistakes to Watch Out For
While solving problems, it’s important to avoid common pitfalls that can lead to incorrect answers. Here are some mistakes to watch out for:
- Not following the order of operations: Always perform operations in the correct sequence–PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction).
- Forgetting to apply the same operation on both sides: Ensure any changes made to one side of the equation are also applied to the other side to maintain balance.
- Incorrect simplification: Double-check all steps to make sure each operation is carried out correctly, especially when simplifying terms.
By breaking down example problems into clear steps and avoiding common mistakes, students can strengthen their understanding of mathematical principles and improve their problem-solving skills.
Visualizing Math with Diagrams

One of the most effective ways to understand complex mathematical concepts is by visualizing them. Diagrams, graphs, and other visual tools can help illustrate abstract ideas, making them easier to grasp. These visual aids allow learners to see relationships between different elements, turning abstract equations into something more tangible and accessible.
For instance, representing an equation as a graph can provide insight into how changes in one variable affect another. Diagrams allow students to better understand geometric relationships, the behavior of functions, and how different operations interact within an equation.
Using Graphs to Understand Equations
Graphs are powerful tools that can visually represent relationships between variables. For example, a linear equation can be plotted on a graph to show its slope and intercepts, helping learners understand the equation’s behavior over time. By observing the line’s movement, students can gain a clearer sense of how the variables are related.
Diagramming for Problem Solving
In more complex problems, diagrams can help break down the elements of the equation and highlight key points. For example, a bar or pie chart can be used to represent proportions, while a number line can demonstrate how different values compare. By visualizing the problem in this way, students are able to quickly identify patterns and connections that might otherwise be difficult to see.
Using visual representations is an excellent way to enhance understanding and retention of mathematical concepts. By turning abstract equations into something visual, students can approach problems with greater clarity and confidence.
Exploring Real-Life Applications of Algebra
Mathematical concepts extend far beyond the classroom, with practical applications in everyday life and various professions. Understanding how mathematical principles work in real-world situations can make the subject more relevant and engaging. From budgeting and finance to engineering and technology, the skills learned in solving equations and analyzing variables are invaluable in many aspects of life.
Everyday Uses of Mathematical Concepts
Here are some common ways that mathematical techniques are applied in day-to-day activities:
- Budgeting and Financial Planning: Tracking income, expenses, and savings often involves equations to determine costs and forecast future financial situations.
- Home Improvement Projects: When renovating or building, measurements, ratios, and proportions help calculate areas, volumes, and costs of materials.
- Cooking and Recipes: Adjusting recipes for different serving sizes requires understanding ratios and proportions to scale ingredients accordingly.
- Shopping Discounts: Calculating discounts, sales tax, and final prices often requires applying simple mathematical formulas.
Career-Related Applications
In many fields, the ability to solve equations and work with variables is essential. Below are a few professions where mathematical principles are commonly applied:
- Engineering: Engineers use complex formulas to design structures, calculate forces, and optimize systems.
- Data Science: Analysts and data scientists use mathematical modeling to interpret data and make predictions based on trends.
- Architecture: Architects apply geometry and measurement to design and plan buildings, ensuring structures are safe and efficient.
- Medicine: Medical researchers use statistical analysis to understand trends in health data and create treatment plans.
As these examples show, the ability to solve mathematical problems opens up a wide range of practical applications. Whether you’re planning a budget, designing a building, or analyzing data, the skills developed in mathematics are invaluable tools that can be applied to a variety of real-world scenarios.
How to Review Chapter 1 Effectively
Reviewing important concepts effectively is key to mastering any subject. When revisiting the initial topics in any math course, it’s essential to focus on understanding the core principles, practicing problem-solving techniques, and reinforcing your grasp on key operations. Here are some strategies for reviewing that can help you improve retention and build confidence.
Step-by-Step Review Process
A structured approach to reviewing ensures you don’t overlook any key areas. Follow this simple process to ensure a comprehensive review:
| Step | Action |
|---|---|
| 1 | Start by reading through your notes or textbook summaries. Pay special attention to definitions, formulas, and examples. |
| 2 | Practice a variety of problems, from easy to challenging. Focus on applying the concepts to different scenarios. |
| 3 | Review common mistakes and try to understand why errors occurred. This will help you avoid them in the future. |
| 4 | Use visual aids like diagrams and graphs to further clarify complex ideas. Visual learning can reinforce your understanding. |
| 5 | Test yourself with practice quizzes or review questions to assess how well you’ve mastered the material. |
Active Engagement During Review
Simply reading through the material isn’t enough. Active engagement helps deepen your understanding. Here are some active review strategies:
- Teach the Material: Explaining concepts to a peer or even to yourself reinforces what you’ve learned.
- Make Flashcards: Use flashcards to memorize key formulas, terms, or steps in solving problems.
- Work in Small Intervals: Break your review time into focused intervals, followed by short breaks to avoid burnout.
- Set Goals: Set specific goals for each study session, such as mastering a particular concept or solving a set number of problems.
By following these strategies, you can efficiently review the material and solidify your understanding of the essential topics covered. Consistent practice and reflection on past mistakes will pave the way for continued progress and mastery.
Understanding Key Formulas and Theorems
Grasping the essential formulas and theorems is a crucial aspect of mastering mathematical concepts. These foundational tools serve as the building blocks for solving a wide range of problems. To navigate through complex calculations and equations, it’s important to not only memorize these formulas but also understand their purpose and application in different contexts.
In any mathematical discipline, formulas are used to simplify and standardize processes. By applying the right formula at the right time, one can solve problems more efficiently and with greater accuracy. Similarly, theorems provide a structured framework for reasoning, helping students to understand how different principles relate to each other and why certain steps in problem-solving are necessary.
Focusing on the key formulas and theorems within a given subject can greatly enhance your ability to tackle complex problems. Whether you’re working with expressions, equations, or systems, understanding the underlying principles behind the formulas you use allows you to apply them in a more flexible and intuitive way.
Preparing for Assessments in Algebra
Effective preparation for evaluations in mathematics involves more than just reviewing notes. It’s about building a deep understanding of concepts and practicing regularly to enhance problem-solving skills. Preparing for assessments requires a strategic approach, where time is spent reinforcing both fundamental ideas and advanced techniques, ensuring readiness for a variety of questions and challenges.
Start by reviewing key topics that are frequently tested, focusing on areas where you feel least confident. Take the time to understand the reasoning behind each step of a problem rather than just memorizing methods. This will make it easier to tackle unfamiliar problems during the assessment. Practice is essential; solving different types of problems will help you become comfortable with the material and improve your ability to apply what you’ve learned in various contexts.
Additionally, practice with past assessments or sample questions. This not only helps you familiarize yourself with the format but also allows you to gauge the level of difficulty you can expect. Time yourself during practice sessions to simulate real exam conditions and work on managing time efficiently. Finally, don’t forget to review solutions to practice problems, paying close attention to any mistakes to prevent them from happening again in the future.
Online Resources to Support Learning
With the wealth of digital tools available today, students can access a variety of online resources that enhance understanding and improve problem-solving skills. These platforms offer a wide range of learning materials, interactive exercises, and video tutorials to support students at all levels. Leveraging these online tools can make mastering mathematical concepts easier and more engaging.
Here are some effective online resources to support your learning:
- Interactive Tutorials and Courses: Websites like Khan Academy and Coursera offer free courses and practice exercises, covering everything from basic concepts to advanced techniques.
- Practice Problem Sets: Platforms such as IXL and Brilliant provide extensive problem sets with step-by-step solutions to reinforce learning and test comprehension.
- Video Explanations: YouTube channels like PatrickJMT and 3Blue1Brown offer clear, concise video tutorials that explain complex concepts in an easy-to-understand way.
- Online Forums and Study Groups: Websites like Stack Exchange and Reddit feature communities where students can ask questions, discuss problems, and get help from peers and experts.
- Math Solvers: Tools like WolframAlpha and Symbolab allow students to solve equations, check solutions, and explore various methods for problem-solving.
By regularly using these resources, students can reinforce their understanding, gain new insights, and improve their confidence in applying mathematical concepts to different scenarios. Combining online support with traditional study methods leads to a more comprehensive and effective learning experience.
How to Use Chapter 1 Answers for Study
Utilizing solutions effectively can significantly enhance your study routine, helping you understand concepts more deeply and identify areas that require further attention. Reviewing provided solutions allows you to compare your own approach to the correct methods, strengthening problem-solving skills and boosting confidence in applying mathematical concepts.
Here’s how you can make the most of the solutions when preparing for exams or assessments:
| Step | Description |
|---|---|
| Compare Solutions | Start by solving the problems on your own. Afterward, compare your approach with the provided solutions to check for any errors or areas of improvement. This helps in identifying common mistakes and understanding the correct methodology. |
| Analyze the Steps | Break down each solution into individual steps. Focus on how each part of the problem is solved and understand why the approach works. This will deepen your conceptual understanding and improve your ability to tackle similar problems. |
| Revisit Problem Areas | If you find that certain types of problems are consistently difficult for you, use the solutions as a reference to revisit and practice similar problems until you gain more confidence. |
| Understand Common Mistakes | Check for common errors in your approach. By recognizing these mistakes, you can focus on avoiding them in the future, improving both your speed and accuracy. |
Using solutions in this way turns them from just an answer key into a valuable learning tool that helps you refine your techniques, improve your problem-solving process, and ultimately become more proficient in applying what you’ve learned.
Improving Your Mathematical Skills Over Time
Improving your proficiency in solving mathematical problems requires a consistent and focused effort over time. The process involves understanding foundational concepts, practicing regularly, and developing strategies to tackle complex challenges. Building these skills progressively helps in mastering techniques and gaining the confidence to apply them effectively.
Key Strategies for Continuous Improvement
- Daily Practice: Make math a part of your daily routine. Regular practice, even for just 15-20 minutes, keeps concepts fresh in your mind and ensures steady progress.
- Focus on Understanding: Instead of memorizing formulas or methods, aim to understand why certain approaches work. This deeper comprehension will allow you to adapt your knowledge to a variety of problems.
- Work on Weak Areas: Identify specific areas where you struggle and devote extra time to those topics. By focusing on your weaknesses, you can turn them into strengths.
Tracking Your Progress
- Keep a Practice Journal: Track the problems you’ve worked on and the methods you’ve used. This will help you monitor your progress and highlight areas that need more focus.
- Review Mistakes: Mistakes are an essential part of the learning process. Review errors and analyze where you went wrong to avoid repeating them in the future.
- Set Achievable Goals: Set small, manageable goals for each study session. This could include mastering a specific concept or solving a set number of problems. Small victories build momentum.
As you continue practicing and refining your skills, you will begin to see improvements not just in your ability to solve problems but in your overall confidence with the material. The more effort you put into refining your skills, the easier it will become to tackle increasingly challenging problems with ease.