Preparing for academic evaluations can be a daunting task, but with the right approach, achieving top performance becomes much more attainable. Whether you’re tackling theoretical concepts or solving practical problems, understanding key principles is crucial. This section offers guidance on how to approach these challenges and maximize your potential in any subject.
Effective preparation involves more than just memorizing formulas or facts. It’s about developing problem-solving strategies, enhancing your critical thinking, and learning how to apply knowledge under pressure. By focusing on the essential skills and techniques, you’ll gain the confidence needed to navigate any challenge.
With careful planning and consistent practice, you can improve your ability to recall information quickly and apply it accurately when required. Let’s explore the best methods for achieving success in your assessments and mastering the material.
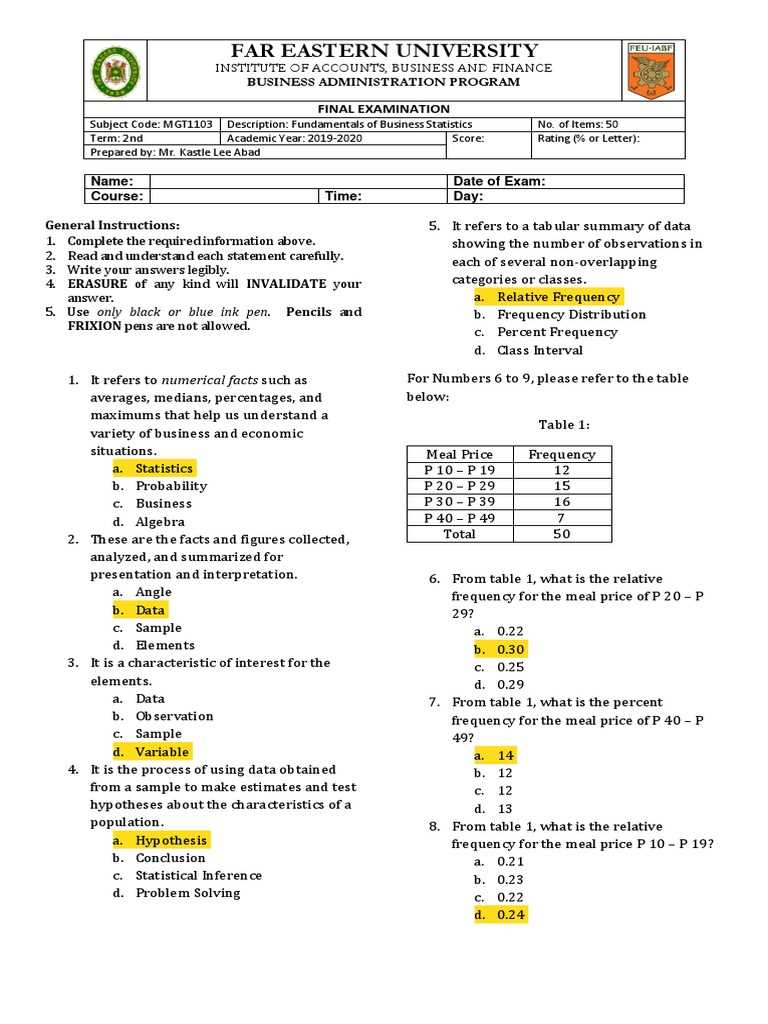
Statistics Exam Answers for Success
Achieving success in any assessment requires a strategic approach that goes beyond simply memorizing content. It’s about understanding the material thoroughly, practicing problem-solving skills, and staying calm under pressure. By developing a methodical study routine, you can ensure you’re prepared to tackle challenges with confidence.
Mastering Key Concepts
To succeed, focus on mastering the core concepts. It’s essential to understand the underlying principles rather than just relying on memorization. Once you grasp the fundamental ideas, you’ll be able to apply them effectively to various problems. Spend time working through examples and practice questions to deepen your understanding.
Time Management and Strategy
Effective time management is crucial for performing well. Allocate specific time blocks for different sections and practice under timed conditions. This not only helps you stay on track but also builds familiarity with the pressure of completing tasks within a limited timeframe. A well-thought-out approach ensures that you maximize every minute of your preparation.
How to Prepare for Your Statistics Exam
Preparing for any assessment requires a well-structured approach that combines review, practice, and a clear understanding of key concepts. A successful preparation plan focuses not only on studying but also on developing effective strategies to solve problems and manage time during the evaluation.
Start by reviewing the core material covered in your course. Break it down into manageable sections and focus on the most challenging topics. It’s essential to identify areas where you feel less confident and dedicate more time to those. Use a variety of resources, such as textbooks, online tutorials, and practice problems, to reinforce your understanding.
Incorporate active learning techniques like summarizing information, teaching others, or creating mind maps. These methods help reinforce knowledge and improve recall. Additionally, practicing problems under timed conditions can simulate the real environment and help you build the speed and accuracy necessary to excel.
Common Statistics Exam Topics You Should Know
Understanding the most frequently covered topics is key to excelling in any assessment. Focusing on these core areas will ensure that you are well-prepared to tackle a wide range of questions. Mastering these essential subjects will also improve your ability to apply concepts in different scenarios.
Here are some of the fundamental topics that often appear in assessments:
- Descriptive Measures: Learn how to calculate and interpret mean, median, mode, range, and standard deviation.
- Probability: Understand probability rules, distributions, and how to calculate probabilities for various events.
- Hypothesis Testing: Know the process of formulating null and alternative hypotheses, conducting tests, and interpreting p-values.
- Correlation and Regression: Learn the basics of correlation coefficients and regression analysis to examine relationships between variables.
- Sampling Methods: Familiarize yourself with different sampling techniques, such as random and stratified sampling, and their applications.
- Confidence Intervals: Understand how to construct and interpret confidence intervals for population parameters.
- Analysis of Variance (ANOVA): Study the concept of comparing means across multiple groups to determine if there are significant differences.
These topics form the foundation of your preparation and will help you tackle a variety of questions with confidence and precision.
Tips for Solving Statistics Problems Effectively
Approaching problems methodically is key to solving them efficiently and accurately. Whether you’re dealing with complex calculations or interpreting data, breaking down each problem into manageable steps will help you stay focused and organized. Here are some strategies to improve your problem-solving skills.
First, always read the problem carefully. Ensure you understand what is being asked before attempting a solution. Identify the key information, such as given values, formulas, and what you need to find. It’s easy to overlook important details, so take your time to review the problem thoroughly.
Next, choose the appropriate method or formula based on the problem type. Whether it’s calculating a mean, testing a hypothesis, or creating a model, knowing which technique to apply will save you time. If you’re unsure, revisit your notes or practice questions to remind yourself of the relevant methods.
Finally, double-check your work. Ensure all calculations are correct and that you have answered the question fully. Mistakes can easily be made, especially when working with multiple steps. Verifying your results not only helps catch errors but also builds confidence in your solutions.
Key Formulas to Memorize for Statistics
To tackle mathematical assessments effectively, it’s essential to commit certain formulas to memory. These key equations will not only help you solve problems quickly but also deepen your understanding of the underlying concepts. Familiarizing yourself with these formulas ensures you are well-equipped for various problem types.
Essential Formulas for Problem Solving
Here are some fundamental formulas that frequently appear in evaluations:
| Formula | Usage |
|---|---|
| Mean = Σx / n | Used to calculate the average of a dataset. |
| Standard Deviation = √(Σ(x – μ)² / n) | Measures the dispersion of a dataset from its mean. |
| Variance = σ² = Σ(x – μ)² / n | Quantifies how spread out the values in a dataset are. |
| Correlation Coefficient = Σ(xy) – (Σx)(Σy) / √[nΣx² – (Σx)²][nΣy² – (Σy)²] | Measures the strength and direction of a linear relationship between two variables. |
| Linear Regression = y = mx + b | Used to predict the value of a variable based on the value of another variable. |
Advanced Formulas for Deeper Analysis
As you advance, you will encounter more complex formulas that are crucial for analyzing data. Here are a few that you should know:
| Formula | Usage |
|---|---|
| Confidence Interval = x̄ ± Z(σ/√n) | Used to estimate a range of values that likely contains the population mean. |
| t-Test = (x̄ – μ) / (s/√n) | Tests whether the sample mean differs significantly from the population mean. |
| Chi-Square Test = Σ[(O – E)² / E] | Used to assess the goodness of fit between observed and expected frequencies. |
By regularly practicing with these formulas, you’ll enhance your ability to solve problems efficiently and accurately. Keep them handy for reference as you prepare and apply them as needed during assessments.
Common Mistakes in Statistics Exams and How to Avoid Them
In any type of academic assessment, it’s easy to make small errors that can lead to larger mistakes. These oversights often occur when under time pressure or when concepts are misunderstood. Recognizing the common pitfalls ahead of time allows you to approach problems more carefully and avoid these mistakes.
One frequent mistake is misinterpreting the question. Often, students jump into solving the problem without fully understanding what is being asked. Carefully read the question several times to ensure you know exactly what information is needed and what the question is aiming for.
Another common error is using the wrong formula. With many different formulas to memorize, it’s easy to select the wrong one or apply it incorrectly. Double-check which formula is appropriate for each situation. If you’re unsure, take a moment to review your notes or the problem’s requirements before proceeding.
Additionally, miscalculating values due to simple arithmetic errors can derail your results. Take your time with calculations and consider reviewing each step as you go. If possible, use a calculator or spreadsheet software to avoid basic math errors that could cost you valuable points.
Finally, a common mistake is failing to check your work. Even if you’re confident in your answers, it’s always a good idea to review your solutions before submitting. Double-checking your work can help catch any overlooked mistakes and improve the accuracy of your final result.
Understanding Hypothesis Testing in Statistics

Hypothesis testing is a fundamental concept used to draw conclusions about populations based on sample data. This method allows you to make inferences and determine whether there is enough evidence to support a particular claim. By using statistical tests, you can assess whether your observations are consistent with a specific hypothesis or if they are due to random chance.
The Process of Hypothesis Testing
The process begins with formulating two competing hypotheses: the null hypothesis (H₀) and the alternative hypothesis (H₁). The null hypothesis typically represents a statement of no effect or no difference, while the alternative hypothesis suggests a potential effect or difference. Your goal is to test the null hypothesis to determine whether there is enough evidence to reject it in favor of the alternative.
The next step is to choose an appropriate statistical test, based on the type of data you have and the hypothesis you’re testing. For example, if you’re comparing means, a t-test might be used; for proportions, a chi-square test may be more suitable. Once the test is selected, you compute the test statistic and compare it to a critical value to decide whether to reject the null hypothesis.
Interpreting Results
After conducting the test, you’ll obtain a p-value, which indicates the probability of observing your data given that the null hypothesis is true. A smaller p-value suggests stronger evidence against the null hypothesis. If the p-value is less than your chosen significance level (usually 0.05), you reject the null hypothesis. However, if the p-value is higher, you fail to reject the null hypothesis, meaning there isn’t sufficient evidence to support the alternative claim.
Understanding how to conduct and interpret hypothesis testing is crucial for making informed decisions based on data. Mastering this technique will improve your ability to analyze and evaluate data accurately in any situation.
How to Manage Time During Your Statistics Exam
Effective time management is crucial for success in any assessment. With a limited amount of time to complete multiple questions, it’s essential to approach the test strategically. By organizing your time well, you can ensure that you have enough opportunity to answer each question thoroughly without rushing at the end.
Plan Your Time Before Starting
Before you begin, take a few minutes to review the entire test and assess the number of questions and their difficulty. Allocate time for each section based on its weight and complexity. For example, if a particular problem type is more challenging, plan to spend more time on it. This strategy ensures that you don’t spend too much time on any one question, leaving you with insufficient time for others.
It’s also helpful to set time checkpoints during the test. For instance, aim to finish the first section within a specific time frame and check your progress every 15 or 20 minutes. This will help you stay on track and avoid spending too long on difficult questions.
Use the Process of Elimination
If you encounter a question that seems time-consuming or particularly tricky, don’t get stuck. Move on to the next question and return to the challenging one later if you have time. This keeps you from wasting precious minutes and helps maintain a steady pace throughout the test. Additionally, using the process of elimination can speed up decision-making on multiple-choice questions, allowing you to focus on answering more questions accurately.
By following these time management strategies, you’ll be able to tackle each problem efficiently, stay calm, and maximize your performance during the assessment.
Best Study Resources for Statistics Students
To excel in any field that involves data analysis, having the right study materials is essential. The right resources can provide clarity on complex concepts and help reinforce key principles. Whether you’re looking for textbooks, online courses, or interactive tools, the following resources can be invaluable in your learning journey.
Top Books for In-Depth Understanding
- “The Art of Data Science” – A great resource for understanding the principles of data analysis, this book breaks down complex ideas into simple, digestible parts.
- “Practical Regression and Anova using R” – This text focuses on applying statistical models using R programming and provides practical insights into real-world data.
- “Introductory Applied Biostatistics” – This book is excellent for beginners looking to understand the basics of data analysis and statistics in health-related fields.
Online Courses and Tutorials
- Khan Academy – Offers free, comprehensive lessons on various topics related to data analysis, from basic concepts to more advanced techniques.
- Coursera: Data Science Specialization – A popular course from Johns Hopkins University, which covers both theory and practice of data science.
- edX: Introduction to Data Science – A beginner-friendly course offering insights into data science with practical applications using Python.
Interactive Learning Platforms
- Wolfram Alpha – A computational knowledge engine that can be used to perform calculations, analyze data, and provide step-by-step solutions for statistical problems.
- Desmos – An interactive graphing calculator that is perfect for visualizing and solving statistical equations.
- RStudio – An integrated development environment (IDE) for R programming, useful for data analysis and statistical modeling.
By utilizing these resources, students can build a solid foundation in data analysis and improve their problem-solving abilities. With a combination of theory, practice, and hands-on tools, success is within reach for any learner in this field.
Statistics Exam Strategies for Maximum Marks
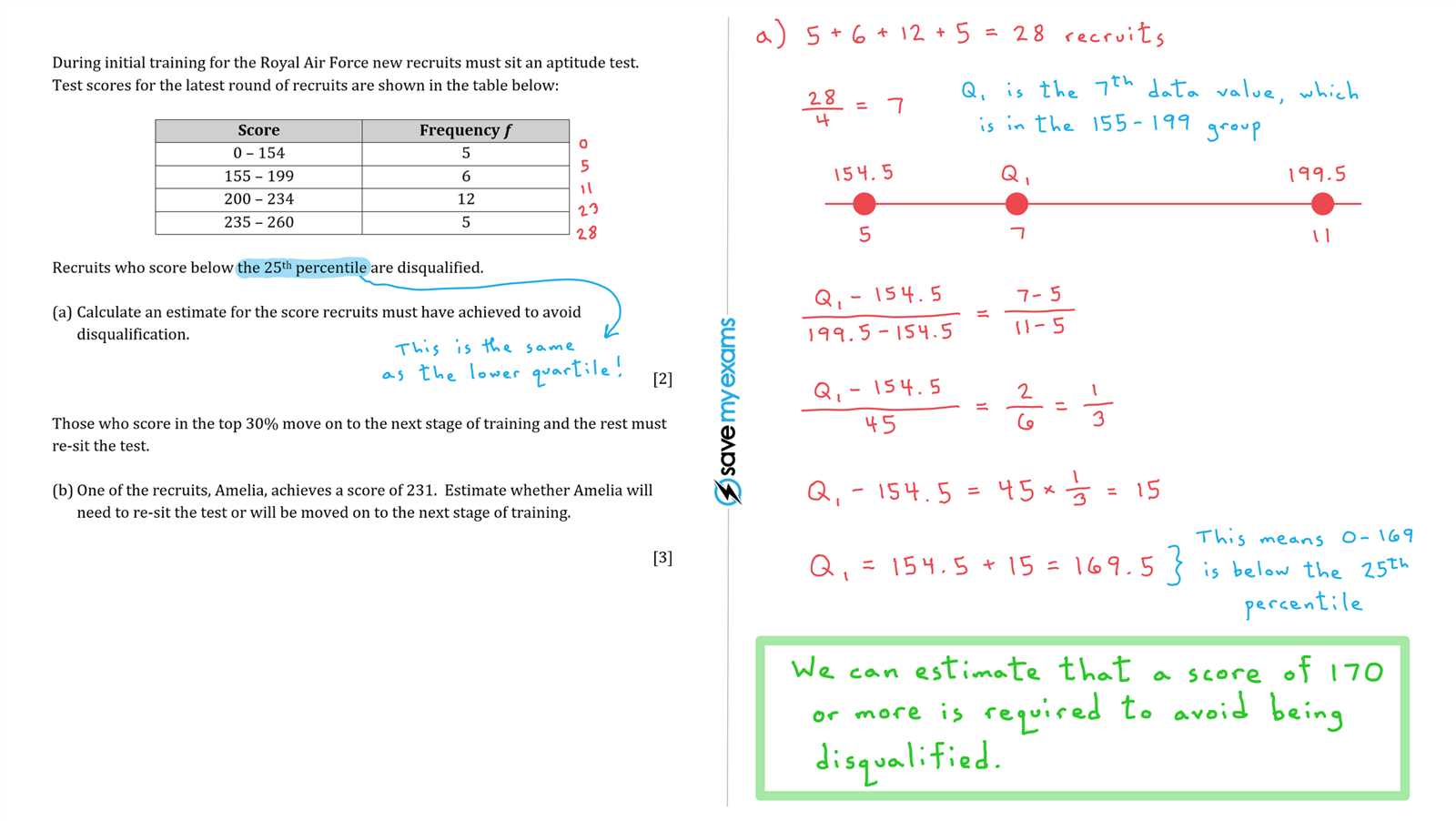
Achieving top marks in any assessment requires a combination of knowledge, strategy, and time management. Understanding key topics, practicing efficiently, and knowing how to approach each question can make a significant difference. By applying the following strategies, you can maximize your potential for success during your test.
Preparation Tips
- Understand Key Concepts – Ensure you have a solid grasp of the fundamental principles and formulas. This will allow you to tackle problems confidently and apply the right methods quickly.
- Practice with Past Papers – Reviewing previous assessments will give you a clear idea of the types of questions likely to appear. It also helps you get used to the format and time constraints.
- Make a Revision Schedule – Break down your study time into manageable chunks and focus on one topic at a time. Spaced repetition is an effective way to reinforce concepts and avoid cramming.
During the Test
- Read Questions Carefully – Before diving into solving, carefully read each question to understand exactly what is being asked. Make sure you don’t miss important details.
- Start with Easier Questions – Begin with the questions you find easiest to answer. This will build confidence and give you more time for the harder questions later.
- Show Your Work – For each problem, clearly show your calculations and reasoning. This not only helps you keep track of your process but also may earn partial credit in case of errors.
- Manage Your Time – Allocate time for each section or question and stick to it. If you’re stuck on a question, move on and return to it later if you have time remaining.
- Double-Check Your Answers – If you have time at the end, review your answers carefully. Look for simple calculation mistakes or missed steps that could cost you valuable points.
By integrating these strategies into your study and test-taking approach, you can significantly improve your performance and boost your chances of achieving maximum marks. With a focused mindset, preparation, and time management, success is well within reach.
How to Interpret Statistical Data in Exams
Understanding how to analyze and draw conclusions from data is a crucial skill in any test involving quantitative information. Being able to extract meaningful insights from raw data and present them effectively can make the difference between a good and great result. This section will guide you through key steps for interpreting data during your assessment, ensuring that you can approach these questions confidently.
Identify the Key Information
The first step in interpreting data is to carefully examine the provided information. Look for important variables and trends that are immediately obvious. Pay attention to labels, units, and scales, as these can help you understand the context of the data. For example, if you are given a graph, check the axes for scales and units to ensure that you interpret the values correctly. Understanding what each piece of data represents is crucial to solving the problem accurately.
Analyze Relationships and Patterns
Once you’ve identified the key elements, focus on any patterns, relationships, or outliers in the data. Look for correlations–do the numbers increase or decrease together? Are there any unusual values that stand out? These observations can provide clues to help solve the problem. For instance, if you are given a set of data points and a question asks you to identify a trend, spotting whether values are generally rising or falling will guide your interpretation.
Finally, be sure to use the appropriate statistical methods or formulas to calculate any necessary values. Whether it’s finding averages, medians, or variances, using the right approach to analyze the data will help you make the correct conclusion.
Preparing for Statistics Multiple Choice Questions
Multiple choice questions often test your ability to recognize the correct answer quickly while eliminating incorrect options. This type of question format can be challenging if you are not prepared, but with the right approach, you can improve your chances of success. Effective preparation involves not just memorizing facts, but developing strategies for selecting the correct answer and avoiding common pitfalls.
Start by familiarizing yourself with the types of questions commonly asked. Review past assessments or practice tests to understand the format and topics that are likely to appear. The more exposure you have to the question styles, the easier it will be to spot familiar patterns. When faced with multiple choices, it’s important to read each option carefully and rule out those that are obviously incorrect. Often, there will be one or two options that are clearly wrong, so narrowing down the choices increases your chances of selecting the right answer.
Additionally, make sure to focus on the key concepts and formulas. If a question involves calculations or specific methodologies, ensure you understand how to apply them in different contexts. Knowing the logic behind the correct answers will make it easier to eliminate distractors. With consistent practice and thoughtful preparation, you’ll be able to approach multiple-choice questions with confidence and improve your performance on test day.
What to Do If You Get Stuck on a Question
Encountering a difficult question during an assessment is a common challenge, but it doesn’t have to derail your progress. Knowing how to handle these moments can help you stay calm and continue moving forward. Instead of panicking or wasting time on a single problem, there are several strategies you can use to manage the situation effectively.
Steps to Take When Stuck
- Skip and Return Later – If you’re unable to make progress on a question, move on to the next one. Sometimes a fresh perspective can help, and you may even find that you can solve the difficult question after answering others.
- Break the Question Down – Reread the question carefully and try to break it into smaller parts. Identify any key information or clues you may have missed the first time. Simplifying the problem can make it easier to solve.
- Eliminate Wrong Answers – If you’re dealing with multiple choice or similar formats, use the process of elimination. Cross out the answers that are clearly incorrect, and focus on the remaining options.
Stay Calm and Focused
- Take a Deep Breath – Stress can cloud your judgment, so take a moment to relax. A calm mind is better able to think clearly and logically.
- Don’t Overthink – Sometimes overthinking a question can lead to confusion. Trust your instincts and choose the answer that feels most reasonable.
- Manage Your Time – Keep track of time and ensure you’re not spending too long on any one question. If you’re stuck, it’s more beneficial to move on and return to it with a fresh mindset.
By following these steps, you can approach tough questions with confidence and avoid wasting valuable time. Staying calm, organized, and focused will help you make the most of your time and perform at your best.
Understanding Probability in Your Statistics Exam
Understanding probability is essential when facing questions that involve predicting outcomes or analyzing random events. It is a core concept that often appears in various forms and can seem complex at first glance. However, once you grasp the fundamental principles, you will find that solving these problems becomes more manageable and systematic. Probability problems are typically based on real-life situations and require you to calculate the likelihood of different events occurring.
When approaching probability questions, it’s important to know how to break down the problem into smaller, more manageable parts. You can use basic rules such as the addition rule and multiplication rule to calculate the chances of events happening together or separately. Additionally, understanding the difference between independent and dependent events will help you select the right formula for the situation at hand.
Here’s a quick overview of some important probability formulas and concepts that you should keep in mind:
| Concept | Formula | Explanation |
|---|---|---|
| Simple Probability | P(A) = Number of favorable outcomes / Total number of possible outcomes | This is the basic formula for calculating the probability of a single event occurring. |
| Addition Rule | P(A or B) = P(A) + P(B) – P(A and B) | Used when calculating the probability of either event A or event B occurring. |
| Multiplication Rule | P(A and B) = P(A) * P(B | A) | This is used for calculating the probability of two events happening together, where one event may depend on the other. |
By mastering these key concepts and practicing a variety of problems, you’ll be better equipped to handle probability questions with confidence. Whether you’re dealing with basic events or more complex probability distributions, a solid understanding will significantly improve your ability to succeed.
How to Check Your Work in Statistics Exams

Verifying your calculations and conclusions is a crucial step when preparing for any assessment that involves data interpretation and problem-solving. Often, students rush through their solutions, missing small mistakes that can significantly affect their final results. To avoid this, developing a systematic approach to reviewing your work is essential for ensuring accuracy and maximizing your performance.
Before you submit your responses, take the time to go back over each problem carefully. Start by double-checking your calculations to ensure that all arithmetic operations, such as addition, subtraction, multiplication, and division, were carried out correctly. Pay attention to signs and units, as small errors here can lead to big discrepancies in the final answers.
Step-by-Step Verification
Here are some strategies to help you review your work effectively:
- Revisit Your Formulas: Ensure that you used the correct formula for each problem. Double-check that all variables were properly accounted for and substituted.
- Check Your Work for Logical Consistency: Ask yourself if your results make sense. For example, if you’re calculating a probability, it should always be between 0 and 1. If it’s outside that range, there’s likely a mistake somewhere.
- Recalculate Key Steps: Sometimes, performing a recalculation from scratch can help you spot errors. This is especially helpful for complex calculations or multi-step problems.
Using Alternative Methods
Whenever possible, try to use an alternative approach to confirm your results. For example, if you’re solving a problem involving averages, check your calculations by adding the data points together and dividing by the number of entries. Additionally, for more advanced problems, consider using different methods or formulas to solve the same question. This not only reinforces your understanding but can also help you identify where things went wrong if your results don’t match up.
By incorporating these checking methods into your study habits and exam routine, you will improve your accuracy and boost your confidence in handling challenging tasks. Always remember that reviewing your work is just as important as the initial solution process itself.
How to Use Graphs and Charts in Statistics
Visual representations are powerful tools for interpreting and presenting data. When faced with complex datasets, graphs and charts can help simplify the information, making it easier to spot trends, relationships, and outliers. Whether you are analyzing patterns or explaining results, these visual aids provide clarity and enhance understanding.
To make the most of graphs and charts, it’s important to select the right type for the data at hand. Each chart type serves a specific purpose, and knowing when to use one over the other can make a significant difference in the quality of your analysis.
For example, bar charts are ideal for comparing quantities across different categories, while line graphs are more effective for showing changes over time. Pie charts can be useful for illustrating proportions, and histograms allow you to understand the distribution of data. Understanding the strengths and limitations of each type is essential to communicating your findings accurately.
When working with these visual aids, always ensure that the axes are labeled correctly, units are included, and the data points are accurate. Providing a clear legend or key will also help viewers understand the data representation, especially when multiple variables are involved. Additionally, it’s important to avoid clutter and ensure that the graph remains easy to interpret.
By mastering the use of graphs and charts, you will be able to present your findings in a more digestible format, improving both your analysis and the communication of your results.
Post-Exam Tips for Reviewing Statistics Answers
Once you’ve completed your test, it’s essential to take some time to thoroughly review your work. Reflecting on your responses allows you to identify areas of strength and areas that may need improvement. Reviewing your performance after the test is not only helpful for learning from mistakes, but it also enhances your understanding of key concepts and boosts your confidence for future assessments.
The first step is to carefully go through each question, checking that your calculations and reasoning are accurate. Pay attention to any parts where you might have rushed or missed important steps. Even small errors in calculations can sometimes lead to incorrect results, so it’s crucial to verify every detail.
Check Your Methodology
When reviewing, focus on your approach to each question. Did you follow the correct methods? Were the formulas you used appropriate for the problem? If you made mistakes in the process, try to pinpoint why and how you could avoid them next time. Understanding the reasoning behind your answers helps to strengthen your problem-solving skills for the future.
Learn from Mistakes
Look for recurring mistakes in your work. Were there specific types of problems that gave you trouble? Were there patterns in how you approached the questions? Identifying these trends will help you target areas for improvement and focus your preparation on the concepts or methods that you struggled with. Remember, mistakes are an opportunity for growth, and each error is a lesson learned.
After reviewing, consider discussing your test with a peer or teacher to get additional insights. Sharing your thought process can reveal new perspectives and provide clarity on areas you might have misunderstood.