Preparing for advanced topics in physics requires a deep understanding of fundamental principles and the ability to apply them to complex situations. This section is dedicated to exploring key concepts, helping students refine their problem-solving skills and gain confidence in tackling challenging scenarios. The focus is on providing a thorough analysis of core theories and their real-world implications.
By reviewing a variety of problems, from foundational to more intricate topics, students will learn how to approach different types of challenges with clarity and precision. Emphasis is placed on breaking down complicated ideas into manageable steps, ensuring that each concept is grasped fully. In-depth exploration of theories and practical applications will offer a comprehensive understanding needed for academic success.
Advanced Physics Problems and Solutions
Mastering the core principles of advanced physical theories requires more than just memorization of formulas. It involves understanding the fundamental concepts, applying them effectively, and being able to approach intricate scenarios with confidence. This section delves into some of the most common challenges students face, providing structured solutions and explanations to enhance their grasp of key topics.
Key Topics to Focus On
- Wave-particle duality and its implications
- Understanding energy quantization in various systems
- Applying the uncertainty principle in real-world examples
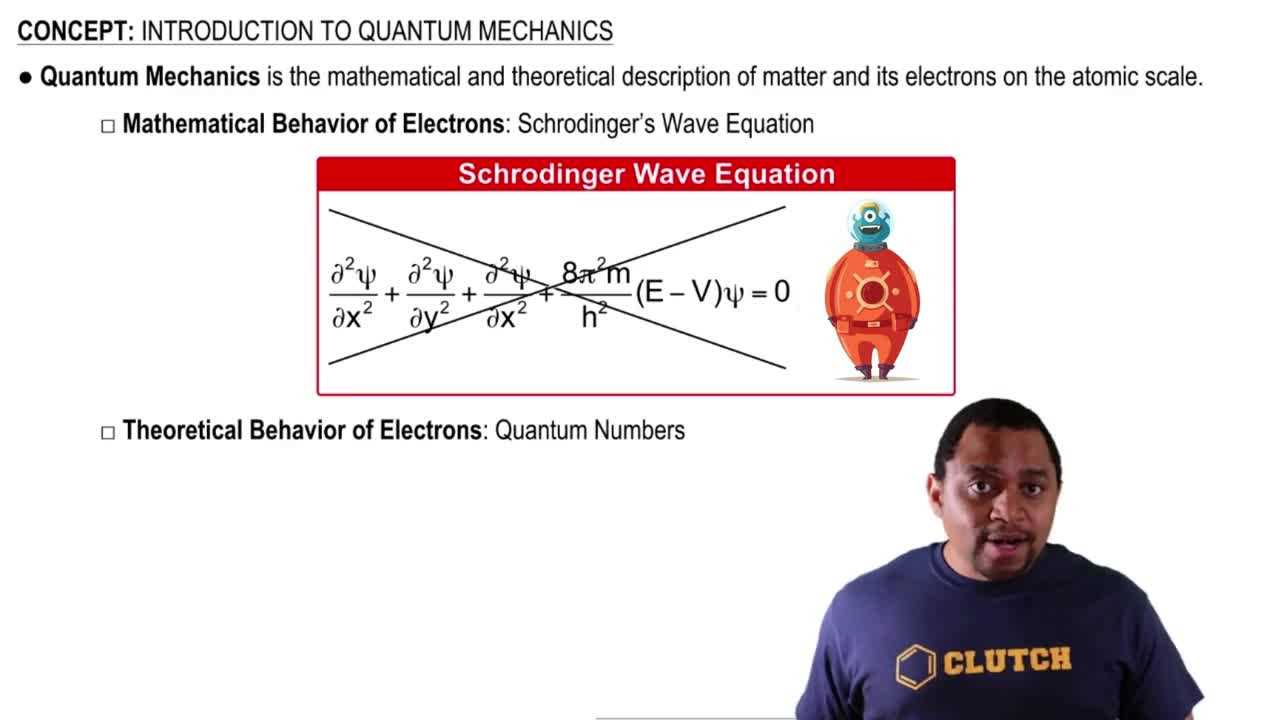
- Solving time-independent Schrödinger’s equation
- Exploring particle behavior in confined spaces
- Understanding the role of spin in quantum systems
Effective Approaches to Problem-Solving
- Break down complex problems into smaller, more manageable parts.
- Carefully analyze the given conditions and constraints.
- Apply appropriate mathematical tools to derive solutions step by step.
- Ensure all variables and constants are correctly interpreted and accounted for.
- Double-check final results for consistency with known physical principles.
These strategies not only help in solving typical problems but also train the mind to think critically, improving the ability to approach new, unforeseen challenges. By continuously practicing and refining these techniques, students will be better equipped to excel in their studies and apply their knowledge to real-world problems in advanced physical sciences.
Understanding Key Physics Concepts
A strong foundation in the fundamental principles of physics is essential for tackling complex problems. This section aims to clarify essential theories that serve as the building blocks of advanced physical understanding. By comprehending these key concepts, students can better approach theoretical models and apply them to various real-world scenarios.
Several core ideas form the basis for deeper exploration into advanced topics. These concepts are not just theoretical but have practical applications that reveal the true power of modern scientific understanding. Below is a breakdown of the most critical concepts to grasp:
| Concept | Definition | Importance |
|---|---|---|
| Wave-particle Duality | The concept that particles exhibit both wave-like and particle-like properties depending on the situation. | Essential for understanding the behavior of particles at microscopic scales. |
| Superposition Principle | A particle can exist in multiple states at once until measured, where it collapses into one state. | Key for grasping how particles behave in uncertain or unmeasured states. |
| Uncertainty Principle | The principle that there are limits to how precisely certain pairs of properties, like position and momentum, can be known simultaneously. | Fundamental for understanding measurement limitations and the behavior of particles. |
| Energy Quantization | The idea that energy is not continuous but comes in discrete levels, especially in confined systems like atoms. | Important for understanding atomic structures and electron behavior. |
| Wavefunction | A mathematical description of the quantum state of a system, used to determine probabilities of outcomes. | Central for calculating outcomes and predicting future behavior of systems. |
Each of these concepts plays a critical role in formulating the theories that describe the behavior of matter at a microscopic scale. A thorough understanding of these ideas is necessary for progressing to more advanced topics and solving intricate problems in the field.
Top Questions on Wave-Particle Duality

The concept of wave-particle duality is one of the most fascinating and challenging ideas in modern physics. It reveals that objects traditionally thought of as particles can also exhibit properties of waves, and vice versa. Understanding this dual nature is crucial for tackling many advanced problems in the field. In this section, we will explore the most common challenges and provide insight into how to approach them.
Common Issues in Understanding Wave-Particle Behavior
- How can a particle behave like a wave in certain experiments?
- What experimental evidence supports the idea of duality?
- Can wave-particle duality be observed in macroscopic objects?
- How does wave-particle duality relate to uncertainty in measurements?
- What is the significance of the de Broglie wavelength?
Approaching Problems Involving Wave-Particle Duality
- Carefully consider the context of the experiment–whether wave-like or particle-like behavior is observed.
- Focus on the key variables, such as momentum and wavelength, when analyzing the dual nature of particles.
- Utilize mathematical models, such as the de Broglie hypothesis, to relate wavelength to particle properties.
- Always keep in mind that duality is not contradictory but a reflection of the complexity of nature at microscopic scales.
By exploring these central questions, students can gain a deeper understanding of the phenomenon and its applications. This knowledge is essential for progressing in the study of physical systems where wave-particle duality plays a critical role.
Important Operators to Study
In the study of advanced physical systems, operators play a central role in describing how quantities like position, momentum, and energy behave in different states. These mathematical tools are essential for analyzing how particles interact with each other and with external forces. Understanding the key operators is crucial for solving problems and making predictions about physical systems. This section highlights some of the most important operators that every student should focus on.
Essential Operators in Physical Systems
- Position Operator: Describes the location of a particle in space.
- Momentum Operator: Relates to the motion of particles and their associated momentum.
- Hamiltonian Operator: Represents the total energy of a system, including both kinetic and potential energy.
- Energy Operator: Helps in understanding the energy levels of a system, especially in bound states.
- Angular Momentum Operator: Defines rotational properties of particles and systems.
Approaches to Working with Operators
- Apply operators to wavefunctions to determine measurable quantities.
- Understand commutation relations between different operators to uncover fundamental properties of systems.
- Use eigenvalues and eigenvectors to find possible outcomes of measurements on physical systems.
- Leverage the Schrödinger equation to see how operators evolve over time in dynamic systems.
Grasping the role and application of these operators is essential for building a deeper understanding of physical systems and solving related problems effectively. These operators are the foundation for much of the analysis in modern physics, allowing scientists to predict and understand phenomena in the microscopic world.
States and Superposition Explained

In the study of advanced physical systems, understanding the behavior of particles requires more than just knowing their position or momentum at any given time. Instead, it involves exploring how particles exist in multiple potential states simultaneously. This concept, known as superposition, is fundamental to describing the probabilistic nature of particles and their interactions. In this section, we will explore the key principles behind quantum states and how superposition allows particles to exhibit a range of possible behaviors until they are measured.
Superposition is one of the most fascinating aspects of particle behavior. It challenges our classical understanding of objects being in one state or another and instead shows that particles can exist in all possible states at once, only collapsing into a single state upon observation. The following table summarizes some of the critical points about states and superposition:
| Concept | Definition | Significance |
|---|---|---|
| Superposition Principle | The idea that a particle can exist in a combination of all possible states until measured. | Enables the calculation of probabilities for different outcomes. |
| Wavefunction | A mathematical function representing a particle’s state, which encodes information about all possible outcomes. | Helps in predicting the likelihood of finding a particle in a particular state when measured. |
| Collapse of the Wavefunction | The process by which a particle’s wavefunction collapses into a definite state when observed. | Represents the transition from a superposition of possibilities to a single outcome. |
| Interference | The phenomenon where different superposed states combine to influence the outcome of an experiment. | Demonstrates the wave-like nature of particles and plays a key role in experiments like the double-slit experiment. |
Understanding the implications of superposition is essential for analyzing complex systems and solving problems in modern physics. The ability to describe particles in terms of multiple potential states allows scientists to model behaviors that would otherwise be impossible to predict using classical methods.
Energy Quantization in Physical Systems

In advanced physical systems, energy is not always continuous as we might expect in classical mechanics. Instead, in many cases, it comes in discrete packets or levels. This concept, known as energy quantization, plays a pivotal role in explaining the behavior of particles in confined systems, such as atoms or molecules. Understanding how energy is restricted to specific values allows us to describe phenomena such as atomic transitions, electron behavior, and the stability of atoms.
Energy quantization is a direct consequence of the boundary conditions and wave-like nature of particles in these systems. This section will examine how energy levels are formed, the mathematical models used to describe them, and their significance in different physical contexts. The table below highlights key aspects of energy quantization in various systems:
| System | Energy Quantization Description | Significance |
|---|---|---|
| Particle in a Box | The energy levels are discrete and proportional to the square of the integer quantum numbers. | Shows how confinement leads to quantized energy states and influences particle behavior. |
| Harmonic Oscillator | The energy levels are equally spaced, with the lowest energy corresponding to a non-zero value. | Used to model molecular vibrations and important for understanding thermal energy distributions. |
| Hydrogen Atom | Energy levels are quantized in a manner dependent on the principal quantum number, with the electron occupying specific orbitals. | Explains the discrete spectral lines observed in atomic emission and absorption spectra. |
| Quantum Well | Energy levels are confined within the well’s boundaries, resulting in a discrete set of possible energy states. | Important for understanding semiconductor devices and optical properties in nanomaterials. |
Energy quantization not only provides a deeper understanding of fundamental atomic structures but also has practical applications in technologies such as lasers, semiconductors, and spectroscopy. Grasping the concept of discrete energy levels is essential for furthering the study of complex physical systems and solving related problems.
Applications of Heisenberg Uncertainty Principle
The principle of uncertainty highlights a fundamental limit to how precisely we can know certain properties of a particle, such as its position and momentum, simultaneously. This limitation has far-reaching implications, not just in theoretical physics but also in practical applications. The inability to measure both properties with perfect accuracy impacts various fields, from particle physics to technology. In this section, we will explore the significance of this principle and how it influences real-world scenarios.
Key Areas Affected by the Uncertainty Principle
- Particle Physics: The uncertainty principle limits the precision with which we can observe the exact state of subatomic particles, influencing particle collision experiments and the design of accelerators.
- Semiconductor Technology: In devices like transistors and microchips, the uncertainty principle governs electron behavior at tiny scales, affecting their efficiency and performance.
- Quantum Cryptography: The principle forms the foundation of secure communication technologies, where any attempt to observe the system disrupts its state, ensuring security.
- Imaging and Microscopy: In high-precision instruments such as electron microscopes, the principle limits the resolution at the microscopic level, affecting the clarity of the images produced.
- Quantum Computing: The uncertainty principle impacts the development of quantum computers, where maintaining superposition and coherence of qubits is key to computation.
Impact on Everyday Technologies
- Laser Technology: The precision in laser systems is influenced by the uncertainty principle, which plays a role in the coherence of light sources.
- GPS Navigation: Although not directly related to particles, the principle influences the accuracy of measurements that are essential in satellite technology and positioning systems.
- Medical Imaging: In techniques like MRI, the uncertainty principle contributes to the limits of spatial resolution, guiding improvements in imaging technology.
Although the uncertainty principle often presents challenges, it also drives innovations in fields that rely on precision measurement. By understanding and working within its limitations, scientists and engineers are constantly finding new ways to adapt and apply these fundamental constraints to advance technology.
Solving Schrödinger’s Equation in Detail

One of the central tasks in understanding the behavior of physical systems at a microscopic scale is solving the fundamental equation that describes how these systems evolve over time. This equation forms the foundation for predicting the behavior of particles in various potential fields. Solving it requires a careful approach, as the equation itself contains terms that describe both the particle’s energy and its spatial distribution within a given system. In this section, we will delve into the process of solving this key equation and the methods used to obtain meaningful results.
The equation can be applied in different scenarios, ranging from simple systems with well-defined potentials to more complex systems where the potential is variable. The solution provides insight into the possible states of the system, from the energy levels to the wave functions that describe the probability of finding a particle in a given state. The following table outlines key steps involved in solving this equation and common techniques used in its application:
| Step | Method | Explanation |
|---|---|---|
| 1 | Separation of Variables | A common technique where the spatial and temporal parts of the wave function are treated separately, simplifying the equation for time-independent cases. |
| 2 | Boundary Conditions | Specifying the conditions at the boundaries of the system, such as potential walls or limits, ensures that solutions remain physically valid and correspond to measurable outcomes. |
| 3 | Wavefunction Solutions | Finding specific wavefunctions that satisfy the equation, often through the use of well-known functions like sinusoidal or exponential forms for simple systems. |
| 4 | Energy Quantization | For systems with discrete boundary conditions, solving the equation often results in quantized energy levels, a direct consequence of boundary constraints. |
| 5 | Approximation Methods | For more complex systems, approximation techniques such as perturbation theory or numerical methods may be necessary to find an approximate solution. |
By applying these steps, scientists and engineers can obtain precise models of physical systems, allowing for the prediction of outcomes in experiments or the development of new technologies. The process may seem challenging, but mastering it is essential for anyone studying the underlying principles of particle dynamics in various fields of science.
Particle in a Box Problem Overview
The “particle in a box” model is one of the simplest and most fundamental problems in the study of particle dynamics. It describes a particle confined within a potential that is zero inside a specified region and infinite outside that region. This idealized scenario helps in understanding the behavior of particles when they are restricted to a particular space, allowing for the exploration of energy states and wave functions. Although a simplified model, it serves as a foundation for more complex systems in theoretical physics.
In this problem, the particle is assumed to be free within the box, with no forces acting upon it except for the boundaries that confine its motion. The solution of this problem reveals crucial insights into the nature of energy quantization, as only certain discrete energy levels are allowed for the particle. The following sections provide a deeper look into the problem’s components and its significance.
Key Features of the Model
- Confinement: The particle is restricted to move within a defined space, typically represented as a one-dimensional box with rigid walls.
- Boundary Conditions: The potential is infinite outside the box, ensuring that the particle cannot escape. Inside the box, the potential is constant and equal to zero.
- Energy Quantization: Due to the confinement, the particle can only possess specific, quantized energy values, derived from the boundary conditions and the wave nature of the particle.
Mathematical Approach to the Problem
- Wave Function: The solution to the problem involves finding the wave function of the particle, which describes its spatial distribution within the box.
- Schrödinger’s Equation: The time-independent Schrödinger equation is used to determine the allowed energy states of the particle. The solutions to this equation are sinusoidal functions that satisfy the boundary conditions.
- Energy Levels: The energy of the particle is quantized, with each possible energy corresponding to a specific mode of vibration of the wave function. These energies are proportional to the square of the integer quantum number.
While the particle in a box problem is a simple model, it provides valuable insight into the behavior of confined particles. Understanding how energy is quantized in this idealized scenario is a critical first step in studying more complex systems where particles interact with other forces and potentials.
Quantum Tunneling and Its Significance

In many systems, particles can exhibit behavior that seems to defy classical expectations. One of the most intriguing phenomena is when particles pass through energy barriers that they would not normally be able to surmount based on their energy alone. This effect, often referred to as tunneling, is a result of the wave-like nature of particles and the inherent uncertainty in their properties. Tunneling plays a crucial role in various physical processes, from atomic interactions to the operation of modern technologies.
The significance of this phenomenon extends beyond theoretical interest. Tunneling is essential in processes like nuclear fusion, where atomic nuclei overcome repulsive forces to fuse together at high temperatures, a process critical for energy production in stars. It also underlies the functioning of devices such as tunnel diodes and scanning tunneling microscopes, which have revolutionized fields such as electronics and surface science.
Understanding how particles can “tunnel” through seemingly insurmountable barriers opens up new possibilities for both fundamental research and practical applications. This effect demonstrates the deep connection between wave dynamics and the behavior of particles, offering insight into the non-intuitive nature of the microscopic world.
Spin and Angular Momentum in Quantum Mechanics
In the study of microscopic systems, certain properties of particles, such as their rotation and intrinsic motion, play a fundamental role in understanding their behavior. These properties, namely spin and angular momentum, are key to describing how particles interact with forces and how they are constrained by physical laws. Both of these quantities have profound implications in fields ranging from atomic physics to particle theory.
Spin refers to an intrinsic form of angular momentum that does not depend on the particle’s motion through space. Unlike classical spinning objects, the spin of a particle is a fundamental property that can only take on discrete values. Angular momentum, on the other hand, is associated with the motion of a particle within a system, and it describes the tendency of the particle to rotate around a point or axis.
Key Features of Spin
- Intrinsic Property: Spin is an inherent characteristic of particles, not related to any external motion.
- Discrete Values: Unlike classical rotational motion, spin can only take specific, quantized values (e.g., ±1/2 for electrons).
- Pauli Exclusion Principle: The behavior of fermions, particles with half-integer spin, follows this principle, which dictates that no two fermions can occupy the same quantum state simultaneously.
Angular Momentum in Systems

- Orbital Angular Momentum: Describes the angular momentum of a particle moving through space, dependent on the particle’s position and momentum.
- Quantization: Just like spin, angular momentum in quantum systems is quantized, with discrete allowed values based on the particle’s orbital configuration.
- Coupling: When multiple particles interact, their individual angular momenta can combine, forming composite systems with total angular momentum.
Understanding spin and angular momentum is crucial for explaining phenomena such as atomic structure, spectral lines, and particle interactions. Their behavior underpins the structure of matter at the smallest scales and is essential for understanding both basic and advanced topics in physics.
Quantum Entanglement and Non-locality Insights
In the realm of subatomic particles, certain phenomena challenge our classical understanding of reality, leading to concepts that seem counterintuitive but are crucial for describing the behavior of particles. One such phenomenon is the entanglement of particles, where two or more particles become interconnected in such a way that the state of one particle directly influences the state of another, regardless of the distance between them. This connection suggests a level of instantaneous interaction that seems to defy the limitations imposed by classical physics, particularly the speed of light.
Non-locality, a term often associated with entanglement, describes the ability of particles to exhibit correlated behaviors that cannot be explained by traditional local interactions. This behavior indicates that information about the particles’ states is shared across space without any physical connection, raising deep questions about the nature of space, time, and causality. While these effects have been experimentally verified, they continue to prompt discussions about the fundamental structure of reality itself.
Entanglement is not only a theoretical concept but also a practical tool in the development of emerging technologies. Quantum computing, for example, leverages the entanglement of qubits to perform computations that are exponentially faster than those possible with classical systems. Similarly, quantum cryptography uses the principles of entanglement to ensure secure communication channels, making the study of non-locality an essential area of research in both foundational and applied physics.
Key Aspects of Quantum Measurement Theory

In the study of microscopic systems, one of the most fundamental questions revolves around how measurements are made and how these measurements influence the systems being observed. Unlike classical systems, where properties can be measured without disturbing the system, in certain subatomic contexts, the act of measurement plays a crucial role in determining the outcome. This creates a complex relationship between the observer and the observed, challenging our classical understanding of measurement and reality.
When a system is measured, its state collapses into one of the possible outcomes, a process that is inherently probabilistic. This behavior is governed by the principles of measurement theory, which aim to explain how physical properties of a system emerge from a range of potential states. One of the key concepts is the superposition principle, where a system exists in multiple states at once, and only upon measurement does it “choose” a specific state.
The measurement problem emerges when we consider the role of the observer. Unlike classical physics, where the observer does not influence the state of the system, in subatomic realms, the observer’s presence can fundamentally change the outcome. This paradox has led to various interpretations, such as the many-worlds hypothesis and the Copenhagen interpretation, each offering different perspectives on the nature of reality and observation.
Furthermore, the observer effect suggests that certain properties, like position or momentum, cannot be simultaneously known with perfect accuracy. This limitation is not due to imperfections in measurement tools, but is a fundamental feature of the systems themselves. This principle is encapsulated in the Heisenberg uncertainty principle, which highlights the intrinsic limitations on measurement precision for complementary properties.
Understanding these principles is crucial for advancing technologies such as quantum computing, where the precision of measurement and control over quantum states can lead to groundbreaking advancements in computational power.
Interpreting the Copenhagen Interpretation

The Copenhagen Interpretation is one of the most well-known frameworks for understanding the behavior of subatomic particles. Developed in the early 20th century by Niels Bohr and Werner Heisenberg, this interpretation offers a way to reconcile the strange and often paradoxical nature of microscopic systems with observable phenomena. It asserts that before a system is measured, it exists in a superposition of all possible states, and only upon measurement does it collapse into a definite state.
One of the central ideas of the Copenhagen Interpretation is that the physical properties of particles, such as position or momentum, are not determined until they are observed. This leads to the notion that the observer plays an active role in shaping the outcome of experiments. The act of measurement, in this view, is not simply a passive recording of information but an event that directly influences the system being studied.
Wave Function and Collapse
At the heart of the Copenhagen Interpretation is the concept of the wave function. This mathematical tool describes the probability distribution of all possible states of a system. Prior to measurement, the system is in a superposition of all these possible states, and the wave function represents the likelihood of each outcome. When a measurement is made, however, the wave function “collapses,” and the system assumes a definite state. This collapse is instantaneous and non-deterministic, governed by probability rather than certainty.
The Role of the Observer

Another crucial aspect of the Copenhagen Interpretation is the observer’s role in shaping reality. In classical physics, the observer is independent of the system being observed, but in this framework, the observer’s intervention is essential to determine the state of a system. This raises philosophical questions about the nature of reality itself and whether it exists independently of observation or is, instead, brought into existence by the act of measurement.
Despite its success in explaining experimental results, the Copenhagen Interpretation has been the subject of debate. Critics argue that the role of the observer is too ambiguous and that the interpretation does not provide a clear picture of what happens during the wave function collapse. Alternative interpretations, such as the many-worlds theory, have been proposed to address these issues, but the Copenhagen Interpretation remains one of the most influential and widely accepted frameworks in the field of subatomic physics.
Understanding Quantum Harmonic Oscillator

The harmonic oscillator is a fundamental model used to describe a wide range of physical systems, from molecular vibrations to electromagnetic fields. It is especially important in subatomic physics, where systems often exhibit oscillatory behavior around an equilibrium position. In this model, a particle is confined to move in a potential well that is symmetric, such as a spring or a system with restoring forces. This idealized system is useful for understanding how particles behave in conditions where their motion is periodic or oscillatory.
In classical mechanics, the harmonic oscillator is described by a simple equation, but in the realm of subatomic particles, the behavior becomes more complex. When analyzed using the principles of wavefunctions and probability, the system behaves in ways that classical physics cannot fully explain. The quantum version of the harmonic oscillator incorporates the concepts of energy quantization, discrete states, and uncertainty, which results in a unique set of behaviors that are observable only at the microscopic level.
Energy Levels and Quantization

One of the key differences between classical and quantum models of the harmonic oscillator is the quantization of energy. In classical mechanics, the energy of the oscillator can take any continuous value, but in the quantum version, only specific discrete energy levels are allowed. These energy levels are evenly spaced and are determined by the frequency of the oscillation and the mass of the particle involved. The lowest energy state, known as the ground state, is not zero but has a minimum amount of energy, often referred to as zero-point energy.
Wavefunctions and Probability Distributions

In the quantum description, the position and momentum of the particle are described by a wavefunction, which provides a probability distribution of where the particle might be found at any given time. These wavefunctions for the harmonic oscillator take the form of standing waves, with the particle being more likely to be found at certain points in its oscillatory motion. The wavefunction solutions for the harmonic oscillator are associated with the different energy levels, and each solution corresponds to a particular quantum state with a distinct energy.
This model provides a critical insight into the behavior of many physical systems. It is particularly important in areas like molecular physics, where atoms in a molecule vibrate with frequencies that are modeled by harmonic oscillators. The harmonic oscillator also plays a vital role in the study of phonons, the quantized modes of vibration in a solid, and other collective phenomena that arise in various physical systems.
Examination of Quantum Mechanics Postulates
The foundational principles of this field rest on a set of fundamental postulates that define the behavior of systems at microscopic scales. These guiding rules provide the structure for understanding how particles behave under different conditions, laying the groundwork for theoretical predictions and experimental interpretations. Each postulate offers a framework for calculating probabilities, energy levels, and interactions, ensuring consistency across various models within the discipline.
Understanding the core assumptions behind the theory is crucial for grasping its wide-reaching implications. These principles govern everything from the superposition of states to the role of measurement, making them essential in explaining phenomena that deviate from classical expectations. By analyzing these postulates, one can better appreciate the abstract yet highly effective nature of this field in describing physical reality.
State Function and its Representation

The first postulate defines the state of a physical system using a mathematical object known as the state function or wavefunction. This function encapsulates all the information needed to describe the system’s properties, such as position, momentum, and energy. The wavefunction is typically a complex-valued function, and its square gives the probability density of finding the system in a particular state. This mathematical description is central to predicting outcomes and understanding the behavior of particles in diverse situations.
Observables and Their Mathematical Operators

Another key principle is the relationship between physical quantities, known as observables, and their corresponding mathematical operators. These operators act on the state function, yielding values that correspond to measurable quantities. For instance, the momentum and energy of a particle are represented by specific operators, which, when applied to the system’s state, give possible results. The eigenvalues of these operators correspond to the discrete outcomes that can be observed in an experiment, while the eigenfunctions describe the state of the system in that particular measurement context.
These postulates help form the backbone of theoretical investigations, allowing predictions about a system’s behavior under various conditions. They provide a consistent framework that has been proven effective in explaining a wide range of phenomena, from atomic transitions to the behavior of particles in complex systems. By studying these foundational principles, one gains a deeper understanding of how physical systems are governed at the smallest scales, leading to more precise and meaningful experiments in the field.
Preparing for Advanced Physics Assessments
Successfully tackling advanced assessments in this field requires more than just memorizing formulas and concepts. A deeper understanding of the underlying principles, as well as the ability to apply them to new problems, is essential. This process involves mastering both theoretical concepts and their practical implications, ensuring you are well-equipped to handle complex scenarios and interpret experimental results accurately.
Strategic preparation is key. It involves revisiting the foundational ideas introduced in the earlier stages of study, reviewing the core concepts thoroughly, and practicing with problems that test your comprehension and problem-solving skills. The more time you spend actively engaging with the material, the easier it will be to recall and apply it under pressure. Key to this preparation is understanding the principles that govern the behavior of systems at microscopic scales, as they form the basis for solving more advanced problems.
Key Topics to Focus On
When preparing, it is crucial to focus on a few key areas that are regularly tested. These topics provide a framework for many of the problems you will encounter:
- State Functions: Understanding how to interpret and manipulate state functions is fundamental for solving many problems in this area. Ensure you are comfortable with the mathematical formulation and physical meaning of wavefunctions.
- Operators and Observables: Be sure you can apply mathematical operators to find measurable quantities like energy, momentum, and position. Understanding the relationship between operators and their eigenvalues is crucial.
- Time Evolution: The ability to solve for the time dependence of a system’s state is essential. Mastering the Schrödinger equation, both in time-dependent and time-independent forms, is key to solving dynamic problems.
- Measurement Theory: Grasping the concept of wavefunction collapse and how measurements influence the state of a system is critical for understanding outcomes of experiments.
- Particle in a Box and Potential Wells: These problems illustrate the core principles of energy quantization and boundary conditions and are commonly used to test understanding of theoretical applications.
Effective Study Strategies

Effective study habits can make a significant difference in your preparation. Here are some strategies to consider:
- Practice, Practice, Practice: The more problems you solve, the better your understanding of the material will be. Try to solve a variety of problems to expose yourself to different types of scenarios and techniques.
- Review Class Notes and Textbooks: Go over the material presented in class, and use textbooks to reinforce your understanding. Pay particular attention to examples worked out in detail, as these often highlight key methods and strategies.
- Group Study: Collaborating with peers can help clarify concepts and provide new perspectives on difficult topics. Explaining concepts to others is an excellent way to solidify your own understanding.
- Work on Past Papers: Going through previous assessments or sample problems helps you become familiar with the format and types of questions you may face. It also gives you a sense of the level of detail expected in your answers.
By focusing on these areas and following these strategies, you will be well on your way to mastering the subject and performing successfully in your assessments.