
In this section, we will explore essential techniques and strategies for improving your skills in solving mathematical problems. Whether you’re tackling basic principles or more complex challenges, developing a strong foundation is crucial to success. By focusing on core concepts and practicing regularly, you will build the confidence needed to excel in your studies.
Understanding the underlying principles is the first step in achieving proficiency. By breaking down problems into smaller, manageable parts, it becomes easier to apply logical reasoning and discover the most efficient solutions. This approach will help you approach each task with clarity and precision, ensuring consistent progress in mastering difficult topics.
Effective practice is key to long-term retention. As you engage with various exercises, pay close attention to the methods and techniques that work best for you. Remember, consistent effort and review of previous lessons are essential in reinforcing your knowledge and boosting your problem-solving abilities.
Through thoughtful analysis and repetition, you will improve your ability to tackle increasingly challenging problems. This ongoing effort will not only help you achieve academic success but also prepare you for real-world applications of mathematical thinking.
Geometry Practice Solutions
This section provides solutions and step-by-step explanations to key problems in the subject. Focusing on core mathematical skills, it aims to clarify the methods used to solve various exercises and reinforce your understanding. By analyzing these solutions, you’ll be able to identify patterns and strategies that will improve your problem-solving approach.
Each example is broken down into manageable parts, ensuring that you can follow along easily and apply similar strategies to other tasks. Consistency in practice is vital, as repetition helps reinforce concepts and techniques. Understanding the reasoning behind each step will allow you to develop a deeper mastery of the material.
Reviewing your work after completing each problem is an essential part of the learning process. It’s important to evaluate your approach, check for accuracy, and identify areas where further improvement may be needed. This reflection helps build confidence and refine your skills for future challenges.
By engaging with these detailed solutions, you’ll gain valuable insights into effective problem-solving and strengthen your ability to tackle a wide range of exercises. The more you practice, the more proficient you’ll become in applying these methods to new and increasingly complex problems.
Understanding Key Geometry Concepts
To master any mathematical field, it’s essential to first grasp the fundamental principles that serve as the building blocks for more complex problems. In this section, we will explore the core ideas that guide how shapes, angles, and sizes are analyzed and manipulated in various problems. A clear understanding of these basics makes it easier to approach challenges with confidence and clarity.
Key concepts that every learner should focus on include:
- Shapes and their properties: Identifying different figures and understanding their attributes, such as sides, angles, and symmetry.
- Angles: Learning how angles are formed and how to measure them, as well as their relationship to different shapes.
- Perimeter and area: Calculating the perimeter and area of various figures, such as triangles, rectangles, and circles.
- Volume and surface area: Understanding how to calculate the volume and surface area of 3D shapes like cubes and spheres.
Each of these concepts plays a crucial role in tackling problems efficiently. When approaching a question, break it down by identifying the shape or figure involved and determine which of these concepts applies. Once the basic elements are understood, more advanced topics can be approached with greater ease.
By focusing on these core ideas, you will build a strong foundation that allows you to tackle even the most intricate problems with ease and accuracy.
Step-by-Step Guide to Mastering Concepts
Understanding the essential principles in mathematics requires a structured approach. In this section, we will break down the process of solving key problems into clear, manageable steps. Each stage will guide you through the logical progression needed to arrive at the correct solution, helping you build a solid grasp of the material and improve your problem-solving skills.
Step 1: Identify the Problem
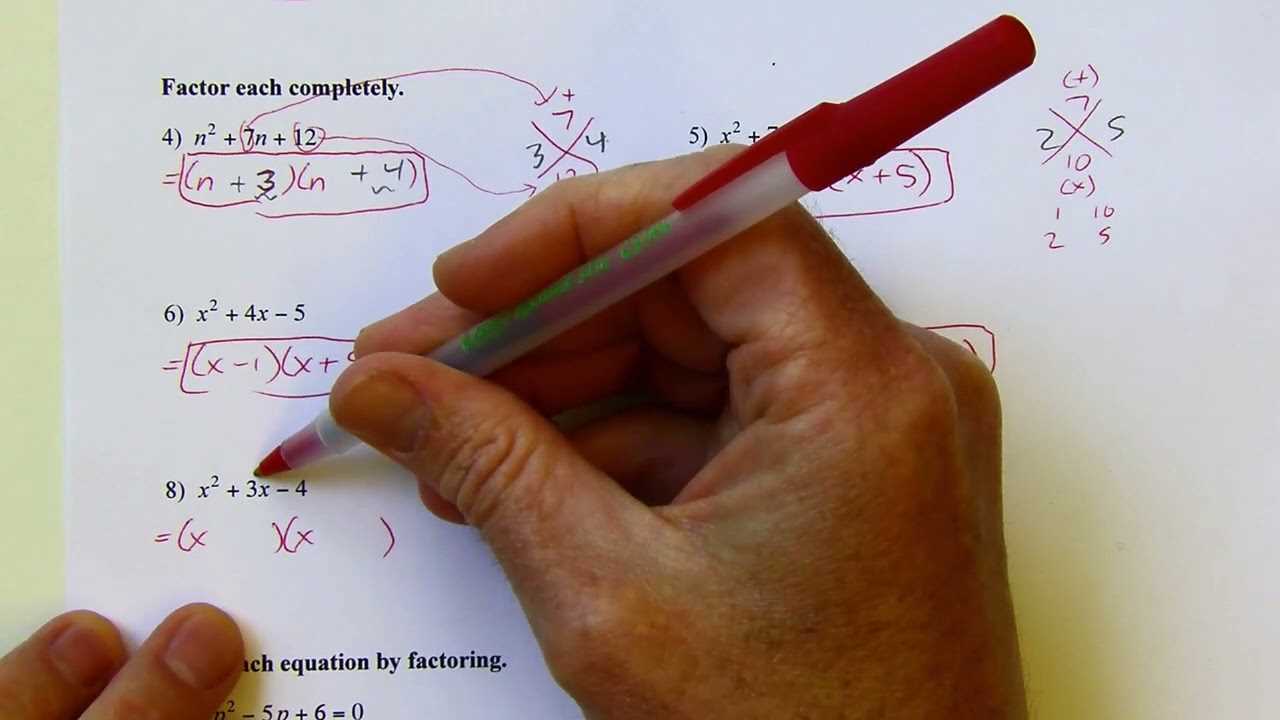
The first step in any problem-solving process is to clearly understand the question. Look for the given information and determine what needs to be solved. This will help you set up the framework for solving the problem and ensure that you stay focused on the most important aspects.
Step 2: Break Down the Solution
Once you understand the problem, break it down into smaller, more manageable parts. Identify which formulas or concepts are relevant, and work through each section methodically. Taking time to solve each part step by step will reduce errors and improve your confidence in finding the correct solution.
By following this step-by-step approach, you’ll be able to navigate even the most challenging problems with greater ease, ensuring accurate results and a deeper understanding of the subject matter.
Common Mistakes in Mathematical Exercises

When working through mathematical problems, it’s easy to make errors that can hinder your progress. These mistakes often arise from misunderstandings of basic concepts, miscalculations, or rushing through steps without fully analyzing the task. Recognizing these common errors can help you avoid them and improve both your accuracy and understanding.
Misinterpreting the Question: One of the most frequent mistakes is failing to understand exactly what the problem is asking. Take time to carefully read and identify key information. Missing a single detail can lead to incorrect conclusions.
Incorrect Application of Formulas: While formulas are essential tools, using them incorrectly can lead to significant mistakes. Ensure that you are applying the right formula to the right situation, and always check that you have the correct values before plugging them in.
Overlooking Units and Measurements: Forgetting to account for units or converting them improperly is another common error. Always double-check that the units match the problem’s requirements and that you’re using them consistently throughout the solution.
Rushing Through Calculations: Sometimes, in an attempt to finish quickly, important details are overlooked. Always take your time to carefully perform calculations, especially when working with complex figures or multiple steps.
By being aware of these common mistakes and adopting a more deliberate and thoughtful approach, you’ll enhance your ability to solve problems accurately and efficiently.
How to Solve Mathematical Problems Effectively
Approaching mathematical problems with a clear strategy can make solving them more manageable and efficient. By following a systematic process, you can break down complex problems into simpler, more understandable steps. The key is to stay organized and ensure that every part of the solution is carefully thought through.
Here are some steps to help you tackle problems more effectively:
| Step | Action |
|---|---|
| 1 | Understand the Problem: Read the question carefully and identify what is given and what needs to be found. This ensures you’re focused on the right objective. |
| 2 | Plan the Approach: Determine which formulas or theorems are applicable. Sketching a diagram or visualizing the problem can also help. |
| 3 | Work Step-by-Step: Break the problem down into smaller, more manageable parts. Solve each part methodically without rushing. |
| 4 | Double-Check Your Work: Always review your calculations to ensure that you haven’t missed anything or made a simple mistake. |
By following this process, you can approach even the most difficult problems with a clearer mindset and greater confidence. Remember, taking the time to plan and review your work can often make the difference between a correct and incorrect solution.
Tips for Mastering Mathematics Concepts
Mastering mathematical concepts requires both consistent practice and a deep understanding of the foundational principles. By adopting the right strategies, you can enhance your learning and improve your problem-solving skills. Below are several tips that can help you succeed in mastering the material effectively.
Build a Strong Foundation
- Focus on understanding the core principles before moving on to more advanced topics.
- Review previous concepts regularly to reinforce your knowledge and ensure continuity in your learning.
- Identify the key formulas and theorems that are frequently used and make sure you understand their applications.
Practice Regularly and Strategically
- Consistent practice is crucial for mastering mathematical problems. Set aside dedicated time each day for solving exercises.
- Start with simple problems and gradually work your way up to more complex ones to build confidence and skill.
- Don’t just focus on completing problems–focus on understanding the steps and reasoning behind each solution.
By applying these strategies, you can steadily improve your understanding and develop a more effective approach to solving challenging problems. Remember, mastery comes with persistence and the willingness to continuously improve.
Breaking Down the Key Theorems in Mathematics
In mathematics, understanding key theorems is essential for solving problems and building a deeper comprehension of the subject. Theorems provide the foundation upon which many solutions are built, offering established principles that can be applied to a wide range of situations. Breaking down these theorems into simpler components helps in mastering their application and improving problem-solving skills.
Each theorem typically involves a set of conditions that must be met and a conclusion that can be drawn when those conditions are satisfied. To effectively use theorems, it’s important to understand both the logic behind them and how they relate to the specific problem at hand. By learning to recognize when and how to apply these rules, you can tackle even the most complex mathematical challenges with greater ease.
Mastering the core theorems gives you the tools to solve a variety of problems, helping you make informed decisions about which approach to take and how to proceed with confidence.
Why Consistent Practice is Essential in Mathematics
Consistent engagement with mathematical problems is vital for mastering key concepts and enhancing problem-solving abilities. By working through a variety of challenges, learners develop the skills necessary to apply theoretical knowledge in real-world scenarios. The more problems you tackle, the more confident and efficient you become in recognizing patterns and employing the right strategies to find solutions.
Reinforcing Understanding
Regular practice: helps reinforce the fundamental concepts that form the basis of more complex topics. The more you work with different problems, the better you understand how these concepts apply across different contexts.
Building Problem-Solving Skills
Familiarity with problem types: comes through repeated exposure. With time, you’ll recognize common patterns, formulas, and strategies that can be used to tackle new challenges with ease. This not only makes you faster but also more accurate in your approach.
Improvement through repetition is key to gaining fluency in any mathematical area. By practicing regularly, you sharpen your skills and build the confidence needed to approach even the most difficult problems with certainty.
Strategies for Tackling Complex Mathematical Questions
When facing intricate problems, breaking them down into smaller, more manageable parts is crucial for understanding and solving them efficiently. Developing a step-by-step strategy helps reduce confusion and ensures that no detail is overlooked. Below are several strategies that can assist in tackling challenging questions with confidence.
Step-by-Step Breakdown
Start by identifying the given information and what needs to be found. Next, devise a plan that breaks the problem into smaller tasks. Addressing each part systematically allows for easier management of the overall challenge.
Utilizing Visual Aids

Diagrams, charts, and graphs are incredibly useful when solving complex problems. They provide a visual representation of the problem and can help you see relationships between different elements that might not be immediately obvious.
| Step | Action |
|---|---|
| 1 | Analyze the Given Information: Carefully note what is provided in the question, including measurements and relationships between shapes. |
| 2 | Identify the Required Outcome: Determine what needs to be calculated or proven. |
| 3 | Apply Relevant Theorems: Use appropriate formulas and concepts to work through the problem step by step. |
| 4 | Review Your Solution: Double-check your calculations and reasoning to ensure everything aligns with the given information. |
By following these strategies and approaching complex problems with patience and organization, you’ll improve your ability to solve challenging tasks with greater accuracy and confidence.
Using Visual Aids in Mathematical Exercises
Incorporating visual tools can significantly enhance your understanding of complex problems. By creating diagrams, sketches, and other representations, abstract concepts become more tangible. Visual aids help clarify relationships between different elements, making it easier to identify patterns and develop strategies for solving problems efficiently.
One of the most effective ways to approach mathematical tasks is by drawing a clear diagram. Whether it’s a shape, graph, or chart, visualizing the problem provides an intuitive way to grasp the underlying concepts and guide you toward the solution. This approach is particularly useful for problems that involve spatial reasoning or multiple relationships between different parts of the figure.
Additionally, using color coding or labels in your visual aids can further organize the information and highlight key components. This added clarity allows you to track progress as you work through the problem, ensuring no details are overlooked.
How to Check Your Mathematical Solutions

Ensuring that your solutions are correct requires a systematic approach to verification. It’s not enough to simply arrive at an answer–double-checking the process and confirming that all steps align with the rules is essential for accuracy. By following a few reliable methods, you can ensure your results are both correct and well-supported.
Revisit the Problem Statement
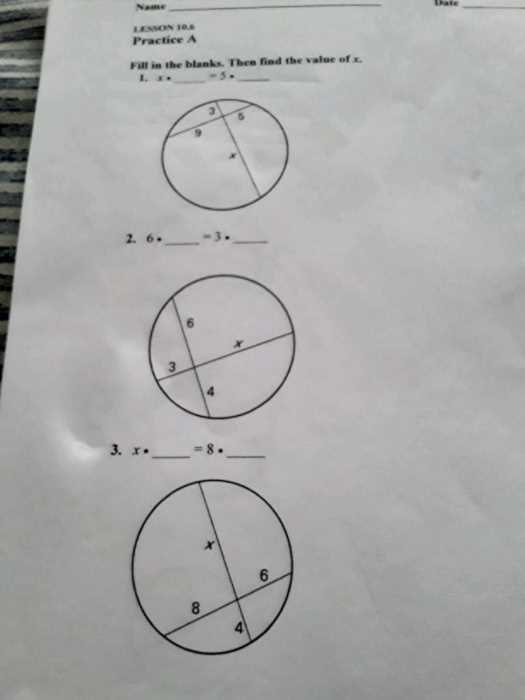
Start by carefully reviewing the original problem. Ensure that you’ve interpreted all the given information correctly and that no details were overlooked. Sometimes, errors stem from misunderstanding or misapplying the information in the question.
Double-Check Your Calculations
Go over your calculations step-by-step to verify each operation. If possible, rework the problem from scratch to confirm that your steps follow logically and consistently. Additionally, consider using a different method or approach to solve the problem as a secondary check. This can help identify any mistakes that might have been missed in the original attempt.
By reviewing your work and confirming each detail, you can confidently ensure the accuracy of your solution and identify any potential errors before finalizing your response.
Improving Accuracy in Mathematical Problem Solving
Achieving precision in problem-solving is essential for correctly applying mathematical concepts and obtaining reliable results. By focusing on careful steps and thoughtful strategies, you can reduce mistakes and enhance your accuracy. The following techniques are helpful in ensuring that your solutions are as accurate as possible.
Focus on Understanding the Problem
The first step in any mathematical task is to clearly understand what is being asked. Ensure that you are not only interpreting the problem correctly but also identifying all relevant information. Misunderstandings at this stage can lead to errors later on.
Use Structured Approaches
Using a step-by-step process can significantly improve your precision. Breaking down complex problems into smaller, manageable tasks makes it easier to stay on track and reduces the chance of skipping important details.
- Read the problem carefully: Take time to understand every part of the question and extract all the necessary information.
- Identify key relationships: Recognize patterns or formulas that can be applied to solve the problem.
- Check your work: After completing each step, review it before moving on to ensure there are no mistakes.
By following these strategies, you can sharpen your problem-solving skills and ensure that your solutions are both accurate and well-supported by logical reasoning.
Review of Important Mathematical Formulas
In mathematical problem-solving, understanding and applying key formulas is crucial for achieving accurate results. These essential equations provide the foundation for solving various types of problems efficiently. Below is a review of some of the most commonly used formulas in this field, which can help streamline your approach to tackling different challenges.
| Formula | Purpose | Example |
|---|---|---|
| Area of a Triangle | Used to calculate the area of a triangle. | Area = 1/2 * base * height |
| Pythagorean Theorem | Used to find the length of a side in a right triangle. | a² + b² = c² |
| Area of a Circle | Used to calculate the area enclosed by a circle. | Area = π * radius² |
| Perimeter of a Rectangle | Used to find the total length around a rectangle. | Perimeter = 2 * (length + width) |
| Volume of a Rectangular Prism | Used to calculate the space inside a rectangular box. | Volume = length * width * height |
Mastering these formulas is essential for tackling a wide range of mathematical problems. By familiarizing yourself with these core equations, you can approach challenges more confidently and with greater efficiency.
How to Study Geometry for Success
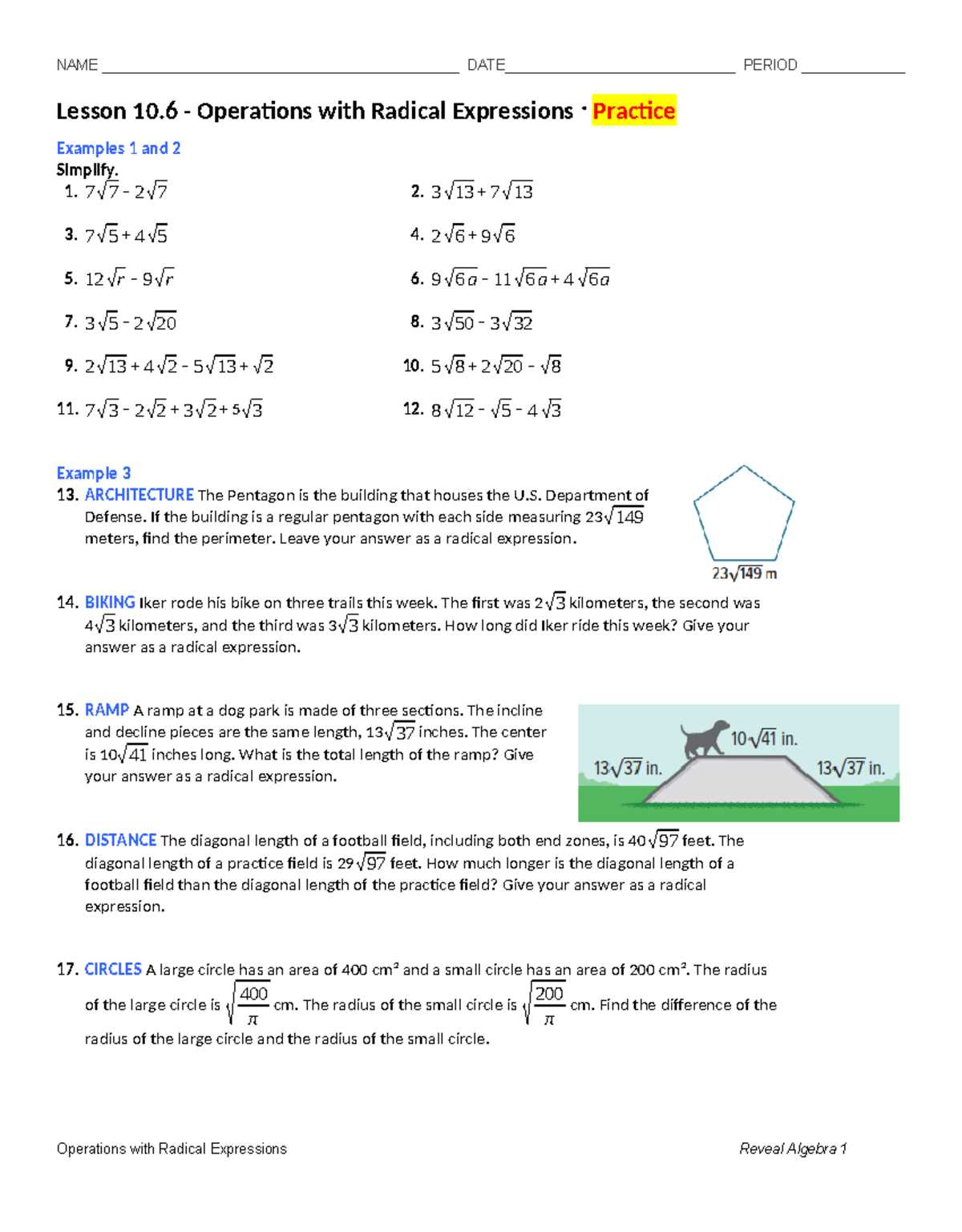
Success in mathematical subjects, particularly those involving shapes, angles, and spatial reasoning, requires a structured approach to studying. By developing a deep understanding of the underlying principles and applying them consistently, you can improve both your comprehension and performance. Below are some effective strategies that will help you master the key concepts and succeed in your studies.
- Focus on Understanding the Concepts – Rather than just memorizing formulas, take the time to understand the logic behind each one. This will help you solve problems more easily and apply the concepts to different situations.
- Break Down Complex Problems – Start by breaking down difficult problems into smaller, manageable parts. This will allow you to tackle each component step by step and find the solution more effectively.
- Practice Regularly – Consistent practice is key to reinforcing your skills. Work through various problems and examples to develop familiarity with different types of questions and scenarios.
- Use Visual Aids – Diagrams and illustrations are powerful tools for visualizing relationships between shapes and angles. Draw them out whenever possible to gain a clearer understanding of the problem.
- Seek Help When Needed – Don’t hesitate to ask for help from teachers, peers, or online resources when you’re struggling. Getting clarification on concepts that are unclear can save you time and frustration later on.
By implementing these strategies, you can enhance your study routine and build a solid foundation for success in mathematical subjects. Stay persistent and patient, and your efforts will lead to improvement over time.
Understanding Geometry Practice Solutions
Mastering any mathematical topic involves not only learning the rules and methods but also understanding how to apply them effectively. When solving problems, it’s crucial to break down the steps and fully grasp the reasoning behind each solution. This understanding helps solidify your knowledge and ensures that you can approach future challenges with confidence.
Solutions are not just about finding the correct answer; they also illustrate the process used to arrive at that answer. By reviewing and analyzing these steps, you can gain insight into different problem-solving techniques, identify common mistakes, and learn how to improve your approach. With a clear understanding of the process, you can become more proficient in solving similar problems in the future.
When working through a solution, focus on the following:
- Identify Key Information: Always start by understanding the problem’s requirements and what information is provided. This helps in formulating a strategy to solve the question.
- Follow the Logical Sequence: Pay attention to the order in which steps are performed. Proper sequencing ensures that all necessary operations are conducted correctly.
- Understand the Methods: Ensure that you comprehend the reasoning behind each step. Understanding why a specific method is used will make it easier to apply in other problems.
- Learn from Mistakes: If you make an error, take the time to review it. Mistakes often reveal misunderstandings that can be corrected by revisiting the principles.
By focusing on the steps that lead to the solution, you develop a deeper understanding of the concepts, which will aid in faster and more accurate problem-solving in the future. Studying solutions is an essential part of the learning process that builds both confidence and competence.
How to Apply Geometry to Real Life
The concepts learned in mathematics, especially those related to shapes, measurements, and spatial relationships, are not confined to the classroom. In fact, they are vital tools used in everyday life. From construction to technology, many aspects of the world around us rely on the principles of space and form. Understanding these concepts allows you to see the practical applications and enhances problem-solving abilities in various situations.
Everyday Applications
Here are several ways in which spatial reasoning is used in day-to-day life:
- Architecture and Construction: Building designs, whether for homes or bridges, depend heavily on the understanding of shapes, areas, and volumes. Accurate measurements ensure structures are stable and functional.
- Art and Design: Artists, architects, and graphic designers regularly use proportions, symmetry, and perspective to create visually pleasing works. Spatial thinking helps in scaling and visualizing designs.
- Navigation: When traveling, either on foot or by vehicle, concepts such as angles, distances, and directions are crucial. Maps and GPS systems are based on geometric principles to calculate the shortest or most efficient route.
- Sports: Many sports require an understanding of angles and distances, from calculating the arc of a basketball shot to determining the best angle for hitting a baseball.
Practical Problem-Solving
By applying mathematical thinking to real-world problems, you not only find solutions but also gain insights into how the world works. Understanding spatial relationships can help you:
- Estimate distances and areas for projects, whether you’re planning a garden or remodeling a room.
- Determine the best layout for furniture or equipment, ensuring that spaces are used efficiently.
- Make better decisions when shopping, such as calculating the most cost-effective material quantities for a home improvement project.
As you continue to explore and apply these principles, you’ll see how valuable they are in various aspects of life. The ability to visualize and calculate will lead to improved decision-making and more effective problem-solving in practical situations.