
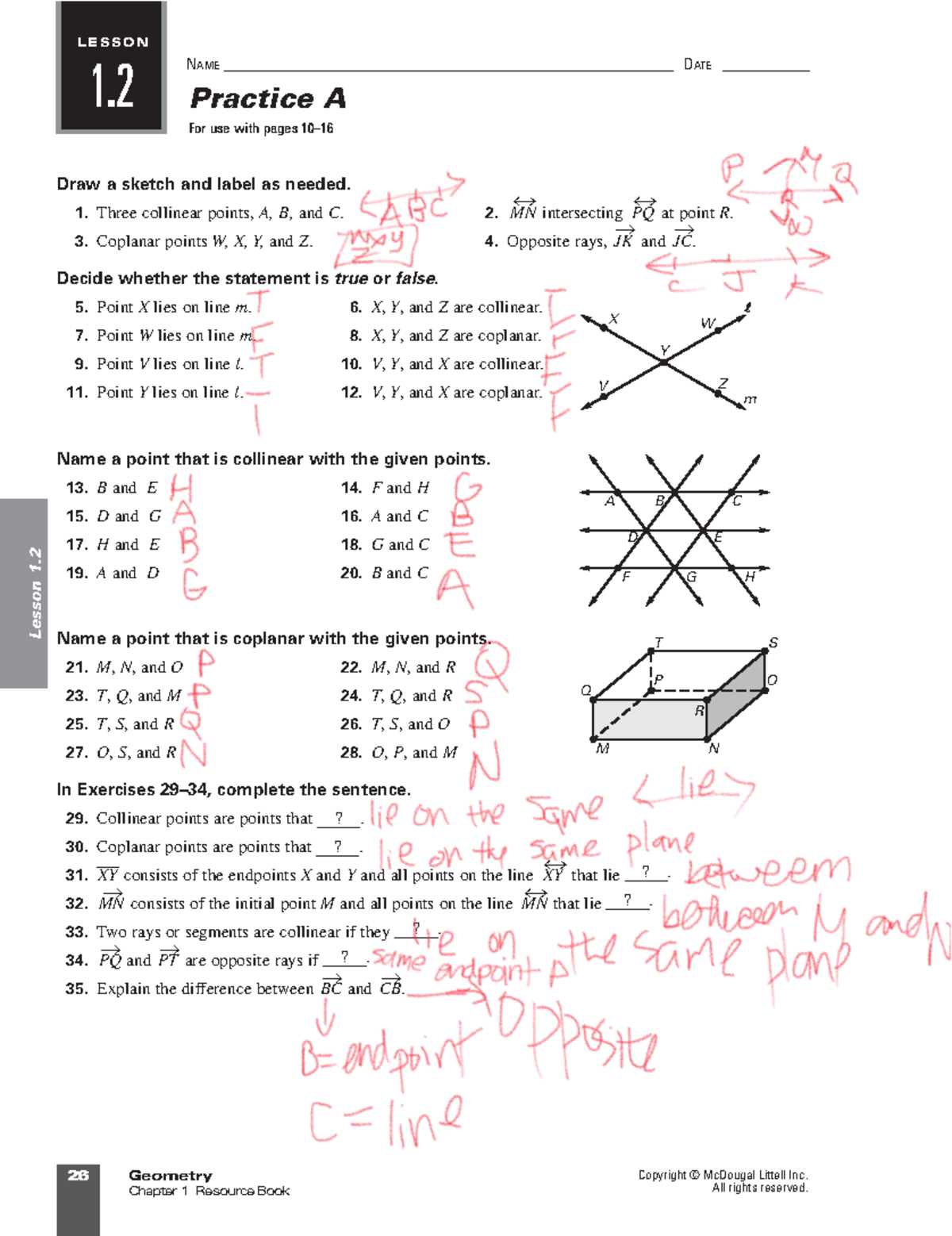
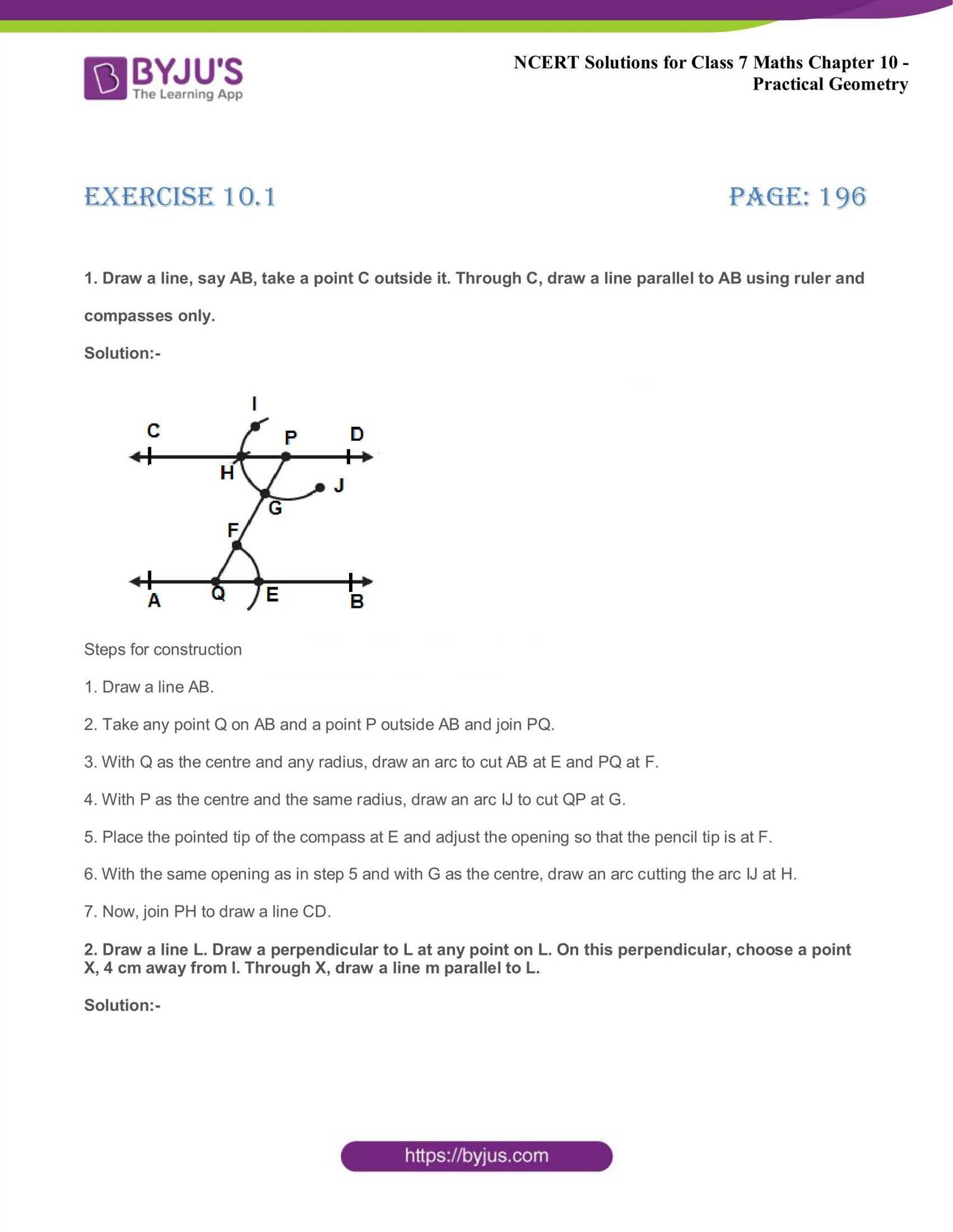
One of the best ways to strengthen your understanding of mathematical principles is through consistent problem-solving. Whether you’re tackling basic operations or complex concepts, solving related tasks sharpens your skills and enhances your ability to think critically. The process of working through challenges allows you to identify patterns, learn from mistakes, and build a solid foundation for more advanced topics.
In this section, we will explore various exercises designed to test your knowledge and improve your problem-solving efficiency. With clear step-by-step solutions and practical techniques, you’ll gain a deeper insight into how to approach and resolve each type of question. These strategies not only reinforce key ideas but also prepare you for exams and real-world applications.
Reviewing detailed solutions and understanding the logic behind each step can be incredibly helpful. By examining the methods used to arrive at the correct answers, you can avoid common pitfalls and refine your technique. This approach ensures that you aren’t just memorizing formulas, but truly grasping the reasoning behind them.
Geometry Practice Book Answer Guide
Understanding how to navigate through various mathematical tasks is essential for mastering key concepts. A solid approach involves not only working through the exercises but also examining the methods used to derive the solutions. This guide is designed to provide you with the necessary tools and insights to effectively solve problems and reinforce your learning.
Breaking Down Problem-Solving Techniques
Each challenge presents a unique opportunity to apply different techniques. Knowing how to break down complex problems into smaller, more manageable steps is crucial for success. Here’s how you can tackle problems efficiently:
- Identify Key Information: Look for important details in the problem that will help guide your solution.
- Choose the Right Method: Select an approach that aligns with the type of task, whether it’s algebraic, visual, or logical.
- Check Your Work: After solving, ensure your results are consistent and make sense in the context of the question.
Effective Learning Strategies
Beyond simply solving individual tasks, it’s important to develop strategies that enhance your overall comprehension. Here are some methods to improve your learning process:
- Practice Regularly: Consistent engagement with different problems helps solidify your understanding and boosts retention.
- Understand the Steps: Focus on the reasoning behind each solution, not just the final result.
- Learn from Mistakes: Review any errors and understand why they occurred to avoid repeating them in the future.
By following these guidelines, you’ll be able to build a deeper understanding and sharpen your skills. The goal is not just to find the correct results, but to understand why those results are correct and how they were derived. This approach will help you grow both in confidence and proficiency.
How to Use Geometry Practice Answers
Having a comprehensive solution guide can be a game-changer when it comes to mastering mathematical concepts. It’s not just about finding the correct results, but about learning how to approach and solve problems step by step. By analyzing the methods behind the solutions, you can enhance your understanding and improve your problem-solving skills.
Step-by-Step Review Process

The key to utilizing any solution guide effectively is to break down each step carefully. Rather than simply copying the solutions, focus on understanding how each step leads to the next. Here’s a structured approach:
- Start with the Problem: Read through the problem statement carefully to ensure you understand what is being asked.
- Follow the Steps: Examine each step in the solution and make sure you can replicate the thought process behind it.
- Understand the Methods: Focus on the techniques and strategies used to solve the problem, rather than memorizing formulas.
Reinforcing Learning with Practice
Once you’ve reviewed the steps, it’s essential to apply what you’ve learned. Try solving similar problems on your own without looking at the solutions right away. This will help solidify your understanding and make sure you can use the methods independently.
- Work on Variations: Try solving problems with different parameters to apply the same principles in different scenarios.
- Review Mistakes: If you make an error, go back and analyze where things went wrong to avoid repeating the same mistake.
By using solution guides in this way, you can gradually build up your skills and gain confidence in your ability to solve complex tasks. Remember, the goal is to internalize the process so that you can apply it in any situation.
Step-by-Step Solutions for Geometry Problems
Breaking down complex tasks into manageable steps is an effective way to approach mathematical challenges. By following a structured method, you can make even the most difficult problems easier to solve. This section will guide you through the process of solving various types of questions by outlining each step clearly and systematically.
Understanding the Problem
The first step in any problem-solving process is to fully understand what is being asked. Here’s how to start:
- Read the Question Carefully: Identify key details and requirements in the statement.
- Highlight Important Information: Mark the data that will help in forming a solution.
- Visualize the Scenario: If possible, draw a diagram or sketch to help clarify the relationships between elements.
Executing the Solution
Once you understand the problem, it’s time to apply the appropriate methods. Follow these steps for efficient problem-solving:
- Choose the Right Approach: Select a method or formula that best fits the problem type.
- Break Down the Task: Divide the work into smaller, sequential steps that build on each other.
- Show Your Work: Write out each calculation or reasoning step clearly to avoid mistakes.
Verifying the Solution
After completing the solution, it’s crucial to ensure the correctness of your work:
- Double-Check Your Steps: Review the calculations and reasoning to identify any possible errors.
- Test Your Results: If applicable, substitute your answer back into the problem to verify it makes sense.
- Review the Context: Ensure the solution aligns with the original problem’s requirements and conditions.
By following these structured steps, you can tackle mathematical challenges with confidence and clarity. The goal is to not just find the answer, but to understand the process behind it, making future problems easier to solve.
Improve Your Geometry Skills with Practice
To master any mathematical discipline, consistent application and problem-solving are essential. The more you engage with different challenges, the better your understanding becomes. By regularly working through exercises, you enhance your ability to identify patterns, apply formulas, and solve problems efficiently. This section will explore how to improve your skills by engaging in focused tasks that reinforce key concepts.
Focus on Core Concepts
Before diving into complex questions, it’s important to ensure a solid grasp of the fundamental principles. Start by revisiting the basics and making sure you understand:
- Key Formulas: Review essential equations and their applications in various scenarios.
- Basic Shapes and Their Properties: Familiarize yourself with the characteristics of common figures like circles, triangles, and quadrilaterals.
- Measurement Techniques: Understand how to calculate areas, perimeters, and angles accurately.
Engage with Varied Problems
As you build your foundation, it’s crucial to challenge yourself with a wide variety of problems. Tackling diverse types of questions helps you apply your knowledge in different contexts and improves your problem-solving ability:
- Solve Word Problems: Work on tasks that require translating real-world scenarios into mathematical solutions.
- Time Yourself: Challenge yourself to solve problems under time constraints to simulate exam conditions and improve speed.
- Analyze Mistakes: Whenever you make an error, take time to understand where you went wrong and how to correct it.
By consistently practicing a range of problems and focusing on both fundamentals and advanced tasks, you can improve your skills and gain confidence in solving more complex challenges. Remember, mastery comes with repetition and reflection.
Common Geometry Problems and Their Solutions
In mathematical problem-solving, certain tasks are commonly encountered due to their foundational nature. These challenges, although varied in appearance, often rely on similar principles and techniques for resolution. By recognizing the types of questions that frequently appear, you can prepare yourself to approach them with confidence and clarity. This section highlights some of the most common problems and provides step-by-step solutions to help you understand the methods behind them.
Solving for Area and Perimeter
Calculating the area and perimeter of shapes is one of the most frequently asked tasks. Whether it’s a rectangle, triangle, or circle, knowing how to apply the correct formula is essential. Here’s a quick review:
- Rectangles: Area = length × width, Perimeter = 2(length + width)
- Triangles: Area = ½ × base × height, Perimeter = sum of all sides
- Circles: Area = π × radius², Circumference = 2π × radius
Working with Angles
Another common challenge involves solving for unknown angles, especially in polygons and when working with parallel lines. Here are a few strategies:
- Complementary Angles: If two angles add up to 90°, they are complementary. For example, if one angle is 30°, the other must be 60°.
- Supplementary Angles: If two angles add up to 180°, they are supplementary. For instance, if one angle is 120°, the other must be 60°.
- Angles in Parallel Lines: Alternate interior angles and corresponding angles are equal when two parallel lines are cut by a transversal.
Working with Pythagorean Theorem
The Pythagorean Theorem is a powerful tool used to solve for unknown sides in right-angled triangles. The formula is:
- Formula: a² + b² = c², where a and b are the lengths of the two legs, and c is the hypotenuse.
For example, if one leg is 3 units and the other leg is 4 units, the hypotenuse will be 5 units, as 3² + 4² = 9 + 16 = 25, and √25 = 5.
By understanding the common types of problems and how to apply basic techniques, you can approach each task with a clearer strategy. Regular practice will further solidify your ability to solve these problems efficiently.
Understanding Geometry Theorems Through Practice
Mathematical theorems are powerful tools that help explain relationships between different elements in shapes and figures. However, these principles are not always easy to grasp without proper application. By engaging with problems and applying theorems in various contexts, you can deepen your understanding and learn how to use these abstract ideas effectively. This section will focus on how consistent engagement with these concepts through exercises can solidify your comprehension.
Exploring the Pythagorean Theorem
One of the most fundamental theorems is the Pythagorean Theorem, which relates the sides of a right triangle. It states that the square of the hypotenuse is equal to the sum of the squares of the other two sides. Through continuous application, you can develop a strong understanding of how this theorem functions in different scenarios:
- Example 1: A right triangle with legs measuring 3 units and 4 units. Using the theorem, you find the hypotenuse as 5 units (3² + 4² = 9 + 16 = 25, √25 = 5).
- Example 2: Given one leg as 5 units and the hypotenuse as 13 units, solve for the missing leg by rearranging the formula: 5² + b² = 13².
Using the Properties of Parallel Lines
Another key concept that can be understood through practice is the relationship between angles when two parallel lines are intersected by a transversal. Theorems such as alternate interior angles and corresponding angles are critical for solving geometric problems:
- Alternate Interior Angles: These angles are congruent when a transversal intersects two parallel lines.
- Corresponding Angles: These angles are also congruent when formed by a transversal crossing parallel lines.
By working through exercises involving these theorems, you not only improve your ability to solve specific problems, but you also gain a deeper understanding of how these principles apply in real-world situations.
Effective Ways to Study Geometry Concepts
Mastering mathematical concepts requires a blend of consistent practice, clear understanding, and strategic learning. When studying topics related to shapes, angles, and spatial reasoning, it’s important to adopt techniques that help reinforce your knowledge and improve problem-solving skills. This section will highlight several effective study methods that can enhance your comprehension and retention of essential concepts.
Utilize Visual Learning
Mathematical problems are often best understood through visualization. Drawing diagrams and sketches can make abstract concepts more concrete and help you identify relationships between elements. Consider the following strategies:
- Draw Diagrams: Visualizing shapes and their properties can simplify problem-solving.
- Use Color Coding: Highlight different parts of a figure (e.g., angles, sides) with distinct colors to make connections more apparent.
- Use Geometric Tools: Tools like rulers, protractors, and compasses help you draw accurate diagrams and measure elements precisely.
Practice with a Variety of Problems
Regular exposure to a wide range of problems is key to reinforcing your skills. The more diverse the problems you tackle, the better your understanding will be. Here’s a structured approach:
| Study Technique | Description |
|---|---|
| Work on Sample Problems | Start with examples that cover basic concepts, then move on to more complex problems that challenge your understanding. |
| Timed Practice | Simulate test conditions by solving problems within a set time limit to build speed and accuracy. |
| Revisit Mistakes | Review errors carefully to identify gaps in knowledge and avoid repeating them in future problems. |
By integrating these methods into your study routine, you can enhance both your understanding and your problem-solving abilities. Consistency and reflection are key to mastering the concepts and excelling in mathematical challenges.
Key Geometry Topics Covered in Practice Books
When studying mathematical concepts related to shapes, sizes, and spatial relationships, there are several fundamental topics that are consistently addressed. These core areas help build a strong foundation for understanding and solving various types of problems. By mastering these subjects, learners can improve their ability to apply key principles in different scenarios. This section highlights the essential topics frequently covered in mathematical guides and exercises.
Among the most important topics are the properties and relationships between different types of shapes, such as triangles, circles, and polygons. Understanding the calculations for areas, volumes, and angles is crucial in solving problems. Additionally, concepts like symmetry, transformations, and congruence are frequently explored, helping learners understand how objects can be manipulated while maintaining their core properties. These subjects often form the basis for more advanced studies in mathematics and physics.
By focusing on these core concepts, learners are better equipped to approach a wide variety of problems with a deeper understanding of the underlying principles.
Solving Geometry Word Problems with Answers
Word problems present unique challenges as they require you to translate real-world scenarios into mathematical expressions and equations. Solving these types of problems involves critical thinking, an understanding of key concepts, and the ability to apply formulas in practical situations. This section will walk you through the process of solving these problems effectively, offering clear explanations and step-by-step solutions to ensure you gain the skills needed to tackle similar challenges.
To successfully solve word problems, follow these key steps:
- Read the Problem Carefully: Understand the context and identify what is being asked. Pay attention to keywords that indicate mathematical operations, such as “sum,” “difference,” or “area.”
- Identify the Relevant Information: Extract the important details, such as measurements, relationships, and any conditions mentioned in the problem.
- Draw a Diagram: If applicable, sketch the figure described in the problem. This will help you visualize the relationships between different elements.
- Choose the Right Formula: Based on the given information, select the appropriate formula or method to solve the problem (e.g., perimeter, area, volume).
- Solve the Equation: Perform the necessary calculations step by step to find the solution.
- Check Your Work: After finding the answer, review the solution to ensure it makes sense in the context of the problem.
Example Problem and Solution
Let’s apply this approach to a sample problem:
- Problem: A rectangle has a length of 8 units and a width of 5 units. What is the area of the rectangle?
Solution:
- Step 1: Identify the formula for the area of a rectangle: Area = length × width.
- Step 2: Substitute the given values into the formula: Area = 8 × 5 = 40 square units.
- Step 3: Check the answer: The area is indeed 40 square units, which is consistent with the problem’s dimensions.
By following these steps, you can solve any word problem involving shapes and spatial relationships. Consistent practice will help you gain confidence and improve your problem-solving skills.
How to Check Your Geometry Solutions
Verifying your solutions is a crucial part of problem-solving. Ensuring that your answers are correct not only boosts confidence but also helps reinforce your understanding of key concepts. There are several methods you can use to double-check your work and make sure you haven’t overlooked important details. In this section, we will explore various techniques to help you review and confirm your solutions effectively.
Here are some strategies to check your solutions:
- Review the Problem Statement: Reread the original question carefully to ensure you fully understand what is being asked. Make sure you’ve addressed every part of the problem.
- Revisit Your Calculations: Go over the steps you took to solve the problem. Check each calculation to ensure there are no arithmetic errors or misapplied formulas.
- Verify Units and Labels: Ensure that all units are consistent and that you’ve labeled all measurements correctly, such as lengths, areas, and angles.
- Use Estimation: Before finalizing your solution, estimate the result to see if it makes sense. If the calculated answer is drastically different from the estimate, review your process.
- Check with a Different Method: If possible, try solving the problem using an alternative approach. This will confirm whether your initial method was correct.
- Compare with Known Results: If applicable, check your solution against known examples or reference materials to verify its correctness.
By incorporating these verification techniques, you can minimize mistakes and improve your overall problem-solving accuracy. Regularly practicing these steps will help you develop stronger mathematical reasoning and attention to detail.
Top Resources for Geometry Practice and Answers
Mastering shapes, angles, and spatial relationships requires the right tools and guidance. A wide variety of materials are available to help individuals strengthen their understanding of these concepts, offering solutions and methods for improvement. Whether for self-study or academic support, finding reliable sources can make a significant difference in skill development.
Online Platforms and Interactive Tools
Several websites provide comprehensive exercises and step-by-step explanations to enhance problem-solving abilities. These interactive platforms often feature tutorials, quizzes, and custom worksheets, allowing learners to engage with the content at their own pace. By testing skills through instant feedback, these tools enable learners to pinpoint areas of weakness and track progress over time.
Educational Videos and Tutorial Series
Visual learners benefit from video lessons that break down complex concepts into manageable segments. Platforms like YouTube offer a range of content creators who specialize in presenting geometric challenges with clear solutions. These videos often include annotations and visual aids, helping viewers grasp abstract concepts more easily. Whether it’s for revising or exploring new topics, such tutorials provide an effective and accessible way to learn.
Practice Geometry with Real-World Examples
Understanding theoretical concepts becomes easier when applied to tangible scenarios. By observing and solving problems encountered in everyday life, individuals can better comprehend the practical use of mathematical principles. These real-world situations provide a more engaging and meaningful context for honing skills and gaining deeper insights.
Examples in Architecture and Design
Structures like buildings, bridges, and homes provide excellent examples of how spatial concepts are utilized in the real world. From measuring angles to determining areas and volumes, each design choice relies on mathematical reasoning. Below is a table showcasing how common architectural features relate to basic principles.
| Feature | Mathematical Principle | Application |
|---|---|---|
| Roof Pitch | Angles, Trigonometry | Calculating the slope and height of roofs for structural integrity |
| Window Shape | Area, Perimeter | Determining the size of windows for design and cost-efficiency |
| Columns and Pillars | Volume, Proportions | Designing stable supports with accurate measurements for strength |
Everyday Objects and Measurements
Even common items around the house or in nature can serve as great practice material. By measuring the height of a tree, the area of a table, or the angles in a staircase, one can apply theoretical knowledge in practical scenarios. This hands-on approach strengthens comprehension and boosts confidence in applying concepts beyond the classroom.
Why Geometry Practice Is Essential for Mastery
Achieving a high level of competence in mathematical concepts requires continuous engagement with the material. Repeated exercises and problem-solving not only reinforce understanding but also build the skills needed to tackle increasingly complex challenges. Regular involvement ensures that concepts are not only memorized but truly internalized, leading to mastery.
Building Strong Foundations
Engaging with exercises helps solidify fundamental principles, ensuring that individuals have a strong foundation to build upon. As more advanced topics are introduced, this solid base allows for a smoother transition and better application of new techniques. Without repeated reinforcement, it is easy to overlook important details that become critical in higher-level problems.
Enhancing Problem-Solving Skills
Through consistent work, individuals develop an intuition for identifying and solving various challenges. Each new problem presents a unique scenario, encouraging the development of creative approaches and critical thinking. Over time, this improves the ability to break down complex situations into manageable parts, enhancing overall problem-solving efficiency.
Benefits of Reviewing Geometry Answers
Going over the solutions to problems is a vital step in mastering any subject. It allows individuals to identify mistakes, understand their reasoning, and reinforce correct methods. This process not only improves accuracy but also deepens comprehension of key concepts, ultimately leading to greater confidence and efficiency in future tasks.
Identifying and Correcting Mistakes
By carefully analyzing each step of a solution, learners can pinpoint where errors occurred. This practice helps to avoid repeating the same mistakes and provides valuable insights into areas that need more focus. Understanding why a mistake was made is as important as solving the problem itself, as it enhances problem-solving skills and critical thinking.
Strengthening Conceptual Understanding
Reviewing completed problems allows individuals to solidify their grasp of the underlying principles. By seeing how theoretical ideas are applied in practice, learners can connect abstract concepts to real-world applications. This deeper understanding not only improves performance but also enables learners to approach new challenges with a more informed perspective.