
In this section, we explore important techniques and methods that will help you tackle a variety of problems. By focusing on fundamental principles, you’ll gain a deeper understanding of how to approach and solve complex tasks. Whether you’re looking to strengthen your skills or build a solid foundation, this guide provides essential insights for mastering essential topics.
Critical thinking and strategic planning are crucial when working through mathematical challenges. Recognizing patterns, breaking down problems into smaller parts, and applying logical steps will allow you to reach the correct solutions efficiently. These skills are valuable not just for academics but for real-world applications where problem-solving is key.
By practicing the techniques outlined here, you’ll be better equipped to solve even the most difficult problems with confidence. Each step is designed to help you grow your mathematical thinking and ensure accuracy in your results. The more you engage with these concepts, the clearer and easier the problem-solving process will become.
Geometry Chapter 6 Resource Book Overview
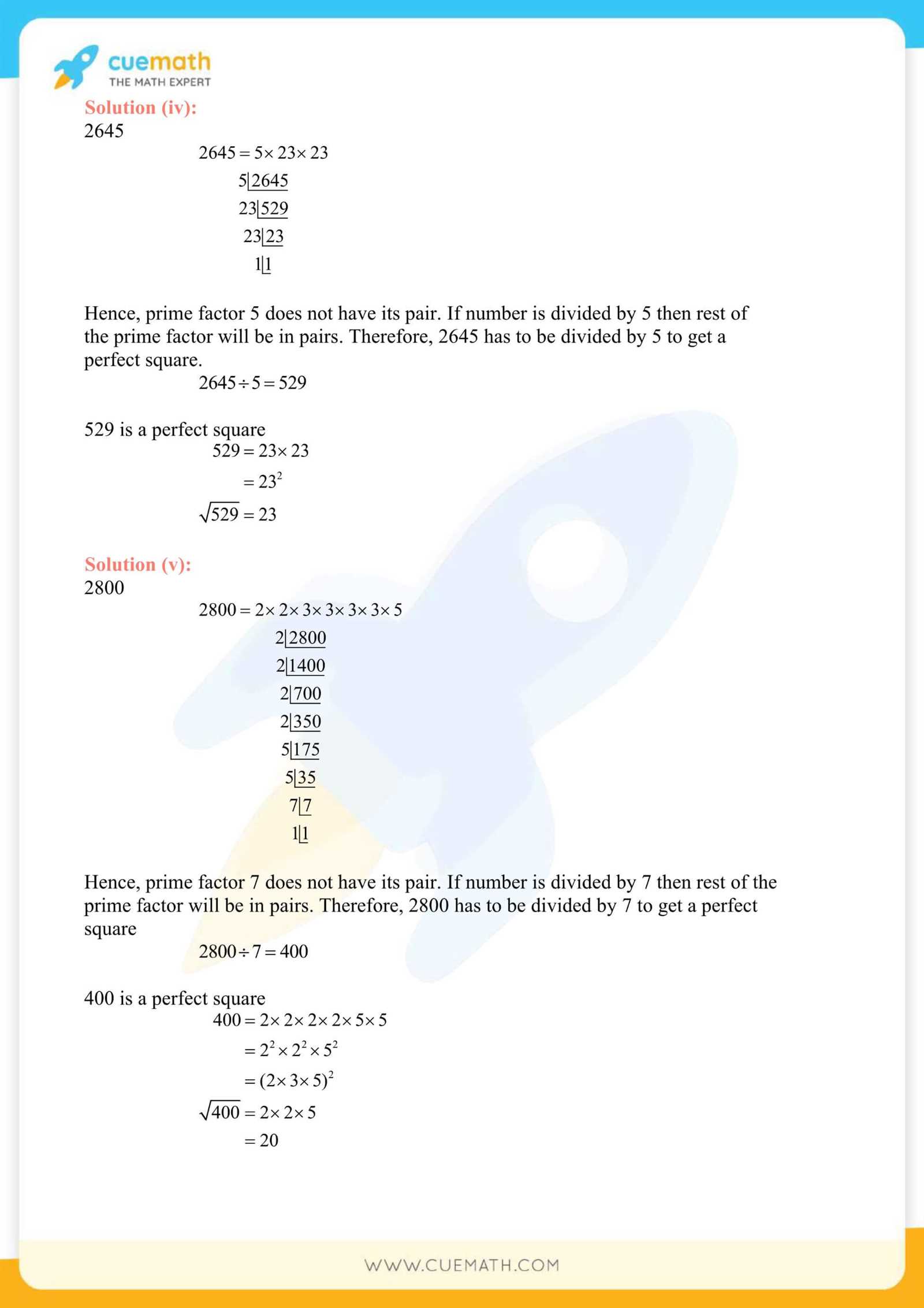
This section provides a comprehensive approach to the key concepts that are essential for mastering the material. It serves as a guide to understanding the core principles and provides various methods to tackle different types of problems effectively. By focusing on these foundational ideas, you will be able to build the necessary skills to solve more complex challenges with ease.
Throughout this resource, the primary goal is to develop both your theoretical knowledge and practical skills. A variety of strategies are introduced to help reinforce your understanding, offering clear steps to apply in problem-solving scenarios. The material is designed to support learning and provide tools that make abstract ideas more accessible and easier to grasp.
The following table summarizes the key sections of the guide and the topics covered in each part:
| Topic | Focus Area |
|---|---|
| Fundamentals | Basic principles and strategies |
| Problem-Solving Techniques | Step-by-step solutions to common problems |
| Advanced Applications | Complex scenarios and real-world applications |
| Practice Exercises | Opportunities for skill reinforcement |
| Review and Tips | Advice for mastering the material |
By engaging with the material and applying the techniques described, you will gain a thorough understanding of the key principles and how to use them in various problem-solving situations. This approach ensures that each concept is well-supported with practical examples, offering both depth and clarity for learners at all levels.
Understanding the Importance of Practice A
Engaging with exercises that reinforce key concepts is an essential part of mastering any subject. These activities provide an opportunity to apply theoretical knowledge to real-world scenarios, helping to solidify your understanding. Through repetition and critical thinking, you begin to grasp the material more deeply, which is vital for success in more advanced topics.
These types of tasks encourage active problem-solving, requiring you to break down complex ideas into simpler, manageable parts. By doing so, you develop not only technical skills but also the confidence to approach future challenges. The more you practice, the more intuitive and accurate your reasoning becomes, preparing you for higher-level thinking and applications.
Incorporating regular exercises into your study routine ensures that the learned concepts stay fresh and are better retained in long-term memory. This approach is a proven method for reinforcing knowledge and improving overall problem-solving abilities. The benefits of consistent practice extend beyond the immediate task, influencing your ability to tackle even the most difficult challenges with ease.
Key Concepts in Lesson 6.3 Explained
Understanding the foundational ideas behind each topic is crucial for building a strong mathematical base. In this section, we break down the main principles and offer clear explanations to help you better grasp the material. These concepts form the core of the subject, allowing you to make connections between abstract theories and practical applications.
Critical thinking and logical reasoning are key to unlocking the full potential of the material. By understanding how to approach problems systematically, you can solve even the most complex tasks with ease. Each concept is introduced step-by-step, emphasizing the relationship between various elements and how they work together.
Once you familiarize yourself with the core ideas, it becomes easier to tackle different scenarios and think critically about how to apply the concepts. Whether you are dealing with simple or advanced problems, having a clear understanding of the underlying principles will guide you through the solution process.
Step-by-Step Guide to Solving Practice A
To effectively tackle the given problems, it’s important to follow a structured approach. This step-by-step guide is designed to break down each task into manageable parts, ensuring clarity and precision in solving each one. By following these steps, you will improve your problem-solving skills and gain a deeper understanding of the core principles involved.
Step 1: Analyze the Problem
The first step in solving any problem is to carefully read and understand the task at hand. Identify the key components and variables, and think about how they relate to each other. Visualization can be extremely helpful here–consider drawing diagrams or making notes to organize your thoughts. Once you have a clear picture, you’ll be better prepared to apply the right techniques.
Step 2: Apply the Relevant Methods
Once you have a clear understanding of the problem, it’s time to choose the appropriate solution strategies. Whether it’s algebraic manipulation, geometric visualization, or logical deduction, using the right method is crucial for success. As you work through the steps, remember to check your calculations regularly to ensure accuracy. Double-checking your work at each stage can prevent errors and lead to more reliable results.
By breaking down the problem and applying the right methods, you’ll find that each task becomes easier to solve, and you will gain confidence in your problem-solving abilities.
How to Approach Geometry Problem Solving
Effective problem-solving starts with a clear strategy that allows you to break down complex situations into simpler, manageable steps. The key is to approach each problem with a structured mindset, using logic and reasoning to navigate through the various elements. By following a methodical process, you can avoid common pitfalls and find solutions more efficiently.
Step 1: Understand the Problem
The first step is to ensure that you fully understand the question or task. Take time to read it carefully and identify the given information and what is being asked. It’s often helpful to underline or highlight important details and variables that may be relevant to the solution. By visualizing the problem, you can better organize the data and plan your next steps.
Step 2: Identify the Appropriate Approach
Once the problem is clear, the next step is selecting the right approach. Depending on the situation, this might involve using formulas, constructing diagrams, or applying logical reasoning. Understanding which technique is best suited for the problem will save time and increase your chances of finding the correct solution.
The following table outlines some common methods for problem-solving:
| Method | When to Use |
|---|---|
| Algebraic Techniques | When the problem involves equations or expressions. |
| Drawing Diagrams | When visualizing the scenario will help clarify the solution. |
| Logical Reasoning | When the problem requires critical thinking and deduction. |
| Using Formulas | When the problem involves known mathematical relationships. |
By carefully analyzing each problem and selecting the appropriate method, you will find that solving challenges becomes a more systematic and rewarding process.
Common Mistakes in Practice A and How to Avoid Them
As with any subject, when working through problems, it’s easy to make mistakes, especially when under time pressure or dealing with complex tasks. Recognizing common pitfalls and knowing how to avoid them is essential for improving your problem-solving abilities. In this section, we’ll examine some typical errors and provide tips on how to prevent them, ensuring more accurate and efficient results.
Misinterpreting the Problem
One of the most frequent mistakes is misunderstanding the problem itself. Sometimes, key details can be overlooked, leading to incorrect assumptions and solutions. To avoid this, always take the time to carefully read through the problem multiple times. Highlight or underline important information and ensure that you fully understand what is being asked before proceeding. Taking a moment to clarify any doubts upfront can save time and prevent errors in the long run.
Rushing Through Calculations
Another common mistake is rushing through calculations, especially when the process involves multiple steps or operations. It’s easy to skip steps or make simple arithmetic errors when you try to work too quickly. To avoid this, focus on accuracy rather than speed. Double-checking each step as you go along can catch mistakes early, ensuring that your final answer is reliable. Patience and precision are key to successful problem-solving.
By taking your time to carefully analyze the problem and check your work, you can significantly reduce the likelihood of these common mistakes. Remember that practice and attention to detail are essential for mastering the material and improving your skills.
Visualizing Problems Effectively
One of the most powerful tools for solving complex problems is the ability to visualize the situation clearly. Creating mental or physical representations of the elements involved can make abstract concepts more tangible, helping you to better understand relationships and connections. Effective visualization not only aids in solving problems but also strengthens overall comprehension of the material.
To visualize problems effectively, follow these key strategies:
- Draw Diagrams – Sketching a diagram of the problem can simplify complex information and help you see how different elements relate to one another.
- Label Important Information – Clearly marking key variables, angles, and distances on your diagram helps you stay focused on the most relevant details.
- Use Color or Different Shapes – Differentiating parts of the diagram with color or distinct shapes can help to highlight critical aspects and prevent confusion.
Visualization not only makes the task easier but also enhances your ability to think critically. Once you have a clear image in mind, it becomes easier to apply the correct techniques and solve the problem systematically. Additionally, practicing this method will improve your spatial reasoning and strengthen your problem-solving skills over time.
By incorporating these visual tools into your approach, you will gain a clearer, more organized perspective on problems, leading to more efficient and accurate solutions.
Mastering the Fundamentals of Geometry
Building a strong foundation in the core principles of any subject is essential for tackling more advanced topics. The fundamentals provide the tools and knowledge necessary to solve problems and understand complex concepts. By mastering these basic elements, you’ll be able to approach more difficult challenges with confidence and efficiency.
Here are some key fundamental concepts that are crucial to understand:
- Understanding Shapes and Their Properties – Recognizing the properties of various shapes such as triangles, circles, and polygons is essential. Each shape has unique characteristics that govern how it can be manipulated or analyzed.
- Learning Key Theorems and Formulas – Knowing and applying important rules like the Pythagorean theorem, area, and perimeter formulas is fundamental for solving problems.
- Identifying Relationships Between Angles – Understanding how angles interact, such as complementary and supplementary angles, is crucial for solving many types of problems.
- Using Logical Reasoning – Being able to reason through a problem step-by-step allows you to arrive at the correct solutions. This is essential in every type of mathematical problem-solving.
By focusing on mastering these core principles, you’ll be well-equipped to tackle more complex problems with greater ease. Solidifying your grasp of these basics ensures that you won’t be overwhelmed by more advanced topics and will enable you to build upon this knowledge as you progress in your studies.
Breaking Down Complex Problems
When faced with intricate problems, the key to success is breaking them down into smaller, more manageable parts. Rather than attempting to solve everything at once, focusing on one element at a time helps prevent confusion and errors. A structured approach allows you to simplify complex situations, making them easier to solve step-by-step.
Step 1: Identify Key Elements
The first step is to carefully read and analyze the problem to identify all the critical components. This includes the known values, unknown variables, and any relationships between them. Marking or underlining the key information can help focus your attention on the most important aspects of the problem. Once you’ve outlined the essentials, you can start organizing your approach.
Step 2: Break It Into Sub-Problems
Next, divide the larger problem into smaller sub-problems that are easier to tackle. For example, if the task involves multiple calculations or relationships, approach each one individually. Focus on solving each smaller part before combining the results. This not only makes the problem feel less overwhelming but also reduces the chances of making mistakes along the way.
By using this step-by-step approach, you’ll be able to tackle even the most complex challenges with clarity and confidence, ensuring more accurate and efficient problem-solving.
Tips for Quick Calculation in Geometry
Speed is often essential when solving mathematical problems, but it’s important not to sacrifice accuracy for the sake of speed. Developing strategies for faster calculation can make problem-solving more efficient, especially when working with complex tasks. Below are some practical tips for enhancing your calculation skills and reducing the time it takes to solve problems.
Master Key Formulas
One of the fastest ways to improve calculation speed is by committing important formulas to memory. Knowing essential equations like area, perimeter, and volume formulas for various shapes allows you to quickly apply them when needed without having to reference them repeatedly.
- Area of a Triangle: 1/2 × base × height
- Area of a Circle: π × radius²
- Volume of a Cylinder: π × radius² × height
Use Estimation to Save Time
Estimation is a powerful tool for quickly solving problems, especially when exact answers aren’t necessary. Estimating values before performing detailed calculations can help you narrow down possible answers and save time by focusing on more likely solutions.
- Round numbers: Round numbers to easier values before performing the calculation, then adjust your result as needed.
- Look for simple patterns: Identifying patterns or symmetries in the problem can allow you to skip certain steps or make quicker assumptions.
By mastering these techniques and practicing regularly, you’ll increase your speed and efficiency, allowing you to tackle even the most challenging problems with greater confidence.
Practical Applications of Geometry in Daily Life
The principles of shapes, sizes, and spatial relationships are not limited to classrooms or textbooks. In fact, these concepts play a crucial role in various aspects of everyday life. Understanding and applying these ideas can make tasks more efficient and help us make informed decisions in real-world situations.
1. Architecture and Design
Whether it’s building a house, designing furniture, or creating a new public space, geometric concepts are essential. Architects and designers rely on precise measurements, angles, and proportions to create functional and aesthetically pleasing structures. These concepts help in:
- Determining the correct dimensions for rooms and furniture.
- Creating accurate blueprints for construction projects.
- Ensuring balance and symmetry in design elements.
2. Navigation and Mapping
When navigating the world, we rely on a variety of geometric concepts. From reading maps to using GPS, understanding the relationships between points, distances, and directions is key to getting from one place to another. Some examples include:
- Using coordinates to pinpoint locations on maps.
- Understanding angles and distances for calculating routes.
- Using geometric shapes to plan out the most efficient paths.
3. Everyday Activities
Even in daily routines, geometric concepts help simplify tasks. From cooking to shopping, geometry is at play:
- Measuring ingredients accurately based on volume or weight.
- Calculating the area for painting walls or laying flooring.
- Estimating quantities when buying fabric or wallpaper based on surface area.
By recognizing the practical applications of these ideas, we can see how essential they are in our daily activities. Mastering the fundamentals allows for more efficient and effective decision-making in various contexts.
Using Geometry Tools for Accurate Solutions
Precision is key when solving problems that involve shapes, sizes, and measurements. Using the right tools can make a significant difference in achieving accurate results, whether you are working on complex tasks or simple calculations. The proper instruments help you to measure, draw, and calculate with greater efficiency and precision, reducing the chances of error.
Essential Tools for Accurate Measurement
There are several tools designed specifically for working with geometric problems. These instruments ensure that your measurements are precise and your drawings are correct. Some of the most common tools include:
- Ruler: A basic tool used to measure straight lines accurately.
- Protractor: Helps in measuring and drawing angles with precision.
- Compass: Useful for creating circles and arcs with exact radii.
- Calipers: Ideal for measuring distances between two points, especially in more detailed work.
Techniques for Using These Tools Effectively
Merely having the right tools is not enough; understanding how to use them properly is equally important. Here are a few techniques to ensure you are using these instruments effectively:
- Check calibrations: Always ensure that tools like protractors and rulers are correctly calibrated to avoid small errors.
- Marking clearly: Use light, precise markings that can be adjusted if necessary but are still visible enough for accurate measurements.
- Work slowly: Take your time when measuring and drawing. Speed can lead to mistakes, so it’s better to proceed carefully and double-check your results.
With practice, you can become more proficient in using these tools, allowing you to solve problems more efficiently and with greater accuracy.
How to Review Your Answers in Geometry
After completing a set of problems or calculations, reviewing your work is a crucial step in ensuring the accuracy of your solutions. It allows you to spot errors, improve your understanding, and reinforce your knowledge. The process of checking your results should be methodical and systematic, helping you identify any mistakes and correct them before finalizing your work.
Steps for Effective Review
Here are some essential steps to follow when reviewing your results:
- Step 1: Verify the accuracy of your calculations by retracing your steps. Ensure that each operation, whether addition, subtraction, or multiplication, has been performed correctly.
- Step 2: Recheck your work by comparing the logic behind each solution. Ask yourself whether the approach makes sense and if there is a simpler method to achieve the same result.
- Step 3: Double-check all measurements, angles, and geometric properties involved. Ensure that the dimensions match the problem’s requirements.
Common Errors to Watch For
While reviewing your work, it’s important to keep an eye out for common mistakes that often occur in geometric problems:
| Error Type | What to Look For |
|---|---|
| Calculation Mistakes | Recheck arithmetic operations and make sure all steps are correct. |
| Misunderstanding of Formulas | Ensure that you have used the correct formulas and applied them properly. |
| Incorrect Units | Verify that all measurements and units are consistent with the problem’s parameters. |
| Logical Errors | Check if the reasoning behind each step is sound and follows a logical sequence. |
By carefully reviewing your answers, you can enhance the accuracy of your work and ensure that you haven’t overlooked any important details.
Improving Your Skills with Practice
Mastering any subject requires continuous effort and regular exercises to reinforce what has been learned. This holds true when working with mathematical concepts that involve shapes, angles, and calculations. By practicing consistently, you not only improve your understanding of core principles but also develop the ability to solve problems faster and more accurately. Regular engagement with exercises is essential for mastering the material and building confidence in your problem-solving abilities.
Effective Techniques for Practice
There are several strategies you can use to enhance your skill set and become more proficient:
- Repetition: Repeat key problems and challenges to build muscle memory and reinforce the processes you’ve learned.
- Challenge Yourself: Tackle problems that push your boundaries, gradually increasing the level of difficulty.
- Review Mistakes: Analyze where you went wrong to understand your mistakes and avoid repeating them.
- Use Different Approaches: Experiment with various methods and strategies to find the most effective one for you.
How Consistent Practice Leads to Improvement
Engaging in regular exercises offers numerous benefits. Here’s how it helps:
- Builds Confidence: As you solve more problems, you will naturally gain confidence in your abilities.
- Improves Speed: With consistent practice, you will become faster at solving problems, reducing the time it takes to arrive at a solution.
- Strengthens Understanding: Practicing regularly helps solidify your understanding of the underlying principles and formulas.
- Prepares for Advanced Concepts: Once you master foundational skills, you’ll be better prepared to tackle more complex topics.
Incorporating regular exercises into your study routine is an excellent way to enhance your problem-solving skills and deepen your understanding of the subject matter.
The Role of Mathematics in Higher Education
Mathematical concepts form the foundation of many fields of study in higher education. Understanding spatial relationships, measurements, and the properties of shapes and structures is crucial for students pursuing a wide range of disciplines. From architecture to engineering, economics to computer science, mathematical knowledge helps students think critically, solve complex problems, and analyze patterns that are essential for their academic and professional success.
Applications in Various Disciplines
Mathematics is not confined to one area of study. Instead, it provides key insights and tools across various academic fields. Here are a few examples:
- Engineering: The study of structural design, mechanics, and system dynamics often relies heavily on advanced mathematical techniques.
- Architecture: Precision in measurements and understanding of spatial relationships are critical in designing functional and aesthetically pleasing structures.
- Economics: Mathematical models help economists forecast trends, calculate risk, and analyze financial data to make informed decisions.
- Computer Science: Algorithms, data structures, and coding practices all benefit from a strong understanding of mathematical principles.
- Physics: The understanding of motion, forces, and energy requires mathematical concepts that describe how the physical world works.
Enhancing Problem-Solving and Critical Thinking
The study of mathematical principles sharpens problem-solving and analytical skills, which are highly valued in many fields. Here are some benefits of learning mathematics in higher education:
- Logical Thinking: Mathematics teaches students how to approach problems systematically, using logical reasoning to find solutions.
- Analytical Skills: Students develop the ability to analyze data and interpret results, which is essential in research and technical fields.
- Attention to Detail: Mathematical study encourages students to pay close attention to every step, reducing errors and improving accuracy.
- Innovation: As students apply mathematical concepts to real-world problems, they are often inspired to create new solutions and innovative approaches.
In conclusion, mathematics plays a pivotal role in higher education, providing students with the tools they need to succeed academically and professionally. Its impact stretches across disciplines, equipping future leaders with critical thinking skills and the ability to navigate complex challenges.
How Geometry Shapes Critical Thinking
Mathematical thinking, particularly through the study of spatial relationships and shapes, has a profound impact on one’s ability to analyze problems logically and systematically. By exploring the properties and structures of various forms, individuals develop skills that extend beyond mere calculations, fostering a deeper understanding of complex concepts and sharpening their decision-making abilities. This discipline encourages learners to break down problems into smaller, more manageable parts and think creatively to solve them effectively.
Building Logical and Analytical Skills
The process of solving spatial puzzles and visualizing complex structures helps students refine their logical reasoning and analytical skills. By regularly engaging with this type of problem-solving, learners strengthen their capacity for:
- Structured Thinking: Approaching problems step-by-step fosters a disciplined method of analysis, ensuring that all relevant factors are considered.
- Pattern Recognition: The ability to identify recurring patterns within different situations enhances one’s ability to predict outcomes and understand underlying relationships.
- Abstract Reasoning: Manipulating abstract concepts such as shapes, sizes, and angles allows individuals to apply these ideas in real-world contexts.
Improving Problem-Solving Abilities
Engaging with spatial challenges trains individuals to think critically when confronted with new problems. Some of the key problem-solving benefits include:
- Creative Solutions: The need to think outside the box when visualizing and manipulating objects leads to innovative approaches in tackling new challenges.
- Attention to Detail: Analyzing shapes and measurements fosters an acute awareness of detail, which is essential for precise and accurate conclusions.
- Flexibility in Approach: Learning to view problems from multiple angles strengthens adaptability and the ability to pivot between different solutions.
Ultimately, by engaging with these types of cognitive exercises, individuals enhance their capacity to think critically, make informed decisions, and approach complex problems with confidence and creativity.
Resources for Further Geometry Practice
To enhance your understanding and proficiency in spatial reasoning, it’s important to utilize various tools and materials that offer a diverse range of exercises. These resources can support ongoing learning and help refine skills by providing challenges of increasing difficulty, along with explanations that foster deeper comprehension. Engaging with such materials regularly can build a strong foundation and promote mastery in handling complex problems.
Online Platforms and Tutorials
There are numerous online resources that offer interactive lessons, videos, and exercises aimed at improving your problem-solving abilities. These platforms often provide instant feedback, which can be particularly helpful in identifying areas that require more attention. Some popular websites include:
| Platform | Features | Best For |
|---|---|---|
| Khan Academy | Free lessons, quizzes, and video tutorials | Beginner to advanced learners |
| Brilliant.org | Interactive problems and in-depth courses | Problem-solving practice and concept mastery |
| IXL | Skill-based practice with immediate feedback | Comprehensive learning for all levels |
Books and Printable Worksheets
For those who prefer offline resources, there are a wide range of workbooks and printable exercises that can be helpful. These materials provide step-by-step problems and solutions, making it easier to work through challenges without the need for constant internet access. Recommended books include:
- “The Art of Problem Solving” by Richard Rusczyk: A series of books that teach mathematical thinking through challenging problems and detailed solutions.
- “Elementary Mathematics for Teachers” by Parker and Baldridge: A comprehensive guide with problems and examples that improve logical thinking and numerical reasoning.
- “Math Olympiad Contest Problems” by George Lenchner: A great collection of problems that test and expand critical thinking and spatial reasoning skills.
By using these resources regularly, you’ll be able to strengthen your skills and increase your confidence in solving complex problems.
Final Thoughts on Lesson 6.3 Practice A
As you work through the exercises and apply the concepts learned, it’s crucial to reflect on the strategies used and the methods that helped solve each problem. Consistent practice and a focused approach to understanding the underlying principles are key to mastering the material. It’s not only about finding the correct solution, but also about understanding the reasoning behind it. This type of problem-solving builds strong analytical skills and improves overall comprehension.
Remember that tackling complex challenges becomes easier with time and practice. Keep revisiting different types of problems and breaking them down into manageable steps. Over time, this will enhance both your confidence and your ability to handle even more advanced topics. Whether you’re working independently or reviewing with peers, the process of continuous improvement is essential for long-term success.