
Preparing for a challenging math assessment requires not only practice but also a deep understanding of core principles. By reviewing past tests and analyzing the structure of various questions, students can improve both their problem-solving skills and test-taking strategies.
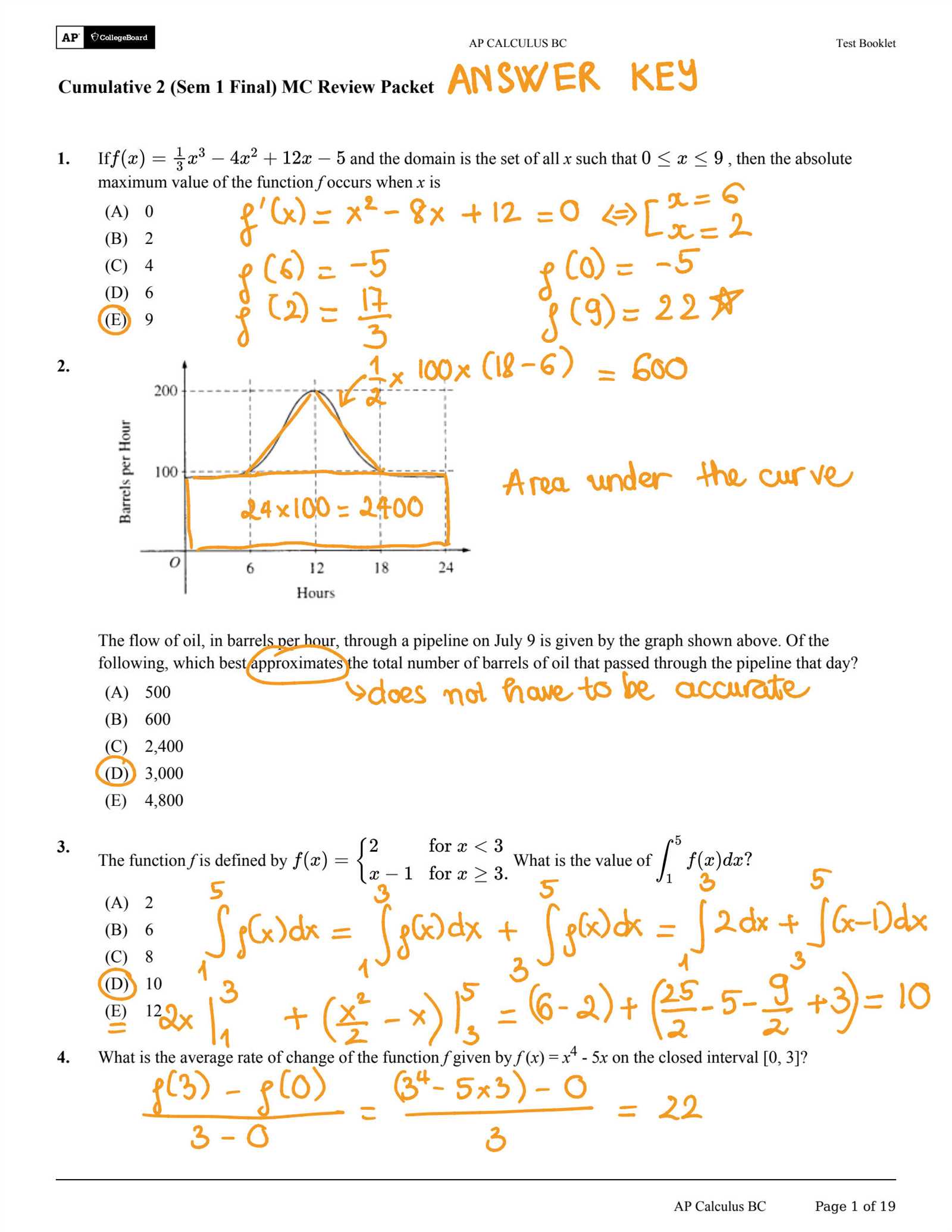
In this section, we focus on key mathematical problems and provide step-by-step solutions. Through this process, you’ll be able to identify the most effective methods for solving complex tasks and learn how to approach different types of questions with confidence.
We will also highlight common mistakes and provide insights into how to avoid them. With the right approach, you can enhance your ability to navigate through mathematical challenges and achieve a strong performance in any assessment.
Comprehensive Guide to AP Calculus AB Exam
Success in advanced mathematics assessments requires a clear understanding of both fundamental concepts and complex problem-solving techniques. To excel in these tests, students must not only recall mathematical rules but also know how to apply them effectively under time constraints. This guide is designed to offer insights into the structure of such tests and provide strategies to approach each type of question with confidence.
We will cover a range of topics that frequently appear in these evaluations, from derivatives to integrals, and explain how to tackle various question formats. Each section will break down the core principles, offering a structured approach to mastering each area of study.
By thoroughly reviewing sample questions and solutions, students will gain a deeper understanding of the types of problems they are likely to encounter. This preparation will not only help in performing well but also in developing a methodical approach to any math challenge.
Overview of the 2012 AP Calculus AB Exam
The assessment in question is designed to evaluate a student’s ability to apply advanced mathematical concepts in a structured and time-constrained environment. It tests both theoretical knowledge and practical skills, requiring students to demonstrate their understanding through a series of multiple-choice and open-ended questions. The challenge lies not only in knowing the material but in being able to efficiently apply it to solve complex problems under pressure.
Structure and Format
The test is divided into two main sections: one focusing on multiple-choice questions and the other on free-response tasks. The multiple-choice section assesses students’ ability to quickly solve problems and identify correct solutions, while the free-response portion requires detailed, step-by-step solutions that show a deeper understanding of the concepts being tested.
Key Topics Covered
This evaluation spans a broad range of mathematical topics, including limits, derivatives, integrals, and the application of these concepts in real-world contexts. Students should be well-versed in both theoretical foundations and practical problem-solving strategies to perform effectively across all sections of the test.
Key Topics Covered in the 2012 Exam

The assessment evaluates a wide range of mathematical principles, each requiring a deep understanding and the ability to apply various techniques. In this section, we will explore the main areas tested, which include both foundational concepts and their practical applications. Mastery of these topics is essential for achieving a high score and excelling in the assessment.
Fundamental Concepts
- Limits – Understanding how functions behave as they approach certain values.
- Derivatives – Techniques for calculating rates of change and understanding the behavior of curves.
- Integrals – Applying methods to calculate areas under curves and solve problems related to accumulation.
Applied Topics
- Applications of Derivatives – Using derivatives to solve real-world problems, such as optimization and motion analysis.
- Applications of Integrals – Utilizing integrals to model and solve problems involving area, volume, and accumulation.
- Approximation Methods – Techniques like numerical integration and the approximation of complex functions.
Strategies for Tackling Multiple Choice Questions
Multiple-choice questions often require quick thinking and the ability to eliminate incorrect options. With limited time, it is crucial to develop effective strategies that help you identify the correct answer and avoid common pitfalls. This section will explore key techniques to approach these questions confidently and efficiently.
Effective Techniques
| Strategy | Description |
|---|---|
| Elimination | Start by eliminating obviously incorrect answers, narrowing your choices to improve the odds of selecting the right option. |
| Guess Smart | If unsure, make an educated guess by considering which answers align best with your knowledge of the subject. |
| Check for Key Words | Look for specific terminology or phrases in the question that directly correspond to the correct answer. |
| Manage Your Time | Avoid spending too much time on a single question. Move on if needed and return later if time allows. |
Practice and Preparation
Regular practice is essential for mastering multiple-choice questions. By solving sample problems and familiarizing yourself with common question patterns, you will be better prepared to navigate through them quickly and accurately. This preparation will not only help you become more efficient but also boost your confidence during the test.
Understanding the Free-Response Section
The free-response portion of a test is designed to assess your ability to solve complex problems and demonstrate a thorough understanding of the material. Unlike multiple-choice questions, which focus on selecting the correct answer from a set of options, free-response questions require you to show your work, explain your reasoning, and arrive at a solution independently. This section tests your problem-solving skills and your ability to communicate mathematical ideas clearly.
Key Strategies for Success
- Show All Steps – Always write down each step of your solution. This will not only help you organize your thoughts but also ensure you earn partial credit if you make a mistake.
- Answer the Question Fully – Read the question carefully and ensure that you answer all parts. Be sure to address every aspect of the problem, as partial answers may lead to partial credit.
- Use Clear Notation – Clear, well-organized work is essential. Avoid messy calculations and use appropriate symbols to represent your work accurately.
- Check Your Work – If time allows, review your solutions for any errors or missed steps before submitting your answers.
Common Question Types
- Application Problems – These questions typically involve applying mathematical concepts to real-world scenarios or theoretical situations.
- Proofs and Justifications – In these questions, you are asked to explain the reasoning behind a given solution or to prove a mathematical statement.
- Graphing and Interpretation – These questions may require you to graph a function or interpret the behavior of a graph in the context of the problem.
Review of Important Calculus Concepts
To excel in any mathematical assessment, it is essential to have a strong foundation in the core concepts. This section will revisit key ideas that are fundamental to understanding how mathematical models describe the world around us. Mastery of these concepts allows students to solve complex problems and approach advanced topics with confidence.
Essential Concepts for Problem Solving
- Limits – Understanding how a function behaves as it approaches a particular value is crucial for grasping many advanced concepts.
- Derivatives – These describe the rate of change of a function, which is vital for solving optimization problems and analyzing motion.
- Integrals – Integrals are used to calculate areas, volumes, and accumulated quantities, making them a key tool for solving a wide range of problems.
Application of Key Principles

- Chain Rule – A method for differentiating composite functions, helping solve more complex derivative problems.
- Fundamental Theorem of Calculus – This theorem connects differentiation and integration, providing the foundation for many important results.
- Integration by Parts – A technique used to integrate products of functions, commonly applied in physics and engineering problems.
Detailed Solutions for 2012 Exam Questions
This section provides in-depth explanations of the solutions to key questions from the assessment, offering step-by-step breakdowns for each problem. Understanding how to approach and solve these questions is essential for mastering the material and improving your performance in similar future tests. By reviewing detailed solutions, you will gain insights into effective problem-solving strategies and learn the proper techniques for applying mathematical principles to different types of questions.
Step-by-Step Solution Approach
- Identify the Problem Type – The first step in solving any question is to understand the type of problem you’re dealing with, whether it’s about optimization, area calculation, or rate of change.
- Apply Core Concepts – Use the fundamental principles that relate to the question, such as limits, derivatives, or integrals, to find the appropriate solution method.
- Show Work Clearly – Step through each part of the solution methodically. Clearly label each step and justify every operation to ensure that your reasoning is transparent.
- Check for Accuracy – After solving the problem, revisit each step to confirm the calculations are correct, and make sure that the final answer is consistent with the problem’s conditions.
Example Breakdown
- Question 1: Optimization Problem – First, identify the equation to optimize, then set up the derivative and solve for critical points. Check for maximum or minimum values using the second derivative test.
- Question 2: Area under a Curve – Set up the integral using the correct limits and function. Perform the integration, simplifying the result to find the area.
- Question 3: Rate of Change – Apply the concept of the derivative to find the rate of change of a function at a specific point. Use the correct formula and substitute the values to obtain the result.
Common Mistakes to Avoid in AP Calculus
When tackling advanced mathematical problems, it is easy to make errors that can derail the entire solution process. Understanding the common mistakes students make can help you avoid them and ensure that your reasoning and calculations are accurate. In this section, we’ll highlight the key pitfalls and provide tips on how to prevent them, allowing you to approach each problem with confidence.
Frequent Calculation Errors
- Incorrect Application of Formulas – Be careful when applying formulas, especially in derivative or integral calculations. Make sure to understand the underlying principles and not just memorize the steps.
- Sign Errors – A common mistake is failing to track negative signs during operations, especially when dealing with powers, roots, or subtraction in equations.
- Misinterpretation of Functions – When dealing with composite functions or piecewise functions, always ensure you’re applying the correct interpretation to the given information.
Logical and Conceptual Mistakes
- Overlooking Key Assumptions – Many problems rely on initial conditions or assumptions that, if ignored, can lead to incorrect conclusions. Always recheck the problem statement to ensure you’ve considered all conditions.
- Skipping Steps – Rushing through problems can lead to skipped steps, which may seem minor but can often lead to incorrect answers. Always show every part of the process, even if you think it’s obvious.
- Failing to Check Results – After arriving at an answer, it’s essential to verify that it makes sense in the context of the problem. If your result seems out of place, retrace your steps to check for errors.
Tips for Time Management During the Exam
Managing your time effectively during a high-stakes assessment is crucial for ensuring that you have enough opportunity to tackle every question. Procrastination or spending too long on one problem can leave you with insufficient time for others. In this section, we’ll share strategies to help you pace yourself and approach the test methodically, allowing you to perform at your best while keeping track of time.
Effective Strategies to Stay on Track
- Familiarize Yourself with the Test Format – Before the test, understand the structure and types of questions you’ll face. Knowing how much time to allocate to each section will help you manage your time more effectively.
- Set Time Limits for Each Section – Break the test into smaller parts, and set strict time limits for each. Stick to these limits, moving on if you find yourself getting stuck on a question.
- Prioritize Questions – Start with the questions that you find easiest or most familiar. This will help you build confidence and save time for more challenging problems.
How to Maximize Time Efficiency
- Skip and Return to Difficult Questions – If you encounter a particularly challenging question, skip it and move on. You can always return to it once you’ve finished the easier ones, ensuring you don’t lose valuable time.
- Keep an Eye on the Clock – Regularly check the time to stay on pace. This will help prevent you from spending too long on any one section.
- Leave Time for Review – Ensure that you leave some time at the end to go over your answers. This will allow you to check for any errors and make corrections if necessary.
How to Approach Integration Problems
Integration problems can often seem daunting, but with the right approach, they become manageable and systematic. Whether you’re dealing with basic functions or more complex expressions, understanding the fundamental strategies will help you tackle these questions with confidence. This section outlines essential techniques for approaching integration problems efficiently and accurately.
Key Steps to Solve Integration Problems
- Identify the Type of Integral – Start by recognizing what kind of problem you’re facing: is it a simple polynomial, a rational function, or a more complex expression requiring substitution or integration by parts?
- Look for Substitution Opportunities – When encountering complex expressions, consider substitution as a way to simplify the integral. This technique is useful when you can recognize a derivative inside the integral.
- Consider Special Formulas – For certain common functions like trigonometric or exponential, there are standard formulas that can be applied directly. Recognizing these can save time and avoid unnecessary steps.
Common Techniques for Integration
- Substitution – If a function is composed of a simpler function and its derivative, substitution can help simplify the integral. Make sure to properly adjust limits or constants when needed.
- Integration by Parts – When dealing with the product of two functions, the integration by parts method is often useful. It’s based on the formula ∫u dv = uv – ∫v du, which allows for breaking down the problem into manageable parts.
- Partial Fractions – For rational functions, decomposing the integrand into partial fractions can help reduce the problem to simpler integrals.
Maximizing Your Score on the AP Exam

Achieving a high score on a rigorous assessment requires not only mastering the content but also employing effective strategies to showcase your knowledge under timed conditions. In this section, we’ll discuss key tactics that can help you maximize your performance, manage your time wisely, and approach each section with confidence. By preparing strategically and staying focused, you can significantly improve your score.
Preparation Strategies for Success

- Review Core Concepts Thoroughly – Focus on understanding the fundamental principles and techniques that form the basis of the test. Make sure you can apply these concepts in various contexts and problem types.
- Practice Regularly – Consistent practice is essential to reinforce what you’ve learned. Work through sample problems, review past questions, and try to identify patterns in the types of questions that frequently appear.
- Take Timed Practice Tests – Simulating real test conditions can help you improve your time management skills and reduce anxiety. Practice under timed conditions to ensure you can work efficiently during the actual test.
Effective Test-Taking Strategies
- Start with the Easiest Questions – Begin with the questions you find most familiar and manageable. This approach helps you build momentum and saves time for the more challenging problems.
- Don’t Get Stuck on Difficult Questions – If you encounter a difficult problem, move on and return to it later. Spending too much time on one question can hurt your overall performance.
- Review Your Work – If time permits, always go back and check your answers. Look for common errors like sign mistakes or missed steps that could cost you valuable points.
Study Resources for AP Preparation
To effectively prepare for a challenging assessment, it’s important to use a variety of study resources. Whether you prefer books, online platforms, or interactive tools, the right materials can enhance your understanding and performance. In this section, we will explore different types of study resources that will help you strengthen your skills and increase your chances of success.
Books and Study Guides
- Textbooks and Course Materials – Your course materials are essential for a strong foundation. Review the chapters, practice problems, and explanations provided by your textbook to reinforce key concepts.
- Review Guides – Many publishers offer comprehensive review guides that break down the material into manageable sections, highlight important formulas, and provide practice questions with detailed solutions.
- Prep Books – Books from well-known test prep companies such as Barron’s, Princeton Review, or Kaplan often include full-length practice tests, tips, and strategies to help you succeed.
Online Resources

- Official Websites – The College Board website offers free resources, including sample questions and test-taking tips. It’s a reliable source for understanding the format and structure of the assessment.
- Interactive Platforms – Websites like Khan Academy provide instructional videos, practice exercises, and explanations, which can be helpful for visual and hands-on learners.
- Online Forums and Study Groups – Engage with online communities like Reddit or specialized Facebook groups to discuss difficult concepts, share study tips, and access additional resources from fellow students.
Other Helpful Resources
- Flashcards – Using flashcards to memorize key concepts, formulas, and important definitions can be an effective way to reinforce learning and improve recall.
- Tutoring Services – If you need extra help, consider working with a tutor or attending study sessions for personalized guidance.
- Mobile Apps – Various apps are available to help you practice problem-solving, time management, and recall. Apps like Wolfram Alpha can help you solve and understand complex problems step-by-step.
Common Pitfalls in the 2012 Assessment
When preparing for a challenging test, it’s essential to be aware of common mistakes that many students make. These errors can significantly impact your performance and understanding. By identifying these pitfalls, you can take proactive steps to avoid them and approach the test with greater confidence. In this section, we will review some of the most frequently encountered issues during the 2012 assessment, helping you be better prepared for what lies ahead.
One common issue is misinterpreting the questions or not fully understanding what is being asked. Often, students rush through questions without carefully reading the instructions or identifying key details, leading to unnecessary errors. Another common mistake is not managing time effectively. Many students spend too much time on difficult problems at the expense of easier ones, leaving themselves with insufficient time to complete all sections.
Additionally, incorrect application of formulas or concepts is a frequent issue. Even if students know the correct method, they may apply it improperly, which leads to wrong answers. Lastly, some students neglect to check their work. Not reviewing answers can lead to simple calculation mistakes or overlooked errors, which are easy to avoid with a quick check at the end.
Breaking Down the Derivative Questions
Derivative-related questions often require a deep understanding of how to analyze and manipulate functions. These questions assess your ability to apply various rules and techniques to determine the rate of change or slope of a function at a specific point. Mastery of these concepts is crucial for success in any assessment that involves such problems. In this section, we will break down key strategies to tackle these types of questions effectively.
To begin with, it’s essential to recognize the different types of problems you may encounter, such as finding the derivative of a given function or interpreting the meaning of a derivative in a real-world context. For straightforward problems, recall the fundamental rules such as the power rule, product rule, and quotient rule. For more complex problems, don’t forget the chain rule, which is often a game-changer when dealing with composite functions.
Another important aspect is the interpretation of the results. Once you calculate the derivative, it’s crucial to understand its significance. Does the derivative represent a slope? Is it related to the velocity of an object? Understanding the context of the problem helps you avoid common mistakes and apply your knowledge appropriately.
Finally, always double-check your calculations. The smallest mistake in differentiation can lead to significant errors in the final answer, so it’s important to take the time to verify each step. With these strategies in mind, you will be well-equipped to approach derivative-related questions with confidence and precision.
Important Theorems to Remember for the Exam
Understanding and memorizing key theorems is crucial for performing well in any assessment that covers advanced mathematical concepts. These theorems serve as the foundation for many problems and are often directly applied to solve a variety of questions. In this section, we will review some of the most essential theorems that you should have at your fingertips for any such test. Having a solid grasp of these principles will not only help you solve problems quickly but also increase your confidence when approaching challenging topics.
The Fundamental Theorem of Calculus

This theorem establishes the deep connection between differentiation and integration. It has two parts: the first part states that an antiderivative of a continuous function can be used to calculate a definite integral, and the second part allows you to compute the value of a definite integral using an antiderivative of the integrand. Understanding how these two operations are related is vital for solving many types of problems involving integrals and derivatives.
The Mean Value Theorem
The Mean Value Theorem is a powerful tool for understanding the behavior of functions over an interval. It states that for any continuous function that is differentiable over a closed interval, there exists at least one point where the instantaneous rate of change (derivative) is equal to the average rate of change over the interval. This theorem is often useful when solving problems related to motion or optimization.
| Theorem | Key Concept | Application |
|---|---|---|
| Fundamental Theorem of Calculus | Connects differentiation and integration | Calculating definite integrals and finding antiderivatives |
| Mean Value Theorem | Relates the average rate of change to instantaneous rate | Solving problems involving motion and rate of change |
By focusing on these theorems and their applications, you’ll be better equipped to handle problems that test your understanding of mathematical principles. Keep practicing their use in various contexts to ensure that you’re prepared for anything that might appear on the test.
Best Practices for Exam Day Success
Success on test day is not just about what you know, but how you manage your time, approach the questions, and maintain focus throughout the assessment. Preparation leading up to the day is important, but there are key strategies you can implement on the actual test day to ensure you’re performing at your best. By following these best practices, you can reduce stress and increase your chances of success.
| Strategy | Why It Works | How to Apply It |
|---|---|---|
| Get Enough Rest the Night Before | A well-rested mind performs better and retains information more effectively. | Aim for 7-8 hours of sleep the night before to be mentally alert during the test. |
| Eat a Healthy Breakfast | A balanced meal fuels your brain and keeps energy levels stable. | Eat a breakfast with protein, whole grains, and fruits to sustain you throughout the test. |
| Arrive Early | Arriving early helps to reduce anxiety and gives you time to settle in. | Plan to arrive at least 15 minutes before the start to get comfortable and focused. |
| Read Instructions Carefully | Understanding the requirements of each question prevents costly mistakes. | Take a moment to read through each question and its instructions thoroughly before answering. |
| Manage Your Time Wisely | Effective time management ensures that you have enough time for all questions. | Divide your time according to the number of questions and prioritize difficult ones later. |
| Stay Calm and Focused | A calm mindset enhances problem-solving ability and minimizes errors. | If you feel stressed, take a deep breath, pause for a moment, and refocus. |
By integrating these strategies into your routine, you’ll be better prepared to tackle the challenges of the assessment with confidence and clarity. Keep a positive mindset, manage your time efficiently, and stay focused on each question to maximize your potential on the day of the test.