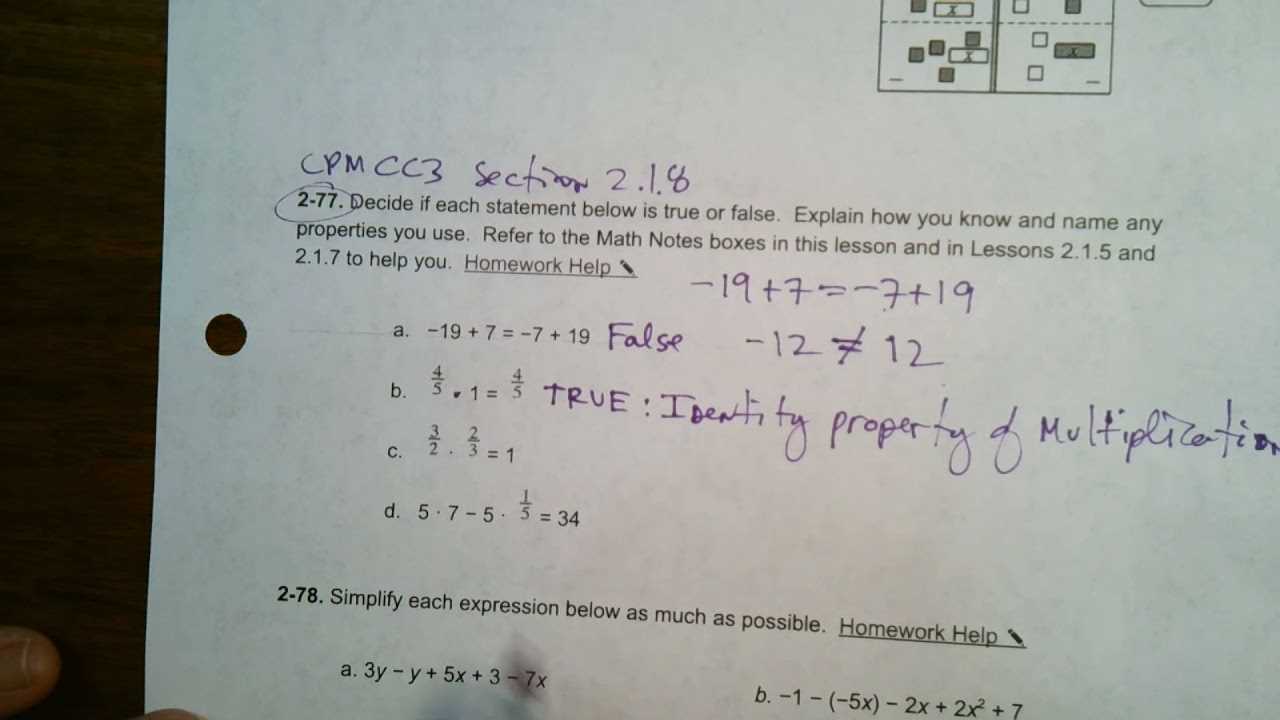
In this section, we explore fundamental strategies for tackling complex mathematical problems. By breaking down each problem into manageable steps, you can gain a clearer understanding of how to approach and solve various challenges. This approach focuses on building critical thinking skills and applying logical reasoning to find accurate solutions.
Understanding the problem-solving process is key to success. Whether you’re dealing with abstract concepts or real-world applications, grasping the underlying principles can greatly enhance your ability to find correct solutions. Each exercise is designed to help you refine your skills and apply them in different contexts.
As you progress, consistency and practice are essential. The more problems you solve, the more familiar you become with common patterns and techniques. This continuous improvement helps solidify your knowledge and boosts confidence in your problem-solving abilities.
CPM Chapter 3 Answers Overview
This section provides a comprehensive look at problem-solving techniques and strategies for tackling the exercises presented in the third unit. The goal is to guide learners through the most effective methods for addressing the tasks, ensuring clarity and precision in finding solutions. By analyzing each question step by step, students can build a solid foundation of skills to apply to similar challenges.
The key to success lies in understanding the approach to each problem. Rather than focusing solely on memorizing formulas or steps, it’s important to develop a deep understanding of the logic behind each solution. This enables learners to adapt their skills to a variety of mathematical situations and enhances their problem-solving capabilities.
Throughout this overview, you will encounter numerous examples that illustrate common techniques and potential pitfalls. By reviewing these examples, you will gain insight into how to approach various types of problems and sharpen your analytical thinking, which is essential for mastering the content of this section.
Key Concepts in CPM Chapter 3
In this section, we focus on the foundational principles that drive the problem-solving techniques explored in the unit. Mastering these concepts is essential for tackling the more complex exercises and understanding how different mathematical ideas interconnect. The goal is to provide a strong theoretical base that students can apply to a variety of problems.
One of the core ideas is recognizing patterns and relationships between numbers, which allows for more efficient problem solving. Understanding how to manipulate variables and equations in different ways can simplify complex tasks and lead to quicker solutions. Moreover, developing an understanding of geometric principles plays a crucial role in solving spatial problems effectively.
Equally important is the ability to break down problems into smaller, more manageable parts. This technique helps prevent feeling overwhelmed and allows for a more systematic approach to finding the correct solution. By mastering these fundamental concepts, learners can approach more advanced topics with greater confidence and clarity.
Step-by-Step Guide to Solving Problems
To solve mathematical problems effectively, it’s essential to follow a structured approach. This guide will break down the steps involved in tackling each exercise, ensuring clarity and precision. By following these steps, learners can improve their problem-solving skills and approach challenges with confidence.
Step 1: Understand the Problem
The first step is to carefully read and analyze the problem. Ensure that you understand what is being asked before proceeding. Pay attention to key information such as numbers, variables, and units. It’s also helpful to underline or highlight important details.
Step 2: Plan Your Approach
Once you understand the problem, decide on the best method to solve it. This might involve identifying relevant formulas, equations, or strategies that will help you find the solution. Having a plan in place will streamline the process and reduce mistakes.
Step 3: Solve the Problem
Apply the chosen method to solve the problem. Be sure to work through each part of the equation or steps logically. If you encounter difficulties, consider breaking the problem into smaller pieces and solving each part individually.
Step 4: Check Your Work
After completing the problem, review your work to ensure accuracy. Verify each step, check calculations, and ensure that your final answer makes sense in the context of the question. If necessary, revisit earlier steps to identify and correct any mistakes.
Key Tips for Efficient Problem Solving
- Always start with the given information and identify what needs to be found.
- Write out each step clearly to avoid confusion or mistakes.
- Don’t rush–take your time to think through the solution process.
- Use diagrams or sketches where applicable to better visualize the problem.
- If stuck, refer back to similar problems or ask for clarification if needed.
Understanding Common Mistakes in CPM
As you work through mathematical exercises, it’s important to recognize and avoid common errors that can hinder progress. Identifying these mistakes early on will help prevent frustration and improve your accuracy. By understanding where students typically go wrong, you can take steps to avoid making the same errors.
One of the most frequent mistakes is misinterpreting the problem. Often, students overlook key details or fail to fully understand what is being asked. Another common error is rushing through calculations without checking for accuracy, leading to simple yet avoidable mistakes.
Common Mistakes and How to Avoid Them

- Misreading the Question: Always read the problem carefully. Highlight or underline important details to ensure you don’t miss crucial information.
- Skipping Steps: It’s tempting to skip steps when you’re confident, but this can lead to mistakes. Write down each step clearly and check your work as you go.
- Incorrect Application of Formulas: Ensure you understand the formula you’re using and apply it correctly. Double-check whether each variable is properly represented.
- Not Checking Your Work: After solving a problem, take time to verify your calculations and ensure your solution makes sense. Small errors often go unnoticed without careful review.
- Overlooking Units: Pay attention to units and ensure that they are consistent throughout the problem. Converting units incorrectly can lead to incorrect solutions.
How to Minimize Errors
- Read each problem multiple times to ensure you fully understand it.
- Break problems into smaller parts to avoid feeling overwhelmed.
- Review each step before moving on to the next one.
- Practice regularly to become familiar with common patterns and solutions.
- Ask for help or refer to resources when needed to clarify any confusion.
Tips for Mastering Chapter 3 Questions
To excel at solving problems in this unit, it’s essential to adopt effective strategies that will enhance your understanding and performance. By focusing on key techniques and regularly practicing problem-solving skills, you can develop a solid grasp of the material and approach each question with confidence. Here are some valuable tips to help you master the exercises presented in this section.
Key Tips for Success
| Tip | Description |
|---|---|
| Understand the Fundamentals | Ensure you have a clear understanding of the basic concepts before attempting more complex problems. Build a strong foundation to tackle the more challenging tasks effectively. |
| Break Problems Into Steps | Divide each problem into smaller, manageable parts. This helps you focus on one element at a time and avoid becoming overwhelmed by the complexity of the entire question. |
| Review Examples | Look over solved examples to understand the process and logic used in reaching the solution. This can provide valuable insight into how to approach similar problems. |
| Practice Regularly | The more you practice, the more familiar you become with the types of problems and solutions. Regular practice helps reinforce concepts and solidifies your understanding. |
| Check Your Work | Always double-check your calculations and logic to catch any mistakes. Reviewing your answers ensures that you haven’t missed anything crucial. |
Additional Strategies
- Take notes and highlight key points to keep track of important details.
- Use visual aids like diagrams and charts to help understand spatial relationships in problems.
- Ask questions when you’re unsure, either to peers or instructors, to clarify any confusion.
- Stay organized and avoid rushing through tasks. Thoroughness is key to achieving accurate results.
Commonly Asked Questions in Chapter 3
As students work through the exercises in this section, they often encounter similar questions or face certain challenges that require additional explanation. Understanding these frequently asked questions can help clarify common points of confusion and provide helpful guidance for tackling problems more effectively. Here are some of the most commonly asked questions and their solutions.
Frequently Encountered Challenges
| Question | Explanation |
|---|---|
| How do I identify key information in word problems? | Start by carefully reading the problem and underlining important numbers, variables, and terms. Focus on what is being asked and look for clues about what formulas or methods should be applied. |
| What if I don’t understand a specific formula? | Make sure you understand the logic behind the formula. Break it down into simpler parts or review related examples. If needed, consult additional resources or ask for clarification. |
| How can I avoid common calculation errors? | Double-check your work at each step. Take your time and ensure every calculation is correct before moving to the next. It helps to work through the problem step by step and avoid skipping any steps. |
| How do I know when to use specific strategies? | Recognize the type of problem you’re dealing with and match it to the appropriate strategy or formula. Look for patterns in the problems you’ve solved to determine which approach works best. |
Tips for Overcoming Common Hurdles
- Work through practice problems regularly to familiarize yourself with common question types.
- Ask for help or clarification when a concept is unclear, whether from a teacher, tutor, or peer.
- Try explaining the concept or problem-solving process aloud to ensure you fully understand it.
- Refer back to your notes and resources to reinforce your understanding of key principles.
How to Approach Word Problems
Word problems can seem overwhelming at first, but with a systematic approach, they become much more manageable. The key is to break the problem down into smaller, understandable parts. By carefully analyzing each detail, identifying the core question, and applying the right mathematical principles, you can work through the problem step by step.
The first step is to read the problem thoroughly. It’s essential to understand exactly what is being asked before jumping into calculations. Highlight important numbers, keywords, and any relevant details that provide clues for the solution. Once you’ve identified the key components, consider how they relate to each other and what kind of operation or strategy might be required to solve the problem.
Next, set up an equation or diagram based on the information provided. This visual or symbolic representation can help clarify relationships between different parts of the problem. Whether it’s a simple addition or a complex geometry problem, organizing the data logically is crucial.
Finally, solve the problem by applying the appropriate methods, but remember to check your work as you go. Re-reading the problem after solving it ensures that you haven’t missed any critical details and that your answer makes sense in the given context.
Key Steps to Solve Word Problems
- Read the Problem Carefully: Make sure you understand the question fully before proceeding.
- Identify Key Information: Highlight numbers, units, and relationships that are essential for solving the problem.
- Choose a Strategy: Decide which mathematical concepts or formulas apply to the problem.
- Write the Solution: Organize your work clearly, using proper notation or diagrams if necessary.
- Review Your Answer: Double-check calculations and ensure the answer aligns with the question.
Helpful Tips for Word Problems
- Stay organized: Write down every piece of information and equation step-by-step.
- Use estimation: Round numbers or estimate answers to see if they are reasonable.
- Look for keywords: Words like “total,” “difference,” “product,” and “ratio” can give clues to the type of operation needed.
- Don’t rush: Take your time to ensure each step is correct before moving to the next.
Strategies for Efficient Problem Solving
To tackle complex problems effectively, it’s essential to use proven strategies that streamline the process and improve accuracy. Efficient problem solving involves not just applying mathematical techniques but also approaching each problem with a clear mindset and logical structure. With the right strategies, you can save time, reduce errors, and enhance your understanding of the material.
The first strategy is to carefully read the problem and break it down into smaller, more manageable parts. Identifying key pieces of information and understanding the relationships between them will make it easier to find a solution. Organizing your work and using visual aids, such as diagrams or charts, can also be helpful for clarifying complex problems.
Another effective approach is to work systematically. Solve the problem step by step, ensuring that each part is completed before moving on to the next. This method helps prevent skipping critical steps or making mistakes due to rushing. Additionally, always double-check your work to verify accuracy and ensure that the solution makes sense in the context of the problem.
Key Strategies for Success
- Understand the Problem: Take time to fully understand what the problem is asking before attempting a solution.
- Break Down the Problem: Divide complex problems into simpler subproblems, making them easier to solve.
- Use Visual Aids: Draw diagrams or charts when necessary to organize information and clarify relationships.
- Work Methodically: Approach the problem step by step and avoid skipping any important steps.
- Verify Your Answer: Always double-check calculations and ensure that the solution makes sense.
Additional Tips for Efficient Problem Solving
- Practice regularly to become familiar with different types of problems and solutions.
- Stay organized by keeping your work neat and labeling each step clearly.
- Use estimation to quickly check if your answer is reasonable before doing the full calculation.
- Be patient and give yourself time to think through each problem carefully.
Breaking Down Complex Problems
When faced with a challenging problem, the key to success lies in breaking it down into smaller, more manageable parts. By approaching complex issues step by step, you can reduce the overwhelming nature of the task and focus on solving each individual aspect. This method helps to identify the core components and make the problem-solving process more straightforward and systematic.
The first step in breaking down a complex problem is to identify the different elements involved. Look for key data points, relationships, and conditions that are part of the problem. By organizing these details, you can create a roadmap for how to approach the solution.
Once you’ve segmented the problem into smaller sections, work through each part methodically. Address each piece individually, ensuring that you fully understand its role in the overall problem. This approach prevents errors and ensures that you are not missing any critical information.
Steps to Decompose a Problem
- Identify Key Elements: Break the problem into smaller, logical parts by recognizing the variables, data, and relationships involved.
- Analyze Each Component: Solve each individual part of the problem, ensuring clarity and accuracy before moving to the next step.
- Look for Patterns: Identify any patterns or similarities in the problem that can help guide your solution process.
- Integrate Solutions: Once all smaller parts are solved, combine them to form the final solution to the larger problem.
Tips for Effective Problem Decomposition
- Approach each subproblem independently to avoid confusion between different steps.
- Use diagrams, charts, or tables to visually organize the elements of the problem.
- Break down the problem into even smaller parts if it still seems too complex.
- Regularly review the problem-solving process to ensure you’re on the right track.
Real-World Applications of CPM Chapter 3
The skills learned in solving complex problems are not only useful in academic contexts but also have significant real-world applications. By mastering the methods introduced in this section, individuals can enhance their ability to approach practical challenges in various fields. These techniques are often used in decision-making processes, project management, engineering, finance, and beyond, where breaking down tasks and analyzing components can lead to more effective solutions.
One of the most valuable uses of these problem-solving strategies is in project management. By understanding how to approach complex tasks and break them down into manageable steps, professionals can plan projects more efficiently. This includes setting milestones, estimating resources, and predicting potential challenges, all of which contribute to the success of a project.
In the field of engineering, these methods help to identify the key factors involved in a design or system. By segmenting a complex system into smaller parts, engineers can troubleshoot and optimize designs, ensuring that each component functions as intended and meets the overall goals.
Applications in Various Industries
- Project Management: Breaking down large projects into smaller tasks helps in allocating resources, scheduling, and monitoring progress.
- Engineering: Decomposing complex systems allows engineers to troubleshoot, improve designs, and test components efficiently.
- Finance: In financial analysis, breaking down data into segments helps in identifying trends, managing risk, and making informed investment decisions.
- Software Development: Complex coding problems can be divided into smaller coding tasks, streamlining the development process and improving functionality.
- Healthcare: In medical research, complex studies can be broken down into smaller trials or phases, making it easier to analyze data and draw conclusions.
How These Techniques Benefit Professionals
- Improves efficiency by organizing complex information into simpler components.
- Helps identify critical steps and areas where resources can be optimized.
- Allows for more accurate predictions and better decision-making based on thorough analysis.
- Enhances collaboration across teams by ensuring everyone understands their specific tasks within the larger framework.
Time Management During Chapter 3 Exercises
Effective time management is crucial when working through complex exercises, especially when the tasks at hand require careful analysis and attention to detail. Allocating time efficiently allows you to focus on each part of the problem without feeling rushed or overwhelmed. By practicing good time management, you can enhance both the quality of your work and your ability to complete tasks within deadlines.
The first step in managing your time effectively is to break down the exercise into smaller tasks. This enables you to assign an appropriate amount of time to each part and helps maintain focus without feeling distracted. Setting clear goals for each section can keep you on track and ensure that you stay organized throughout the process.
Another important aspect of time management is prioritization. Identify the most challenging or time-consuming parts of the exercise and allocate more time to these areas. By tackling the harder tasks first, you’ll be able to address them when your concentration and energy levels are at their peak, leaving the easier components for later.
Finally, it’s essential to build in short breaks to avoid mental fatigue. Taking brief moments to relax can help recharge your focus and prevent burnout. Managing your time well during exercises not only improves performance but also builds long-term efficiency in problem-solving.
Using Resources to Improve Your Skills
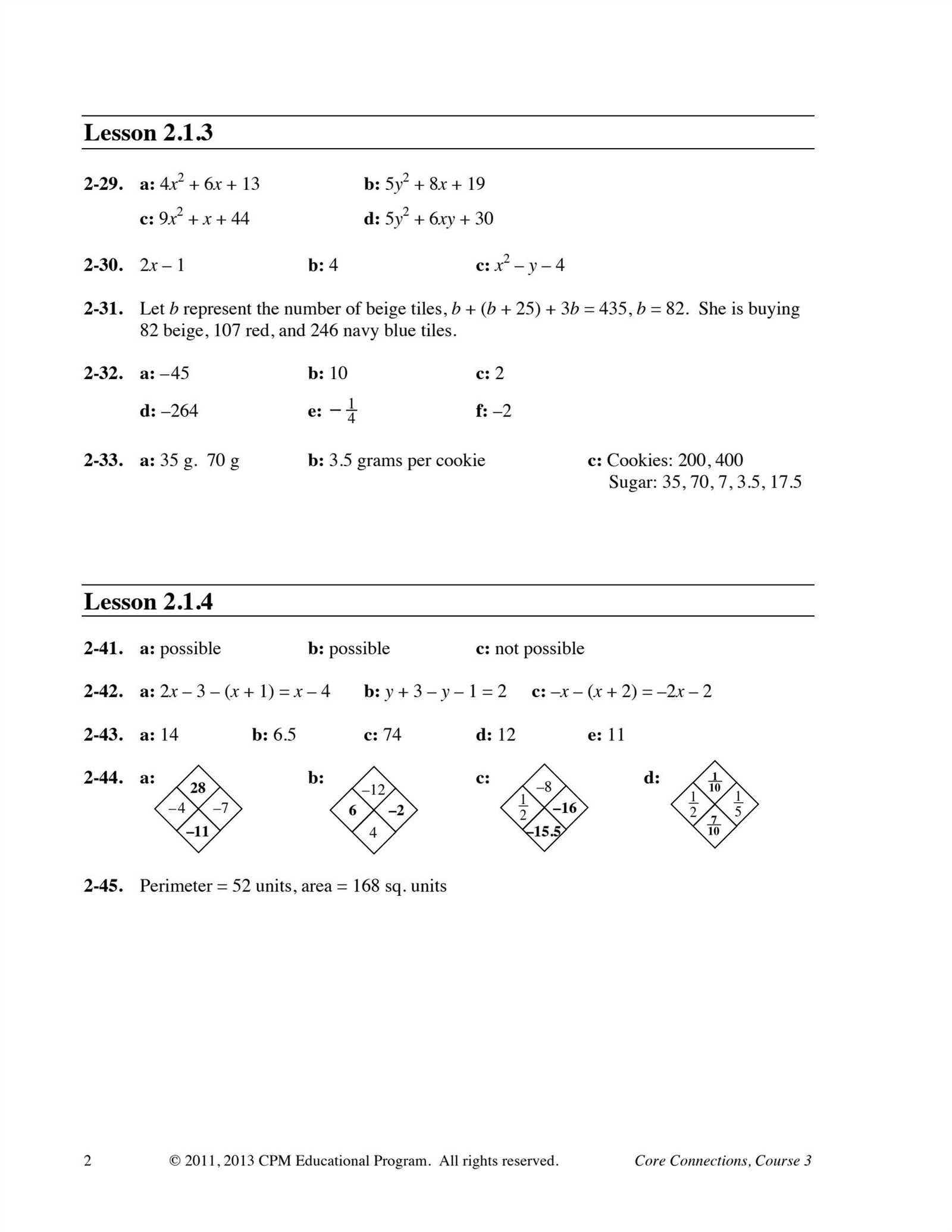
To enhance your problem-solving abilities and grasp challenging concepts, it is important to leverage a variety of resources. These tools can provide additional insights, practice opportunities, and alternative approaches, all of which contribute to better comprehension and skill development. Whether you’re tackling specific exercises or seeking to broaden your understanding, the right resources can make a significant difference in your learning journey.
One of the most effective resources is practice material. Consistently working through different problems allows you to apply what you’ve learned and gain experience in problem-solving. Solutions manuals and online exercises offer step-by-step breakdowns, helping you understand complex steps in detail. Additionally, interactive platforms and apps can provide instant feedback, helping you identify areas where you need further improvement.
Another valuable resource is peer collaboration. Discussing problems with others, whether in study groups or online forums, can help you view the material from different perspectives. Collaboration encourages critical thinking and can lead to more creative solutions. It also helps you stay motivated and on track with your learning goals.
Finally, don’t overlook reference materials such as textbooks, educational websites, and instructional videos. These resources can clarify concepts that may be unclear and offer a deeper dive into the theory behind the exercises. Using a combination of resources will help reinforce your understanding and ensure that you are fully equipped to handle more complex tasks with confidence.
Understanding Key Formulas
Mastering key formulas is essential when working through mathematical or problem-solving exercises. These formulas serve as the foundation for solving complex problems, allowing you to quickly and accurately apply the right approach. Understanding how each formula works and when to use it can significantly improve your efficiency and problem-solving skills.
Each formula typically has a specific application, and grasping its meaning is crucial for proper implementation. For example, knowing how to apply basic principles such as the distributive property, the quadratic formula, or geometric equations enables you to break down complicated tasks into simpler steps. It’s important to not only memorize these formulas but also to understand their derivations and underlying concepts.
To effectively use formulas, practice is key. By consistently solving problems that require specific formulas, you build familiarity with their structure and application. Over time, this practice will help you recognize patterns in problems and identify which formula is needed for each situation. Additionally, understanding how formulas relate to one another can deepen your insight into the subject and enhance your ability to solve even more advanced problems.
Reviewing Example Problems for Clarity
Going through example problems is an essential step in mastering problem-solving skills. These examples offer a structured way to understand the thought process behind each solution. By breaking down the steps in the examples, you can gain a clearer perspective on how to approach similar tasks on your own.
Reviewing example problems helps to highlight key strategies, such as identifying the correct method, using appropriate formulas, and applying logical reasoning. When you see how each problem is tackled systematically, it becomes easier to replicate the process and avoid common mistakes in your own work.
To maximize the benefit of reviewing example problems, follow these steps:
- Identify the core concept: Focus on understanding the underlying principle that the example is demonstrating.
- Break down the steps: Review each step carefully to see how it leads to the final solution. This will help you understand the problem-solving flow.
- Look for patterns: Try to identify patterns that can be applied to similar problems. This can save you time and effort in future exercises.
- Rework the example: After reviewing, attempt to solve the example on your own without looking at the solution. This reinforces your understanding.
By continuously reviewing examples and reflecting on the problem-solving process, you can build confidence and improve your ability to tackle new and more challenging problems with ease.
How to Stay Motivated While Studying
Maintaining motivation during study sessions can be challenging, especially when the material becomes more difficult or the workload increases. However, staying focused and motivated is key to mastering complex topics and achieving your academic goals. By implementing the right strategies, you can keep yourself energized and on track throughout your studies.
One effective approach is setting clear, manageable goals. Breaking down larger tasks into smaller, more achievable steps makes studying feel less overwhelming. Additionally, rewarding yourself after reaching each milestone can provide positive reinforcement and keep you motivated. These small wins add up over time and contribute to long-term success.
Techniques to Boost Motivation
- Create a study routine: Consistency can help you stay focused. Set a specific time each day for studying and stick to it.
- Find a study group: Collaborating with others can keep you engaged and offer support when you face challenges.
- Stay positive: Focus on your progress, not on setbacks. Celebrate each step forward, no matter how small.
- Mix up your study methods: Experiment with different techniques like flashcards, summarization, or teaching the material to others to keep things fresh.
Setting and Tracking Goals
| Goal | Target Date | Reward |
|---|---|---|
| Complete 10 practice problems | End of the week | Take a 15-minute break |
| Review chapter material | Two days before test | Watch an episode of your favorite show |
Staying motivated is about creating a study environment that works for you. By setting goals, staying consistent, and using positive reinforcement, you can overcome challenges and stay on track to achieve success in your studies.
Summary of Key Takeaways
In this section, we summarize the core concepts and strategies that have been covered, focusing on the most important lessons. The ability to effectively tackle mathematical challenges and improve problem-solving skills relies on understanding the key techniques and frameworks discussed. A strong grasp of these principles will aid in navigating through the exercises and applying them to real-world scenarios.
Important Concepts
- Problem Decomposition: Breaking down complex problems into smaller, manageable parts helps simplify the process and makes it easier to identify the steps required for a solution.
- Strategic Planning: Developing a clear approach before diving into a problem ensures that the solution is both efficient and effective.
- Formulas and Procedures: Understanding and memorizing key formulas is essential for solving problems quickly and accurately.
- Time Management: Allocating sufficient time to each part of the problem-solving process ensures that no detail is overlooked.
Application of Skills
It is crucial to apply these skills regularly in order to build confidence and proficiency. By practicing consistently and reviewing key strategies, learners can reinforce their understanding and improve their performance. Regular reflection on progress and identifying areas of improvement can also contribute to long-term success.