As students progress through their studies, they often encounter complex mathematical challenges that require a deeper understanding and sharper problem-solving skills. These challenges can seem daunting at first, but with the right resources and strategies, they become more manageable. This guide offers a structured approach to mastering these advanced topics, providing solutions and insights that will aid in developing a solid foundation.
Effective learning goes beyond simply solving individual problems. It’s about understanding the principles and methods that make those solutions possible. By working through practice exercises and applying key concepts, you can enhance your ability to tackle even the most difficult problems. Whether you’re preparing for exams or aiming to improve your skills, a methodical approach is essential for long-term success.
Mastering these topics not only boosts your confidence but also lays the groundwork for future mathematical pursuits. With a variety of tools at your disposal, such as detailed solutions and step-by-step explanations, you’ll be able to approach each problem with clarity and precision. Embrace the learning process, and watch your understanding grow as you continue to refine your problem-solving techniques.
Math Nation Algebra 2 Answers Guide
When tackling advanced mathematical challenges, having the right resources can make all the difference. This guide provides a comprehensive roadmap to help students navigate complex topics, from foundational principles to more intricate problem-solving techniques. By understanding the process behind each solution, students can build a stronger grasp of the material and improve their performance in assignments and exams.
Key Strategies for Success
Success in advanced mathematics comes from a combination of consistent practice, deep comprehension of underlying principles, and the ability to break down complex problems into manageable steps. To maximize your learning potential, focus on the following strategies:
- Understand the theory: Before solving problems, ensure you grasp the core concepts behind each topic. This will make it easier to approach new problems and identify patterns.
- Practice regularly: Constant exposure to different types of problems helps reinforce concepts and improve problem-solving speed.
- Work step-by-step: Break each problem into smaller steps to avoid overwhelming yourself. This approach ensures no key concept is overlooked.
- Use additional resources: Leverage online platforms and textbooks for extra examples and explanations that can enhance your understanding.
Utilizing Solutions Effectively
Solutions are more than just the end result–they are a tool for learning. Reviewing detailed solutions offers an opportunity to learn the techniques used to arrive at the correct answer. By studying these solutions, you can:
- Identify common methods: Notice the approaches used repeatedly, and practice applying them to similar problems.
- Avoid mistakes: Understand where errors are typically made and how to avoid them in the future.
- Improve comprehension: Revisit any steps you don’t fully understand, and use supplementary materials to clarify these points.
By incorporating these practices, students can approach advanced topics with confidence and improve both their understanding and problem-solving abilities.
Understanding Algebra 2 Concepts
Mastering advanced mathematical topics requires more than just solving problems–it involves understanding the core principles that underpin the exercises. Whether you’re dealing with equations, functions, or graphing techniques, grasping the theoretical concepts is essential to solving complex problems with ease. This section highlights key ideas that are crucial for success in higher-level math courses and provides a foundation for more advanced studies.
The first step is to identify the key concepts and their applications. From working with polynomial equations to analyzing exponential growth, recognizing how each concept fits into the broader picture allows students to tackle new challenges confidently. It’s also important to understand how various topics connect, as this will enable a deeper understanding and improve problem-solving efficiency.
Once the fundamentals are in place, continuous practice and review will help solidify the knowledge. By revisiting these ideas through practice problems, students can reinforce their understanding and recognize patterns that make future problems easier to approach. A strong conceptual foundation is the key to both academic success and long-term retention in mathematics.
Key Strategies for Solving Algebra 2 Problems
When approaching complex mathematical problems, having a solid strategy can make all the difference. Whether you’re solving equations, working with functions, or analyzing graphs, using the right approach helps break down challenges into manageable steps. This section explores key strategies that will improve problem-solving skills and ensure more efficient and accurate solutions.
Start by organizing the given information clearly and identifying what is being asked. Recognizing the type of problem you’re dealing with can guide you toward the appropriate methods and formulas. Once you’ve identified the core principles, apply them step-by-step, ensuring no detail is overlooked. Consistent practice with these strategies will build confidence and speed over time.
| Strategy | Description |
|---|---|
| Break down the problem | Divide the problem into smaller, easier-to-handle steps to avoid confusion and ensure clarity in each stage. |
| Use known formulas | Leverage formulas that are relevant to the problem at hand, ensuring you’re applying the correct ones for each type of equation. |
| Check for patterns | Look for patterns or similarities in the problem that can help simplify your work, such as recognizing common terms or structures. |
| Verify solutions | Always check your results to ensure they are correct, especially when dealing with multiple steps or complex calculations. |
By following these strategies, students can approach each problem more systematically, reduce errors, and improve their overall problem-solving skills.
Common Challenges in Algebra 2
As students progress through advanced mathematical courses, they often encounter specific obstacles that can make certain topics particularly difficult to grasp. These challenges may arise due to the increasing complexity of problems, unfamiliarity with new concepts, or difficulties in applying previously learned methods to more advanced scenarios. Identifying and addressing these challenges is essential for success in higher-level mathematics.
One of the most common difficulties students face is working with complex equations, especially when they involve multiple variables or require advanced techniques to solve. Additionally, understanding abstract concepts such as exponential functions or polynomial equations can be overwhelming without a solid foundation in the basics. Another hurdle often encountered is graphing, as interpreting and plotting more intricate curves demands a deeper understanding of both theoretical and practical aspects of the material.
To overcome these challenges, it’s crucial to approach each topic systematically, breaking down problems into manageable parts and practicing regularly. By consistently revisiting difficult concepts and seeking clarification when needed, students can gradually build confidence and improve their problem-solving abilities.
How to Use Math Nation Effectively
Maximizing the effectiveness of an online learning platform requires more than just browsing through available resources. To truly benefit from the materials and tools provided, it’s important to adopt a focused and strategic approach. Using such platforms effectively can accelerate learning, improve understanding, and help overcome challenges in complex subjects.
Start by familiarizing yourself with the layout and navigation of the platform. Understand where the key resources, such as practice problems, step-by-step solutions, and instructional videos, are located. Once you’re comfortable with the platform’s structure, set specific goals for each session. This can range from mastering a particular concept to practicing a specific set of problems.
Incorporate regular practice into your study routine by using the platform’s exercises to test your knowledge. Don’t just read through the solutions–take time to actively work through the problems, and refer to the explanations only when necessary. Additionally, make use of any available progress tracking tools to monitor improvements and identify areas that need more attention.
Step-by-Step Algebra 2 Solutions
Breaking down complex problems into clear, manageable steps is a crucial skill in advanced mathematics. By following a structured approach, students can tackle even the most difficult problems with confidence. This section will guide you through the process of solving various types of problems, ensuring that each solution is reached logically and efficiently.
General Approach to Solving Problems
To solve any problem effectively, start by thoroughly understanding what is being asked. Then, systematically break down the problem into smaller parts. Use known formulas or techniques, applying them step by step, ensuring each action logically follows from the previous one.
| Step | Action |
|---|---|
| 1 | Read the problem carefully and identify the key information. |
| 2 | Choose the appropriate method or formula based on the problem type. |
| 3 | Solve the problem step by step, checking for accuracy as you go. |
| 4 | Double-check the final answer to ensure it meets the requirements of the question. |
Example Problem Breakdown
Let’s consider an example problem where you need to solve for an unknown variable in an equation. Begin by isolating the variable on one side of the equation, applying the appropriate operations such as addition, subtraction, multiplication, or division. Always perform operations on both sides of the equation to maintain balance.
By following this structured, step-by-step approach, you can gain a clearer understanding of how to approach various types of problems and develop your problem-solving skills further.
Tips for Mastering Algebra 2 Topics
Mastering advanced mathematical concepts requires dedication, focus, and a strategic approach. While the material may initially seem challenging, with the right techniques and consistent practice, students can develop a deeper understanding and build confidence in their problem-solving abilities. This section offers useful tips to help you excel in complex topics and improve your performance.
One of the key strategies for success is to approach each topic with a clear, structured method. Break down the material into smaller, manageable chunks and ensure a solid grasp of each before moving on to the next. Additionally, review and practice regularly to reinforce the concepts and identify areas that need further attention.
| Tip | Description |
|---|---|
| Understand the Fundamentals | Before tackling advanced topics, ensure you have a firm grasp on the basic principles, as they form the foundation for more complex concepts. |
| Practice Regularly | Consistent practice is key to mastering any subject. Work through problems daily to reinforce concepts and improve your problem-solving skills. |
| Learn from Mistakes | When you make errors, take the time to understand where you went wrong and correct your approach. This helps avoid repeating mistakes and strengthens your understanding. |
| Seek Help When Needed | If you’re struggling with a particular topic, don’t hesitate to ask for help. Use additional resources, such as tutors or online forums, to clarify difficult concepts. |
| Review Key Concepts | Regularly revisit important topics to ensure retention. Going over previously learned material strengthens your overall understanding and prepares you for more advanced topics. |
By following these tips and committing to consistent practice, you will be better prepared to handle the challenges of advanced mathematical topics and achieve success in your studies.
Working Through Algebra 2 Practice Problems
Practicing mathematical problems is one of the most effective ways to strengthen your skills and build confidence. By working through a variety of problems, you develop a deeper understanding of key concepts and improve your ability to apply different techniques. This section focuses on how to effectively tackle practice problems, ensuring that you gain the most from each exercise.
When approaching a problem, start by carefully reading the instructions and identifying the information provided. Break the problem down into smaller steps and determine the best method to solve it. Whether you are dealing with an equation, graph, or function, take your time to follow each step methodically. Don’t rush–taking the time to understand the process will lead to more accurate results.
It’s also important to review your work once you’ve completed a problem. Check your steps for accuracy and ensure that the solution makes sense in the context of the original problem. If you encounter difficulties, try to pinpoint where you went wrong, and use this as an opportunity to reinforce your understanding of the material. Over time, consistent practice and review will help you tackle even the most challenging problems with ease.
Why Algebra 2 Is Important
Advanced mathematical concepts are crucial not only for academic success but also for developing critical thinking skills. A deeper understanding of complex problem-solving techniques prepares students for a variety of real-world applications. Mastering advanced topics helps individuals make informed decisions, analyze data, and think analytically in everyday life.
Application in Higher Education
Many college programs, especially in fields such as engineering, economics, computer science, and natural sciences, require knowledge of advanced mathematical methods. A solid foundation in these areas equips students with the skills needed for success in their chosen career paths. Additionally, these skills are valuable for standardized tests like the SAT and ACT, where analytical reasoning plays a major role.
Real-World Relevance
- Problem-solving: The techniques learned in advanced math courses are widely applicable in problem-solving across various industries.
- Financial management: Understanding concepts such as percentages and equations aids in budgeting and personal finance.
- Technology: Many technological advancements are rooted in mathematical principles, from programming to data analysis.
In conclusion, mastering these topics is not just about passing exams but about acquiring valuable skills that are essential for academic, professional, and personal growth.
Essential Algebra 2 Formulas and Theorems
Advanced mathematical study involves understanding and applying a variety of essential formulas and theorems that form the backbone of complex problem-solving. These key concepts provide the tools necessary for tackling more difficult equations and relationships between variables. A strong grasp of these formulas is crucial for progressing in higher-level mathematics and for real-world applications in fields such as science, engineering, and finance.
Key Formulas
There are several core formulas that every student should know. These include quadratic formulas, geometric series, and logarithmic equations, among others. Understanding how to manipulate and apply these formulas will help solve a wide range of problems effectively.
| Formula | Use |
|---|---|
| Quadratic Formula | Used to solve quadratic equations in the form ax² + bx + c = 0 |
| Binomial Theorem | Used to expand expressions of the form (a + b)ⁿ |
| Exponential Growth Formula | Used to model growth or decay processes, such as population or investment returns |
| Logarithmic Identity | Helps simplify expressions involving logarithms |
Important Theorems
Theorems in advanced mathematics provide the logical foundation for the formulas and methods used. These theorems explain the relationships between different mathematical elements and offer a deeper understanding of why formulas work the way they do.
- Pythagorean Theorem: Relates the sides of a right triangle.
- Fundamental Theorem of Algebra: Every non-constant polynomial equation has at least one complex root.
- Rational Root Theorem: Helps identify potential rational roots of polynomial equations.
By memorizing and understanding these essential formulas and theorems, students will be better equipped to handle complex mathematical challenges and perform well in advanced coursework.
How to Prepare for Algebra 2 Exams
Preparing for exams in advanced mathematics requires a strategic approach that emphasizes understanding key concepts, practicing regularly, and managing time effectively. With the right study habits, students can increase their chances of success and gain the confidence needed to tackle complex problems. This section outlines effective strategies to help you prepare thoroughly for your upcoming test.
Effective Study Techniques

When preparing for an exam, it is essential to focus on both understanding the theory and applying practical problem-solving methods. Here are some useful techniques to guide your study sessions:
- Review Key Concepts: Go over the main topics covered in class, making sure you understand the fundamental ideas behind each one.
- Practice Problem-Solving: The more problems you work through, the more comfortable you’ll become with applying different strategies and methods.
- Create a Study Schedule: Plan your study sessions ahead of time to ensure adequate coverage of all the material without cramming at the last minute.
- Use Flashcards: Create flashcards to memorize formulas, theorems, and key concepts for quick recall during the exam.
Test-Taking Tips
Once you’re well-prepared, it’s crucial to implement effective test-taking strategies to maximize your performance during the exam:
- Read the Questions Carefully: Take time to understand what is being asked before jumping into solving.
- Manage Your Time: Allocate specific amounts of time to each section of the exam to avoid spending too long on any one question.
- Check Your Work: If time allows, review your answers to catch any mistakes and ensure your calculations are correct.
- Stay Calm: Keep a clear mind during the test. Stay confident in your preparation and don’t let anxiety take over.
By following these strategies, you’ll be able to approach your exam with a sense of readiness and confidence, significantly improving your chances of achieving a strong score.
Utilizing Online Resources for Algebra 2
With the growing availability of online platforms, students now have access to a wealth of tools and materials that can significantly aid their learning process. Whether you are looking to deepen your understanding of complex topics or seeking extra practice to master difficult concepts, the internet offers numerous resources that can enhance your study experience. This section explores how to effectively use online tools to support your learning in advanced mathematical courses.
Types of Online Resources
There are various online tools available, each serving a different purpose. These resources can range from instructional videos to interactive practice problems, and each can help you better grasp challenging material:
- Tutorial Videos: Websites like YouTube offer countless tutorials that break down difficult concepts into simpler steps. Watching these videos can provide visual explanations and examples of how to solve different types of problems.
- Interactive Practice Websites: Platforms like Khan Academy or similar sites provide a vast array of problems that you can solve directly on the platform, offering instant feedback on your solutions.
- Online Calculators: Tools like WolframAlpha can help with solving equations, checking work, and verifying results, making them ideal for cross-checking your answers.
- Study Forums: Participating in online discussion forums can allow you to engage with peers and tutors who can clarify doubts and offer new perspectives on challenging problems.
How to Maximize the Use of Online Tools
While online resources are valuable, it’s important to use them effectively to ensure they enhance, rather than detract from, your learning. Here are a few tips for maximizing the benefits of these tools:
- Set Clear Goals: Before using online resources, identify specific topics or problems you need help with, so you can use the tools efficiently.
- Practice Regularly: Consistent practice is key to mastering advanced mathematical concepts. Make sure to actively engage with practice problems to reinforce your understanding.
- Take Notes: While watching videos or solving problems online, take notes on important steps or strategies, which can help with future revision.
- Join Online Communities: Engage with others who are also learning or experts in the field to get tips, explanations, and support.
By incorporating these online resources into your study routine, you can gain a deeper understanding of the material and improve your problem-solving skills, making your learning experience more effective and enjoyable.
Improving Problem-Solving Speed
Developing the ability to solve complex problems quickly is a crucial skill, especially in advanced mathematical studies. Speed doesn’t just come from knowing the right methods but also from practicing them under time pressure. This section will explore strategies to help you improve your problem-solving efficiency and boost your performance during exams or timed exercises.
Key Strategies for Faster Solutions
There are several approaches you can take to increase your problem-solving speed:
- Master the Basics: A solid understanding of fundamental principles is essential. The more you practice basic techniques, the faster you can recall and apply them when tackling more difficult problems.
- Identify Patterns: Many problems follow predictable patterns. Recognizing these patterns allows you to quickly apply known solutions, reducing the time spent on figuring out each new problem from scratch.
- Work on Mental Math: Being able to perform calculations in your head, rather than relying on a calculator, can save valuable time. Regular practice with mental arithmetic can greatly improve your speed.
- Practice with Timed Exercises: Regularly challenging yourself with timed problems will help you build speed. Set a timer for each exercise to simulate exam conditions and track your progress.
Effective Practice Techniques
Speed doesn’t come overnight, but consistent, focused practice will yield results. Here are some effective techniques to accelerate your progress:
- Break Down Problems: Practice breaking problems into smaller steps. Solving each smaller part of the problem quickly will help you build momentum as you progress through the entire question.
- Skip When Stuck: If you’re stuck on a particular problem, move on and return to it later. This will prevent you from wasting time on a single question and help you maintain a steady pace.
- Use the Process of Elimination: If you’re unsure about the correct answer, eliminate obviously incorrect options first. This can often narrow down your choices and save time in multiple-choice questions.
- Review Mistakes: After completing practice sessions, review your mistakes carefully. Understanding why you made an error will help you avoid repeating it and improve your accuracy, which ultimately leads to faster solving.
By focusing on these strategies and dedicating time to practicing under timed conditions, you will gradually improve your problem-solving speed and become more confident in tackling complex problems efficiently.
Applying Algebra 2 in Real Life
Understanding and mastering advanced mathematical concepts can have a profound impact beyond the classroom. These skills are widely applicable to various real-world scenarios, from financial planning to engineering projects. In this section, we will explore how the principles and techniques learned in advanced studies can be used to solve everyday problems and make informed decisions.
Practical Uses in Everyday Situations
Advanced mathematical methods can be applied in numerous ways to improve everyday life:
- Budgeting and Financial Planning: Understanding equations and inequalities can help you calculate expenses, savings, and loans, allowing for more effective financial management.
- Home Renovations: When working on home improvement projects, mathematical concepts like geometry and measurement can help you accurately estimate the materials needed and the costs involved.
- Cooking and Recipe Adjustments: Ratios and proportions are frequently used in cooking to scale recipes. Being able to adjust ingredient quantities based on serving sizes requires a strong grasp of these concepts.
- Time Management: Algebraic equations can help optimize schedules, calculate travel time, or determine the most efficient way to allocate resources for a project.
Applications in Professional Fields
In the professional world, advanced mathematical concepts play a significant role in various careers and industries:
- Engineering: Structural engineers use complex equations to design buildings, bridges, and other infrastructures, ensuring safety and durability.
- Computer Science: Algorithms and coding rely heavily on abstract mathematical concepts to develop software, solve problems, and create efficient systems.
- Data Science: Understanding and interpreting data is a fundamental skill in many industries. Advanced mathematics is used to analyze trends, predict outcomes, and make data-driven decisions.
- Physics and Medicine: Both fields rely on complex mathematical models to understand phenomena, create medical devices, and simulate experimental conditions.
By applying these concepts in practical scenarios, individuals can enhance their problem-solving abilities, streamline daily tasks, and improve their professional performance. The skills learned in advanced studies are not just theoretical but are essential for navigating the challenges of everyday life and achieving long-term success.
Correcting Mistakes in Algebra 2
Making mistakes is a natural part of learning, especially when dealing with complex mathematical concepts. Understanding where and why errors occur is key to improving your skills. In this section, we will explore common mistakes made while solving advanced equations and provide strategies for identifying and correcting them.
Identifying Common Errors
Several types of mistakes can occur while working through problems:
- Sign Errors: Misplacing or forgetting negative signs can drastically change the outcome of an equation. It’s essential to carefully check each term for correct sign placement.
- Misapplication of Formulas: Using the wrong formula for a specific problem or applying a formula incorrectly is a frequent error. Always ensure you understand the context of the formula before using it.
- Missteps in Simplification: When simplifying expressions, it’s easy to overlook certain steps or apply operations incorrectly, leading to wrong results. Take your time with each simplification process.
- Overlooking Parentheses: Parentheses play a crucial role in determining the order of operations. Neglecting parentheses or forgetting to distribute them properly can result in incorrect answers.
Strategies for Correcting Mistakes
To reduce the number of mistakes and correct them effectively:
- Double-Check Calculations: Review each step methodically. Work backward from the final result to ensure no errors were made during simplification or solving.
- Understand the Concept: If you find yourself repeatedly making similar mistakes, it may indicate a gap in understanding the underlying concept. Take time to revisit the theory and examples.
- Practice Regularly: The more you practice, the more familiar you become with common pitfalls and how to avoid them. Regular practice solidifies techniques and improves accuracy.
- Ask for Help: If you’re stuck on a particular problem, don’t hesitate to ask a teacher or peer for clarification. Sometimes, a fresh perspective can reveal where the mistake occurred.
Correcting mistakes is an essential part of mastering advanced mathematical concepts. By being patient, methodical, and persistent, you can significantly reduce errors and improve your overall problem-solving skills.
Algebra 2 Review and Reinforcement Tips
Reviewing advanced concepts is crucial for mastering them. Whether you’re preparing for an exam or simply strengthening your understanding, reinforcing what you’ve learned is key to retention. In this section, we will outline effective strategies for revisiting material, improving problem-solving skills, and ensuring long-term success in more advanced topics.
Effective Review Strategies
To reinforce your knowledge and ensure you retain key concepts, follow these proven strategies:
- Break Down Complex Problems: Start with simpler problems and gradually work up to more challenging ones. This helps you understand the underlying steps involved and boosts confidence.
- Summarize Key Concepts: Create concise summaries of important formulas, theorems, and methods. Writing down key points helps reinforce memory and gives you quick access to information.
- Practice Regularly: Regular practice is essential for reinforcement. Set aside time each day to work on different topics to avoid forgetting crucial details.
- Use Flashcards: Flashcards are a great tool for memorizing formulas and key steps. Reviewing them regularly can help cement your understanding and make recalling information quicker.
Strengthening Problem-Solving Skills
Problem-solving is a skill that improves with practice. To reinforce this ability, consider these approaches:
- Work on a Variety of Problems: Expose yourself to a wide range of problems. This helps you apply your knowledge in different scenarios and improves flexibility in problem-solving.
- Check Your Work: After solving a problem, review each step carefully. This process helps identify any mistakes and reinforces the correct approach.
- Focus on Weak Areas: Identify which topics or types of problems give you the most trouble and dedicate extra time to practicing them. Reinforcement in these areas can significantly improve your overall performance.
- Use Step-by-Step Solutions: If you’re stuck, look at detailed solutions to understand the steps involved. Working through solutions helps clarify complex methods and teaches you effective strategies for tackling similar problems.
By consistently reviewing and practicing, you can enhance your understanding of more advanced topics. Reinforcing the material through different methods ensures that you’re not only able to solve problems but also fully grasp the concepts behind them.
How Algebra 2 Answers Help Your Learning
Finding solutions to complex problems plays a crucial role in deepening your understanding of advanced mathematical concepts. Being able to check your work and analyze the steps leading to a correct outcome provides valuable insights that help solidify your knowledge. This process not only reinforces problem-solving techniques but also enhances your overall learning experience by showing you the correct approach to various challenges.
Understanding the Correct Approach
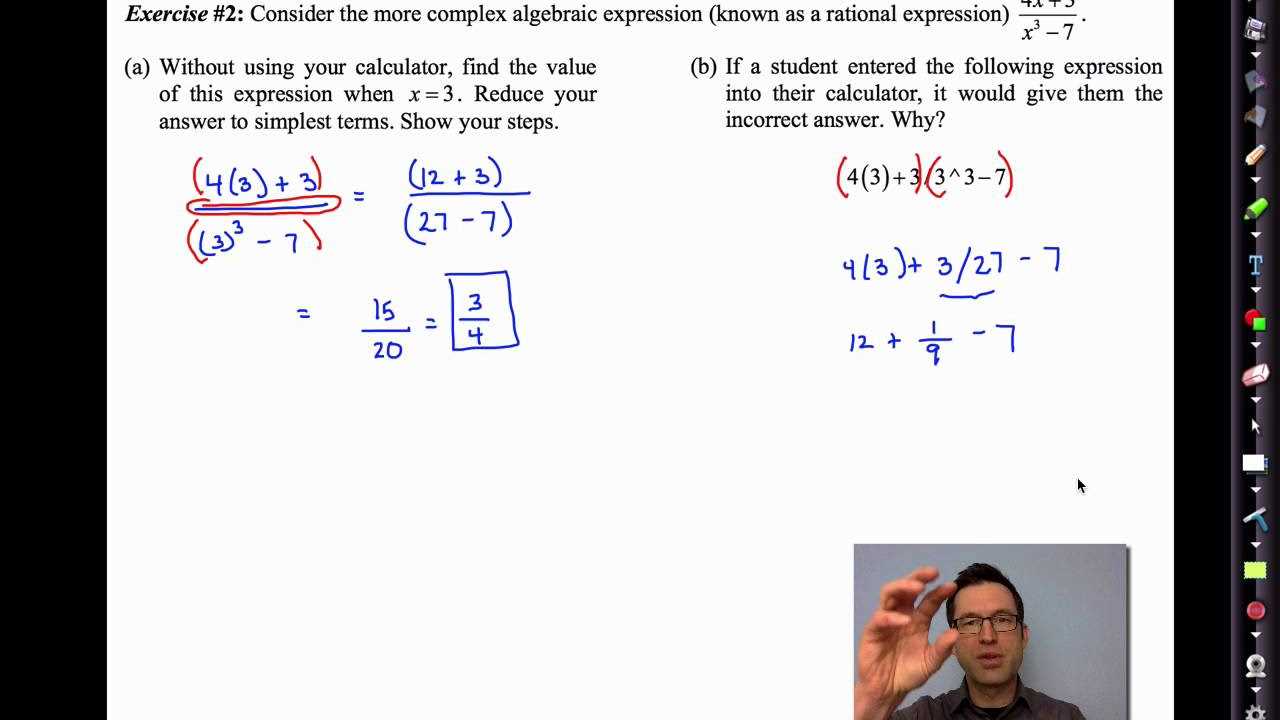
When you encounter a challenging problem, seeing the solution helps you understand the methodology behind it. By breaking down each step, you gain clarity on how to approach similar problems in the future. This understanding builds confidence and improves your ability to tackle difficult questions on your own.
- Visualizing the Solution Process: Observing a well-structured solution allows you to visualize the problem-solving process. This makes it easier to replicate the approach when faced with similar tasks.
- Identifying Common Mistakes: Reviewing solutions can help you recognize common errors that may occur during calculations or reasoning. This awareness prevents you from repeating these mistakes in the future.
- Building Problem-Solving Skills: By learning from the step-by-step breakdown of solutions, you gradually improve your ability to approach problems methodically and efficiently.
Boosting Retention and Mastery
Reviewing solutions also enhances long-term retention. By repeatedly checking your work and verifying the accuracy of your answers, you reinforce key concepts and formulas. This repetition strengthens neural connections, helping you recall important techniques when needed.
- Reinforcing Key Concepts: Seeing the application of formulas and theorems in real problems reinforces the connection between theory and practice.
- Improving Test Performance: Familiarity with correct solutions helps you perform better on exams by allowing you to work faster and more confidently under pressure.
- Identifying Knowledge Gaps: Reviewing solutions can also highlight areas where your understanding may be lacking, allowing you to focus your studies more effectively.
By actively engaging with solutions, you deepen your understanding and develop a more intuitive grasp of complex topics. This not only improves your academic performance but also builds skills that are valuable in various real-life situations.
Staying Motivated in Algebra 2
Maintaining enthusiasm during the study of advanced mathematical concepts can often be challenging. However, finding strategies that keep you engaged and motivated is key to not only succeeding but enjoying the learning process. Staying focused on the bigger picture, setting achievable goals, and celebrating small victories can help overcome obstacles and build momentum.
Set Clear Goals and Track Progress
One effective way to stay motivated is by breaking down the learning material into smaller, more manageable sections. Setting specific goals for each topic and tracking your progress can help you maintain a sense of accomplishment.
- Set Milestones: Break down your study sessions into smaller tasks, such as mastering specific formulas or completing practice problems.
- Track Achievements: Keep a record of your progress, whether through a planner, a digital tool, or simply crossing off completed topics.
- Celebrate Success: Reward yourself when you reach a goal, whether it’s taking a break or treating yourself to something special.
Engage with Practical Applications
Connecting abstract concepts to real-world applications can make learning feel more meaningful. By understanding how the skills you’re developing will be useful beyond the classroom, you’re more likely to stay motivated and engaged.
- Relate Concepts to Real Life: Think about how the mathematical principles you’re learning are used in various fields like engineering, finance, or even technology.
- Use Interactive Tools: There are many online resources and apps that make learning more interactive and fun. Experimenting with different learning tools can keep the material fresh and exciting.
- Find Study Partners: Collaborating with others not only helps you stay motivated but also gives you the opportunity to learn from peers and see different perspectives on solving problems.
By applying these techniques, you can stay motivated throughout your studies. Focus on progress rather than perfection, and remember that mastering complex concepts takes time and persistence. Each step you take brings you closer to your goal of mastering the material and succeeding in the course.