
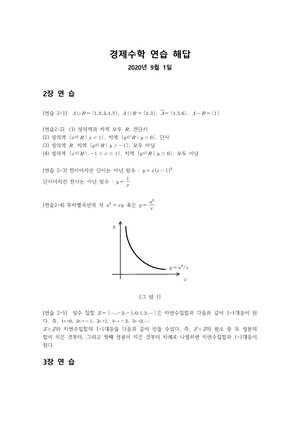
Understanding complex problem-solving methods is essential for mastering any subject. As students progress through various topics, it’s crucial to grasp the foundational principles and build confidence through practice. Breaking down difficult problems into manageable steps can make even the most challenging tasks achievable.
One effective way to improve comprehension is by reviewing detailed solutions. By examining each step, students can identify patterns and understand the reasoning behind each answer. This approach not only helps in solving similar problems but also strengthens critical thinking skills for future challenges.
In this guide, we will explore essential techniques and provide step-by-step breakdowns of key exercises. Whether you’re looking to clarify difficult concepts or simply need practice material, these resources will help enhance your understanding and boost overall performance. Emphasizing accuracy and clarity, the goal is to offer a deeper insight into each solution.
Big Ideas Math 2.1 Overview
Understanding the core concepts and building a solid foundation is crucial for progressing in any subject. The focus of this section is to explore the key components that help students master essential problem-solving techniques. This section offers a comprehensive look into the principles that guide effective learning in this area.
Core Principles and Approach
This section emphasizes a structured approach to solving problems. The primary objective is to break down complex exercises into understandable steps, allowing learners to recognize patterns and apply strategies more efficiently. By working through foundational principles, students can gain a deeper understanding and improve their problem-solving abilities.
- Focus on step-by-step solutions to increase clarity
- Identify patterns and strategies for easier problem resolution
- Use a methodical approach to tackle challenges with confidence
Skills and Techniques to Master
As students progress through exercises, they build valuable skills that can be applied to other subjects. These include logical thinking, pattern recognition, and critical analysis. With each new concept, students develop the ability to tackle problems from multiple angles, enhancing their overall comprehension.
- Logical thinking and reasoning for complex problem-solving
- Recognizing patterns and relationships between elements
- Refining analytical skills for deeper understanding
Key Concepts in Big Ideas Math
Mastering any subject requires a deep understanding of fundamental concepts. In this section, we focus on the essential principles that form the foundation for tackling various exercises and problems. By grasping these key ideas, students can build a stronger knowledge base and enhance their ability to approach challenges with confidence.
Important Foundations for Problem Solving
At the heart of successful problem solving lies the ability to break down complex situations into manageable parts. Key concepts often revolve around understanding relationships between variables, recognizing patterns, and applying strategies for logical reasoning. Students who focus on these elements will find it easier to work through new material and develop effective solutions.
- Breaking complex problems into simpler steps
- Identifying key relationships and their significance
- Applying systematic strategies for effective solutions
Critical Skills for Success
In addition to the core concepts, certain skills are essential for progressing through exercises and strengthening understanding. Logical reasoning, pattern recognition, and strategic thinking are crucial in mastering each concept and applying it in different contexts. These skills not only help with solving individual problems but also lay the groundwork for tackling more advanced material.
- Developing logical reasoning for clearer conclusions
- Recognizing recurring patterns for more efficient solutions
- Strategic thinking for tackling complex challenges
How to Approach Big Ideas Math 2.1
Approaching any set of exercises requires a thoughtful, step-by-step process to ensure thorough understanding. Whether the goal is mastering a specific topic or reinforcing general skills, it’s essential to follow a structured method that makes tackling each problem manageable and effective. This section highlights the strategies for building a solid foundation and confidently moving through exercises.
Step-by-Step Problem Solving
Effective problem-solving starts with breaking down each task into smaller, more digestible parts. Focusing on one element at a time helps prevent feeling overwhelmed. By addressing each component of the problem, students can piece together the full solution without missing any crucial steps.
- Read the problem carefully and identify key elements
- Divide complex tasks into smaller, manageable steps
- Check each step to ensure accuracy before moving forward
Building Strong Foundations
It’s important to understand the underlying principles before jumping into the problems themselves. Review the essential concepts and practice similar exercises to ensure clarity. By strengthening your grasp of core ideas, you’ll be able to approach more difficult questions with greater ease.
- Review fundamental principles before starting the exercises
- Practice solving simpler problems to build confidence
- Ensure a deep understanding of each key concept
Step-by-Step Problem Solving
Effective problem-solving involves breaking down complex tasks into smaller, more manageable steps. This approach not only helps to maintain focus but also ensures accuracy at each stage. By following a structured method, learners can build their confidence and tackle even the most challenging questions with ease.
Understanding the Problem
Before diving into solving, it’s essential to fully understand what the problem is asking. Take a moment to identify the key information and the goal of the task. By recognizing these critical components, you can prevent confusion and approach the exercise with a clear direction.
- Identify all given information and variables
- Understand the problem’s main objective
- Highlight important details that will guide the solution
Breaking Down the Solution
Once the problem is understood, the next step is to divide it into smaller, logical parts. This makes the entire process less overwhelming and allows for a more methodical approach. Tackle each section one at a time, verifying accuracy before moving forward. By following this pattern, you can avoid skipping steps and ensure that no details are missed.
- Divide the problem into smaller, logical sections
- Work through each part methodically and check your work
- Piece together the individual solutions to form the final answer
Tip: Keep track of your thought process as you solve each part. Writing down intermediate steps or making brief notes can help maintain clarity and prevent errors in the solution.
Understanding the Math Problems
To successfully solve any problem, it’s essential to first fully understand the situation being presented. The key lies in recognizing the underlying concepts and relationships within the task. Without a clear grasp of the problem, even simple tasks can become overwhelming, which is why careful analysis is crucial before jumping into solutions.
Breaking Down the Information
Start by identifying all the critical elements of the problem. This includes variables, constants, and any conditions or constraints that are mentioned. Understanding how these elements interact with one another is vital for developing a plan of action.
- Highlight the key pieces of information provided in the problem
- Look for relationships or connections between different parts
- Identify any hidden assumptions or requirements
Clarifying the Objective
Next, focus on understanding what the problem is asking for. The objective should be clear and concise, whether it involves finding a specific value, proving a relationship, or demonstrating a concept. This clarity will guide your approach to the solution.
- Determine what the problem is ultimately asking you to solve
- Understand whether the task is about computation or theory
- Ensure that you know the expected format for the answer
Tip: Re-read the problem several times to ensure complete comprehension before attempting a solution. This helps avoid common mistakes caused by misinterpreting key details.
Common Challenges in 2.1
While working through exercises and concepts, many learners encounter common obstacles that can hinder progress. These challenges often arise from misunderstandings of key principles or difficulties in applying the right strategies. Recognizing these issues early allows students to take corrective action and improve their approach to solving problems.
Misinterpreting Problem Statements
A frequent challenge is misunderstanding the question or failing to identify the essential information. Often, students may get caught up in details that are not critical to the solution, leading to confusion or incorrect answers. Clear comprehension of the problem is crucial to formulating the right approach.
- Failing to identify what is being asked
- Focusing on unnecessary details that complicate the solution
- Missing out on key relationships between variables
Difficulty Applying Concepts

Another common hurdle is the struggle to apply theoretical knowledge to practical problems. Students may understand the concepts but find it challenging to use them in the context of a specific problem. This gap often occurs when learners are unfamiliar with how to manipulate variables or connect abstract principles to concrete solutions.
- Challenges in applying formulas and equations correctly
- Difficulty recognizing patterns that simplify the process
- Not utilizing the most efficient methods for solving the problem
Using Big Ideas Math for Practice
Reinforcing understanding through practice is essential for mastering any subject. By consistently working through exercises and applying learned concepts, students can deepen their comprehension and improve their problem-solving abilities. Regular practice helps to identify weaknesses, sharpen skills, and build confidence in tackling more complex tasks.
Maximizing Learning with Regular Practice
Frequent engagement with exercises allows students to apply newly learned strategies and test their grasp of key concepts. The more a student practices, the more comfortable they become with recognizing patterns and applying solutions efficiently. This repetitive process solidifies learning and ensures that concepts are well understood.
- Set aside time each day to work on new problems
- Start with easier tasks and gradually progress to more difficult ones
- Review errors to understand where mistakes were made and avoid them in the future
Utilizing Resources for Better Results
Additional resources, such as online tools and practice tests, can complement regular study sessions. These resources provide diverse ways to approach the material and offer instant feedback, helping students adjust their methods and reinforce their knowledge. Making use of these tools allows for a well-rounded practice experience.
- Use practice problems to simulate real exam conditions
- Seek help from online platforms or peers when encountering difficulty
- Track progress over time to identify areas of improvement
Effective Study Tips
Studying efficiently requires more than just reading through materials; it involves developing strategies that enhance focus, retention, and understanding. By adopting effective study habits, students can maximize their learning and improve their performance. These tips focus on creating an environment and approach that promotes success in problem-solving and concept mastery.
Create a Structured Study Plan
Planning ahead is key to making the most of study time. A well-structured schedule allows students to allocate enough time for each topic and avoid last-minute cramming. Prioritizing areas that need the most attention ensures a more balanced and effective approach to learning.
- Set realistic, manageable goals for each study session
- Break study time into focused intervals with short breaks in between
- Prioritize harder topics earlier in the session when focus is at its peak
Use Active Learning Techniques
Active learning engages the brain in a way that passive reading cannot. Instead of simply memorizing information, students should actively work with the material by practicing problems, summarizing concepts in their own words, and teaching others. These activities reinforce knowledge and improve long-term retention.
- Practice solving problems to apply learned concepts
- Summarize key points after each study session
- Teach a concept to someone else to test understanding
Tip: Regularly assess your understanding by taking practice quizzes or discussing topics with peers to identify any gaps in knowledge.
Real-Life Applications of Math 2.1
Understanding mathematical concepts goes beyond solving exercises; it has practical applications in various real-world scenarios. The ability to apply these concepts helps individuals make informed decisions, solve everyday problems, and enhance their critical thinking. Whether managing personal finances or designing technology, these skills are essential in navigating daily life effectively.
Financial Management and Budgeting
One of the most direct applications of mathematical concepts is in managing personal finances. Budgeting, calculating interest rates, and tracking expenses all rely on core principles that students learn in their studies. Understanding how to apply these concepts ensures more effective financial planning and decision-making.
- Creating and managing a budget using basic arithmetic
- Calculating loan interest rates or savings growth
- Analyzing expenses to optimize financial resources
Engineering and Design
From architecture to technology, the design process involves applying mathematical concepts to create precise models and solutions. Engineers and designers rely on geometry, algebra, and data analysis to ensure structures are safe, products function properly, and systems operate efficiently.
- Designing blueprints for buildings and infrastructure
- Calculating measurements and material requirements
- Optimizing systems for better performance and cost efficiency
Data Analysis and Statistics
In today’s data-driven world, analyzing information is vital for making informed decisions. Statistical methods and mathematical modeling are used across industries to interpret data, forecast trends, and improve products or services. These techniques are used in everything from healthcare to marketing strategies.
- Interpreting survey results and market research data
- Forecasting sales and consumer behavior trends
- Assessing risks and probabilities in business decisions
Why It Matters in Daily Life
Understanding and applying mathematical concepts has a significant impact on everyday decision-making. From budgeting finances to planning a trip, the skills learned in problem-solving are useful in real-world scenarios. These concepts help individuals approach challenges with a structured and logical mindset, ensuring better outcomes in both personal and professional settings.
Practical Use in Financial Decisions
One of the most immediate ways math impacts daily life is in personal finance. Managing expenses, calculating savings, or even comparing prices all involve mathematical reasoning. Without this foundational knowledge, making sound financial decisions becomes challenging.
| Financial Decision | Mathematical Concept Used | Real-Life Example |
|---|---|---|
| Budgeting | Arithmetic | Determining monthly income and expenses |
| Saving for a goal | Percentages | Calculating interest rates for savings accounts |
| Comparing prices | Proportions and ratios | Determining which store offers the best deal |
Impact on Time Management and Planning
Effective time management often requires the use of calculations and measurements. Whether you’re planning a trip, organizing a project, or simply scheduling your day, math helps you allocate resources and time efficiently. Understanding how to balance tasks and deadlines ensures that everything is completed on time and within budget.
Answers to 2.1 Exercises Explained
Solving exercises is an essential part of mastering any topic, but understanding the solutions is just as important. By breaking down the steps involved in each problem, students can gain deeper insights into the underlying concepts. This section will provide a thorough explanation of the process used to arrive at the correct answers, ensuring clarity and a better grasp of the material.
Each exercise involves specific strategies and techniques that help simplify complex problems. Whether it’s applying algebraic methods, using logical reasoning, or interpreting data, knowing how to approach each type of question is crucial. The goal is not just to find the correct result, but also to understand why and how it is obtained.
By focusing on the steps, patterns, and rules used to solve each problem, learners can strengthen their critical thinking and problem-solving skills. This approach will not only help with exercises but also with practical applications in real life.
Solutions for Key Questions

Addressing the core questions within a topic allows for a deeper understanding of the material. By focusing on the essential concepts and systematically solving the problems, students can reinforce their knowledge and enhance their problem-solving abilities. This section will guide you through the solutions to the key questions, offering a clear and structured approach to finding the correct answers.
Breaking Down Complex Problems
Complex problems often require breaking them down into smaller, manageable parts. By isolating each component, it becomes easier to apply the right method and reach the solution step by step. Whether it’s simplifying equations or understanding relationships between variables, focusing on each part individually ensures a thorough understanding.
Common Mistakes to Avoid
While solving key questions, it’s important to be mindful of common mistakes. Misinterpreting the problem, skipping steps, or applying the wrong method can lead to incorrect results. Identifying and understanding these pitfalls will help in avoiding errors and ensuring accurate solutions.
Strategies for Mastering 2.1
Achieving proficiency in any subject requires a combination of effective strategies and consistent practice. Mastering a set of concepts involves not just understanding the core principles, but also applying them in various contexts. This section outlines key approaches to help solidify your understanding and enhance your problem-solving skills.
1. Break Down Problems into Steps
One of the most effective methods for mastering complex problems is to break them down into smaller, manageable parts. By focusing on one step at a time, you can reduce confusion and better understand each stage of the solution process. This approach makes even challenging problems more approachable.
2. Use Visual Aids and Diagrams
Sometimes, abstract concepts can be difficult to grasp without a clear visual representation. Sketching diagrams, charts, or graphs can help you see relationships more clearly. This method is especially useful in subjects that involve spatial reasoning or data analysis.
3. Practice Regularly
Consistency is key when it comes to mastering any skill. The more you practice, the more familiar you will become with the material, making it easier to solve problems quickly and accurately. Regular practice also helps reinforce key concepts, making them second nature.
4. Seek Clarification When Needed
If you encounter a concept that is difficult to understand, don’t hesitate to ask for help. Whether it’s from a teacher, tutor, or online resource, gaining clarification can help prevent misunderstandings and ensure you’re on the right track.
5. Review Mistakes
Looking over errors can be an invaluable learning experience. Analyzing what went wrong and understanding why helps you avoid repeating the same mistakes. This reflective approach is critical for continuous improvement.
Helpful Techniques for Success
Achieving success in any subject relies on adopting a set of effective techniques that enhance learning and improve retention. By incorporating the right strategies, students can approach challenges with confidence and improve their performance. Below are some key techniques that can make a significant difference in mastering complex topics and solving problems efficiently.
Effective Time Management
Proper time management is crucial for success. Organizing study sessions and allowing time for review ensures a balanced approach to learning. By creating a study schedule and sticking to it, you can maximize your focus and avoid feeling overwhelmed.
Active Learning Strategies
Passive reading often leads to limited understanding. Engaging with the material actively through methods like summarizing, questioning, or teaching others helps reinforce concepts and improves retention. Active learning encourages deeper thinking and better problem-solving skills.
Collaborative Study Sessions

Studying with peers can provide new perspectives and insights into difficult concepts. Discussing problems and sharing solutions in a group setting can help solidify your own understanding while exposing you to different approaches. Collaborative learning encourages critical thinking and teamwork.
| Technique | Benefit |
|---|---|
| Time Management | Helps avoid procrastination and ensures consistent study. |
| Active Learning | Promotes deeper understanding and memory retention. |
| Collaborative Study | Fosters teamwork and exposes you to different problem-solving methods. |
By applying these techniques consistently, students can build a strong foundation for success. These methods not only help in solving problems but also in developing a deeper understanding of the subject matter, which is essential for long-term academic growth.
Understanding Key Terms in Math 2.1
In any subject, mastering the key concepts and terminology is essential for success. A clear understanding of these terms provides the foundation for solving problems and applying theoretical knowledge to real-world situations. This section focuses on explaining important concepts that are fundamental to the lessons and exercises at this level.
Basic Concepts
At the core of any lesson are fundamental principles that shape the way problems are approached. These concepts serve as the building blocks for more advanced topics. A solid grasp of the basics ensures that students can tackle complex problems with ease.
Mathematical Operations
Different operations form the foundation of problem-solving. Whether it is addition, subtraction, multiplication, or division, each operation plays a specific role in helping to find solutions. Understanding how and when to apply these operations is critical for solving equations and understanding their applications.
Being able to identify and define key terms allows learners to navigate lessons more effectively. Without a clear understanding of these concepts, it becomes difficult to build on the knowledge and proceed to more challenging material. Therefore, mastering the terminology is a crucial step in becoming proficient in any area of study.
Glossary of Essential Concepts
Having a clear understanding of key terms is vital for grasping any subject. This section provides a glossary of fundamental concepts that are crucial for successfully navigating lessons and solving related problems. Familiarity with these terms will not only enhance comprehension but also allow for a smoother progression through more complex topics.
| Term | Definition |
|---|---|
| Equation | A mathematical statement that shows the equality of two expressions, typically involving variables and constants. |
| Variable | A symbol, often a letter, that represents an unknown or changing value in an expression or equation. |
| Coefficient | A numerical factor that multiplies a variable in an algebraic expression. |
| Solution | The value or set of values that satisfy an equation or inequality. |
| Expression | A combination of numbers, variables, and operators that represents a value but does not include an equality sign. |
| Factor | A number or expression that divides another number or expression evenly. |
| Linear Equation | An equation that represents a straight line when graphed, typically in the form of ax + b = c. |
| Function | A relationship between input and output where each input is related to exactly one output. |
| Graph | A visual representation of data or mathematical relationships, typically plotted on a coordinate plane. |
Understanding these key concepts is essential for successfully tackling exercises and applying them to real-world situations. By learning and using these terms, students can navigate complex topics with greater ease and clarity.
Practice Problems for Math 2.1
Engaging in practice problems is one of the most effective ways to reinforce understanding and develop problem-solving skills. By regularly applying learned concepts to various exercises, students can improve their grasp of core principles and refine their analytical abilities. This section provides a range of problems designed to test and strengthen the skills acquired throughout the lessons.
Problem Set 1: Basic Operations
Solve the following problems involving basic arithmetic operations:
- 1. Solve for x: 3x + 5 = 20
- 2. Simplify: 12 ÷ (3 + 1)
- 3. Multiply: (4 + 2) × 5
- 4. Subtract: 18 – 7 × 2
Problem Set 2: Algebraic Expressions

Work through the following algebraic expressions:
- 1. Simplify: 2a + 3b – 5a + 7b
- 2. Solve for y: 4y – 8 = 16
- 3. Factor: x² – 5x + 6
- 4. Expand: (x – 3)(x + 2)
Problem Set 3: Graphing and Functions
Practice graphing and solving function-related problems:
- 1. Plot the equation y = 2x + 3 on a graph.
- 2. Find the slope and y-intercept of the equation y = -4x + 7.
- 3. Determine if the equation y = x² + 4x + 4 represents a parabola. If so, graph it.
- 4. Solve for y: 2x – 3y = 6
Working through these exercises will solidify your understanding of core principles and improve your ability to apply them in various contexts. Regular practice is key to mastering concepts and excelling in future challenges.
Additional Exercises to Try
To further enhance your skills and deepen your understanding, it’s essential to challenge yourself with additional exercises. These problems are designed to extend your knowledge and help you become more comfortable with applying concepts in different scenarios. By tackling these exercises, you can strengthen areas where you may need more practice and improve your overall problem-solving ability.
- 1. Solve for x: 5(x – 3) = 2x + 7
- 2. Simplify the expression: 6x² – 3x + 4x² + 2x
- 3. Find the value of y when y = 3x + 5 and x = -2.
- 4. Solve for z: 2z/3 + 4 = 10
- 5. Factor the quadratic expression: x² – 7x + 12
- 6. Simplify: (3a + 5)² – (a – 2)²
- 7. Graph the linear function: y = -2x + 4 and identify the slope and y-intercept.
By regularly solving problems of varying complexity, you’ll build confidence and refine your skills. The more problems you attempt, the more proficient you’ll become at identifying patterns and developing efficient strategies for problem-solving.