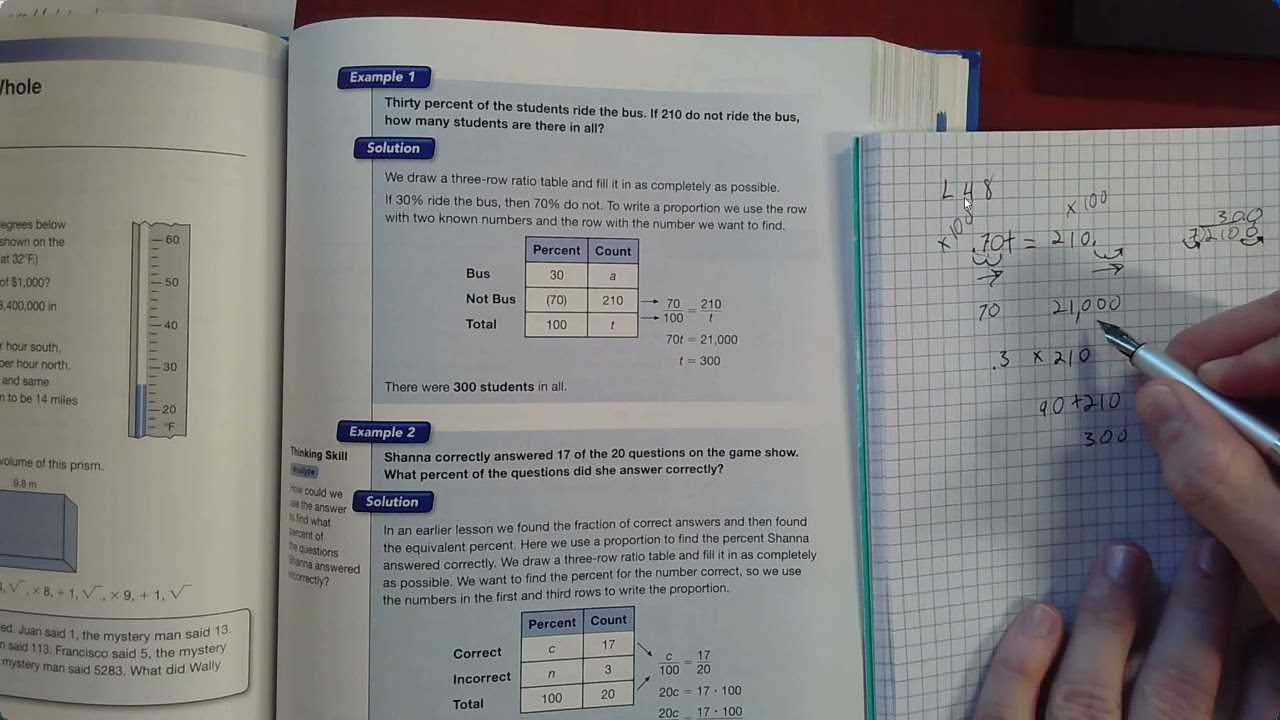
Mastering the concepts of advanced mathematics requires a structured approach, where practice, understanding, and strategy combine to foster deeper knowledge. This section provides comprehensive explanations to help learners navigate complex problems and reinforce their skills. Whether you’re solving algebraic expressions or tackling geometry, having access to clear solutions is key to mastering the material.
Clarifying concepts through systematic problem-solving enables a deeper understanding of the principles behind each lesson. It’s not just about getting the right result, but about grasping the reasoning and methods that lead to that outcome. With step-by-step breakdowns, students can improve their problem-solving abilities and enhance retention.
In addition to step-by-step instructions, effective practice is vital for reinforcing learning. Regularly engaging with a variety of questions allows for better adaptability and boosts confidence in applying techniques across different scenarios. This section aims to guide learners through their challenges, providing practical insights that will aid in building a solid mathematical foundation.
Understanding Saxon Math Course 3

Grasping the foundational principles of advanced mathematics requires a methodical approach that balances theory with practice. The curriculum is designed to guide students through progressively more complex topics, ensuring each concept is fully understood before moving to the next. This method allows learners to build strong problem-solving skills and gain confidence in tackling a wide range of mathematical challenges.
Key Topics and Skills
The study materials encompass several critical areas that are vital for further progression in mathematics. By focusing on core topics, students will not only master the necessary operations but also develop the ability to apply these skills in various scenarios. Here are some of the major topics covered:
- Algebraic expressions and equations
- Understanding fractions and decimals
- Geometric principles and shapes
- Measurement and data analysis
- Problem-solving techniques
Why This Approach Works
One of the unique aspects of this approach is its emphasis on consistent reinforcement. Each lesson builds on the previous one, ensuring that students remain engaged while mastering key concepts. This method allows for better retention and understanding, enabling students to apply their knowledge with greater ease as they progress.
Incorporating regular review exercises also helps solidify learning. By revisiting challenging concepts through guided practice, learners can identify areas of weakness and address them promptly. This repetition fosters confidence and a deeper understanding of the material.
Overview of Course Structure
The structure of this educational program is carefully crafted to ensure a comprehensive learning experience, with a logical flow from basic to advanced concepts. Each unit introduces new skills while reinforcing previous knowledge, allowing students to build a solid foundation before moving on to more complex topics. The material is organized in a way that encourages consistent practice and mastery through repetition.
Progressive Learning Approach
Throughout the lessons, students will encounter a variety of topics designed to develop both computational skills and critical thinking. The curriculum begins with fundamental principles, then gradually introduces more sophisticated concepts. This method ensures that learners fully grasp each topic before progressing to more challenging material, enabling a smoother transition through the learning stages.
Key Features of the Structure
Several elements are incorporated into the program to enhance understanding and retention:
- Clear explanations and definitions for each concept
- Frequent practice exercises to reinforce learning
- Real-world applications to connect theory with practical use
- Regular assessments to gauge progress and identify areas for improvement
By integrating these features, the structure supports gradual mastery of essential skills, ensuring learners are prepared for more advanced mathematical challenges.
Key Concepts Covered in Course 3
This section delves into the core topics explored in the program, providing a deep understanding of fundamental principles while preparing students for more advanced study. The material emphasizes both theoretical knowledge and practical application, ensuring that learners can effectively apply their skills to solve a wide range of problems. Each key concept is introduced in a clear, digestible format, with plenty of opportunities for practice and mastery.
Core Areas of Focus
The curriculum covers a wide variety of topics essential for building a solid mathematical foundation. Key areas include:
- Operations with fractions and decimals
- Solving equations and inequalities
- Introduction to geometric concepts and shapes
- Working with ratios and proportions
- Understanding percentages and their applications
- Exploring basic probability and statistics
Important Skills Developed
Beyond content knowledge, students will develop critical problem-solving skills. Some of the key abilities nurtured throughout the program include:
- Breaking down complex problems into manageable steps
- Applying logical reasoning to find solutions
- Interpreting mathematical results and making connections
- Using formulas and strategies to solve real-world challenges
By mastering these concepts and skills, learners will be well-equipped to handle more advanced topics in the future.
Step-by-Step Solutions for Exercises
Effective learning relies heavily on understanding the process behind each solution, not just arriving at the final answer. By breaking down complex problems into manageable steps, students can learn how to approach similar tasks in the future with confidence. This section provides clear, methodical instructions to guide learners through various exercises, ensuring a deeper understanding of the concepts being applied.
Each solution is broken down into logical steps, allowing students to follow the reasoning behind each action. By focusing on the methodology, learners not only achieve the correct result but also gain the skills to solve new problems independently. This approach fosters critical thinking and improves overall problem-solving abilities, setting a solid foundation for tackling more advanced challenges.
Whether it’s an algebraic equation or a geometric problem, the process remains consistent. By following a step-by-step format, students can see how to organize their work, check their reasoning, and apply the appropriate techniques at each stage. This methodical approach enhances both retention and mastery of the material.
How to Approach Word Problems
Word problems often present a challenge due to their real-world context and the need for careful interpretation. To solve these types of problems, it’s important to break them down into smaller, manageable parts. By analyzing the information given and identifying what needs to be found, students can develop a systematic approach that leads to a solution.
The key to solving word problems is understanding the relationships between the given data. Start by identifying the question being asked and highlighting the important details. Next, translate these details into mathematical expressions or equations. This process involves recognizing patterns, understanding how quantities relate, and choosing the appropriate operation to apply.
Additionally, it’s helpful to check the solution in the context of the problem to ensure it makes sense. This step not only confirms the accuracy of the answer but also strengthens the problem-solving process. By practicing this approach, learners can improve their ability to solve word problems confidently and efficiently.
Common Mistakes to Avoid
When working through mathematical problems, it’s easy to fall into certain traps that can lead to incorrect solutions. These errors often stem from misunderstanding the problem, skipping important steps, or applying the wrong techniques. Being aware of these common mistakes can help students avoid them and improve their accuracy in solving problems.
Misinterpreting the Question
One of the most frequent mistakes is failing to fully understand what is being asked. Many problems include extra information that can distract from the core question. It’s essential to read the problem carefully, highlight key details, and focus on identifying the exact goal. Misunderstanding the requirements can lead to the wrong method or answer.
Rushing Through Steps
Another common error is moving too quickly through the steps without fully considering each one. Skipping intermediate steps or rushing through calculations can lead to careless mistakes. Taking the time to work through each part methodically ensures that nothing is overlooked, and the solution is more likely to be accurate.
By staying aware of these common pitfalls and practicing a careful, thoughtful approach, learners can minimize errors and build a stronger foundation in solving problems effectively.
Using Online Resources for Extra Help
In today’s digital age, students have access to a wealth of online tools that can provide valuable support when facing difficulties with various topics. These resources offer explanations, tutorials, and practice exercises that can enhance understanding and provide alternative ways to approach problems. Utilizing these online platforms can complement traditional learning methods and help students progress more efficiently.
From interactive problem solvers to video tutorials, there are numerous websites that cater to different learning styles. Some platforms provide detailed step-by-step solutions, while others offer practice exercises with instant feedback. These resources are designed to address common areas of struggle, such as understanding complex concepts or improving problem-solving skills.
Additionally, online forums and discussion boards allow learners to ask questions and engage with a community of peers or experts. This collaborative environment can provide insights and tips that may not be covered in textbooks, helping students gain a deeper understanding of the material. By taking advantage of these online tools, students can supplement their studies and overcome challenges more effectively.
Tips for Mastering Algebra in Course 3
Algebra is a key area in mathematics that often requires both logical reasoning and practice to master. The process of solving equations, working with variables, and understanding mathematical relationships can be challenging, but with the right strategies, students can gain confidence and proficiency. This section provides practical tips for improving your algebraic skills and making the learning process more effective.
Building a Strong Foundation
Success in algebra begins with mastering the basic principles. Without a solid understanding of foundational concepts, it can be difficult to tackle more advanced topics. Focus on these essential skills:
- Understand the meaning of variables and constants
- Practice solving simple equations to build confidence
- Learn how to manipulate expressions and simplify them
- Get comfortable with basic operations such as addition, subtraction, multiplication, and division of algebraic terms
Effective Problem-Solving Strategies
Once the basics are clear, it’s important to approach problems methodically. These strategies will help streamline the process:
- Read the problem carefully and identify the unknowns
- Set up the equation step by step, ensuring that all operations are correct
- Check your work at each stage to avoid small mistakes
- Practice regularly to improve speed and accuracy
By applying these tips consistently, students can gain a deeper understanding of algebra and develop the skills needed to solve more complex problems with ease.
Understanding Fractions and Decimals
Both fractions and decimals are essential tools for representing parts of a whole. These two formats allow us to express numerical relationships and make calculations involving divisions, proportions, and measurements. Understanding how to work with both forms and convert between them is key to solving a wide variety of problems. This section explores how fractions and decimals function and how to manipulate them effectively.
Fractions and decimals represent the same concept in different ways: fractions use a numerator and denominator to show the relationship between the parts and the whole, while decimals express this relationship using a base-10 system. Both are useful in different contexts, and mastering both forms enhances overall numerical fluency.
Converting Between Fractions and Decimals

Knowing how to convert between these two forms is vital. Here’s a simple guide to converting fractions to decimals and vice versa:
| Fraction | Decimal |
|---|---|
| 1/2 | 0.5 |
| 3/4 | 0.75 |
| 1/5 | 0.2 |
| 2/3 | 0.666… |
To convert a fraction to a decimal, divide the numerator by the denominator. On the other hand, to convert a decimal to a fraction, write the decimal as a ratio of integers. For example, 0.75 can be written as 75/100, which simplifies to 3/4.
Practical Uses of Fractions and Decimals

Fractions and decimals are used in many everyday applications, from cooking and construction to finance and statistics. Whether you’re dividing a pizza into equal slices or calculating a discount on a purchase, understanding how to work with both forms is essential. Regular practice with fractions and decimals helps build confidence and ensures accuracy in both academic and real-world situations.
Geometry Skills You Need to Know

Geometry plays a crucial role in understanding the properties and relationships of shapes, angles, and space. Whether you are working with simple figures or tackling more complex problems, having a strong foundation in geometric concepts is essential. In this section, we will cover key skills and techniques that are vital for mastering geometry and solving related problems effectively.
From calculating areas and volumes to understanding theorems and working with different types of angles, geometry is not just about memorizing formulas but about developing a deeper understanding of spatial reasoning. Mastery of geometric skills is necessary for solving practical problems in various fields, including architecture, engineering, and design.
Key Geometric Concepts

To build your geometry skills, it’s important to focus on several core concepts:
- Understanding basic shapes such as triangles, rectangles, and circles
- Knowing how to calculate areas and perimeters of different figures
- Mastering the Pythagorean theorem and its applications
- Identifying and calculating angles in various polygons
- Understanding symmetry and transformations like translation and rotation
Practical Applications of Geometry
Geometry is not only useful in theoretical problems but also has many real-world applications. From designing buildings and bridges to creating art and navigation systems, geometric principles are applied in numerous industries. By practicing and applying geometric skills, you develop a valuable toolkit that can be used in both academic and professional contexts.
Practice Tests and Answer Keys
Regular practice is key to mastering any subject, and working through tests is one of the best ways to reinforce knowledge and improve problem-solving skills. Practice tests help simulate the conditions of a real exam, allowing students to familiarize themselves with the format and types of questions they will encounter. Using answer keys provides an essential resource for checking solutions and understanding mistakes, leading to better learning outcomes.
By completing practice tests and reviewing the answers, students can assess their progress, identify weak areas, and focus their efforts on topics that need improvement. The goal is not just to complete exercises but to gain a deeper understanding of the underlying concepts and techniques.
Sample Practice Test
Here is an example of a practice test that can help solidify understanding of key concepts. The test includes various types of problems designed to cover a range of topics and difficulty levels.
| Question | Topic | Difficulty |
|---|---|---|
| What is the area of a rectangle with length 5 and width 3? | Geometry | Easy |
| Solve for x: 2x + 5 = 15 | Algebra | Medium |
| Find the perimeter of a triangle with sides 7, 8, and 10. | Geometry | Medium |
| Convert 0.75 to a fraction. | Decimals/Fractions | Easy |
Answer Key and Explanation

After completing the test, it’s crucial to review the solutions to understand any errors and improve accuracy. Here’s how to check your work:
| Question | Correct Answer | Explanation |
|---|---|---|
| What is the area of a rectangle with length 5 and width 3? | 15 | Area = length × width = 5 × 3 = 15 |
| Solve for x: 2x + 5 = 15 | x = 5 | Subtract 5 from both sides, then divide by 2: 2x = 10, x = 5 |
| Find the perimeter of a triangle with sides 7, 8, and 10. | 25 | Perimeter = 7 + 8 + 10 = 25 |
| Convert 0.75 to a fraction. | 3/4 | Write 0.75 as 75/100 and simplify: 75/100 = 3/4 |
By consistently working through practice tests and checking solutions, students can track their improvement and develop a more thorough understanding of the subject matter.
Strategies for Retaining Math Knowledge

Mastering a subject requires more than just understanding the concepts; it requires effective strategies to retain and apply the knowledge over time. Retention in any discipline, particularly in areas involving problem-solving and calculations, hinges on consistent practice, active engagement, and the use of diverse techniques that promote long-term memory.
One of the most powerful methods for retaining knowledge is active repetition. Regular practice not only reinforces concepts but also allows learners to apply them in various contexts, strengthening their ability to recall and use the information effectively. Another important approach is conceptual learning, which focuses on understanding the underlying principles rather than just memorizing formulas or procedures.
Reinforce with Practice

Practice should be viewed as more than a means of testing knowledge–it is essential for mastery. The key is not just to practice more but to practice effectively. Here’s how:
- Daily exercises: Set aside time each day for practice. Even brief sessions can help reinforce concepts and improve recall.
- Variety in problems: Work on different types of problems to ensure that you can apply the concepts in multiple ways and under different conditions.
- Mock tests: Taking full-length practice tests can help simulate exam conditions and build confidence.
Active Engagement Techniques
Learning by actively engaging with the material ensures that it is stored in long-term memory. This can be done through several methods:
- Teach what you learn: Explaining concepts to others forces you to fully understand the material and identify any gaps in your knowledge.
- Visualization: Use diagrams, graphs, or charts to visualize problems and solutions. This can make abstract concepts more concrete and easier to remember.
- Use real-world examples: Apply the learned concepts to real-world scenarios to see their practical value, making them easier to recall when needed.
Incorporating these strategies into your study routine can significantly enhance your ability to retain and apply mathematical concepts, leading to better results in both short-term learning and long-term mastery.
How to Improve Problem-Solving Speed
Being able to solve problems quickly and accurately is a valuable skill, especially when faced with time constraints. Improving speed in solving challenges requires a combination of practice, strategic thinking, and a deep understanding of the methods used. The more familiar you are with the different types of problems and approaches, the faster and more efficiently you can solve them.
One key to improving speed is developing an intuition for recognizing patterns. This allows you to quickly identify the best approach for a given problem without needing to go through every possible method. Additionally, practicing common techniques under time pressure can help to build confidence and minimize hesitation.
Techniques for Speed Improvement

There are several strategies that can be employed to boost your problem-solving speed:
- Practice regularly: The more you practice, the more familiar you become with various problem types, which leads to quicker recognition of patterns and solutions.
- Understand the underlying principles: Gaining a deep understanding of the core concepts behind the problems allows you to apply techniques quickly and with more accuracy.
- Break down the problem: When faced with complex issues, break them into smaller, manageable parts. This reduces the feeling of being overwhelmed and allows you to address each part more efficiently.
- Time yourself: Practice solving problems with a timer. This will help you get used to working under time constraints and improve your ability to think quickly.
Minimizing Mistakes Under Pressure
Speed should not come at the expense of accuracy. While it’s important to work quickly, you must also ensure that you don’t make unnecessary errors. To avoid this:
- Stay organized: Keep your work neat and well-organized. This makes it easier to spot mistakes before they become larger issues.
- Double-check key steps: For each solution, quickly review the major steps to ensure no errors have been made.
- Use shortcuts: Learn efficient techniques for common operations (like mental math) to save time and reduce the risk of mistakes.
With consistent practice and the application of these strategies, problem-solving speed will improve, allowing you to tackle challenges more effectively and efficiently.
Utilizing Saxon Math Answer Manuals
Reference guides are essential tools for students working through challenging exercises. These manuals not only provide the correct solutions but also offer a structured approach to understanding the steps involved. By using these resources effectively, learners can improve their comprehension, identify errors, and reinforce their problem-solving skills. The key to making the most of these guides is understanding how to use them as a learning tool, rather than just for checking answers.
One of the primary benefits of these manuals is their ability to break down complex problems into manageable steps. By following each solution carefully, students can learn the process behind each problem type and apply it to similar questions. This not only helps with current assignments but also builds a stronger foundation for future topics.
To maximize the value of these reference materials, consider the following strategies:
- Work through problems independently first: Attempt each exercise on your own before consulting the manual. This promotes active learning and problem-solving practice.
- Study the solution process: Focus on the steps provided in the manual. Understand why each step is taken and how it leads to the final solution.
- Identify patterns: As you progress through exercises, take note of common methods used for solving similar problems. This will make future exercises easier to tackle.
- Review mistakes: If you make an error, revisit the solution guide to understand where you went wrong. This helps reinforce concepts and correct misunderstandings.
In addition to helping with homework, these guides can be an excellent resource for preparing for exams. By regularly reviewing solutions and practicing similar problems, students can enhance their skills and build confidence in their abilities.
How to Review Mistakes Effectively
Analyzing errors is a crucial part of the learning process. Instead of simply identifying where you went wrong, reviewing mistakes with a focused approach can deepen your understanding and prevent the same mistakes in the future. The key is to break down each mistake systematically and understand why it occurred, then apply the lessons learned to future exercises. This process helps reinforce concepts and boosts confidence in solving similar problems.
When reviewing your mistakes, consider the following strategies to maximize learning:
- Identify the root cause: Ask yourself why the mistake happened. Was it a misunderstanding of the concept, a miscalculation, or a lapse in following steps? Understanding the cause will help you avoid the same error in the future.
- Rework the problem: After identifying the mistake, try solving the problem again from scratch. This reinforces the correct method and helps solidify the concept in your mind.
- Take notes: Write down key points about the mistake and how to avoid it next time. Keeping a record of common errors can be useful for future reference and exam preparation.
- Seek alternative explanations: Sometimes, reviewing the concept from a different perspective can help clarify any confusion. Look for other examples or explanations that might offer new insights.
- Practice similar problems: Once you understand the mistake, practice solving similar problems. Repetition will help solidify the correct method and improve your problem-solving speed.
By actively engaging in error review, you create a learning cycle that leads to continual improvement. Not only will this help you become more accurate, but it will also build your ability to approach problems with confidence and clarity.
Helpful Study Techniques for Success
Effective study habits are essential for mastering new material and excelling in exams. The way you approach your study sessions can make a significant difference in how well you retain information and solve problems. By implementing structured techniques and using proven strategies, you can enhance your understanding and boost your performance.
Here are some study techniques that can help you achieve success:
- Active Recall: Testing yourself on the material rather than passively rereading notes helps strengthen memory. Practice solving problems from memory to ensure you fully understand the concepts.
- Spaced Repetition: Review material multiple times over increasing intervals. This technique is proven to improve long-term retention and helps prevent forgetting.
- Break Down Complex Topics: When faced with challenging content, break it into smaller, more manageable parts. Tackle one section at a time and build your understanding gradually.
- Teach Someone Else: Explaining concepts to others forces you to think critically and reinforces your understanding. It’s a great way to spot any gaps in your knowledge.
- Use Visual Aids: Diagrams, charts, and tables can help you better understand relationships and processes. Visualizing the material often makes complex ideas easier to grasp.
- Practice Consistently: Consistent, focused practice is key to mastering any subject. Set aside regular time each day for studying and review previous topics regularly.
The key to success is not only working hard but also working smart. Applying these techniques can help you study more efficiently, retain information longer, and approach challenges with greater confidence. Use a combination of these methods to find what works best for you.
| Study Technique | Benefits |
|---|---|
| Active Recall | Improves long-term memory retention and reinforces learning. |
| Spaced Repetition | Helps prevent forgetting and enhances retention over time. |
| Breaking Down Topics | Makes complex material more approachable and manageable. |
| Teaching Others | Strengthens understanding and reveals any gaps in knowledge. |
| Using Visual Aids | Improves comprehension by providing a clearer perspective on concepts. |
| Consistent Practice | Develops proficiency and ensures long-term retention. |
Tracking Progress Throughout the Course
Monitoring your development throughout an educational journey is crucial for staying on track and ensuring continued improvement. By regularly assessing your understanding and performance, you can identify areas of strength as well as those that may require additional focus. This process not only helps you gauge your progress but also motivates you to stay engaged and committed to your studies.
Here are some strategies to effectively track your progress:
- Set Clear Goals: Start by defining specific learning objectives for each section. This allows you to measure how well you’ve met each goal and adjust your study plan as needed.
- Keep a Learning Journal: Document your learning process by noting down key concepts, challenges, and breakthroughs. Regular reflection helps you track progress and reinforces retention.
- Regular Self-Quizzes: Test your knowledge frequently to assess how well you understand the material. Self-assessment is a valuable tool to identify areas needing improvement before moving on to new topics.
- Use Progress Tracking Tools: Many online platforms and learning management systems offer built-in progress trackers that visually represent your learning path. Utilize these tools to keep an overview of your advancement.
- Analyze Mistakes: When errors occur, take time to understand why they happened. Reviewing mistakes helps you identify misconceptions and reinforces correct methods.
- Seek Feedback: Regularly discuss your progress with teachers, tutors, or peers. Feedback from others provides fresh insights and can help you improve more quickly.
By following these methods, you will create a comprehensive system to track your growth and identify areas that require more attention. Regular tracking not only helps you stay organized but also keeps you motivated throughout your learning experience.