
Approaching an important assessment can often be overwhelming, but with the right preparation, you can approach it with confidence. A solid understanding of the key concepts and a well-thought-out study plan are crucial for success. This section is designed to help you navigate the most important areas to focus on as you prepare.
Throughout this guide, you’ll find helpful tips, strategies, and a breakdown of the core topics to review. From tackling complex equations to mastering geometric shapes, each section will provide the tools you need to approach the test effectively. Whether you are reviewing for the first time or refining your skills, these insights will help you achieve your best possible performance.
Stay organized, stay focused, and trust in your abilities.
Study Guide for Your Upcoming Assessment
Effective preparation is essential for performing well in an important evaluation. To succeed, it’s vital to focus on key concepts, practice problem-solving techniques, and manage your time wisely. This guide will help you navigate the essential topics that are likely to appear and offer advice on how to tackle them.
Core Topics to Focus On
Understanding and mastering the primary topics is crucial. Focus on the areas that are most frequently tested, and allocate more time to those that you find most challenging. Below is a list of essential topics to review:
| Topic | Key Concepts |
|---|---|
| Algebra | Linear equations, inequalities, and systems of equations |
| Geometry | Angles, shapes, area, and volume |
| Statistics | Mean, median, mode, probability |
| Functions | Understanding and graphing different types of functions |
Effective Study Strategies
To maximize your study sessions, use active learning techniques such as practice tests and quizzes. Spend time working through sample problems to identify areas of weakness and reinforce your strengths. Remember, consistent practice is key to retaining information and improving your problem-solving abilities.
Essential Topics to Focus On
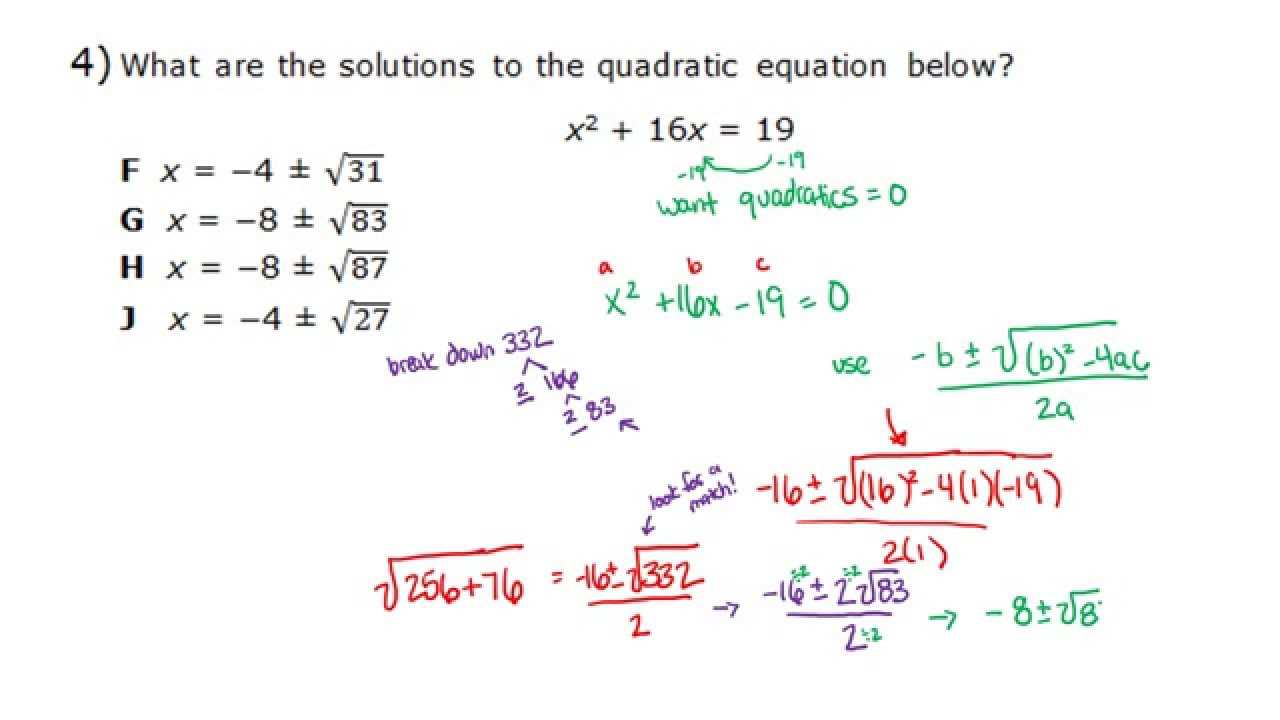
To prepare effectively for your upcoming evaluation, it’s crucial to prioritize the most important concepts. Focusing on key areas will help you strengthen your skills and ensure you are well-equipped to tackle a variety of questions. The following topics are essential for building a strong foundation and achieving success.
| Topic | Key Concepts |
|---|---|
| Algebraic Expressions | Simplifying, factoring, and solving equations |
| Geometry | Shapes, angles, areas, and volumes |
| Linear Relationships | Slope, intercepts, and graphing |
| Number Operations | Fractions, decimals, and percentages |
| Word Problems | Translating problems into mathematical equations |
Understanding Key Algebra Concepts
Algebra forms the foundation of many mathematical problems, and understanding its core principles is essential for solving a wide range of questions. Mastering the basics of algebra helps to build problem-solving skills and enables you to approach more complex tasks with confidence.
Here are some key areas to focus on when studying algebra:
- Variables and Constants: Understanding the role of variables and constants in equations and expressions.
- Expressions and Equations: Learning how to simplify, expand, and solve algebraic expressions and equations.
- Linear Equations: Solving equations with one variable and understanding their graphical representation.
- Factoring: Recognizing common patterns and factoring quadratic expressions and polynomials.
- Systems of Equations: Solving sets of linear equations using methods like substitution and elimination.
By practicing these core topics, you will develop the skills needed to handle a variety of algebraic problems with ease. The more you familiarize yourself with these concepts, the better prepared you will be to tackle questions in future assessments.
Mastering Geometry for the Assessment
Geometry plays a significant role in many assessments, and having a strong understanding of its key concepts is vital. By focusing on the basic principles of shapes, angles, and measurements, you can develop the skills needed to tackle geometric problems with confidence. This section will help you focus on the areas most likely to appear, ensuring you’re fully prepared to handle any related questions.
Key Concepts to Review
When preparing for geometric problems, it’s essential to be familiar with the following topics:
- Angles: Understand types of angles (acute, obtuse, right) and how to calculate missing angles in various shapes.
- Triangles: Focus on the properties of different types of triangles (equilateral, isosceles, and scalene) and learn how to apply the Pythagorean theorem.
- Area and Perimeter: Practice calculating the area and perimeter of various shapes, including squares, rectangles, circles, and triangles.
- Volume: Review formulas for the volume of 3D shapes such as cubes, cylinders, and spheres.
- Coordinate Geometry: Be able to plot points on the coordinate plane and solve problems involving the distance and midpoint formulas.
Effective Problem-Solving Strategies
To successfully solve geometric problems, it’s important to apply the correct approach. Start by identifying the key information in the problem and determining which formulas are needed. Practice sketching diagrams to visualize the situation, and always check your work for accuracy.
Tips for Solving Word Problems
Word problems often present challenges, but with the right approach, they can become much easier to solve. These problems require you to translate real-world situations into mathematical expressions, and the key to success is understanding how to break down the information and apply the appropriate strategies. This section will guide you through the steps to efficiently tackle word problems.
Step-by-Step Approach
Follow these steps to solve word problems effectively:
- Read Carefully: Carefully read the problem to identify key information. Pay attention to units, numbers, and any relationships between them.
- Identify What Is Being Asked: Focus on what the problem is asking for. This will help you determine which operation or method to use.
- Translate Into Equations: Convert the words into a mathematical equation or expression. Look for keywords that indicate operations, such as “sum,” “difference,” or “product.”
- Solve the Equation: Once the equation is set, solve it as you would with any other problem. Be sure to double-check your work.
- Check Your Answer: Verify that your answer makes sense in the context of the problem. If the result doesn’t seem logical, recheck your work for errors.
Common Pitfalls to Avoid
When solving word problems, there are several common mistakes to watch out for:
- Misinterpreting the Question: Ensure you fully understand what is being asked before attempting to solve the problem.
- Skipping Units: Always include units in your calculations and answer. Forgetting them can lead to confusion.
- Overcomplicating the Problem: Simplify the problem step by step. Avoid jumping ahead or overthinking the solution.
By practicing these strategies and avoiding common mistakes, you’ll be better prepared to handle word problems with ease.
Common Mistakes to Avoid
Even with thorough preparation, certain mistakes can undermine your performance. Being aware of these common errors can help you avoid them during your assessment. By recognizing potential pitfalls and understanding how to prevent them, you can improve both your accuracy and confidence.
Common Errors in Problem Solving
Here are some frequent mistakes to watch out for when solving mathematical problems:
- Rushing Through the Question: It’s easy to misinterpret a problem when you’re in a hurry. Always take a moment to read the question thoroughly and ensure you understand what is being asked.
- Forgetting to Simplify: After solving an equation or expression, be sure to simplify your answer. Not doing so can lead to incomplete or incorrect results.
- Misapplying Formulas: Double-check that you’re using the correct formula for the problem at hand. Applying the wrong one can lead to confusion and mistakes in your calculations.
- Neglecting Units: Always keep track of units, whether it’s length, volume, or time. Omitting or confusing units can lead to significant errors in your answer.
- Skipping Checkpoints: Skipping intermediate steps can lead to mistakes. Verify each stage of your solution to ensure accuracy before moving on.
Tips to Prevent These Errors
To minimize these common mistakes, try the following tips:
- Practice Time Management: Ensure you allocate enough time for each question, but avoid rushing through them. Pace yourself to maintain focus and accuracy.
- Review Your Work: After solving a problem, take a moment to go over your solution. Checking your work can catch small errors before they become big problems.
- Stay Organized: Write out every step clearly and logically. This will help prevent errors and make it easier to spot mistakes.
By staying mindful of these common pitfalls, you’ll be better prepared to perform at your best.
Effective Time Management Strategies
Time management is a crucial skill when preparing for a significant assessment. Properly allocating your time can help you cover all necessary topics, avoid last-minute stress, and ensure that you have enough time to review your work. With effective planning and strategic time allocation, you can enhance your performance and reduce anxiety during the process.
Here are some key strategies to help you manage your time efficiently:
- Create a Study Schedule: Plan your study sessions ahead of time. Break down the material into manageable sections and assign a specific amount of time to each one. Be realistic about what you can cover within each session.
- Prioritize Difficult Topics: Focus on areas where you feel least confident. Tackling these topics first, when you are most alert, will make them feel less overwhelming later on.
- Set Time Limits: Allocate a fixed amount of time for each question or task during practice sessions. This will help you get used to working within time constraints and improve your speed during the actual assessment.
- Take Breaks: Studying for long periods without breaks can lead to burnout. Schedule short breaks every 25-30 minutes to refresh your mind and maintain focus.
- Review Regularly: Set aside time each week for review. Consistent revision helps reinforce concepts and reduces the amount of cramming needed closer to the assessment.
By following these time management strategies, you can improve your efficiency and enter the assessment with a clear, focused mind, prepared to tackle the challenge with confidence.
How to Practice Math Problems
Effective practice is the key to mastering any subject, and working through problems is one of the most efficient ways to reinforce your understanding. Regular practice not only helps solidify concepts but also improves problem-solving speed and accuracy. This section outlines how to practice effectively and maximize your study sessions.
Start with the Basics
Begin by revisiting fundamental concepts. Understanding the basics is crucial before tackling more complex problems. Here’s how to approach your practice:
- Review Core Formulas: Make sure you’re comfortable with essential formulas and theorems. Practice applying them in different scenarios to increase familiarity.
- Solve Simple Problems: Start with easier problems to build confidence. As you improve, gradually increase the difficulty level to challenge yourself.
- Understand the Steps: Don’t just memorize solutions. Focus on understanding the steps involved in solving a problem, as this will help you apply the same methods to different types of questions.
Increase Problem Variety
To gain proficiency, it’s important to expose yourself to a wide range of problems. This will help you recognize patterns and better understand how different concepts interrelate.
- Use Practice Sets: Look for problem sets that cover a variety of topics. This will ensure you’re not just repeating the same type of problem but encountering new challenges.
- Simulate Real Conditions: Time yourself while solving practice problems to simulate the actual assessment environment. This can help improve your time management and reduce test-day anxiety.
- Analyze Mistakes: After completing a problem, review any mistakes and understand where you went wrong. This will help prevent similar errors in the future.
By practicing consistently and strategically, you will improve your ability to solve problems quickly and accurately, making you well-prepared for any challenge ahead.
Reviewing Functions and Equations
Understanding the relationship between variables is essential for solving many problems. Functions and equations are fundamental concepts that describe how different quantities interact with each other. A solid grasp of these ideas allows you to solve a wide range of problems efficiently. This section will help you review key principles related to functions and equations, providing you with the tools you need to tackle them confidently.
When reviewing functions and equations, focus on the following key concepts:
- Functions and Their Graphs: A function represents a relationship where each input has a unique output. Understanding how to graph a function is crucial for visualizing these relationships. Pay attention to common function types, such as linear, quadratic, and exponential, and how they behave on a graph.
- Solving Linear Equations: Linear equations involve variables raised only to the first power. Practice solving these equations by isolating the variable, using techniques like addition, subtraction, multiplication, and division. Mastering these techniques will allow you to solve more complex problems.
- Systems of Equations: A system of equations involves multiple equations that are solved simultaneously. Learn how to solve these systems using substitution, elimination, and graphical methods. Practice solving for both variables in various scenarios.
By reviewing these concepts, you’ll reinforce your understanding and become more comfortable solving problems involving functions and equations. Consistent practice will help you recognize patterns and apply the right methods with ease.
How to Tackle Graphing Questions
Graphing questions test your ability to translate mathematical relationships into visual representations. Being able to graph correctly is essential for understanding how different variables interact with each other. Whether you’re plotting a simple line or a more complex curve, knowing the right approach will help you answer these questions accurately and efficiently.
Here are some tips for successfully tackling graphing questions:
- Understand the Equation: Before you start graphing, take time to analyze the equation you’re working with. Identify the type of function (linear, quadratic, etc.) and recognize key features, such as slopes, intercepts, and symmetry.
- Plot Key Points: For most functions, it’s helpful to calculate a few key points and plot them on the graph. For linear equations, start by plotting the y-intercept and using the slope to find additional points. For other functions, substitute values for x and solve for y to get coordinate pairs.
- Draw the Graph: Once you’ve plotted enough points, connect them smoothly. For linear equations, use a straight line, and for curves, make sure the shape matches the behavior of the function. Pay attention to any turning points or asymptotes that are relevant.
- Label Your Graph: Ensure that you label both the x-axis and y-axis clearly, indicating the scale and units where applicable. If the problem requires, label the key points you’ve plotted and any important features of the graph, such as intercepts or vertices.
By following these steps, you’ll be better equipped to handle any graphing question with confidence. Practice regularly to become familiar with different types of functions and their graphs, and soon the process will feel like second nature.
Best Study Resources for Success
Having access to the right resources can make a significant difference when preparing for a challenging assessment. The right tools help you grasp complex concepts, practice effectively, and gain confidence. This section highlights some of the best study resources that can support your learning and boost your chances of success.
Here are some valuable study resources to consider:
- Textbooks and Study Guides: A well-organized textbook or a detailed study guide provides a comprehensive overview of the material. Look for guides that explain key concepts, offer practice problems, and summarize important theories.
- Online Platforms: Websites and apps such as Khan Academy, Coursera, and YouTube offer free video tutorials on various topics. These resources provide step-by-step explanations and allow you to learn at your own pace, helping you reinforce difficult concepts.
- Practice Tests: Taking practice tests helps you become familiar with the format and time constraints. Many websites offer free or paid practice questions, allowing you to simulate the test environment and track your progress.
- Study Groups: Collaborating with classmates in study groups can enhance your learning experience. Group discussions allow you to share ideas, solve problems together, and explain concepts in a way that strengthens your own understanding.
- Tutoring Services: If you’re struggling with certain topics, consider seeking additional help from a tutor. Many schools and online platforms offer tutoring services that can provide personalized support tailored to your needs.
Using a combination of these resources will help you approach your studies from multiple angles, increasing both your understanding and retention of the material. Find what works best for you and make the most of these tools to maximize your preparation.
Preparing for Multiple Choice Questions
Multiple choice questions are a common assessment format that tests your ability to recall and apply concepts quickly. They often require you to recognize the correct answer from a list of possible choices, which means preparation must focus on accuracy, understanding, and time management. This section provides strategies to help you tackle multiple choice questions with confidence.
Here are some key strategies to prepare for these types of questions:
- Read Each Question Carefully: The first step in answering multiple choice questions is to fully understand the question being asked. Pay close attention to keywords and phrases that might indicate the right answer, and avoid rushing through the question.
- Eliminate Incorrect Options: Often, you can rule out one or more answer choices right away. By eliminating the clearly incorrect options, you increase your chances of selecting the correct one. This method is especially helpful when you’re unsure about the answer.
- Focus on Key Concepts: Review the main ideas and important principles from the material. Multiple choice questions are designed to test your understanding of key concepts, so reinforcing these areas will make it easier to spot the correct answers.
- Practice Similar Questions: Familiarize yourself with the format and types of questions by practicing with past papers or sample tests. The more you practice, the better you’ll be at recognizing question patterns and narrowing down answer choices.
- Don’t Overthink: If you’re unsure of an answer, avoid second-guessing yourself too much. Trust your first instinct unless you find a clear reason to change your answer. Overthinking can lead to unnecessary mistakes.
With consistent practice and careful strategy, you’ll improve your ability to approach multiple choice questions effectively. These techniques will help you maximize your performance and tackle questions with confidence on test day.
Reviewing Key Theorems and Formulas
Mastering the essential theorems and formulas is crucial for solving problems efficiently. These foundational concepts act as the building blocks for various mathematical topics and help simplify complex questions. In this section, we will highlight some of the most important principles you should focus on when preparing for a challenging assessment.
Here are some of the key theorems and formulas to review:
- Pythagorean Theorem: This fundamental theorem relates the sides of a right triangle. It states that the square of the hypotenuse is equal to the sum of the squares of the other two sides: a² + b² = c².
- Quadratic Formula: To solve quadratic equations, use the quadratic formula: x = (-b ± √(b² – 4ac)) / 2a. This formula gives the solutions for any quadratic equation of the form ax² + bx + c = 0.
- Area and Perimeter Formulas: Make sure to memorize formulas for calculating the area and perimeter of various shapes, including circles, triangles, and rectangles. For example, the area of a circle is πr², and the perimeter of a rectangle is 2(l + w).
- Slope Formula: The slope of a line is determined by the formula m = (y₂ – y₁) / (x₂ – x₁), where m is the slope and (x₁, y₁) and (x₂, y₂) are the coordinates of two points on the line.
- Exponent Rules: Understanding how to apply exponent rules is vital for simplifying expressions. Key rules include: a^m × a^n = a^(m+n), (a^m)^n = a^(m×n), and a^0 = 1.
To solidify your understanding, practice applying these theorems and formulas to a variety of problems. Reviewing and memorizing them will allow you to approach problems with confidence and speed during the test.
How to Stay Calm During the Test
Test anxiety can be a challenge, but maintaining composure is key to performing well. Staying calm during a challenging assessment requires a combination of mental preparation, breathing techniques, and a focused mindset. In this section, we will explore some strategies to help you stay composed and confident as you work through the questions.
Practice Relaxation Techniques
One of the most effective ways to stay calm is by using relaxation exercises before and during the test. Simple breathing techniques, such as deep breathing, can help reduce tension and anxiety. Try taking a few slow, deep breaths to center yourself if you start to feel overwhelmed. Inhale for a count of four, hold for a count of four, then exhale for a count of four. Repeat as necessary.
Focus on the Present Moment
Rather than thinking about the entire test, focus on each question individually. Break the test down into smaller tasks to make it feel more manageable. Avoid dwelling on questions that seem difficult at first. If you get stuck, move on to the next one and come back later. Trust in your preparation and know that you have the skills needed to tackle each problem as it comes.
Additionally, remember that you are allowed to take breaks if needed. A brief moment of rest can help refresh your mind and keep you focused for the remaining portion of the assessment.
Test-Taking Strategies for Math
Approaching an assessment that involves problem-solving and logical thinking requires more than just understanding the content. Effective strategies can help you navigate through the questions with confidence and efficiency. By using smart techniques, you can maximize your performance and minimize errors. In this section, we’ll explore some key test-taking strategies to enhance your chances of success.
Read the Instructions Carefully
Before diving into the questions, take the time to read through the instructions thoroughly. Understanding the specific requirements for each question will prevent mistakes and ensure you’re following the correct approach. Pay attention to important details, such as whether you need to show your work or if multiple answers are required.
Start with the Easiest Questions
Begin by tackling the questions that seem easiest to you. This will boost your confidence and help you gain momentum as you move forward. When you’re confident in your answers, it also leaves more time to deal with more difficult problems later. Don’t waste too much time on questions that you’re unsure about–leave them for later and come back if time permits.
Eliminate Clearly Wrong Answers
If the assessment involves multiple-choice questions, use the process of elimination to narrow down your options. Even if you’re unsure about the correct answer, eliminating clearly incorrect choices increases the likelihood of choosing the right one. By focusing on plausible answers, you can make a more educated guess.
Show Your Work
Even if the problem seems straightforward, showing your steps can help prevent small errors and allow you to track your reasoning. In many cases, partial credit may be awarded for the steps you take, so demonstrating your understanding can make a difference in the final score. Ensure that each step is clear and logical.
Review Your Answers
Once you’ve completed all the questions, if time allows, go back and review your answers. Check for simple mistakes, such as calculation errors or misread questions. Sometimes a second look can reveal details that were missed the first time. Don’t rush through the review–carefully reconsider each problem before submitting your work.
How to Improve Your Math Skills
Developing strong problem-solving abilities and logical thinking takes time and consistent practice. Whether you struggle with complex calculations or need to strengthen your understanding of concepts, there are effective strategies to enhance your capabilities. By focusing on the right areas, engaging in targeted practice, and staying disciplined, you can see noticeable improvement in your skills.
1. Practice Regularly
One of the most effective ways to improve is through consistent practice. Set aside dedicated time each day to solve problems and review concepts. The more problems you work through, the more familiar you become with different types of questions and techniques. Regular practice also helps to identify areas that need more attention.
2. Understand, Don’t Memorize
While memorization can help with some formulas, true understanding is key to solving problems efficiently. Focus on understanding the “why” behind each method and concept. Once you understand the underlying principles, applying them to various problems becomes easier and more intuitive.
3. Use Visual Aids
Sometimes, seeing the problem from a different perspective can make a world of difference. Using diagrams, charts, and graphs can help simplify complex problems and provide a visual understanding of abstract concepts. These tools are especially helpful when dealing with spatial problems or abstract relationships between variables.
4. Work on Your Weak Areas
Identify the areas where you struggle the most and devote extra time to practicing those topics. Whether it’s geometry, algebra, or problem-solving strategies, focusing on your weak points will help you build confidence and improve your overall performance.
5. Seek Additional Resources
Sometimes, the materials provided in class may not be enough. Seek out additional resources such as online tutorials, study guides, and practice tests. Online platforms often offer interactive problem sets, step-by-step explanations, and forums where you can ask questions and discuss with others.
6. Review Mistakes and Learn from Them
When reviewing your work, pay close attention to mistakes. Understand why an answer was incorrect and what steps led to that mistake. By learning from errors, you prevent them from happening again and strengthen your problem-solving skills.
| Strategy | Benefit |
|---|---|
| Regular Practice | Improves familiarity and fluency with problem-solving |
| Understanding Concepts | Promotes deeper comprehension and quicker application |
| Visual Aids | Helps in grasping abstract concepts and simplifying complex ideas |
| Focused Review | Strengthens weak areas and boosts overall confidence |
By integrating these strategies into your study routine, you’ll gradually notice significant progress in your ability to solve problems and understand complex topics. Be patient and consistent with your efforts, and improvement will follow.
Planning Your Test Day Routine
Preparing for a big assessment involves more than just reviewing material; managing your day effectively can significantly impact your performance. The key to staying calm and focused on the day of the test is to have a well-structured routine that allows you to feel confident and ready. From the moment you wake up until you finish the test, each step plays a vital role in helping you perform at your best.
1. Get a Good Night’s Sleep
Rest is essential for mental clarity and concentration. Try to get a full night of sleep before the test day. Avoid staying up late cramming, as this can make you more tired and less able to focus during the assessment.
2. Eat a Healthy Breakfast
A nutritious breakfast fuels your body and mind. Include a combination of protein, fiber, and healthy fats to keep your energy levels steady throughout the test. Avoid sugary foods or heavy meals that may lead to a crash in energy.
3. Gather Your Materials
Ensure that you have everything you need before the test begins. Double-check that you have your writing tools, any required identification, and any allowed materials such as calculators or notes. Being organized will help reduce any last-minute stress.
4. Arrive Early
Arriving ahead of time allows you to settle in and get comfortable. You’ll avoid the stress of rushing, and you can use any extra time to mentally prepare and relax before the test starts.
5. Take a Few Deep Breaths
Just before the test begins, take a moment to relax. Deep breathing can calm your nerves and help you focus. If you feel overwhelmed during the test, pause and take a few deep breaths to regain your concentration.
6. Review Your Strategy
Before diving into the questions, take a moment to look over the entire test. If possible, start with the sections or questions you feel most confident about. This will help you build momentum and avoid wasting time on difficult questions early on.
7. Stay Positive
Keep a positive attitude throughout the day. Stay focused on doing your best, and remember that one test doesn’t define your abilities. If you encounter difficult questions, take a deep breath and move forward; you can always come back to them later.
- Sleep well: Rest helps your brain perform at its best.
- Eat wisely: Choose foods that sustain your energy.
- Be organized: Gather all necessary materials in advance.
- Arrive early: Give yourself time to adjust and prepare.
- Stay calm: Relax with deep breaths when you feel anxious.
- Have a strategy: Tackle easier questions first to build confidence.
- Keep a positive mindset: Focus on your strengths and abilities.
By following these steps, you’ll feel prepared, confident, and ready to perform at your best. Planning your routine in advance ensures you enter the testing environment with a clear mind and a sense of calm.