
As you approach the end of your coursework, it’s crucial to consolidate your understanding of the material covered throughout the term. This section provides a comprehensive collection of problems and solutions designed to test your grasp of key mathematical concepts. Whether you’re focusing on solving equations, working with functions, or applying advanced techniques, this guide is here to support you in refining your skills.
Success in mathematics relies not just on memorizing formulas but also on practicing problem-solving strategies. By engaging with a variety of challenges, you’ll gain the confidence needed to tackle any question that may arise. Each solution is designed to help clarify concepts and offer a deeper insight into the problem-solving process.
As you progress through this material, take your time to understand the reasoning behind each solution rather than simply memorizing the steps. This approach will help you think critically and respond effectively in any situation that requires mathematical reasoning.
Final Preparation for Mathematics Assessment
In the final stages of your studies, it’s essential to solidify your knowledge and ensure you’re ready to demonstrate your proficiency in solving complex mathematical problems. This phase focuses on reinforcing the most important concepts and methodologies that you’ve encountered throughout your coursework. By reviewing key topics and practicing a range of problems, you’ll be well-equipped to handle a variety of questions that may appear in the assessment.
Strengthening Core Concepts
Familiarize yourself with the foundational topics you’ve studied, such as equations, functions, and graph analysis. These areas often form the basis of more advanced questions, so it’s crucial to fully understand their principles. Revisiting these topics will also help identify any areas where you may need further clarification or practice.
Developing Effective Problem-Solving Strategies
Mathematics requires both skill and strategy. Focus on honing your approach to solving different types of problems. Practice breaking down complex questions into manageable steps and applying appropriate methods for each case. The more problems you solve, the more confident you’ll become in tackling any challenge that arises.
Key Concepts to Review for Algebra 2
To ensure a comprehensive understanding of the material, it’s crucial to revisit the most important topics that have been covered in your coursework. Mastering these core concepts will help you approach problems with confidence and accuracy. Below are the fundamental areas you should focus on as you prepare for the upcoming assessment:
- Solving equations and inequalities
- Graphing and analyzing functions
- Working with exponential and logarithmic expressions
- Factoring polynomials and quadratic expressions
- Understanding rational expressions and their applications
- Solving systems of equations and inequalities
- Manipulating radical and rational functions
By reviewing and practicing these key areas, you will be better prepared to approach a variety of problems with the necessary tools and techniques. Be sure to not only memorize formulas but also understand the underlying principles behind each method.
Understanding the Algebra 2 Assessment Format
Before stepping into the assessment, it’s essential to familiarize yourself with its structure. Knowing what to expect in terms of question types and layout will help you approach the test with greater confidence and efficiency. In this section, we’ll break down the typical format, so you know exactly what areas to focus on during your preparation.
The assessment is usually divided into multiple sections, each targeting different skills. You may encounter multiple-choice questions, short answer problems, and problem-solving tasks. Each type is designed to test your understanding of key concepts and your ability to apply mathematical techniques in various contexts.
It’s important to practice timed conditions to manage your time effectively during the test. Knowing how long you should spend on each section will prevent you from rushing or spending too much time on any single question.
Important Formulas for Algebra 2 Assessments
Having a solid grasp of the essential formulas is key to performing well in mathematics. These formulas serve as the foundation for solving a wide range of problems, from solving equations to analyzing functions. In this section, we will review the most important formulas you should remember as you prepare for your upcoming test.
Key Formulas to Know

| Formula | Description |
|---|---|
| Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a | Used to find the solutions of quadratic equations. |
| Standard Form of a Line: y = mx + b | Represents the equation of a straight line, where m is the slope and b is the y-intercept. |
| Exponent Rule: a^m × a^n = a^(m+n) | Allows you to simplify expressions with the same base. |
| Factorization Formula: (a + b)(a – b) = a² – b² | Used for expanding binomial expressions. |
Additional Key Concepts
In addition to the fundamental formulas, it’s important to understand their applications and how they relate to one another. Practice using these formulas in various scenarios to become more comfortable and efficient in applying them during the test.
How to Use Your Study Materials Effectively
To maximize your preparation and enhance your understanding of the material, it’s crucial to approach your study resources strategically. Instead of simply going through each question, focus on how you can deepen your grasp of each concept. The goal is not just to find the solutions but to understand the steps that lead to those solutions. By doing so, you’ll be better equipped to apply your knowledge under pressure.
Start with a Strong Foundation
Begin by reviewing the most important topics that you find challenging. Break down each section and try to identify the key concepts, formulas, and methods that are essential for solving the problems. Don’t rush through the material–take your time to ensure that you truly understand each part before moving on to the next.
Practice with Purpose
After working through the problems, focus on analyzing your mistakes. If a question feels difficult, revisit the related material to reinforce your understanding. Use the solutions to guide your thought process, but avoid memorizing answers without fully grasping the reasoning behind them. This method will help you think critically and approach similar problems with more confidence.
Common Algebra 2 Mistakes to Avoid
While studying complex mathematical concepts, it’s easy to overlook certain details that can lead to errors. These mistakes, if left unchecked, can undermine your confidence and hinder your progress. Recognizing these common pitfalls in advance can help you stay focused and reduce the likelihood of making the same mistakes repeatedly.
Misunderstanding the Order of Operations
One of the most frequent mistakes is neglecting the proper order of operations when solving problems. Always remember to follow the correct sequence–parentheses, exponents, multiplication/division, and addition/subtraction (PEMDAS). Skipping this crucial step can lead to incorrect answers, especially in more complex expressions.
Incorrectly Applying Formulas
Another common mistake is incorrectly using formulas or forgetting to adapt them to specific situations. For example, when applying the quadratic formula, be sure to check that you correctly identify the coefficients and signs. Even small mistakes in setting up formulas can lead to completely incorrect results.
Double-checking your work and understanding the underlying principles behind each formula is essential to avoid these types of errors. Taking the time to carefully review each step can save you from many avoidable mistakes.
Strategies for Solving Algebra 2 Word Problems
Word problems can often seem overwhelming, but with the right approach, they can become manageable. The key to solving these types of problems is breaking them down into smaller, more understandable steps. By identifying the variables, interpreting the information, and applying appropriate methods, you can successfully tackle any problem that comes your way.
Steps to Approach Word Problems
- Read the problem carefully: Make sure you fully understand the situation before jumping into calculations. Take note of all the given information and what is being asked.
- Identify variables: Assign variables to unknown quantities, such as x or y, to represent the values you’re trying to solve for.
- Translate into an equation: Convert the word problem into a mathematical expression or equation using the information you’ve gathered.
- Choose the right method: Depending on the problem, decide whether to use systems of equations, quadratic formulas, or other techniques that apply to the situation.
- Check your work: Once you have a solution, revisit the problem to ensure it makes sense in the context of the question. Check for errors and verify the results.
Additional Tips for Success
- Practice regularly: The more you practice, the more familiar you will become with the types of word problems you’ll encounter.
- Visualize the problem: If possible, draw a diagram or graph to represent the situation, making it easier to interpret the data and see relationships between the variables.
- Break complex problems into parts: If the problem is multi-step, break it into smaller sections and solve each part one by one.
With consistent practice and a strategic approach, solving word problems will become more intuitive, helping you improve your problem-solving skills and perform better in assessments.
Reviewing Functions and Graphs
Understanding functions and their graphical representations is crucial for solving a wide range of mathematical problems. Functions are a way to model relationships between variables, and graphs provide a visual way to interpret these relationships. In this section, we’ll revisit key concepts related to functions and their graphs to ensure you are well-prepared for any related questions.
Types of Functions to Focus On
Several types of functions are commonly encountered in math problems, each with unique characteristics. Make sure you understand the general form and behavior of the following:
- Linear functions: These functions create straight lines when graphed, and their equation is typically written as y = mx + b, where m is the slope and b is the y-intercept.
- Quadratic functions: Represented by equations like y = ax² + bx + c, these functions produce parabolic graphs, opening either upward or downward depending on the value of a.
- Exponential functions: These functions have the form y = ab^x, where a is a constant and b is the base. Their graphs show rapid growth or decay.
- Rational functions: These functions are ratios of polynomials and their graphs may include asymptotes and discontinuities.
Graphing Techniques and Tips
When working with graphs, there are several key points to keep in mind:
- Identify key features: For each function, recognize important features such as intercepts, slope, asymptotes, and vertex points.
- Plot points carefully: Use the function’s equation to calculate points that you can plot on the graph, paying attention to symmetry and transformations.
- Understand transformations: Be familiar with how changes to the equation affect the graph, such as shifting, stretching, or reflecting the curve.
Mastering these concepts will not only help you understand the material more deeply but also enable you to apply these techniques to solve problems involving functions and graphs with ease.
Mastering Quadratic Equations in Algebra 2
Quadratic equations are a fundamental concept that appear frequently in advanced mathematics. They represent relationships that involve squared terms and often model real-world situations, such as projectile motion or profit and loss. Mastering the techniques for solving quadratic equations will greatly enhance your problem-solving skills and provide a strong foundation for more complex topics.
Methods for Solving Quadratic Equations
There are several approaches to solving quadratic equations, each suited to different types of problems. Understanding when and how to apply these methods is essential for efficiently finding solutions.
- Factoring: When possible, factoring is one of the quickest methods. If the equation can be written as a product of two binomials, you can solve for the variables by setting each factor equal to zero.
- Completing the Square: This method involves rearranging the equation so that the left-hand side becomes a perfect square trinomial. It is particularly useful when the equation is not easily factorable.
- Quadratic Formula: The quadratic formula, x = (-b ± √(b² – 4ac)) / 2a, provides a general solution to any quadratic equation, making it a reliable fallback when other methods are not applicable.
Key Points to Remember
When working with quadratic equations, keep these important points in mind:
- Discriminant: The value under the square root in the quadratic formula, b² – 4ac, is called the discriminant. It determines the nature of the roots: if it is positive, there are two real roots; if zero, there is one real root; if negative, the roots are complex.
- Vertex Form: Quadratic equations can also be expressed in vertex form y = a(x – h)² + k, where (h, k) represents the vertex of the parabola. This form is useful for graphing and analyzing the equation’s properties.
Example of Solving a Quadratic Equation
Here’s an example of solving a quadratic equation using the quadratic formula:
| Equation | x² – 4x – 5 = 0 |
|---|---|
| Quadratic Formula | x = (-(-4) ± √((-4)² – 4(1)(-5))) / (2(1)) |
| Simplified | x = (4 ± √(16 + 20)) / 2 |
| Solution | x = (4 ± √36) / 2 |
| Final Answer | x = (4 ± 6) / 2 |
| Possible Solutions | x = 5 or x = -1 |
By practicing these methods and focusing on the underlying principles, you’ll develop a strong understanding of quadratic equations and be able to solve them efficiently in any context.
Exploring Exponents and Polynomials
Understanding the behavior of exponents and polynomials is essential for mastering higher-level mathematical concepts. These two areas are closely related, with exponents influencing the growth and properties of polynomials. By gaining a deeper understanding of the rules and techniques for manipulating exponents and polynomials, you can tackle more complex problems with ease.
Rules of Exponents
Exponents are used to represent repeated multiplication of a number by itself. Mastery of the rules for manipulating exponents is critical for simplifying expressions and solving problems efficiently. Here are some key rules:
- Product Rule: a^m × a^n = a^(m + n) – When multiplying terms with the same base, add the exponents.
- Quotient Rule: a^m / a^n = a^(m – n) – When dividing terms with the same base, subtract the exponents.
- Power of a Power Rule: (a^m)^n = a^(m × n) – When raising a power to another power, multiply the exponents.
- Zero Exponent Rule: a^0 = 1 – Any non-zero number raised to the power of zero equals 1.
- Negative Exponent Rule: a^(-n) = 1/a^n – A negative exponent indicates the reciprocal of the base raised to the positive exponent.
Understanding Polynomials
Polynomials are algebraic expressions consisting of terms, each involving a variable raised to a power. The highest power of the variable is called the degree of the polynomial. Working with polynomials involves simplifying, adding, subtracting, multiplying, and factoring them. A solid grasp of polynomial operations is crucial for solving equations and modeling real-world situations.
- Adding and Subtracting Polynomials: Combine like terms, which are terms with the same variable raised to the same power.
- Multiplying Polynomials: Use the distributive property (also known as the FOIL method for binomials) to expand the product of two polynomials.
- Factoring Polynomials: Factoring involves finding the expressions that multiply to give the original polynomial. Common methods include factoring out the greatest common factor (GCF) and using special formulas such as the difference of squares.
Example of Simplifying an Expression
Let’s simplify the following expression:
Expression: 3x² × 2x³
Solution: Using the product rule for exponents, we add the exponents of the same base:
3x² × 2x³ = 6x^(2 + 3) = 6x⁵
This example illustrates how exponents and basic multiplication can simplify expressions quickly. By practicing such problems, you’ll strengthen your ability to handle more complex polynomial equations and operations.
Working with Rational Expressions
Rational expressions involve fractions where the numerator and the denominator are both polynomials. Understanding how to manipulate these expressions is essential for solving a wide range of mathematical problems. The primary operations with rational expressions include simplifying, multiplying, dividing, adding, and subtracting them. Mastery of these operations allows you to handle more complex equations and mathematical modeling tasks with ease.
Simplifying Rational Expressions
To simplify a rational expression, you first need to factor both the numerator and the denominator. After factoring, you can cancel out any common factors between the numerator and denominator. This process reduces the expression to its simplest form. Here’s a general step-by-step approach:
- Factor both the numerator and denominator.
- Cancel out any common factors.
- Rewrite the expression using the remaining factors.
For example:
Expression: (2x² + 6x) / (4x)
Factoring: Factor the numerator and denominator:
(2x(x + 3)) / (4x)
Simplifying: Cancel the common factor of 2x:
(x + 3) / 2
This is the simplified form of the expression.
Operations with Rational Expressions
In addition to simplifying, rational expressions often require performing basic operations such as addition, subtraction, multiplication, and division. Each operation requires a specific method to ensure the correct result. Below is a brief explanation of these operations:
Multiplying Rational Expressions
Multiplying rational expressions involves multiplying the numerators together and the denominators together, then simplifying if necessary.
Example: Multiply (3x / 4) by (8 / x²):
Solution: Multiply the numerators and denominators:
(3x * 8) / (4 * x²) = 24x / 4x²
Simplify:
24x / 4x² = 6 / x
Dividing Rational Expressions
Dividing rational expressions requires multiplying by the reciprocal of the divisor. Here’s how to approach it:
Example: Divide (2x / 3) by (4x² / 5):
Solution: Multiply (2x / 3) by the reciprocal of (4x² / 5):
(2x / 3) * (5 / 4x²) = (10x) / (12x²)
Simplify:
10x / 12x² = 5 / 6x
Common Mistakes to Avoid
When working with rational expressions, it’s important to watch out for common mistakes:
- Forgetting to factor the numerator and denominator.
- Incorrectly canceling terms that are not factors.
- Failing to check for restrictions, such as values that make the denominator equal to zero.
Conclusion
Rational expressions are a powerful tool in solving mathematical problems. By mastering the rules for simplifying and performing operations with them, you can tackle more advanced topics and challenges in mathematics with confidence.
Factoring Techniques You Should Know
Factoring is a crucial skill for simplifying and solving various types of equations. Being able to factor expressions efficiently is key to tackling more complex problems. Whether you’re simplifying polynomials or solving quadratic equations, mastering factoring methods can make a significant difference in your success. Below, we’ll cover several essential factoring techniques that you should be familiar with to handle a variety of mathematical tasks.
The most common methods include factoring by grouping, using the difference of squares, and applying the quadratic formula. Each of these techniques has its specific applications and can be used in combination to solve more complicated problems. Understanding when and how to use each technique is essential for achieving accurate results in algebraic expressions.
Factoring by Grouping
Factoring by grouping is a method that can be applied when you have four terms in a polynomial. The idea is to group the terms in pairs, factor out the greatest common factor (GCF) from each group, and then factor out the common binomial factor. Here’s how you can apply this technique:
- Group the terms in pairs.
- Factor out the GCF from each pair.
- Factor out the common binomial factor.
For example, for the expression x² + 5x + 2x + 10, you would group it as (x² + 5x) and (2x + 10), then factor each group:
(x(x + 5)) + 2(x + 5)
Now, factor out the common binomial factor:
(x + 5)(x + 2)
This is the factored form of the original expression.
Difference of Squares
The difference of squares is a special case of factoring that applies when you have two terms that are both perfect squares separated by a subtraction sign. The general formula for factoring the difference of squares is:
a² – b² = (a – b)(a + b)
For example, if you have the expression 9x² – 25, notice that both 9x² and 25 are perfect squares. You can apply the difference of squares formula:
9x² – 25 = (3x – 5)(3x + 5)
By recognizing the pattern of perfect squares and applying the formula, you can quickly factor these types of expressions.
Factoring Quadratics
Factoring quadratic expressions is one of the most important skills in algebra. The general form of a quadratic expression is ax² + bx + c, and factoring it involves finding two binomials that multiply together to give the original expression. One of the most common methods for factoring quadratics is to use the AC method, where you multiply the first and last coefficients, then find two numbers that multiply to this product and add to the middle coefficient.
For example, for the quadratic expression x² + 7x + 10, you would look for two numbers that multiply to 10 and add to 7. These numbers are 5 and 2, so you can factor the expression as:
(x + 5)(x + 2)
This method works well for most quadratics where the leading coefficient is 1. For quadratics with higher coefficients, other techniques may be required, such as splitting the middle term or using the quadratic formula.
Conclusion
Mastering factoring techniques is essential for solving a wide range of algebraic problems. By learning how to factor by grouping, recognize the difference of squares, and apply methods like the AC method for quadratics, you’ll be well-prepared for handling any factoring challenges you encounter. With practice, these techniques will become second nature, allowing you to solve equations quickly and accurately.
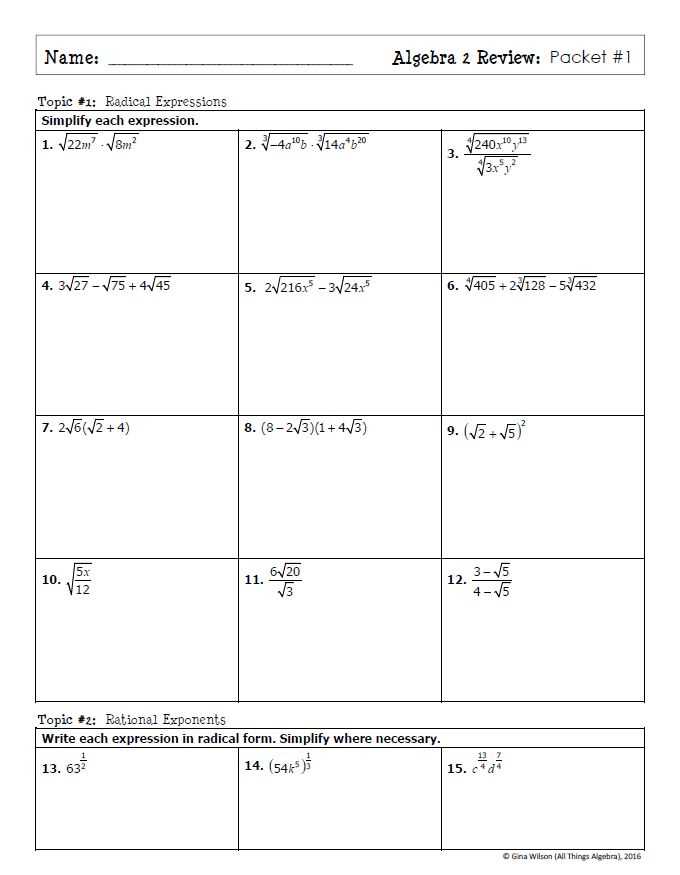
Tips for Tackling Radical Expressions
When working with expressions that involve roots, it’s important to understand the basic rules and techniques to simplify and solve them efficiently. Radical expressions can appear complex, but with the right approach, they become much more manageable. This section covers key strategies for handling square roots, cube roots, and other radical expressions effectively.
One of the first steps when simplifying radical expressions is to ensure all the radicals are in their simplest form. This may involve factoring numbers under the radical sign, simplifying square roots, and eliminating any negative numbers inside the radical. Additionally, when performing operations with radicals, such as addition or multiplication, it’s important to ensure that the expressions are like terms or have the same index for proper simplification.
Simplifying Square Roots
Simplifying square roots involves breaking down the number under the radical into its prime factors. When you find a pair of prime factors, you can move one number from under the square root sign to the outside. Here’s a general process:
- Factor the number under the radical into prime factors.
- Pair the prime factors (for square roots, look for pairs of identical factors).
- Move the paired factors outside of the radical.
- If there are any remaining factors under the radical, leave them inside.
For example, to simplify the square root of 72, you first factor it as:
72 = 2 × 2 × 2 × 3 × 3
Next, pair the factors (2 × 2) and (3 × 3), and move them outside the radical:
√72 = 2 × 3√2 = 6√2
Rationalizing Denominators
Another important technique when working with radical expressions is rationalizing the denominator. This involves eliminating radicals from the denominator of a fraction. You can do this by multiplying both the numerator and the denominator by a suitable radical expression that will allow you to simplify the denominator. For example, if you have:
1 / √3
Multiply both the numerator and denominator by √3:
1 / √3 × √3 / √3 = √3 / 3
This process ensures that the denominator no longer contains a radical, making the expression simpler and more standard.
Operations with Radical Expressions
When performing operations with radical expressions, such as addition or multiplication, it’s essential to combine like terms. Like radicals can only be added or subtracted if they have the same index and the same radicand (the number under the radical). For example, you can combine 3√2 and 5√2, but you cannot combine 3√2 with 4√3 because the radicands are different.
In terms of multiplication, when you multiply two radicals with the same index, you multiply the numbers under the radical sign and simplify the result:
√2 × √3 = √(2 × 3) = √6
| Expression | Simplified Form |
|---|---|
| √8 × √2 | √(8 × 2) = √16 = 4 |
| √5 + √5 | 2√5 |
| √12 × √3 | √(12 × 3) = √36 = 6 |
Conclusion
By mastering these techniques, such as simplifying square roots, rationalizing denominators, and performing operations with radical expressions, you’ll be able to approach even the most challenging problems with confidence. With practice, simplifying and manipulating radical expressions will become a straightforward task, improving your ability to solve complex mathematical problems efficiently.
Linear Systems and Their Solutions
A system of linear equations consists of two or more equations that share common variables. The goal when solving such systems is to find the values of these variables that satisfy all the equations simultaneously. There are several methods available for solving linear systems, each suited to different types of problems. Understanding how to approach these systems efficiently is crucial for solving a variety of mathematical and real-world problems.
There are three main types of solutions that a system of linear equations can have: one solution, no solution, or infinitely many solutions. A system with a unique solution has a single point of intersection, where the values of the variables satisfy all the equations. A system with no solution occurs when the lines are parallel and never meet. If the system has infinitely many solutions, the equations represent the same line or overlapping lines, and any point on the line will satisfy all equations in the system.
To solve a system of linear equations, you can use different techniques such as substitution, elimination, or graphical methods. Each method has its advantages, depending on the complexity of the system and the form of the equations.
Substitution Method
The substitution method involves solving one equation for one variable and then substituting that expression into the other equation(s). This method is often used when one of the equations is easy to manipulate. Here’s how it works:
- Choose one of the equations and solve it for one variable (usually the easiest one).
- Substitute this expression into the other equation.
- Solve the resulting equation for the remaining variable.
- Substitute the value of the second variable back into the first equation to find the first variable.
For example, given the system:
y = 2x + 3
3x + y = 12
Substitute y = 2x + 3 into the second equation:
3x + (2x + 3) = 12
Simplify and solve for x:
3x + 2x + 3 = 12
5x + 3 = 12
5x = 9
x = 9/5
Now substitute x = 9/5 into the first equation to find y:
y = 2(9/5) + 3 = 18/5 + 15/5 = 33/5
The solution is: x = 9/5, y = 33/5.
Elimination Method
The elimination method works by adding or subtracting the equations to eliminate one variable, making it easier to solve for the other variable. Here’s the basic process:
- Multiply or divide one or both equations to align the coefficients of one variable.
- Add or subtract the equations to eliminate one variable.
- Solve the resulting equation for the remaining variable.
- Substitute the value of that variable into one of the original equations to find the other variable.
For example, given the system:
2x + 3y = 12
4x – 3y = 6
Add the two equations to eliminate y:
(2x + 3y) + (4x – 3y) = 12 + 6
6x = 18
x = 3
Substitute x = 3 into the first equation to find y:
2(3) + 3y = 12
6 + 3y = 12
3y = 6
y = 2
The solution is: x = 3, y = 2.
Graphical Method
The graphical method involves plotting each equation on a coordinate plane and identifying the point where the lines intersect. The coordinates of this point represent the solution to the system. While this method is visual and useful for understanding the relationship between equations, it may not always provide precise results unless done accurately with graphing tools or software.
For example, if you graph the equations:
y = 2x + 1
y = -x + 4
The point where the two lines intersect is the solution to the system. In this case, the solution is approximately (1, 3).
By practicing these methods and understanding when to apply each one, you can solve any system of linear equations effectively and efficiently.
Preparing for Algebra 2 Multiple Choice Questions
Multiple choice questions assess your understanding of key concepts and your ability to apply them efficiently. To perform well, it’s important to approach each question strategically, ensuring that you not only understand the material but can also interpret and select the correct option. A structured approach and practice can help you become more confident and accurate when answering these types of questions.
Here are some essential strategies to prepare for multiple choice questions effectively:
- Understand the Core Concepts: Focus on the main ideas and formulas that are frequently tested. Be sure to review key topics and their applications so that you can recognize how they fit into various question formats.
- Identify Key Words: Read each question carefully and look for keywords that indicate the specific operation or concept being tested, such as “simplify,” “solve,” or “factor.” This will help you focus your efforts and avoid misinterpretation.
- Process of Elimination: If you’re unsure of the answer,
How to Manage Your Exam Time
Effective time management is crucial when faced with a timed assessment. It ensures that you can thoughtfully answer each question, allocate your time wisely, and minimize stress. By planning ahead and developing strategies to stay on track, you can approach the test with confidence and increase your chances of success.
Here are some key strategies for managing your time effectively:
- Understand the Time Constraints: Begin by knowing how much time you have and how many questions need to be answered. This will help you allocate your time accordingly. For instance, if you have one hour and 40 questions, aim to spend around one minute on each question.
- Prioritize Easier Questions: Start with the questions that you feel most confident about. This will help you build momentum and ensure that you get the easier points first, leaving more time for difficult problems.
- Don’t Get Stuck: If you encounter a question that seems particularly challenging, move on and return to it later. Spending too much time on one problem can prevent you from completing the rest of the test.
- Use a Timer: Consider setting a timer for each section or each group of questions to stay on track. A timer can help remind you when it’s time to move on to the next part of the assessment.
How to Handle Different Sections
- Multiple Choice: These questions are generally quicker to answer. Don’t overthink the options, but be careful with each choice. If you’re unsure, eliminate the obvious wrong answers and make an educated guess.
- Problem Solving and Long-Form Questions: Allocate more time for these questions. After reading the problem, quickly outline a solution strategy. This approach will help you stay organized and focused while working through more complex problems.
In the final minutes, review your answers and make sure that you’ve completed every question. Double-check for any mistakes, especially those involving calculations or small details. Managing your time wisely will not only help you finish the test but also allow you to give each question the attention it deserves.
What to Do After Completing Your Review Packet
Once you’ve finished working through your practice material, the next step is to ensure you’ve fully understood the content and are prepared for the upcoming assessment. It’s not just about finishing the questions–it’s about reinforcing your knowledge and addressing any gaps that may remain.
Here are some effective steps to take after completing your preparation material:
- Review Your Mistakes: Carefully go over the problems you found challenging or answered incorrectly. Analyze your mistakes and identify where you went wrong. Understanding your errors will help you avoid repeating them during the actual test.
- Seek Clarification: If there are concepts you still don’t fully grasp, seek help. This could be from a teacher, tutor, or a study group. Don’t hesitate to ask questions about anything that seems unclear, as a solid understanding is crucial for tackling similar problems in the future.
- Revisit Difficult Topics: Focus on areas that you struggled with the most. Spend extra time reviewing these concepts, whether it’s through textbooks, online resources, or additional practice problems. Repetition will help reinforce your understanding.
- Practice Under Time Constraints: Try solving some problems again, but this time, simulate the time pressure you’ll experience during the actual test. This will help you work more efficiently and improve your time management skills.
- Take a Break: Once you’ve reviewed your mistakes and reinforced your weak points, give yourself some time to relax. A break can help reduce stress and allow you to come back with a clearer mind.
Final Preparations
- Make a Final Checklist: Before the assessment, create a checklist of essential formulas, concepts, and strategies. This will serve as a quick reference and help you stay organized.
- Stay Positive: A confident mindset can greatly impact your performance. Trust in the effort you’ve put into your preparation and approach the upcoming challenge with calm and focus.
By following these steps, you can ensure that your preparation is not only thorough but also effective, leaving you ready for success.
Using Practice Tests to Build Confidence
One of the most effective ways to boost your self-assurance before an important assessment is through consistent practice. Simulating real test conditions by completing practice exercises can help you become familiar with the types of questions you’ll face and the format you’ll encounter. This approach not only reinforces your understanding of the material but also allows you to gauge your progress, identify weak areas, and improve your time management skills.
Here are some key benefits of using practice exercises to strengthen your confidence:
- Familiarity with the Test Format: Practice tests mimic the structure of the actual assessment, allowing you to become comfortable with how questions are presented. Knowing what to expect reduces test anxiety and helps you stay focused during the real thing.
- Identifying Weak Areas: By working through practice problems, you can pinpoint areas where you may need more review. This targeted approach enables you to focus your efforts on the topics that require the most attention, ultimately boosting your performance.
- Improving Speed and Accuracy: Practicing under timed conditions can help you develop a sense of urgency and improve your ability to work efficiently. It also allows you to test your accuracy and adjust strategies for faster problem-solving.
- Building Test-Taking Strategies: Practice exercises allow you to experiment with different techniques, such as eliminating incorrect choices or identifying patterns in questions. These strategies can make a significant difference in how you approach the actual test.
By incorporating practice exercises into your preparation, you can build both your knowledge and confidence, ensuring you’re ready for the challenges ahead. The more you practice, the more you’ll trust in your ability to succeed, which is a key element for performing well in any test.