
In the fourth level of elementary learning, students are introduced to a variety of challenging problems that require critical thinking and problem-solving skills. This section focuses on offering solutions and explanations to help learners navigate these complexities with ease. Whether you’re a student seeking clarity or a teacher looking for effective methods, this guide provides valuable insights to strengthen understanding.
As students work through various exercises, they are encouraged to apply logical reasoning and reinforce their comprehension of important concepts. By reviewing solutions step by step, learners can grasp essential skills necessary for progressing in their studies. The goal is to provide an organized approach to mastering the topics covered in this learning phase.
Mastering the material not only builds confidence but also prepares students for more advanced topics in their educational journey. This guide simplifies difficult concepts and encourages a hands-on approach to problem-solving, making learning both enjoyable and rewarding.
Comprehensive Solutions for Level 4 Concepts
This section provides detailed solutions to the exercises encountered in the fourth level of elementary learning. By following a structured approach, students can better understand how to solve problems and apply techniques effectively. The guide covers important lessons, helping learners master concepts and navigate through challenging tasks with confidence.
Understanding the Process
Each exercise is broken down into manageable steps, ensuring students can follow along and grasp the necessary methods. The process is designed to help learners identify patterns, solve problems systematically, and reinforce their skills. By reviewing each step, students gain the ability to approach similar tasks in the future with greater ease.
Step-by-Step Solution Breakdown
The following table outlines solutions to key problems, showing the breakdown of each exercise. This detailed approach allows for a deeper understanding of the problem-solving process and aids in mastering the essential techniques required for success.
| Problem | Solution | Explanation |
|---|---|---|
| Problem 1 | Solution A | Step 1: Break down the fractions… Step 2: Simplify… |
| Problem 2 | Solution B | Step 1: Identify the unknown… Step 2: Apply operations… |
| Problem 3 | Solution C | Step 1: Set up the equation… Step 2: Solve for the variable… |
By carefully following these solutions, students can reinforce their learning and gain confidence in solving complex problems. This guide ensures that every step is clearly outlined, allowing learners to master the material effectively.
Overview of Fourth-Level Learning Concepts
The fourth level of elementary education introduces students to a broad range of mathematical concepts designed to enhance their problem-solving and reasoning skills. This stage focuses on building a strong foundation in both basic and advanced topics, preparing students for future challenges. Through a variety of exercises and lessons, learners gain essential knowledge and techniques that will support their academic growth.
In this stage, students engage with a diverse set of concepts, including:
- Understanding and manipulating numbers in different forms
- Applying strategies to solve complex problems
- Working with fractions, decimals, and whole numbers
- Developing logical reasoning and critical thinking
As they progress through these topics, students are encouraged to actively participate in their learning and explore various methods to arrive at solutions. Teachers and parents play a vital role in guiding students through these exercises, ensuring they develop a thorough understanding of each concept.
The lessons in this level are designed to foster confidence and competency, laying the groundwork for more advanced topics in future stages. With continued practice and support, learners are well-equipped to tackle more complex material as they move forward in their educational journey.
Key Concepts in Module 5
This section focuses on the essential ideas and methods that students will encounter as they progress through the fifth unit of their learning. The goal is to ensure that learners develop a solid understanding of the fundamental concepts needed to solve more complex problems. Each key idea is built upon the previous one, allowing students to develop their skills in a structured way.
Among the core topics in this section, students will explore:
- Understanding fractions and their relationships
- Adding and subtracting fractions with unlike denominators
- Multiplying fractions and whole numbers
- Solving word problems that involve fractions
The following table outlines the primary concepts covered, helping to visualize their application and importance:
| Concept | Explanation | Application |
|---|---|---|
| Fractions | Understanding how parts of a whole are represented | Used in real-world problems like dividing resources or portions |
| Adding Fractions | Combining fractions with unlike denominators | Applied in measuring and combining different quantities |
| Multiplying Fractions | Multiplying fractions with whole numbers | Used in situations like scaling recipes or area calculations |
| Word Problems | Applying concepts to real-life situations | Critical for developing reasoning and problem-solving skills |
By mastering these core concepts, students will be able to solve more complex problems and apply their knowledge to real-world scenarios with confidence.
How to Use the Answer Guide
Using a solution guide effectively is essential for reinforcing the concepts learned during lessons and helping students gain a deeper understanding of the material. The guide not only provides the correct solutions but also offers a structured way to approach and solve problems step by step. By following this approach, students can identify where they may have made mistakes and understand the reasoning behind each step.
Step-by-Step Review
Each exercise should be reviewed one at a time, comparing your solution with the provided guide. Focus on the following:
- Method: Ensure you used the correct process to arrive at your solution.
- Steps: Compare each individual step to confirm you followed the right sequence of actions.
- Errors: Identify and correct any mistakes in your approach or calculations.
Practical Tips for Effective Use
For optimal results, follow these practical strategies:
- Start by attempting the problem independently before consulting the guide.
- Use the guide as a learning tool to understand the reasoning behind each solution.
- If necessary, revisit earlier steps to clarify any misunderstandings.
- Practice similar problems to reinforce your understanding and improve problem-solving skills.
By actively engaging with the guide, students not only improve their understanding of the current topic but also build a stronger foundation for future lessons.
Lesson Breakdown for Module 5
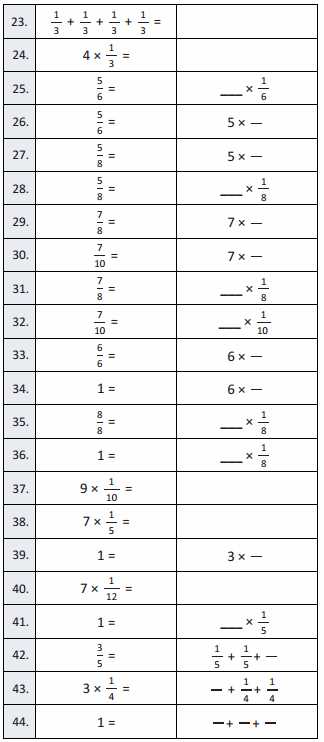
This section provides a detailed breakdown of the lessons covered in the fifth unit, focusing on essential concepts and their applications. Each lesson is designed to build on the previous one, reinforcing knowledge and developing skills that students will need to tackle more complex problems. The goal is to ensure a thorough understanding of each topic, step by step, so that learners can progress confidently.
Lesson 1: Introduction to Fractions
The first lesson introduces students to the basic idea of fractions, helping them understand how parts make up a whole. Key topics include:
- Identifying fractions and their parts
- Understanding numerator and denominator
- Comparing simple fractions
Lesson 2: Adding and Subtracting Fractions
In this lesson, students learn how to add and subtract fractions, with a focus on the following skills:
- Finding common denominators
- Adding and subtracting fractions with like denominators
- Solving word problems involving addition and subtraction of fractions
Lesson 3: Multiplying Fractions
The third lesson covers multiplication of fractions, including:
- Multiplying fractions by whole numbers
- Visualizing multiplication with models
- Real-life applications of fraction multiplication
Lesson 4: Solving Word Problems
This lesson emphasizes applying learned concepts to solve word problems. Topics covered include:
- Breaking down word problems step by step
- Identifying key information in word problems
- Using fractions in real-world contexts
Lesson 5: Review and Practice
The final lesson focuses on reviewing all the concepts covered in previous lessons and practicing the skills learned. Students will:
- Complete a series of practice exercises
- Reinforce concepts through interactive activities
- Prepare for assessments on fraction concepts
By the end of the unit, students will have a solid understanding of fractions, addition, subtraction, and multiplication of fractions, as well as the ability to apply these skills in various problem-solving scenarios.
Common Challenges in Module 5
As students progress through this section of their learning, they may encounter several challenges that can hinder their understanding of key concepts. These obstacles are common and can often be overcome with focused practice and the right strategies. Understanding these challenges is the first step in overcoming them and achieving success in the unit.
Understanding Fractions and Their Relationships
One of the most common difficulties faced by students is grasping the concept of fractions, particularly when it comes to comparing and manipulating them. Key struggles include:
- Identifying and understanding numerators and denominators
- Comparing fractions with different denominators
- Visualizing fractions and their equivalent forms
Adding and Subtracting Fractions with Unlike Denominators
Another significant challenge is adding and subtracting fractions that have different denominators. Common issues include:
- Finding a common denominator
- Misunderstanding how to adjust fractions before performing operations
- Struggling with the concept of simplifying fractions after adding or subtracting
These challenges are normal, and with consistent practice and support, students can overcome them and strengthen their understanding of key concepts. Utilizing visual aids, practice exercises, and step-by-step explanations can be very helpful in addressing these difficulties.
Understanding Fractions in Module 5
In this section, students explore the fundamental concept of fractions, learning to identify, compare, and manipulate parts of a whole. A solid understanding of fractions is essential for solving more complex problems in later stages of their learning. This topic covers the relationship between numerators and denominators, as well as the various ways fractions can be expressed and compared.
Key Concepts in Fractions
The following concepts are central to understanding fractions:
- Parts of a Whole: A fraction represents a part of a whole, where the numerator indicates the number of parts and the denominator represents the total number of equal parts.
- Equivalent Fractions: Fractions that represent the same value, even if they appear different. For example, 1/2 is equivalent to 2/4.
- Comparing Fractions: Fractions can be compared by examining their numerators and denominators, or by finding a common denominator.
Applying Fractions in Real-Life Situations
Fractions are not just abstract concepts; they are used in everyday life. Some examples of how fractions are applied include:
- Dividing food or other items into equal parts, such as slicing a pizza or pie.
- Measuring ingredients for recipes or construction projects.
- Sharing resources fairly, such as dividing time or materials among a group.
Through consistent practice and real-life applications, students can develop a stronger understanding of fractions and their role in both academic and everyday situations.
Strategies for Solving Word Problems
Word problems require students to apply their understanding of concepts to real-world situations. These problems often involve multiple steps and can be challenging, especially when the information is presented in a narrative format. To solve them effectively, students must develop strategies to break down the problem, organize the given information, and use the appropriate mathematical operations.
Steps for Solving Word Problems
Following a clear and systematic approach can help simplify word problems. Here are the key steps:
- Read the Problem Carefully: Understand what is being asked and highlight the important details.
- Identify the Information: Look for numbers, units, and any relationships mentioned in the problem.
- Choose the Right Operation: Decide if you need to add, subtract, multiply, or divide based on the context.
- Set Up the Equation: Translate the words into a mathematical expression or equation.
- Solve the Problem: Perform the necessary calculations to find the solution.
- Check Your Work: Verify the result by checking if it makes sense in the context of the problem.
Example Word Problem
Here is a simple example of how these steps can be applied to a word problem:
| Step | Explanation |
|---|---|
| Read the Problem | “John has 5 apples. He buys 3 more apples. How many apples does he have now?” |
| Identify Information | John has 5 apples, and he buys 3 more apples. |
| Choose the Right Operation | Since the problem involves finding the total number of apples, we need to add. |
| Set Up the Equation | 5 + 3 = ? |
| Solve the Problem | 5 + 3 = 8 |
| Check Your Work | John has 8 apples, which matches the context of the problem. |
By following these strategies, students can approach word problems with confidence, ensuring they understand the key information and select the correct approach for solving them.
Tips for Parents and Teachers
Supporting students as they navigate through their lessons is crucial for their success. Both parents and teachers play an important role in reinforcing key concepts, providing encouragement, and ensuring that students feel confident in their abilities. By working together and applying effective strategies, they can help children overcome challenges and achieve a deeper understanding of the material.
Effective Strategies for Parents
Parents can make a significant impact on their child’s learning by fostering a positive and supportive environment. Here are some tips for parents:
- Encourage Regular Practice: Consistent practice reinforces learning and builds confidence. Set aside time each day for students to work on their tasks.
- Be Patient and Supportive: Recognize that learning can take time. Offer help when needed, but allow the child to work through problems independently as well.
- Use Real-Life Examples: Help children connect concepts to everyday situations. This can make abstract ideas more relatable and easier to understand.
Tips for Teachers in the Classroom
Teachers can implement a variety of methods to make lessons more engaging and effective. Here are some strategies that can be used in the classroom:
- Break Down Complex Concepts: Use simple language and visual aids to explain challenging ideas. Breaking down problems into smaller steps can help students grasp the material more easily.
- Incorporate Group Activities: Collaborative work allows students to learn from one another. Group discussions and peer tutoring can improve comprehension.
- Provide Positive Reinforcement: Praise students for their efforts and progress. Positive feedback motivates students to keep working hard.
Collaborating for Success
By maintaining open communication between parents and teachers, both parties can ensure that students receive the support they need. Regular updates on progress and specific areas of challenge allow for targeted help and greater success. With a unified approach, students are more likely to achieve their full potential.
Activities to Reinforce Learning
Engaging activities can help solidify the concepts students learn in the classroom, making abstract ideas more tangible and memorable. These activities encourage active participation, reinforce skills, and create a deeper understanding of the material. By incorporating various types of exercises into everyday routines, both at home and in the classroom, students can strengthen their knowledge and boost their confidence.
Hands-On Learning Activities
Interactive exercises allow students to apply what they have learned in a practical context. Here are some effective hands-on activities:
- Building Models: Encourage students to create physical representations of problems or concepts, such as drawing fractions on paper plates or using blocks for geometry.
- Real-Life Application: Involve students in everyday tasks, such as measuring ingredients for a recipe or calculating the total cost of groceries, to connect classroom concepts with the real world.
- Games and Puzzles: Use board games or online quizzes that challenge students to solve problems in a fun and competitive way.
Creative Practice Exercises
For students who thrive on repetition or need additional practice, creative exercises can help reinforce learning:
- Flashcards: Use flashcards to practice facts, such as multiplication or division, in a quick and efficient manner. Flashcards can be used alone or in pairs for a more interactive experience.
- Story Problems: Create custom word problems based on students’ interests, encouraging them to think critically and apply their knowledge in new situations.
- Worksheets with Variety: Provide worksheets that mix multiple concepts, allowing students to practice solving different types of problems in one exercise.
By incorporating these activities into the learning process, students can strengthen their understanding, develop problem-solving skills, and enjoy a more engaging educational experience.
How to Check Your Answers
Reviewing your work is an essential step in the learning process, as it ensures the solutions you’ve arrived at are correct and well-understood. Checking your results helps reinforce the material and provides opportunities for improvement. By adopting systematic strategies for reviewing, you can build confidence in your problem-solving abilities and avoid common errors.
Step-by-Step Verification
To ensure the correctness of your solutions, follow these steps:
- Revisit the Problem: Read the problem carefully again to confirm you understand what is being asked. Sometimes, a simple misunderstanding can lead to incorrect results.
- Check Your Calculations: Go over your calculations one by one. Double-check any arithmetic steps, ensuring no simple mistakes were made in the process.
- Estimate the Answer: Before fully checking, use estimation to predict a reasonable answer. This can help you spot glaring errors quickly, especially in larger problems.
Using Alternative Methods
Approaching the problem from a different angle can provide an effective check on your work. Here’s how:
- Reverse the Operation: If you’ve solved an addition problem, try subtracting the answer from the original number to see if it matches the expected result. For multiplication or division, perform the inverse operation.
- Use a Different Method: If possible, solve the problem in a different way. For example, if you used a standard algorithm, try using a number line or another strategy to verify your result.
- Cross-Check with a Peer: If available, compare your results with a classmate or teacher. Sometimes a fresh perspective can help identify errors you might have missed.
By following these strategies, you can ensure that your answers are accurate, deepening your understanding and reinforcing your learning in the process.
Exploring Problem-Solving Techniques
Developing strong problem-solving skills is essential for tackling complex challenges. Understanding various techniques can make solving problems more efficient and less daunting. By applying the right approach to different types of questions, students can increase their chances of finding the correct solution. In this section, we will explore several strategies that can help students become more effective problem solvers.
Visualization and Diagrams
One of the most effective strategies is to visualize the problem. This method helps students break down complex problems into smaller, more manageable parts. Here are some ways to implement this technique:
- Drawing Diagrams: Use drawings or sketches to represent numbers, shapes, or relationships in the problem. This makes abstract concepts more concrete.
- Using Number Lines: A number line can be particularly useful for understanding addition, subtraction, or fractions, making the problem easier to understand visually.
- Creating Tables: For problems involving patterns or sequences, tables can help organize the information, making it simpler to find a solution.
Working Backwards
Sometimes, starting from the solution and working backwards can help students better understand the problem and identify the right steps. This technique is useful when the problem involves unknown variables. Steps include:
- Identify the Desired Outcome: Begin with the end goal and think about the steps needed to get there.
- Reverse the Process: Work backwards through the operations or logic used in the problem to determine the original values or relationships.
- Check for Consistency: As you reverse the steps, make sure each one makes sense and leads logically to the solution.
Trial and Error
Trial and error is another useful technique for solving problems, especially when there is no clear formula or method. This involves testing different solutions until the correct one is found. Here’s how to apply it effectively:
- Start with an Estimate: Make an initial guess or estimate that seems reasonable based on the information provided.
- Test the Guess: Apply the guess and check if it fits the conditions of the problem. If not, adjust and try again.
- Refine the Process: Use the feedback from previous attempts to make better guesses or refine your approach until the correct solution is found.
By exploring and applying these various problem-solving techniques, students can approach challenges with more confidence and creativity, increasing their problem-solving capabilities.
Common Mistakes and How to Avoid Them
In problem-solving, making errors is a natural part of the learning process, but recognizing common mistakes can help students avoid them in the future. Understanding why certain errors occur and how to address them is essential for improving accuracy and boosting confidence. In this section, we will discuss some frequent pitfalls and provide strategies to prevent them.
Misunderstanding the Problem
One of the most common mistakes is failing to fully comprehend the question before starting. Often, students focus too quickly on numbers or operations without first understanding the context or the problem’s requirements.
- Tip: Take a moment to carefully read the problem. Break it down by identifying key information and what is being asked. It helps to underline or highlight important parts of the question.
- Tip: Rephrase the problem in your own words to ensure complete understanding before proceeding.
Rushing Through Calculations
Another mistake students often make is rushing through calculations. Whether it’s addition, subtraction, or fractions, speed can sometimes lead to careless errors like incorrect steps or missed operations.
- Tip: Work through each step methodically and double-check your calculations. Slow down to ensure accuracy.
- Tip: Use scratch paper to work out intermediate steps clearly, so nothing is overlooked.
Overlooking Unit Conversions
Unit conversions are frequently missed, especially when dealing with measurements. Failing to convert units correctly can lead to incorrect answers, particularly in word problems involving distance, time, or volume.
- Tip: Always check that you’re working with consistent units before performing calculations. If necessary, convert measurements to the same units.
- Tip: Practice converting between units frequently to reinforce understanding of conversion factors.
Misinterpreting Negative Signs
When dealing with negative numbers or subtraction, students sometimes misinterpret the negative sign, which can lead to incorrect results. This often happens when students confuse subtraction with a negative sign or forget to apply the correct sign in an equation.
- Tip: Pay close attention to negative signs, especially when subtracting or working with equations that involve both positive and negative values.
- Tip: Double-check every step that involves a negative number to ensure that the sign is applied correctly.
Incorrectly Applying Formulas
Using formulas incorrectly is another common mistake, especially when students forget to substitute values correctly or mix up the variables. It’s important to follow each formula step-by-step and be consistent with the variables used.
- Tip: Familiarize yourself with the formula before applying it. Write down each variable and ensure that you substitute the correct values.
- Tip: After completing the formula, double-check that all units and values have been entered correctly.
By being mindful of these common mistakes and applying the suggested strategies, students can significantly improve their accuracy and problem-solving skills.
How Module 5 Prepares for Future Lessons
The concepts introduced in this section lay the foundation for more advanced topics that students will encounter in the future. By mastering the key skills and strategies from this unit, learners are equipped to tackle increasingly complex problems and build on their understanding in subsequent lessons. This section serves as an essential bridge, linking current knowledge with future mathematical concepts.
Building Problem-Solving Skills
Students develop critical thinking and problem-solving abilities that are essential for future learning. The exercises in this section encourage learners to analyze problems from multiple angles and choose the best approach, skills they will continue to use in more challenging situations.
- Enhances logical thinking by encouraging students to break down complex problems into manageable steps.
- Teaches students how to select appropriate strategies, which is crucial for success in more advanced topics.
Mastering Key Mathematical Operations
This section emphasizes the importance of fluency in basic operations, such as addition, subtraction, multiplication, and division, all of which are foundational for more advanced work. Students practice these skills in varied contexts, preparing them for the more intricate computations they will encounter in the future.
- Develops speed and accuracy in performing calculations, which is essential for higher-level problem-solving tasks.
- Provides students with a deeper understanding of operations, allowing them to handle more complex mathematical relationships.
Introducing Advanced Number Concepts
In addition to reinforcing basic skills, this section introduces more advanced number concepts, such as fractions, decimals, and negative numbers. These topics serve as stepping stones to future lessons, where students will apply these concepts in real-world scenarios and more challenging mathematical environments.
- Students are introduced to new concepts in a way that connects to prior knowledge, making it easier to grasp advanced topics later.
- Understanding fractions and decimals helps build a stronger foundation for algebra and geometry.
Strengthening Analytical Thinking
The focus on analyzing problems and looking for patterns helps to develop students’ analytical skills. These skills will be used in future lessons where students will have to interpret data, work with algebraic expressions, and solve more complex equations.
- Encourages students to observe patterns and relationships in numbers, which are key in future mathematical studies.
- Builds reasoning skills that are essential for problem-solving in more complex mathematical and scientific contexts.
By providing a comprehensive and thorough understanding of the fundamentals, this section ensures that students are prepared to tackle future lessons with confidence and clarity. The skills and knowledge gained here are directly applicable to more advanced topics, allowing learners to approach future challenges with a solid foundation.
Additional Resources for Students
To further enhance understanding and mastery of the concepts covered, students can benefit from a variety of supplementary materials. These resources provide extra practice, deeper explanations, and interactive opportunities that complement the lessons and reinforce key skills. Whether students need additional support or are looking to challenge themselves further, these resources can make a significant difference in their learning experience.
Interactive Learning Platforms
Online platforms offer engaging, interactive lessons and exercises that help solidify understanding. These websites often provide immediate feedback, making it easier for students to identify areas for improvement and progress at their own pace.
- Interactive exercises: Websites like ABCmouse and Khan Academy offer interactive practice activities tailored to specific topics.
- Video tutorials: Sites like Teachers Pay Teachers offer videos that explain difficult concepts in a visual, step-by-step manner.
Worksheets and Practice Books
Additional worksheets and practice books are excellent tools for reinforcing the concepts learned in class. These materials often include a variety of problem types that encourage students to apply their knowledge in different ways.
- Printable worksheets: Websites like Education.com offer free downloadable worksheets on various topics, including operations, fractions, and problem-solving.
- Practice books: Books such as Workbook for the Busy Student and Practice Makes Perfect series provide structured exercises to enhance skills.
Parent and Teacher Guides
Guides designed for parents and teachers offer detailed instructions on how to support students’ learning. These guides provide strategies for explaining concepts, as well as tips on how to encourage students and track their progress.
- Instructional guides: Guides available on educational sites like Scholastic offer detailed breakdowns of lesson plans and teaching strategies.
- Home support: Teachers often provide resources or advice on how parents can help their children practice key skills at home.
Educational Apps
Mobile apps designed for learning can provide a fun and convenient way to practice key skills on the go. These apps often feature games, quizzes, and challenges that keep students engaged while reinforcing important concepts.
- Skill-building apps: Apps like Prodigy and Mathway offer interactive challenges to practice specific skills like fractions or word problems.
- Time-management apps: Apps such as Todoist and Focus Booster help students plan and organize their study sessions.
By utilizing these resources, students can gain a deeper understanding of the topics covered and continue to improve their skills both inside and outside of the classroom. With additional practice and support, learners are better equipped to tackle new challenges and achieve success in future lessons.
How to Stay Organized While Learning
Staying organized is essential for successful learning. It allows students to keep track of their progress, efficiently manage their time, and reduce stress. By establishing good habits, students can stay focused and ensure they are fully prepared for each lesson or task. Proper organization also helps prevent confusion and improves overall retention of information.
Creating a Structured Study Plan
One of the best ways to stay on top of learning tasks is by creating a clear study plan. This plan helps students break down their work into manageable sections, ensuring that no part of the subject is overlooked.
- Set clear goals: Identify specific learning objectives for each session. This makes it easier to track progress and stay motivated.
- Prioritize tasks: Tackle the most challenging or time-consuming tasks first, then move on to simpler activities.
- Time management: Allocate time for each subject and take regular breaks to stay refreshed and focused.
Organizing Study Materials
Keeping materials organized is equally important. When all resources are in order, it’s easier to find what you need and stay on track with assignments.
- Use folders or binders: Organize worksheets, notes, and assignments in separate folders or binders for easy access.
- Label everything: Clearly label all materials, notebooks, and documents to avoid confusion.
- Digital organization: For online resources, create specific folders on your computer or cloud storage to keep everything sorted by topic.
By implementing these strategies, students can create an organized environment that promotes effective learning and reduces unnecessary distractions. A well-planned approach helps ensure that learning goals are met with greater efficiency and less stress.
Improving Skills with Module 5
Developing strong problem-solving abilities is a key aspect of academic success. The lessons and activities in this section provide students with the tools they need to enhance their understanding and tackle more complex tasks with confidence. By practicing fundamental concepts and applying them in real-world scenarios, learners can reinforce their abilities and make significant progress.
One of the most effective ways to strengthen skills is through consistent practice. By working through problems that challenge different aspects of the subject, students can build a deeper comprehension. This structured approach also helps identify areas of weakness that can be addressed with focused effort. Repetition of various techniques increases familiarity, making it easier to apply the concepts independently in future lessons.
Another key to improving performance is developing a strong foundation. By mastering foundational concepts, students gain the confidence needed to tackle more advanced topics. Applying strategies such as breaking down problems into smaller, more manageable parts, using visual aids, and reviewing previous lessons can all help build a stronger understanding of the subject matter.
With these strategies in place, students can progressively improve their problem-solving abilities and become more efficient learners. Regular review, hands-on exercises, and thoughtful analysis of mistakes all contribute to long-term growth and academic success.