
Improving your problem-solving abilities in mathematics requires a deep understanding of various concepts and consistent practice. The process of mastering these skills involves recognizing patterns, applying methods, and verifying results. This approach is essential for strengthening mathematical thinking and achieving success in advanced topics.
In this section, we explore the best strategies to evaluate your progress and enhance your knowledge. With the help of detailed solutions and explanations, you can identify areas that need attention and gain confidence in solving complex problems. By reviewing key methods and techniques, you’ll be able to approach challenges with a more analytical mindset and sharpen your overall mathematical skills.
Algebra Nation Answer Key Overview
This section provides an essential guide to help you understand how to check and review your progress. It focuses on evaluating your responses to exercises and uncovering areas where improvements can be made. By referencing detailed solutions, you gain clarity on where you might have gone wrong and how to approach similar problems more effectively in the future.
How to Use This Guide
The solutions provided here break down each problem step by step. This breakdown allows you to follow along and grasp the reasoning behind each answer, which is crucial for building a stronger foundation in mathematics. With a clearer understanding, you’ll be able to tackle more complex problems with confidence.
Key Features of the Overview
| Feature | Description |
|---|---|
| Clear Explanations | Each problem is solved with detailed steps and logical explanations for a better understanding of the methods used. |
| Problem Breakdown | Problems are broken into smaller, manageable parts to ensure easier comprehension and application of strategies. |
| Practice Tips | Guidelines on how to improve your technique and avoid common mistakes are included to help you refine your skills. |
Understanding Section 2 Test Yourself
This part of the material focuses on helping you gauge your knowledge and identify areas that need improvement. It presents a series of challenges that encourage independent thinking and reinforce concepts previously learned. By working through these exercises, you’ll develop the necessary skills to handle more complex problems with ease and confidence.
How to Approach the Exercises
To get the most out of the exercises, it is essential to read each question carefully and apply the strategies you’ve studied. Don’t rush through the process; instead, take your time to analyze each problem and think critically about the methods that will lead to the correct solution. Patience and attention to detail are key to mastering these challenges.
Tracking Your Progress
As you complete the exercises, keep track of your performance. If you find areas where you struggled, make note of them and revisit the related concepts. Consistent review of these weaker areas will help reinforce your understanding and improve your problem-solving techniques over time.
How to Use the Answer Key
The guide provided allows you to evaluate your solutions and compare them with the correct outcomes. It is a helpful tool for identifying mistakes and understanding why a particular approach is correct. Using this resource effectively can significantly improve your problem-solving skills and help you grasp complex concepts with greater clarity.
Step-by-Step Solution Review
As you review each solution, focus on the steps taken to reach the final answer. Don’t just memorize the result–take the time to understand the logic behind each decision. This deeper comprehension will allow you to replicate the process on similar exercises and avoid making the same mistakes in the future. Attention to detail during this review is essential for mastering the material.
Common Mistakes and How to Avoid Them
While reviewing, pay attention to frequent errors that may arise. Whether it’s misinterpreting a problem or skipping a critical step, recognizing these common pitfalls can help you correct them. Identifying patterns in your mistakes will make it easier to avoid repeating them and ensure more accurate results in future exercises.
Improving Your Math Skills
Enhancing your problem-solving abilities requires consistent practice and a focused approach to mastering the key concepts. By building a strong foundation and refining your techniques, you’ll be able to tackle more complex challenges with ease. Here are some effective strategies to help you improve:
- Practice regularly: Set aside time each day to solve problems and reinforce your skills. The more you practice, the more confident you’ll become in your abilities.
- Focus on understanding the process: Rather than memorizing solutions, aim to understand the reasoning behind each step. This will allow you to apply the same techniques to new problems.
- Review your mistakes: Analyze errors to identify where you went wrong. Understanding your mistakes will help prevent them in the future.
- Ask for help when needed: Don’t hesitate to seek guidance from teachers, tutors, or peers if you’re struggling with a particular concept.
Effective Practice Techniques
One of the most important aspects of improving your skills is knowing how to practice effectively. Instead of just completing problems mindlessly, focus on:
- Breaking down complex problems: Split them into smaller, more manageable parts to make them easier to solve.
- Repetition: Regularly revisit previously solved problems to reinforce your understanding and retention of key concepts.
- Challenge yourself: Gradually increase the difficulty of the problems you work on to push your limits and improve your abilities.
By following these strategies and maintaining a disciplined approach, you’ll steadily improve your problem-solving skills and become more proficient in mathematics.
Common Mistakes in Section 2
When working through math exercises, it’s easy to make errors that can impact your overall understanding and performance. Recognizing these common pitfalls is essential for improving accuracy and avoiding recurring mistakes. Below are some frequent errors that students often make in this particular section, along with tips on how to prevent them:
Frequent Errors to Watch For
- Misinterpreting the question: It’s easy to overlook key details in a problem. Always read the question thoroughly before attempting to solve it.
- Skipping steps: Some problems require a series of steps to reach the correct solution. Skipping any of these steps can lead to incorrect answers.
- Rushing through calculations: Speeding through the math can cause careless mistakes, especially with basic operations like addition or multiplication.
- Overlooking negative signs: Negative numbers are often overlooked, leading to incorrect results in calculations.
How to Avoid These Mistakes
- Take your time: Carefully read through the entire problem and ensure you understand what is being asked before starting the solution.
- Work step-by-step: Break down complex problems into smaller, manageable parts. Always follow the proper order of operations.
- Double-check your work: After completing a problem, go back and verify each step to ensure no mistakes were made.
- Practice regularly: The more you practice, the more familiar you will become with common problem types and how to avoid mistakes.
By staying mindful of these common errors and incorporating these strategies into your practice, you will improve your accuracy and understanding in future exercises.
Step-by-Step Solution Breakdown
Breaking down each problem into smaller, manageable steps is crucial for understanding the process and reaching the correct solution. This approach helps you track your progress, identify where things may go wrong, and build a stronger understanding of the techniques involved. Below, we will go through the essential steps to solve typical problems effectively.
| Step | Action | Purpose |
|---|---|---|
| Step 1 | Read the problem carefully | Ensure you understand the question and identify key information needed for the solution. |
| Step 2 | Identify the method to apply | Choose the correct strategy or formula based on the problem’s requirements. |
| Step 3 | Perform calculations | Follow the chosen method and perform the necessary calculations in the correct order. |
| Step 4 | Check intermediate results | Verify each result as you go to ensure accuracy before moving forward. |
| Step 5 | Final verification | Double-check the entire solution to confirm everything is correct and complete. |
By following this structured approach, you can confidently solve problems with precision and clarity. This step-by-step breakdown also helps in reinforcing your understanding of key concepts, making it easier to tackle even more complex challenges in the future.
How the Test Enhances Learning
Engaging with assessments not only measures your progress but also plays a crucial role in reinforcing the material you’ve studied. By reviewing your performance and understanding where you made mistakes, you gain valuable insights into how to improve. This process helps strengthen key concepts, build problem-solving skills, and ultimately enhance your ability to tackle new challenges.
Benefits of Regular Practice
- Identifies Weak Areas: Completing assessments allows you to pinpoint areas where you might be struggling, so you can focus your attention on improving these aspects.
- Improves Retention: The act of recalling information and applying learned concepts reinforces memory and enhances long-term retention.
- Boosts Confidence: Successfully completing exercises helps build self-assurance, making you feel more capable in future tasks.
- Refines Problem-Solving Skills: Continuous practice hones your ability to approach and solve complex problems more effectively.
How Practice Helps Master Concepts
When you challenge yourself with practical exercises, you get a deeper understanding of the methods and techniques involved. The more you practice, the more familiar you become with the various types of problems and the strategies that work best for solving them. Regular engagement with exercises allows you to:
- Recognize Patterns: With each exercise, you begin to identify recurring themes or strategies that simplify problem-solving.
- Apply Techniques More Effectively: As you continue practicing, you refine your approach, improving your ability to apply solutions quickly and accurately.
- Increase Speed and Efficiency: Over time, consistent practice leads to faster and more efficient problem-solving, which is essential for more advanced exercises.
By incorporating regular assessments into your study routine, you create a continuous feedback loop that promotes deeper understanding and mastery of the material.
Reviewing Key Concepts in Mathematics
Mastering essential mathematical concepts is fundamental to succeeding in problem-solving exercises. By revisiting these core ideas, you reinforce your understanding and ensure that you can apply them effectively in a variety of situations. Regular review allows you to stay sharp and tackle more complex problems with confidence.
Core Concepts to Focus On
- Equations and Inequalities: Understanding how to solve and manipulate equations is crucial for solving many types of problems. Practice balancing both sides and applying the correct operations.
- Functions and Graphs: Knowing how to interpret and plot functions helps visualize relationships between variables, which is essential for more advanced topics.
- Factoring and Expanding: Mastering how to factor expressions and expand polynomials is vital for simplifying problems and finding solutions quickly.
- Exponents and Radicals: These concepts are foundational in many algebraic manipulations, and being able to simplify expressions involving powers and roots is essential.
How Reviewing Helps Retain Information
Revisiting these topics regularly enhances retention and deepens your understanding. The more frequently you engage with the material, the easier it becomes to recall when needed. Active review strategies such as:
- Explaining concepts to others: Teaching someone else reinforces your own understanding and helps clarify any confusion.
- Solving practice problems: Working through examples reinforces the steps involved and helps identify areas that may need further clarification.
- Using visual aids: Diagrams, graphs, and charts can help visualize abstract concepts and make them easier to understand.
Consistent review solidifies these key ideas, making it easier to approach even the most complex problems with confidence and clarity.
Strategies for Success in Section 2
Achieving success in mathematical exercises requires more than just understanding the core principles. It’s about applying effective strategies that enhance your performance and help you overcome challenges with confidence. By focusing on specific techniques and approaching problems strategically, you can improve both your accuracy and efficiency.
Key Approaches to Excel
- Break Down the Problem: Start by simplifying the problem into smaller, manageable parts. This makes complex tasks easier to tackle and reduces the likelihood of errors.
- Plan Your Steps: Before diving into calculations, take a moment to outline the steps you’ll need to take. A clear plan of action can help prevent mistakes and keep you on track.
- Work on Time Management: Allocate a specific amount of time for each question. Practicing under time constraints prepares you to work quickly without compromising quality.
- Review Your Work: Always double-check your solutions before finalizing them. This helps catch simple mistakes and ensures your work is as accurate as possible.
Mindset and Focus
Maintaining the right mindset is crucial for success. Here are some techniques to help you stay focused and motivated:
- Stay Positive: Approach each problem with a positive attitude, even if it’s challenging. Confidence plays a key role in solving problems effectively.
- Don’t Rush: While time management is important, rushing through the exercises can lead to mistakes. Take your time to ensure accuracy.
- Seek Help When Needed: If you find yourself stuck, don’t hesitate to ask for help. Whether from a teacher, tutor, or peer, discussing tough problems can provide new insights and strategies.
By following these strategies, you can significantly improve your performance and develop a more effective approach to solving mathematical problems in any given exercise.
What to Do After the Test
Once you have completed an assessment, the work doesn’t stop there. It’s essential to reflect on your performance, analyze your results, and use the feedback to guide your future study efforts. Taking the time to review your mistakes and reinforce your strengths can significantly enhance your learning progress.
Review Your Results
Start by going over your responses and identifying areas where you struggled. This allows you to pinpoint any misunderstandings or concepts that need further attention. Make sure to:
- Analyze Incorrect Answers: Look closely at each mistake to understand why you chose the wrong approach. This reflection helps prevent similar errors in the future.
- Highlight Strengths: Recognize the areas where you performed well. Understanding your strengths boosts confidence and reinforces successful strategies.
- Seek Clarification: If there were questions you couldn’t answer correctly, don’t hesitate to ask for help or find additional resources to clarify these concepts.
Plan for Improvement
Once you’ve identified areas for growth, it’s time to create a plan to address these weaknesses. Consider the following strategies:
- Set Specific Goals: Focus on one concept or skill at a time. Set achievable goals that will help you gradually improve over time.
- Practice Regularly: The more you practice, the more confident you will become. Repetition solidifies understanding and improves your problem-solving skills.
- Utilize Additional Resources: If necessary, find supplementary materials such as guides, practice problems, or tutorials to help reinforce your learning.
By reflecting on your performance and planning your next steps, you ensure continuous improvement and stay on track for achieving greater mastery in the subject.
Common Topics Covered
In many mathematical exercises, there are certain topics that consistently appear due to their foundational importance. Mastering these concepts is key to building a strong understanding of the subject. These core areas help develop problem-solving skills and provide a solid framework for tackling more advanced material.
Key Concepts to Focus On
- Linear Equations: Understanding how to solve equations with one or more variables is fundamental. This includes both simple and complex equations with various operations.
- Quadratic Equations: Recognizing the structure of quadratic equations and knowing how to solve them, either by factoring, completing the square, or using the quadratic formula, is essential.
- Functions and Graphs: Learning how different types of functions behave and how to represent them visually is crucial for understanding relationships between variables.
- Systems of Equations: Being able to solve systems, whether linear or nonlinear, is a valuable skill that is frequently tested in exercises.
- Inequalities: Solving inequalities and understanding their graphical representations is another important area that often appears in practice problems.
Advanced Topics
- Polynomials: Working with polynomials, including operations like addition, subtraction, multiplication, and factoring, is a common challenge in more advanced topics.
- Rational Expressions: Simplifying and solving problems with rational expressions, which involve fractions with polynomials in the numerator and denominator, is a key area of study.
- Exponents and Radicals: Manipulating exponents, including laws of exponents and simplifying expressions involving square roots or higher roots, is an essential skill.
Focusing on these core topics allows you to build a strong foundation, making it easier to approach more complex problems with confidence. Understanding these key concepts is not only important for solving individual problems, but also for improving your overall mathematical thinking.
How to Track Your Progress
Monitoring your growth and improvement is an essential part of the learning process. By tracking your progress, you can identify areas where you are excelling and areas that need more attention. This allows you to focus your efforts on improving specific skills while reinforcing what you’ve already mastered.
One effective way to track progress is to regularly assess your understanding of the material. Take note of any patterns in the mistakes you make, as this can help you understand the areas where further practice or review is necessary. Additionally, you can measure your success by comparing your performance over time, noting improvements as you tackle increasingly difficult problems.
Another helpful strategy is to keep a learning journal or log. Document each study session by noting the topics you covered, any challenges you faced, and the methods you used to overcome them. This will help you reflect on your learning process and identify strategies that work best for you.
Finally, setting short- and long-term goals can provide a clear roadmap for your progress. Regularly review these goals and adjust them as needed to ensure continuous improvement. With persistence and self-reflection, you’ll be able to see how far you’ve come and stay motivated to keep advancing.
Tips for Efficient Practice
Practicing efficiently is key to improving your skills in any subject. By using the right strategies, you can maximize your study time and make steady progress. Instead of simply going through exercises, focus on methods that enhance retention, build confidence, and reinforce key concepts.
Effective Strategies for Focused Practice
- Break Down Complex Problems: Start by breaking down complicated problems into smaller, manageable steps. This makes it easier to understand the solution and reduces overwhelm.
- Practice Regularly: Consistency is important. Set aside a regular time each day or week to practice, ensuring that you reinforce what you’ve learned.
- Prioritize Weak Areas: Focus more on the topics or problem types that you find difficult. This ensures you’re improving in areas where you need the most help.
- Use Active Recall: Instead of passively reviewing material, try recalling it from memory. This strengthens your ability to retain and apply what you’ve learned.
- Work with Timed Sessions: Simulate real-world conditions by setting a timer for practice sessions. This helps improve time management and builds your ability to solve problems under pressure.
Incorporating Feedback for Growth
- Review Mistakes: After completing an exercise, thoroughly review the mistakes you made. Understand why they occurred and how to avoid them in the future.
- Seek External Resources: If you’re stuck, look for explanations or additional examples online or in textbooks. Sometimes a different perspective can help clarify a concept.
- Get Feedback from Others: Discuss your approach with a tutor, study partner, or teacher. External feedback can provide new insights and strategies for improvement.
By using these methods, you can optimize your practice sessions, making them more productive and less stressful. With focused, efficient effort, you’ll see continual improvement in your abilities and gain more confidence in solving problems.
How to Avoid Test Anxiety
Feeling nervous before assessments is common, but managing those nerves is key to performing at your best. Test anxiety can cause stress, hinder concentration, and make it harder to recall what you’ve studied. By adopting certain strategies, you can minimize these feelings and approach each challenge with confidence and clarity.
Preparation Techniques to Calm Your Nerves
- Start Early: Begin reviewing material well in advance to avoid cramming. Spacing out your study sessions helps to reduce stress and enhances long-term retention.
- Create a Study Plan: Organize your study time effectively. Break down tasks into smaller, manageable sections and stick to a set schedule to avoid last-minute pressure.
- Practice Relaxation Exercises: Deep breathing, meditation, and progressive muscle relaxation are excellent ways to calm your body and mind. Practice these techniques regularly to help reduce anxiety.
- Get Enough Rest: Never underestimate the power of sleep. Restful sleep boosts memory retention and concentration, helping you feel more confident on the day of the assessment.
Mindset Shifts for Reducing Stress
- Reframe Your Thoughts: Instead of viewing the assessment as a source of fear, try to see it as an opportunity to showcase your knowledge. Embrace challenges as a natural part of the learning process.
- Focus on the Present: Don’t dwell on past mistakes or worry about future outcomes. Concentrate on what you can control right now–your preparation and performance in the moment.
- Practice Positive Self-Talk: Remind yourself of your strengths and abilities. Replace negative thoughts like “I can’t do this” with empowering affirmations like “I am prepared and capable.”
By following these strategies, you can significantly reduce feelings of anxiety and approach any challenge with a clearer mind. Remember, confidence and calmness come from consistent preparation, a positive mindset, and learning to manage stress effectively.
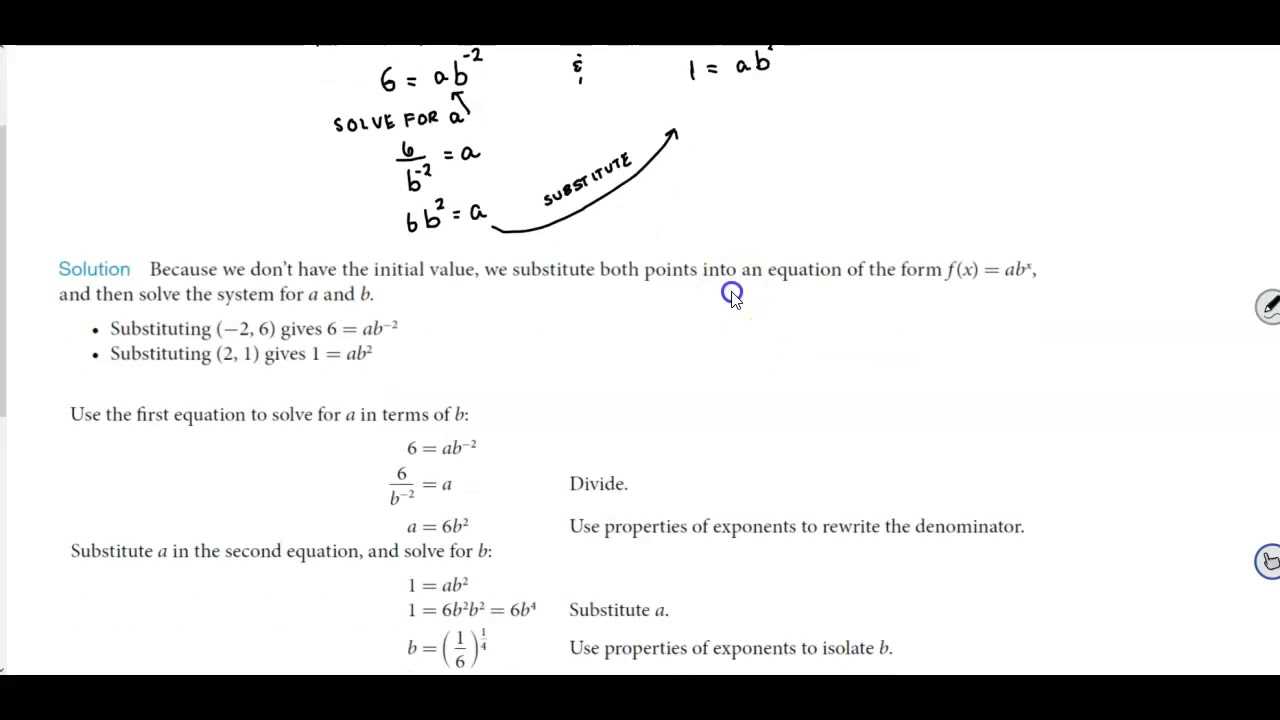
Understanding Algebraic Equations
Mathematical equations are fundamental tools used to express relationships between numbers or variables. They provide a way to represent unknown values and solve problems systematically. At the core of solving these equations is the process of finding the value of the unknown that satisfies the equation’s conditions. Understanding the structure and logic behind these expressions is crucial for progressing in various areas of mathematics.
Equations typically consist of two sides separated by an equal sign. Each side of the equation contains terms that may include numbers, variables, and mathematical operations. The goal is to manipulate the equation in such a way that the variable is isolated on one side, revealing its value. By following specific rules and operations, you can solve these expressions and uncover unknown values.
Common types of equations include linear equations, where the highest power of the variable is one, and quadratic equations, which involve terms with the variable raised to the second power. As you learn to manipulate these expressions, you’ll develop strategies for solving them more efficiently, enhancing your problem-solving skills in mathematics.
Preparing for Algebra Tests Effectively
Preparing for assessments in mathematics requires a structured approach that focuses on understanding key concepts and practicing problem-solving techniques. By organizing your study time and actively engaging with the material, you can build confidence and improve your ability to tackle complex problems. Effective preparation isn’t just about memorization; it’s about developing a deep understanding of the subject matter and honing your skills through consistent practice.
One of the most effective ways to prepare is to review and practice the types of problems that are commonly featured in exams. This will help you familiarize yourself with the format and identify any areas where you may need additional review. Setting a study schedule, breaking down large topics into smaller, manageable chunks, and regularly testing your knowledge will ensure that you’re fully prepared for the upcoming evaluation.
Key Strategies for Efficient Preparation
- Consistent Practice: Practice problems regularly to reinforce your understanding of key concepts.
- Review Mistakes: Carefully review your errors and understand where you went wrong to avoid repeating them.
- Use Study Guides: Refer to study guides or textbooks to reinforce your knowledge and fill in gaps in understanding.
- Seek Help: Don’t hesitate to ask for clarification from teachers or tutors if you’re struggling with specific topics.
Study Plan Template

| Study Task | Time Allocation | Notes |
|---|---|---|
| Review key concepts | 30 minutes | Focus on the most challenging topics |
| Practice problems | 1 hour | Work on problems from past assessments |
| Test yourself | 30 minutes | Simulate the actual test environment |
| Review errors | 30 minutes | Understand and correct mistakes |
By implementing these strategies and following a structured study plan, you can maximize your preparation efforts and perform confidently in your assessments. Remember, the key to success is consistency and practice.
Further Resources for Mastery
To fully grasp complex mathematical concepts, it’s important to seek out additional materials that complement your learning journey. While class materials and standard textbooks are essential, external resources can provide diverse perspectives, varied exercises, and deeper explanations that enrich your understanding. Exploring these options will not only solidify your foundation but also introduce you to new techniques that can enhance your problem-solving abilities.
Whether you prefer digital tools, reference books, or interactive learning platforms, there is a wealth of resources available. These can guide you through difficult topics, offer practice opportunities, and provide explanations tailored to different learning styles. Incorporating these into your study routine can make a significant difference in your overall performance and mastery of the subject.
Recommended Online Resources
- Khan Academy: A free, comprehensive online platform offering video lessons, practice exercises, and personalized learning dashboards to track progress.
- Wolfram Alpha: A computational engine that can help with solving problems and showing step-by-step solutions, perfect for checking your work.
- Desmos: A graphing tool that lets you visualize mathematical functions and equations, making complex concepts easier to understand.
Books for In-Depth Learning

- “Introduction to Mathematical Thinking” by Keith Devlin: This book provides a deep dive into mathematical reasoning, ideal for those looking to strengthen their logic and critical thinking skills.
- “The Art of Problem Solving” series by Richard Rusczyk: A series designed for students aiming for a deeper understanding of math, including advanced topics and challenging exercises.
- “Precalculus: Mathematics for Calculus” by James Stewart: A popular textbook that thoroughly covers foundational mathematical principles, providing clear explanations and practical exercises.
By utilizing these resources, you can gain additional insight, practice new skills, and explore concepts from different angles. Building a strong grasp of mathematical concepts requires consistent effort, and these tools can support your growth along the way.